Let
d
∈
N
{\displaystyle d\in \mathbb {N} }
B
⊆
R
d
{\displaystyle B\subseteq \mathbb {R} ^{d}}
partial differential equation on
B
{\displaystyle B}
looks like this:
∀
(
x
1
,
…
,
x
d
)
∈
B
:
h
(
x
1
,
…
,
x
d
,
u
(
x
1
,
…
,
x
d
)
,
∂
x
1
u
(
x
1
,
…
,
x
d
)
,
…
,
∂
x
d
u
(
x
1
,
…
,
x
d
)
,
∂
x
1
2
u
(
x
1
,
…
,
x
d
)
,
…
⏞
arbitrary and arbitrarily finitely many partial derivatives,
n
inputs of
h
in total
)
=
0
{\displaystyle \forall (x_{1},\ldots ,x_{d})\in B:h(x_{1},\ldots ,x_{d},u(x_{1},\ldots ,x_{d}),\overbrace {\partial _{x_{1}}u(x_{1},\ldots ,x_{d}),\ldots ,\partial _{x_{d}}u(x_{1},\ldots ,x_{d}),\partial _{x_{1}}^{2}u(x_{1},\ldots ,x_{d}),\ldots } ^{{\text{arbitrary and arbitrarily finitely many partial derivatives, }}n{\text{ inputs of }}h{\text{ in total}}})=0}
h
{\displaystyle h}
R
n
{\displaystyle \mathbb {R} ^{n}}
R
{\displaystyle \mathbb {R} }
n
∈
N
{\displaystyle n\in \mathbb {N} }
B
{\displaystyle B}
u
:
B
→
R
{\displaystyle u:B\to \mathbb {R} }
In the whole theory of partial differential equations, multiindices are extremely important. Only with their help we are able to write down certain formulas a lot briefer.
Definitions 1.1 :
A
d
{\displaystyle d}
multiindex is a vector
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
N
0
{\displaystyle \mathbb {N} _{0}}
If
α
=
(
α
1
,
…
,
α
d
)
{\displaystyle \alpha =(\alpha _{1},\ldots ,\alpha _{d})}
absolute value
|
α
|
{\displaystyle |\alpha |}
|
α
|
:=
∑
k
=
1
d
α
k
{\displaystyle |\alpha |:=\sum _{k=1}^{d}\alpha _{k}}
If
α
{\displaystyle \alpha }
d
{\displaystyle d}
B
⊆
R
d
{\displaystyle B\subseteq \mathbb {R} ^{d}}
u
:
B
→
R
{\displaystyle u:B\to \mathbb {R} }
∂
α
u
{\displaystyle \partial _{\alpha }u}
α
{\displaystyle \alpha }
u
{\displaystyle u}
∂
α
u
:=
∂
x
1
α
1
⋯
∂
x
d
α
d
u
{\displaystyle \partial _{\alpha }u:=\partial _{x_{1}}^{\alpha _{1}}\cdots \partial _{x_{d}}^{\alpha _{d}}u}
We classify partial differential equations into several types, because for partial differential equations of one type we will need different solution techniques as for differential equations of other types. We classify them into linear and nonlinear equations, and into equations of different orders.
Definition 1.3 :
Let
n
∈
N
{\displaystyle n\in \mathbb {N} }
n
{\displaystyle n}
n
{\displaystyle n}
∀
(
x
1
,
…
,
x
d
)
∈
B
⊆
R
d
:
h
(
x
1
,
…
,
x
d
,
u
(
x
1
,
…
,
x
d
)
,
∂
x
1
u
(
x
1
,
…
,
x
d
)
,
…
,
∂
x
d
u
(
x
1
,
…
,
x
d
)
,
∂
x
1
2
u
(
x
1
,
…
,
x
d
)
,
…
⏞
partial derivatives at most up to order
n
)
=
0
{\displaystyle \forall (x_{1},\ldots ,x_{d})\in B\subseteq \mathbb {R} ^{d}:h(x_{1},\ldots ,x_{d},u(x_{1},\ldots ,x_{d}),\overbrace {\partial _{x_{1}}u(x_{1},\ldots ,x_{d}),\ldots ,\partial _{x_{d}}u(x_{1},\ldots ,x_{d}),\partial _{x_{1}}^{2}u(x_{1},\ldots ,x_{d}),\ldots } ^{{\text{partial derivatives at most up to order }}n})=0}
Now we are very curious what practical examples of partial differential equations look like after all.
Theorem and definition 1.4 :
If
g
:
R
→
R
{\displaystyle g:\mathbb {R} \to \mathbb {R} }
c
∈
R
{\displaystyle c\in \mathbb {R} }
u
:
R
2
→
R
,
u
(
t
,
x
)
:=
g
(
x
+
c
t
)
{\displaystyle u:\mathbb {R} ^{2}\to \mathbb {R} ,u(t,x):=g(x+ct)}
solves the one-dimensional homogenous transport equation
∀
(
t
,
x
)
∈
R
2
:
∂
t
u
(
t
,
x
)
−
c
∂
x
u
(
t
,
x
)
=
0
{\displaystyle \forall (t,x)\in \mathbb {R} ^{2}:\partial _{t}u(t,x)-c\partial _{x}u(t,x)=0}
Proof : Exercise 2.
We therefore see that the one-dimensional transport equation has many different solutions; one for each continuously differentiable function in existence. However, if we require the solution to have a specific initial state, the solution becomes unique.
Theorem and definition 1.5 :
If
g
:
R
→
R
{\displaystyle g:\mathbb {R} \to \mathbb {R} }
c
∈
R
{\displaystyle c\in \mathbb {R} }
u
:
R
2
→
R
,
u
(
t
,
x
)
:=
g
(
x
+
c
t
)
{\displaystyle u:\mathbb {R} ^{2}\to \mathbb {R} ,u(t,x):=g(x+ct)}
is the unique solution to the initial value problem for the one-dimensional homogenous transport equation
{
∀
(
t
,
x
)
∈
R
2
:
∂
t
u
(
t
,
x
)
−
c
∂
x
u
(
t
,
x
)
=
0
∀
x
∈
R
:
u
(
0
,
x
)
=
g
(
x
)
{\displaystyle {\begin{cases}\forall (t,x)\in \mathbb {R} ^{2}:&\partial _{t}u(t,x)-c\partial _{x}u(t,x)=0\\\forall x\in \mathbb {R} :&u(0,x)=g(x)\end{cases}}}
Proof :
Surely
∀
x
∈
R
:
u
(
0
,
x
)
=
g
(
x
+
c
⋅
0
)
=
g
(
x
)
{\displaystyle \forall x\in \mathbb {R} :u(0,x)=g(x+c\cdot 0)=g(x)}
∀
(
t
,
x
)
∈
R
2
:
∂
t
u
(
t
,
x
)
−
c
∂
x
u
(
t
,
x
)
=
0
{\displaystyle \forall (t,x)\in \mathbb {R} ^{2}:\partial _{t}u(t,x)-c\partial _{x}u(t,x)=0}
Now suppose we have an arbitrary other solution to the initial value problem. Let's name it
v
{\displaystyle v}
(
t
,
x
)
∈
R
2
{\displaystyle (t,x)\in \mathbb {R} ^{2}}
μ
(
t
,
x
)
(
ξ
)
:=
v
(
t
−
ξ
,
x
+
c
ξ
)
{\displaystyle \mu _{(t,x)}(\xi ):=v(t-\xi ,x+c\xi )}
is constant:
d
d
ξ
v
(
t
−
ξ
,
x
+
c
ξ
)
=
(
∂
t
v
(
t
−
ξ
,
x
+
c
ξ
)
∂
x
v
(
t
−
ξ
,
x
+
c
ξ
)
)
(
−
1
c
)
=
−
∂
t
v
(
t
−
ξ
,
x
+
c
ξ
)
+
c
∂
x
v
(
t
−
ξ
,
x
+
c
ξ
)
=
0
{\displaystyle {\frac {d}{d\xi }}v(t-\xi ,x+c\xi )={\begin{pmatrix}\partial _{t}v(t-\xi ,x+c\xi )&\partial _{x}v(t-\xi ,x+c\xi )\end{pmatrix}}{\begin{pmatrix}-1\\c\end{pmatrix}}=-\partial _{t}v(t-\xi ,x+c\xi )+c\partial _{x}v(t-\xi ,x+c\xi )=0}
Therefore, in particular
∀
(
t
,
x
)
∈
R
2
:
μ
(
t
,
x
)
(
0
)
=
μ
(
t
,
x
)
(
t
)
{\displaystyle \forall (t,x)\in \mathbb {R} ^{2}:\mu _{(t,x)}(0)=\mu _{(t,x)}(t)}
, which means, inserting the definition of
μ
(
t
,
x
)
{\displaystyle \mu _{(t,x)}}
∀
(
t
,
x
)
∈
R
2
:
v
(
t
,
x
)
=
v
(
0
,
x
+
c
t
)
=
initial condition
g
(
x
+
c
t
)
{\displaystyle \forall (t,x)\in \mathbb {R} ^{2}:v(t,x)=v(0,x+ct){\overset {\text{initial condition}}{=}}g(x+ct)}
, which shows that
u
=
v
{\displaystyle u=v}
v
{\displaystyle v}
◻
{\displaystyle \Box }
In the next chapter, we will consider the non-homogenous arbitrary-dimensional transport equation.
Have a look at the definition of an ordinary differential equation (see for example the Wikipedia page on that ) and show that every ordinary differential equation is a partial differential equation.
Prove Theorem 1.4 using direct calculation.
What is the order of the transport equation?
Find a function
u
:
R
2
→
R
{\displaystyle u:\mathbb {R} ^{2}\to \mathbb {R} }
∂
t
u
−
2
∂
x
u
=
0
{\displaystyle \partial _{t}u-2\partial _{x}u=0}
∀
x
∈
R
:
u
(
0
,
x
)
=
x
3
{\displaystyle \forall x\in \mathbb {R} :u(0,x)=x^{3}}
In the first chapter, we had already seen the one-dimensional transport equation. In this chapter we will see that we can quite easily generalise the solution method and the uniqueness proof we used there to multiple dimensions. Let
d
∈
N
{\displaystyle d\in \mathbb {N} }
inhomogenous
d
{\displaystyle d}
looks like this:
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
u
(
t
,
x
)
−
v
⋅
∇
x
u
(
t
,
x
)
=
f
(
t
,
x
)
{\displaystyle \forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:\partial _{t}u(t,x)-\mathbf {v} \cdot \nabla _{x}u(t,x)=f(t,x)}
, where
f
:
R
×
R
d
→
R
{\displaystyle f:\mathbb {R} \times \mathbb {R} ^{d}\to \mathbb {R} }
v
∈
R
d
{\displaystyle \mathbf {v} \in \mathbb {R} ^{d}}
The following definition will become a useful shorthand notation in many occasions. Since we can use it right from the beginning of this chapter, we start with it.
Before we prove a solution formula for the transport equation, we need a theorem from analysis which will play a crucial role in the proof of the solution formula.
Theorem 2.2 : (Leibniz' integral rule)
Let
O
⊆
R
{\displaystyle O\subseteq \mathbb {R} }
B
⊆
R
d
{\displaystyle B\subseteq \mathbb {R} ^{d}}
d
∈
N
{\displaystyle d\in \mathbb {N} }
f
∈
C
1
(
O
×
B
)
{\displaystyle f\in {\mathcal {C}}^{1}(O\times B)}
for all
x
∈
O
{\displaystyle x\in O}
∫
B
|
f
(
x
,
y
)
|
d
y
<
∞
{\displaystyle \int _{B}|f(x,y)|dy<\infty }
for all
x
∈
O
{\displaystyle x\in O}
y
∈
B
{\displaystyle y\in B}
d
d
x
f
(
x
,
y
)
{\displaystyle {\frac {d}{dx}}f(x,y)}
there is a function
g
:
B
→
R
{\displaystyle g:B\to \mathbb {R} }
∀
(
x
,
y
)
∈
O
×
B
:
|
∂
x
f
(
x
,
y
)
|
≤
|
g
(
y
)
|
and
∫
B
|
g
(
y
)
|
d
y
<
∞
{\displaystyle \forall (x,y)\in O\times B:|\partial _{x}f(x,y)|\leq |g(y)|{\text{ and }}\int _{B}|g(y)|dy<\infty }
hold, then
d
d
x
∫
B
f
(
x
,
y
)
d
y
=
∫
B
d
d
x
f
(
x
,
y
)
{\displaystyle {\frac {d}{dx}}\int _{B}f(x,y)dy=\int _{B}{\frac {d}{dx}}f(x,y)}
We will omit the proof.
Theorem 2.3 :
If
f
∈
C
1
(
R
×
R
d
)
{\displaystyle f\in {\mathcal {C}}^{1}(\mathbb {R} \times \mathbb {R} ^{d})}
g
∈
C
1
(
R
d
)
{\displaystyle g\in {\mathcal {C}}^{1}(\mathbb {R} ^{d})}
v
∈
R
d
{\displaystyle \mathbf {v} \in \mathbb {R} ^{d}}
u
:
R
×
R
d
→
R
,
u
(
t
,
x
)
:=
g
(
x
+
v
t
)
+
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle u:\mathbb {R} \times \mathbb {R} ^{d}\to \mathbb {R} ,u(t,x):=g(x+\mathbf {v} t)+\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
solves the inhomogenous
d
{\displaystyle d}
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
u
(
t
,
x
)
−
v
⋅
∇
x
u
(
t
,
x
)
=
f
(
t
,
x
)
{\displaystyle \forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:\partial _{t}u(t,x)-\mathbf {v} \cdot \nabla _{x}u(t,x)=f(t,x)}
Note that, as in chapter 1, that there are many solutions, one for each continuously differentiable
g
{\displaystyle g}
Proof :
1.
We show that
u
{\displaystyle u}
g
(
x
+
v
t
)
{\displaystyle g(x+\mathbf {v} t)}
t
,
x
1
,
…
,
x
d
{\displaystyle t,x_{1},\ldots ,x_{d}}
∂
x
n
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
,
n
∈
{
1
,
…
,
d
}
{\displaystyle \partial _{x_{n}}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds,n\in \{1,\ldots ,d\}}
follows from the Leibniz integral rule (see exercise 1). The expression
∂
t
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle \partial _{t}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
we will later in this proof show to be equal to
f
(
t
,
x
)
+
v
⋅
∇
x
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle f(t,x)+\mathbf {v} \cdot \nabla _{x}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
which exists because
∇
x
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle \nabla _{x}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
just consists of the derivatives
∂
x
n
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
,
n
∈
{
1
,
…
,
d
}
{\displaystyle \partial _{x_{n}}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds,n\in \{1,\ldots ,d\}}
2.
We show that
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
u
(
t
,
x
)
−
v
⋅
∇
x
u
(
t
,
x
)
=
f
(
t
,
x
)
{\displaystyle \forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:\partial _{t}u(t,x)-\mathbf {v} \cdot \nabla _{x}u(t,x)=f(t,x)}
in three substeps.
2.1
We show that
∂
t
g
(
x
+
v
t
)
−
v
⋅
∇
x
g
(
x
+
v
t
)
=
0
(
∗
)
{\displaystyle \partial _{t}g(x+\mathbf {v} t)-\mathbf {v} \cdot \nabla _{x}g(x+\mathbf {v} t)=0~~~~~(*)}
This is left to the reader as an exercise in the application of the multi-dimensional chain rule (see exercise 2).
2.2
We show that
∂
t
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
−
v
⋅
∇
x
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
=
f
(
t
,
x
)
(
∗
∗
)
{\displaystyle \partial _{t}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds-\mathbf {v} \cdot \nabla _{x}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds=f(t,x)~~~~~(**)}
We choose
F
(
t
,
x
)
:=
∫
0
t
f
(
s
,
x
−
v
s
)
d
s
{\displaystyle F(t,x):=\int _{0}^{t}f(s,x-\mathbf {v} s)ds}
so that we have
F
(
t
,
x
+
v
t
)
=
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle F(t,x+\mathbf {v} t)=\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
By the multi-dimensional chain rule, we obtain
d
d
t
F
(
t
,
x
+
v
t
)
=
(
∂
t
F
(
t
,
x
+
v
t
)
∂
x
1
F
(
t
,
x
+
v
t
)
⋯
∂
x
d
F
(
t
,
x
+
v
t
)
)
(
1
v
)
=
∂
t
F
(
t
,
x
+
v
t
)
+
v
⋅
∇
x
F
(
t
,
x
+
v
t
)
{\displaystyle {\begin{aligned}{\frac {d}{dt}}F(t,x+\mathbf {v} t)&={\begin{pmatrix}\partial _{t}F(t,x+\mathbf {v} t)&\partial _{x_{1}}F(t,x+\mathbf {v} t)&\cdots &\partial _{x_{d}}F(t,x+\mathbf {v} t)\end{pmatrix}}{\begin{pmatrix}1\\\mathbf {v} \end{pmatrix}}\\&=\partial _{t}F(t,x+\mathbf {v} t)+\mathbf {v} \cdot \nabla _{x}F(t,x+\mathbf {v} t)\end{aligned}}}
But on the one hand, we have by the fundamental theorem of calculus, that
∂
t
F
(
t
,
x
)
=
f
(
t
,
x
−
v
t
)
{\displaystyle \partial _{t}F(t,x)=f(t,x-\mathbf {v} t)}
∂
t
F
(
t
,
x
+
v
t
)
=
f
(
t
,
x
)
{\displaystyle \partial _{t}F(t,x+\mathbf {v} t)=f(t,x)}
and on the other hand
∂
x
n
F
(
t
,
x
+
v
t
)
=
∂
x
n
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle \partial _{x_{n}}F(t,x+\mathbf {v} t)=\partial _{x_{n}}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
, seeing that the differential quotient of the definition of
∂
x
n
{\displaystyle \partial _{x_{n}}}
d
d
t
F
(
t
,
x
+
v
t
)
=
∂
t
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle {\frac {d}{dt}}F(t,x+\mathbf {v} t)=\partial _{t}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
, the second part of the second part of the proof is finished.
2.3
We add
(
∗
)
{\displaystyle (*)}
(
∗
∗
)
{\displaystyle (**)}
◻
{\displaystyle \Box }
Theorem and definition 2.4 :
If
f
∈
C
1
(
R
×
R
d
)
{\displaystyle f\in {\mathcal {C}}^{1}(\mathbb {R} \times \mathbb {R} ^{d})}
g
∈
C
1
(
R
d
)
{\displaystyle g\in {\mathcal {C}}^{1}(\mathbb {R} ^{d})}
u
:
R
×
R
d
→
R
,
u
(
t
,
x
)
:=
g
(
x
+
v
t
)
+
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle u:\mathbb {R} \times \mathbb {R} ^{d}\to \mathbb {R} ,u(t,x):=g(x+\mathbf {v} t)+\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
is the unique solution of the initial value problem of the transport equation
{
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
u
(
t
,
x
)
−
v
⋅
∇
x
u
(
t
,
x
)
=
f
(
t
,
x
)
∀
x
∈
R
d
:
u
(
0
,
x
)
=
g
(
x
)
{\displaystyle {\begin{cases}\forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:&\partial _{t}u(t,x)-\mathbf {v} \cdot \nabla _{x}u(t,x)=f(t,x)\\\forall x\in \mathbb {R} ^{d}:&u(0,x)=g(x)\end{cases}}}
Proof :
Quite easily,
u
(
0
,
x
)
=
g
(
x
+
v
⋅
0
)
+
∫
0
0
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
=
g
(
x
)
{\displaystyle u(0,x)=g(x+\mathbf {v} \cdot 0)+\int _{0}^{0}f(s,x+\mathbf {v} (t-s))ds=g(x)}
u
{\displaystyle u}
Assume that
v
{\displaystyle v}
v
=
u
{\displaystyle v=u}
We define
w
:=
u
−
v
{\displaystyle w:=u-v}
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
w
(
t
,
x
)
−
v
⋅
∇
x
w
(
t
,
x
)
=
(
∂
t
u
(
t
,
x
)
−
v
⋅
∇
x
u
(
t
,
x
)
)
−
(
∂
t
v
(
t
,
x
)
−
v
⋅
∇
x
v
(
t
,
x
)
)
=
f
(
t
,
x
)
−
f
(
t
,
x
)
=
0
(
∗
)
∀
x
∈
R
d
:
w
(
0
,
x
)
=
u
(
0
,
x
)
−
v
(
0
,
x
)
=
g
(
x
)
−
g
(
x
)
=
0
(
∗
∗
)
{\displaystyle {\begin{array}{llll}\forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:&\partial _{t}w(t,x)-\mathbf {v} \cdot \nabla _{x}w(t,x)&=(\partial _{t}u(t,x)-\mathbf {v} \cdot \nabla _{x}u(t,x))-(\partial _{t}v(t,x)-\mathbf {v} \cdot \nabla _{x}v(t,x))&\\&&=f(t,x)-f(t,x)=0&~~~~~(*)\\\forall x\in \mathbb {R} ^{d}:&w(0,x)=u(0,x)-v(0,x)&=g(x)-g(x)=0&~~~~~(**)\end{array}}}
Analogous to the proof of uniqueness of solutions for the one-dimensional homogenous initial value problem of the transport equation in the first chapter, we define for arbitrary
(
t
,
x
)
∈
R
×
R
d
{\displaystyle (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}}
μ
(
t
,
x
)
(
ξ
)
:=
w
(
t
−
ξ
,
x
+
v
ξ
)
{\displaystyle \mu _{(t,x)}(\xi ):=w(t-\xi ,x+\mathbf {v} \xi )}
Using the multi-dimensional chain rule, we calculate
μ
(
t
,
x
)
′
(
ξ
)
{\displaystyle \mu _{(t,x)}'(\xi )}
μ
(
t
,
x
)
′
(
ξ
)
:=
d
d
ξ
w
(
t
−
ξ
,
x
+
v
ξ
)
by defs. of the
′
symbol and
μ
=
(
∂
t
w
(
t
−
ξ
,
x
+
v
ξ
)
∂
x
1
w
(
t
−
ξ
,
x
+
v
ξ
)
⋯
∂
x
d
w
(
t
−
ξ
,
x
+
v
ξ
)
)
(
−
1
v
)
chain rule
=
−
∂
t
w
(
t
−
ξ
,
x
+
v
ξ
)
+
v
⋅
∇
x
w
(
t
−
ξ
,
x
+
v
ξ
)
=
0
(
∗
)
{\displaystyle {\begin{aligned}\mu _{(t,x)}'(\xi )&:={\frac {d}{d\xi }}w(t-\xi ,x+\mathbf {v} \xi )&{\text{ by defs. of the }}'{\text{ symbol and }}\mu \\&={\begin{pmatrix}\partial _{t}w(t-\xi ,x+\mathbf {v} \xi )&\partial _{x_{1}}w(t-\xi ,x+\mathbf {v} \xi )&\cdots &\partial _{x_{d}}w(t-\xi ,x+\mathbf {v} \xi )\end{pmatrix}}{\begin{pmatrix}-1\\\mathbf {v} \end{pmatrix}}&{\text{chain rule}}\\&=-\partial _{t}w(t-\xi ,x+\mathbf {v} \xi )+\mathbf {v} \cdot \nabla _{x}w(t-\xi ,x+\mathbf {v} \xi )&\\&=0&(*)\end{aligned}}}
Therefore, for all
(
t
,
x
)
∈
R
×
R
d
{\displaystyle (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}}
μ
(
t
,
x
)
(
ξ
)
{\displaystyle \mu _{(t,x)}(\xi )}
∀
(
t
,
x
)
∈
R
×
R
d
:
w
(
t
,
x
)
=
μ
(
t
,
x
)
(
0
)
=
μ
(
t
,
x
)
(
t
)
=
w
(
0
,
x
+
v
t
)
=
(
∗
∗
)
0
{\displaystyle \forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:w(t,x)=\mu _{(t,x)}(0)=\mu _{(t,x)}(t)=w(0,x+\mathbf {v} t){\overset {(**)}{=}}0}
, which shows that
w
=
u
−
v
=
0
{\displaystyle w=u-v=0}
u
=
v
{\displaystyle u=v}
◻
{\displaystyle \Box }
Let
f
∈
C
1
(
R
×
R
d
)
{\displaystyle f\in {\mathcal {C}}^{1}(\mathbb {R} \times \mathbb {R} ^{d})}
v
∈
R
d
{\displaystyle \mathbf {v} \in \mathbb {R} ^{d}}
n
∈
{
1
,
…
,
d
}
{\displaystyle n\in \{1,\ldots ,d\}}
∂
x
n
∫
0
t
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle \partial _{x_{n}}\int _{0}^{t}f(s,x+\mathbf {v} (t-s))ds}
is equal to
∫
0
t
∂
x
n
f
(
s
,
x
+
v
(
t
−
s
)
)
d
s
{\displaystyle \int _{0}^{t}\partial _{x_{n}}f(s,x+\mathbf {v} (t-s))ds}
and therefore exists.
Let
g
∈
C
1
(
R
d
)
{\displaystyle g\in {\mathcal {C}}^{1}(\mathbb {R} ^{d})}
v
∈
R
d
{\displaystyle \mathbf {v} \in \mathbb {R} ^{d}}
∂
t
g
(
x
+
v
t
)
{\displaystyle \partial _{t}g(x+\mathbf {v} t)}
Find the unique solution to the initial value problem
{
∀
(
t
,
x
)
∈
R
×
R
3
:
∂
t
u
(
t
,
x
)
−
(
2
3
4
)
⋅
∇
x
u
(
t
,
x
)
=
t
5
+
x
1
6
+
x
2
7
+
x
3
8
∀
x
∈
R
3
:
u
(
0
,
x
)
=
x
1
9
+
x
2
10
+
x
3
11
{\displaystyle {\begin{cases}\forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{3}:&\partial _{t}u(t,x)-{\begin{pmatrix}2\\3\\4\end{pmatrix}}\cdot \nabla _{x}u(t,x)=t^{5}+x_{1}^{6}+x_{2}^{7}+x_{3}^{8}\\\forall x\in \mathbb {R} ^{3}:&u(0,x)=x_{1}^{9}+x_{2}^{10}+x_{3}^{11}\end{cases}}}
Before we dive deeply into the chapter, let's first motivate the notion of a test function. Let's consider two functions which are piecewise constant on the intervals
[
0
,
1
)
,
[
1
,
2
)
,
[
2
,
3
)
,
[
3
,
4
)
,
[
4
,
5
)
{\displaystyle [0,1),[1,2),[2,3),[3,4),[4,5)}
Let's call the left function
f
1
{\displaystyle f_{1}}
f
2
{\displaystyle f_{2}}
Of course we can easily see that the two functions are different; they differ on the interval
[
4
,
5
)
{\displaystyle [4,5)}
∫
R
φ
(
x
)
f
1
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} }\varphi (x)f_{1}(x)dx}
∫
R
φ
(
x
)
f
2
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} }\varphi (x)f_{2}(x)dx}
for functions
φ
{\displaystyle \varphi }
X
{\displaystyle {\mathcal {X}}}
We proceed with choosing
X
{\displaystyle {\mathcal {X}}}
f
1
≠
f
2
{\displaystyle f_{1}\neq f_{2}}
A
⊆
R
{\displaystyle A\subseteq \mathbb {R} }
characteristic function of
A
{\displaystyle A}
is defined as
χ
A
(
x
)
:=
{
1
x
∈
A
0
x
∉
A
{\displaystyle \chi _{A}(x):={\begin{cases}1&x\in A\\0&x\notin A\end{cases}}}
With this definition, we choose the set of functions
X
{\displaystyle {\mathcal {X}}}
X
:=
{
χ
[
0
,
1
)
,
χ
[
1
,
2
)
,
χ
[
2
,
3
)
,
χ
[
3
,
4
)
,
χ
[
4
,
5
)
}
{\displaystyle {\mathcal {X}}:=\{\chi _{[0,1)},\chi _{[1,2)},\chi _{[2,3)},\chi _{[3,4)},\chi _{[4,5)}\}}
It is easy to see (see exercise 1), that for
n
∈
{
1
,
2
,
3
,
4
,
5
}
{\displaystyle n\in \{1,2,3,4,5\}}
∫
R
χ
[
n
−
1
,
n
)
(
x
)
f
1
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} }\chi _{[n-1,n)}(x)f_{1}(x)dx}
equals the value of
f
1
{\displaystyle f_{1}}
[
n
−
1
,
n
)
{\displaystyle [n-1,n)}
f
2
{\displaystyle f_{2}}
[
n
−
1
,
n
)
,
n
∈
{
1
,
2
,
3
,
4
,
5
}
{\displaystyle [n-1,n),n\in \{1,2,3,4,5\}}
f
1
=
f
2
⇔
∀
φ
∈
X
:
∫
R
φ
(
x
)
f
1
(
x
)
d
x
=
∫
R
φ
(
x
)
f
2
(
x
)
d
x
{\displaystyle f_{1}=f_{2}\Leftrightarrow \forall \varphi \in {\mathcal {X}}:\int _{\mathbb {R} }\varphi (x)f_{1}(x)dx=\int _{\mathbb {R} }\varphi (x)f_{2}(x)dx}
This obviously needs five evaluations of each integral, as
#
X
=
5
{\displaystyle \#{\mathcal {X}}=5}
Since we used the functions in
X
{\displaystyle {\mathcal {X}}}
f
1
{\displaystyle f_{1}}
f
2
{\displaystyle f_{2}}
test functions . What we ask ourselves now is if this notion generalises from functions like
f
1
{\displaystyle f_{1}}
f
2
{\displaystyle f_{2}}
In order to write down the definition of a bump function more shortly, we need the following two definitions:
Now we are ready to define a bump function in a brief way:
These two properties make the function really look like a bump, as the following example shows:
The standard mollifier
η
{\displaystyle \eta }
d
=
1
{\displaystyle d=1}
Example 3.4: The standard mollifier
η
{\displaystyle \eta }
η
:
R
d
→
R
,
η
(
x
)
=
1
c
{
e
−
1
1
−
‖
x
‖
2
if
‖
x
‖
2
<
1
0
if
‖
x
‖
2
≥
1
{\displaystyle \eta :\mathbb {R} ^{d}\to \mathbb {R} ,\eta (x)={\frac {1}{c}}{\begin{cases}e^{-{\frac {1}{1-\|x\|^{2}}}}&{\text{ if }}\|x\|_{2}<1\\0&{\text{ if }}\|x\|_{2}\geq 1\end{cases}}}
, where
c
:=
∫
B
1
(
0
)
e
−
1
1
−
‖
x
‖
2
d
x
{\displaystyle c:=\int _{B_{1}(0)}e^{-{\frac {1}{1-\|x\|^{2}}}}dx}
As for the bump functions, in order to write down the definition of Schwartz functions shortly, we first need two helpful definitions.
Now we are ready to define a Schwartz function.
Definition 3.7 :
We call
ϕ
:
R
d
→
R
{\displaystyle \phi :\mathbb {R} ^{d}\to \mathbb {R} }
Schwartz function iff the following two conditions are satisfied:
ϕ
∈
C
∞
(
R
d
)
{\displaystyle \phi \in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
∀
α
,
β
∈
N
0
d
:
‖
x
α
∂
β
ϕ
‖
∞
<
∞
{\displaystyle \forall \alpha ,\beta \in \mathbb {N} _{0}^{d}:\|x^{\alpha }\partial _{\beta }\phi \|_{\infty }<\infty }
By
x
α
∂
β
ϕ
{\displaystyle x^{\alpha }\partial _{\beta }\phi }
x
↦
x
α
∂
β
ϕ
(
x
)
{\displaystyle x\mapsto x^{\alpha }\partial _{\beta }\phi (x)}
f
(
x
,
y
)
=
e
−
x
2
−
y
2
{\displaystyle f(x,y)=e^{-x^{2}-y^{2}}}
Example 3.8 :
The function
f
:
R
2
→
R
,
f
(
x
,
y
)
=
e
−
x
2
−
y
2
{\displaystyle f:\mathbb {R} ^{2}\to \mathbb {R} ,f(x,y)=e^{-x^{2}-y^{2}}}
is a Schwartz function.
Theorem 3.9 :
Every bump function is also a Schwartz function.
This means for example that the standard mollifier is a Schwartz function.
Proof :
Let
φ
{\displaystyle \varphi }
φ
∈
C
∞
(
R
d
)
{\displaystyle \varphi \in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
R
>
0
{\displaystyle R>0}
supp
φ
⊆
B
R
(
0
)
¯
{\displaystyle {\text{supp }}\varphi \subseteq {\overline {B_{R}(0)}}}
, as in
R
d
{\displaystyle \mathbb {R} ^{d}}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
‖
x
α
∂
β
φ
(
x
)
‖
∞
:=
sup
x
∈
R
d
|
x
α
∂
β
φ
(
x
)
|
=
sup
x
∈
B
R
(
0
)
¯
|
x
α
∂
β
φ
(
x
)
|
supp
φ
⊆
B
R
(
0
)
¯
=
sup
x
∈
B
R
(
0
)
¯
(
|
x
α
|
|
∂
β
φ
(
x
)
|
)
rules for absolute value
≤
sup
x
∈
B
R
(
0
)
¯
(
R
|
α
|
|
∂
β
φ
(
x
)
|
)
∀
i
∈
{
1
,
…
,
d
}
,
(
x
1
,
…
,
x
d
)
∈
B
R
(
0
)
¯
:
|
x
i
|
≤
R
<
∞
Extreme value theorem
{\displaystyle {\begin{aligned}\|x^{\alpha }\partial _{\beta }\varphi (x)\|_{\infty }&:=\sup _{x\in \mathbb {R} ^{d}}|x^{\alpha }\partial _{\beta }\varphi (x)|&\\&=\sup _{x\in {\overline {B_{R}(0)}}}|x^{\alpha }\partial _{\beta }\varphi (x)|&{\text{supp }}\varphi \subseteq {\overline {B_{R}(0)}}\\&=\sup _{x\in {\overline {B_{R}(0)}}}\left(|x^{\alpha }||\partial _{\beta }\varphi (x)|\right)&{\text{rules for absolute value}}\\&\leq \sup _{x\in {\overline {B_{R}(0)}}}\left(R^{|\alpha |}|\partial _{\beta }\varphi (x)|\right)&\forall i\in \{1,\ldots ,d\},(x_{1},\ldots ,x_{d})\in {\overline {B_{R}(0)}}:|x_{i}|\leq R\\&<\infty &{\text{Extreme value theorem}}\end{aligned}}}
◻
{\displaystyle \Box }
Now we define what convergence of a sequence of bump (Schwartz) functions to a bump (Schwartz) function means.
Definition 3.11 :
We say that the sequence of Schwartz functions
(
ϕ
i
)
i
∈
N
{\displaystyle (\phi _{i})_{i\in \mathbb {N} }}
ϕ
{\displaystyle \phi }
∀
α
,
β
∈
N
0
d
:
‖
x
α
∂
β
ϕ
i
−
x
α
∂
β
ϕ
‖
∞
→
0
,
i
→
∞
{\displaystyle \forall \alpha ,\beta \in \mathbb {N} _{0}^{d}:\|x^{\alpha }\partial _{\beta }\phi _{i}-x^{\alpha }\partial _{\beta }\phi \|_{\infty }\to 0,i\to \infty }
Theorem 3.12 :
Let
(
φ
i
)
i
∈
N
{\displaystyle (\varphi _{i})_{i\in \mathbb {N} }}
φ
i
→
φ
{\displaystyle \varphi _{i}\to \varphi }
φ
i
→
φ
{\displaystyle \varphi _{i}\to \varphi }
Proof :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
(
φ
l
)
l
∈
N
{\displaystyle (\varphi _{l})_{l\in \mathbb {N} }}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
φ
l
→
φ
∈
D
(
O
)
{\displaystyle \varphi _{l}\to \varphi \in {\mathcal {D}}(O)}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
K
⊂
R
d
{\displaystyle K\subset \mathbb {R} ^{d}}
supp
φ
l
{\displaystyle {\text{supp }}\varphi _{l}}
supp
φ
⊆
K
{\displaystyle {\text{supp }}\varphi \subseteq K}
‖
φ
l
−
φ
‖
∞
≥
|
c
|
{\displaystyle \|\varphi _{l}-\varphi \|_{\infty }\geq |c|}
c
∈
R
{\displaystyle c\in \mathbb {R} }
φ
{\displaystyle \varphi }
K
{\displaystyle K}
φ
l
→
φ
{\displaystyle \varphi _{l}\to \varphi }
In
R
d
{\displaystyle \mathbb {R} ^{d}}
K
⊂
B
R
(
0
)
{\displaystyle K\subset B_{R}(0)}
R
>
0
{\displaystyle R>0}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
‖
x
α
∂
β
φ
l
−
x
α
∂
β
φ
‖
∞
=
sup
x
∈
R
d
|
x
α
∂
β
φ
l
(
x
)
−
x
α
∂
β
φ
(
x
)
|
definition of the supremum norm
=
sup
x
∈
B
R
(
0
)
|
x
α
∂
β
φ
l
(
x
)
−
x
α
∂
β
φ
(
x
)
|
as
supp
φ
l
,
supp
φ
⊆
K
⊂
B
R
(
0
)
≤
R
|
α
|
sup
x
∈
B
R
(
0
)
|
∂
β
φ
l
(
x
)
−
∂
β
φ
(
x
)
|
∀
i
∈
{
1
,
…
,
d
}
,
(
x
1
,
…
,
x
d
)
∈
B
R
(
0
)
¯
:
|
x
i
|
≤
R
=
R
|
α
|
sup
x
∈
R
d
|
∂
β
φ
l
(
x
)
−
∂
β
φ
(
x
)
|
as
supp
φ
l
,
supp
φ
⊆
K
⊂
B
R
(
0
)
=
R
|
α
|
‖
∂
β
φ
l
(
x
)
−
∂
β
φ
(
x
)
‖
∞
definition of the supremum norm
→
0
,
l
→
∞
since
φ
l
→
φ
in
D
(
O
)
{\displaystyle {\begin{aligned}\|x^{\alpha }\partial _{\beta }\varphi _{l}-x^{\alpha }\partial _{\beta }\varphi \|_{\infty }&=\sup _{x\in \mathbb {R} ^{d}}\left|x^{\alpha }\partial _{\beta }\varphi _{l}(x)-x^{\alpha }\partial _{\beta }\varphi (x)\right|&{\text{ definition of the supremum norm}}\\&=\sup _{x\in B_{R}(0)}\left|x^{\alpha }\partial _{\beta }\varphi _{l}(x)-x^{\alpha }\partial _{\beta }\varphi (x)\right|&{\text{ as }}{\text{supp }}\varphi _{l},{\text{supp }}\varphi \subseteq K\subset B_{R}(0)\\&\leq R^{|\alpha |}\sup _{x\in B_{R}(0)}\left|\partial _{\beta }\varphi _{l}(x)-\partial _{\beta }\varphi (x)\right|&\forall i\in \{1,\ldots ,d\},(x_{1},\ldots ,x_{d})\in {\overline {B_{R}(0)}}:|x_{i}|\leq R\\&=R^{|\alpha |}\sup _{x\in \mathbb {R} ^{d}}\left|\partial _{\beta }\varphi _{l}(x)-\partial _{\beta }\varphi (x)\right|&{\text{ as }}{\text{supp }}\varphi _{l},{\text{supp }}\varphi \subseteq K\subset B_{R}(0)\\&=R^{|\alpha |}\left\|\partial _{\beta }\varphi _{l}(x)-\partial _{\beta }\varphi (x)\right\|_{\infty }&{\text{ definition of the supremum norm}}\\&\to 0,l\to \infty &{\text{ since }}\varphi _{l}\to \varphi {\text{ in }}{\mathcal {D}}(O)\end{aligned}}}
Therefore the sequence converges with respect to the notion of convergence for Schwartz functions.
◻
{\displaystyle \Box }
In this section, we want to show that we can test equality of continuous functions
f
,
g
{\displaystyle f,g}
∫
R
d
f
(
x
)
φ
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}f(x)\varphi (x)dx}
∫
R
d
g
(
x
)
φ
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}g(x)\varphi (x)dx}
for all
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
φ
∈
S
(
R
d
)
{\displaystyle \varphi \in {\mathcal {S}}(\mathbb {R} ^{d})}
D
(
O
)
⊂
S
(
R
d
)
{\displaystyle {\mathcal {D}}(O)\subset {\mathcal {S}}(\mathbb {R} ^{d})}
But before we are able to show that, we need a modified mollifier, where the modification is dependent of a parameter, and two lemmas about that modified mollifier.
Definition 3.13 :
For
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
η
R
:
R
d
→
R
,
η
R
(
x
)
=
η
(
x
R
)
/
R
d
{\displaystyle \eta _{R}:\mathbb {R} ^{d}\to \mathbb {R} ,\eta _{R}(x)=\eta \left({\frac {x}{R}}\right){\big /}R^{d}}
Lemma 3.14 :
Let
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
supp
η
R
=
B
R
(
0
)
¯
{\displaystyle {\text{supp }}\eta _{R}={\overline {B_{R}(0)}}}
Proof :
From the definition of
η
{\displaystyle \eta }
supp
η
=
B
1
(
0
)
¯
{\displaystyle {\text{supp }}\eta ={\overline {B_{1}(0)}}}
Further, for
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
x
R
∈
B
1
(
0
)
¯
⇔
‖
x
R
‖
≤
1
⇔
‖
x
‖
≤
R
⇔
x
∈
B
R
(
0
)
¯
{\displaystyle {\begin{aligned}{\frac {x}{R}}\in {\overline {B_{1}(0)}}&\Leftrightarrow \left\|{\frac {x}{R}}\right\|\leq 1\\&\Leftrightarrow \|x\|\leq R\\&\Leftrightarrow x\in {\overline {B_{R}(0)}}\end{aligned}}}
Therefore, and since
x
∈
supp
η
R
⇔
x
R
∈
supp
η
{\displaystyle x\in {\text{supp }}\eta _{R}\Leftrightarrow {\frac {x}{R}}\in {\text{supp }}\eta }
, we have:
x
∈
supp
η
R
⇔
x
∈
B
R
(
0
)
¯
{\displaystyle x\in {\text{supp }}\eta _{R}\Leftrightarrow x\in {\overline {B_{R}(0)}}}
◻
{\displaystyle \Box }
In order to prove the next lemma, we need the following theorem from integration theory:
Theorem 3.15 : (Multi-dimensional integration by substitution)
If
O
,
U
⊆
R
d
{\displaystyle O,U\subseteq \mathbb {R} ^{d}}
ψ
:
U
→
O
{\displaystyle \psi :U\to O}
∫
O
f
(
x
)
d
x
=
∫
U
f
(
ψ
(
x
)
)
|
det
J
ψ
(
x
)
|
d
x
{\displaystyle \int _{O}f(x)dx=\int _{U}f(\psi (x))|\det J_{\psi }(x)|dx}
We will omit the proof, as understanding it is not very important for understanding this wikibook.
Lemma 3.16 :
Let
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
∫
R
d
η
R
(
x
)
d
x
=
1
{\displaystyle \int _{\mathbb {R} ^{d}}\eta _{R}(x)dx=1}
Proof :
∫
R
d
η
R
(
x
)
d
x
=
∫
R
d
η
(
x
R
)
/
R
d
d
x
Def. of
η
R
=
∫
R
d
η
(
x
)
d
x
integration by substitution using
x
↦
R
x
=
∫
B
1
(
0
)
η
(
x
)
d
x
Def. of
η
=
∫
B
1
(
0
)
e
−
1
1
−
‖
x
‖
d
x
∫
B
1
(
0
)
e
−
1
1
−
‖
x
‖
d
x
Def. of
η
=
1
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}\eta _{R}(x)dx&=\int _{\mathbb {R} ^{d}}\eta \left({\frac {x}{R}}\right){\big /}R^{d}dx&{\text{Def. of }}\eta _{R}\\&=\int _{\mathbb {R} ^{d}}\eta (x)dx&{\text{integration by substitution using }}x\mapsto Rx\\&=\int _{B_{1}(0)}\eta (x)dx&{\text{Def. of }}\eta \\&={\frac {\int _{B_{1}(0)}e^{-{\frac {1}{1-\|x\|}}}dx}{\int _{B_{1}(0)}e^{-{\frac {1}{1-\|x\|}}}dx}}&{\text{Def. of }}\eta \\&=1\end{aligned}}}
◻
{\displaystyle \Box }
Now we are ready to prove the ‘testing’ property of test functions:
Theorem 3.17 :
Let
f
,
g
:
R
d
→
R
{\displaystyle f,g:\mathbb {R} ^{d}\to \mathbb {R} }
∀
φ
∈
D
(
O
)
:
∫
R
d
φ
(
x
)
f
(
x
)
d
x
=
∫
R
d
φ
(
x
)
g
(
x
)
d
x
{\displaystyle \forall \varphi \in {\mathcal {D}}(O):\int _{\mathbb {R} ^{d}}\varphi (x)f(x)dx=\int _{\mathbb {R} ^{d}}\varphi (x)g(x)dx}
then
f
=
g
{\displaystyle f=g}
Proof :
Let
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
ϵ
∈
R
>
0
{\displaystyle \epsilon \in \mathbb {R} _{>0}}
f
{\displaystyle f}
δ
∈
R
>
0
{\displaystyle \delta \in \mathbb {R} _{>0}}
∀
y
∈
B
δ
(
x
)
¯
:
|
f
(
x
)
−
f
(
y
)
|
<
ϵ
{\displaystyle \forall y\in {\overline {B_{\delta }(x)}}:|f(x)-f(y)|<\epsilon }
Then we have
|
f
(
x
)
−
∫
R
d
f
(
y
)
η
δ
(
x
−
y
)
d
y
|
=
|
∫
R
d
(
f
(
x
)
−
f
(
y
)
)
η
δ
(
x
−
y
)
d
y
|
lemma 3.16
≤
∫
R
d
|
f
(
x
)
−
f
(
y
)
|
η
δ
(
x
−
y
)
d
y
triangle ineq. for the
∫
and
η
δ
≥
0
=
∫
B
δ
(
0
)
¯
|
f
(
x
)
−
f
(
y
)
|
η
δ
(
x
−
y
)
d
y
lemma 3.14
≤
∫
B
δ
(
0
)
¯
ϵ
η
δ
(
x
−
y
)
d
y
monotony of the
∫
≤
ϵ
lemma 3.16 and
η
δ
≥
0
{\displaystyle {\begin{aligned}\left|f(x)-\int _{\mathbb {R} ^{d}}f(y)\eta _{\delta }(x-y)dy\right|&=\left|\int _{\mathbb {R} ^{d}}(f(x)-f(y))\eta _{\delta }(x-y)dy\right|&{\text{lemma 3.16}}\\&\leq \int _{\mathbb {R} ^{d}}|f(x)-f(y)|\eta _{\delta }(x-y)dy&{\text{triangle ineq. for the }}\int {\text{ and }}\eta _{\delta }\geq 0\\&=\int _{\overline {B_{\delta }(0)}}|f(x)-f(y)|\eta _{\delta }(x-y)dy&{\text{lemma 3.14}}\\&\leq \int _{\overline {B_{\delta }(0)}}\epsilon \eta _{\delta }(x-y)dy&{\text{monotony of the }}\int \\&\leq \epsilon &{\text{lemma 3.16 and }}\eta _{\delta }\geq 0\end{aligned}}}
Therefore,
∫
R
d
f
(
y
)
η
δ
(
x
−
y
)
d
y
→
f
(
x
)
,
δ
→
0
{\displaystyle \int _{\mathbb {R} ^{d}}f(y)\eta _{\delta }(x-y)dy\to f(x),\delta \to 0}
∫
R
d
g
(
y
)
η
δ
(
x
−
y
)
d
y
→
g
(
x
)
,
δ
→
0
{\displaystyle \int _{\mathbb {R} ^{d}}g(y)\eta _{\delta }(x-y)dy\to g(x),\delta \to 0}
∀
δ
∈
R
>
0
:
∫
R
d
g
(
y
)
η
δ
(
x
−
y
)
d
y
=
∫
R
d
f
(
y
)
η
δ
(
x
−
y
)
d
y
{\displaystyle \forall \delta \in \mathbb {R} _{>0}:\int _{\mathbb {R} ^{d}}g(y)\eta _{\delta }(x-y)dy=\int _{\mathbb {R} ^{d}}f(y)\eta _{\delta }(x-y)dy}
As limits in the reals are unique, it follows that
f
(
x
)
=
g
(
x
)
{\displaystyle f(x)=g(x)}
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
f
=
g
{\displaystyle f=g}
◻
{\displaystyle \Box }
Remark 3.18 :
Let
f
,
g
:
R
d
→
R
{\displaystyle f,g:\mathbb {R} ^{d}\to \mathbb {R} }
∀
φ
∈
S
(
R
d
)
:
∫
R
d
φ
(
x
)
f
(
x
)
d
x
=
∫
R
d
φ
(
x
)
g
(
x
)
d
x
{\displaystyle \forall \varphi \in {\mathcal {S}}(\mathbb {R} ^{d}):\int _{\mathbb {R} ^{d}}\varphi (x)f(x)dx=\int _{\mathbb {R} ^{d}}\varphi (x)g(x)dx}
then
f
=
g
{\displaystyle f=g}
Proof :
This follows from all bump functions being Schwartz functions, which is why the requirements for theorem 3.17 are met.
◻
{\displaystyle \Box }
Let
b
∈
R
{\displaystyle b\in \mathbb {R} }
f
:
R
→
R
{\displaystyle f:\mathbb {R} \to \mathbb {R} }
[
b
−
1
,
b
)
{\displaystyle [b-1,b)}
∀
y
∈
[
b
−
1
,
b
)
:
∫
R
χ
[
b
−
1
,
b
)
(
x
)
f
(
x
)
d
x
=
f
(
y
)
{\displaystyle \forall y\in [b-1,b):\int _{\mathbb {R} }\chi _{[b-1,b)}(x)f(x)dx=f(y)}
Prove that the standard mollifier as defined in example 3.4 is a bump function by proceeding as follows:
Prove that the function
x
↦
{
e
−
1
x
x
>
0
0
x
≤
0
{\displaystyle x\mapsto {\begin{cases}e^{-{\frac {1}{x}}}&x>0\\0&x\leq 0\end{cases}}}
is contained in
C
∞
(
R
)
{\displaystyle {\mathcal {C}}^{\infty }(\mathbb {R} )}
Prove that the function
x
↦
1
−
‖
x
‖
{\displaystyle x\mapsto 1-\|x\|}
is contained in
C
∞
(
R
d
)
{\displaystyle {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
Conclude that
η
∈
C
∞
(
R
d
)
{\displaystyle \eta \in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
Prove that
supp
η
{\displaystyle {\text{supp }}\eta }
supp
η
{\displaystyle {\text{supp }}\eta }
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
ϕ
∈
S
(
R
d
)
{\displaystyle \phi \in {\mathcal {S}}(\mathbb {R} ^{d})}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
∂
α
φ
∈
D
(
O
)
{\displaystyle \partial _{\alpha }\varphi \in {\mathcal {D}}(O)}
x
α
∂
β
ϕ
∈
S
(
R
d
)
{\displaystyle x^{\alpha }\partial _{\beta }\phi \in {\mathcal {S}}(\mathbb {R} ^{d})}
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
φ
1
,
…
,
φ
n
∈
D
(
O
)
{\displaystyle \varphi _{1},\ldots ,\varphi _{n}\in {\mathcal {D}}(O)}
c
1
,
…
,
c
n
∈
R
{\displaystyle c_{1},\ldots ,c_{n}\in \mathbb {R} }
∑
j
=
1
n
c
j
φ
j
∈
D
(
O
)
{\displaystyle \sum _{j=1}^{n}c_{j}\varphi _{j}\in {\mathcal {D}}(O)}
Let
ϕ
1
,
…
,
ϕ
n
{\displaystyle \phi _{1},\ldots ,\phi _{n}}
c
1
,
…
,
c
n
∈
R
{\displaystyle c_{1},\ldots ,c_{n}\in \mathbb {R} }
∑
j
=
1
n
c
j
ϕ
j
{\displaystyle \sum _{j=1}^{n}c_{j}\phi _{j}}
Let
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
p
(
x
)
:=
∑
ς
≤
α
c
ς
x
ς
{\displaystyle p(x):=\sum _{\varsigma \leq \alpha }c_{\varsigma }x^{\varsigma }}
ϕ
l
→
ϕ
{\displaystyle \phi _{l}\to \phi }
p
ϕ
l
→
p
ϕ
{\displaystyle p\phi _{l}\to p\phi }
Theorem 4.3 :
Let
T
{\displaystyle {\mathcal {T}}}
T
{\displaystyle {\mathcal {T}}}
Proof :
Let
T
{\displaystyle {\mathcal {T}}}
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
1.
We show that
T
(
φ
)
{\displaystyle {\mathcal {T}}(\varphi )}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
Due to theorem 3.9, every bump function is a Schwartz function, which is why the expression
T
(
φ
)
{\displaystyle {\mathcal {T}}(\varphi )}
makes sense for every
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
2.
We show that the restriction is linear.
Let
a
,
b
∈
R
{\displaystyle a,b\in \mathbb {R} }
φ
,
ϑ
∈
D
(
O
)
{\displaystyle \varphi ,\vartheta \in {\mathcal {D}}(O)}
φ
{\displaystyle \varphi }
ϑ
{\displaystyle \vartheta }
∀
a
,
b
∈
R
,
φ
,
ϑ
∈
D
(
O
)
:
T
(
a
φ
+
b
ϑ
)
=
a
T
(
φ
)
+
b
T
(
ϑ
)
{\displaystyle \forall a,b\in \mathbb {R} ,\varphi ,\vartheta \in {\mathcal {D}}(O):{\mathcal {T}}(a\varphi +b\vartheta )=a{\mathcal {T}}(\varphi )+b{\mathcal {T}}(\vartheta )}
due to the linearity of
T
{\displaystyle {\mathcal {T}}}
T
{\displaystyle {\mathcal {T}}}
3.
We show that the restriction of
T
{\displaystyle {\mathcal {T}}}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
φ
l
→
φ
{\displaystyle \varphi _{l}\to \varphi }
φ
l
→
φ
{\displaystyle \varphi _{l}\to \varphi }
T
{\displaystyle {\mathcal {T}}}
T
(
φ
l
)
→
T
(
φ
)
{\displaystyle {\mathcal {T}}(\varphi _{l})\to {\mathcal {T}}(\varphi )}
◻
{\displaystyle \Box }
The convolution of two functions may not always exist, but there are sufficient conditions for it to exist:
Theorem 4.5 :
Let
p
,
q
∈
[
1
,
∞
]
{\displaystyle p,q\in [1,\infty ]}
1
p
+
1
q
=
1
{\displaystyle {\frac {1}{p}}+{\frac {1}{q}}=1}
f
∈
L
p
(
R
d
)
{\displaystyle f\in L^{p}(\mathbb {R} ^{d})}
g
∈
L
q
(
R
d
)
{\displaystyle g\in L^{q}(\mathbb {R} ^{d})}
y
∈
O
{\displaystyle y\in O}
∫
R
d
f
(
x
)
g
(
y
−
x
)
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}f(x)g(y-x)dx}
has a well-defined real value.
Proof :
Due to Hölder's inequality ,
∫
R
d
|
f
(
x
)
g
(
y
−
x
)
|
d
x
≤
(
∫
R
d
|
f
(
x
)
|
p
d
x
)
1
/
p
(
∫
R
d
|
g
(
y
−
x
)
|
q
d
x
)
1
/
q
<
∞
{\displaystyle \int _{\mathbb {R} ^{d}}|f(x)g(y-x)|dx\leq \left(\int _{\mathbb {R} ^{d}}|f(x)|^{p}dx\right)^{1/p}\left(\int _{\mathbb {R} ^{d}}|g(y-x)|^{q}dx\right)^{1/q}<\infty }
◻
{\displaystyle \Box }
We shall now prove that the convolution is commutative, i. e.
f
∗
g
=
g
∗
f
{\displaystyle f*g=g*f}
Proof :
We apply multi-dimensional integration by substitution using the diffeomorphism
x
↦
y
−
x
{\displaystyle x\mapsto y-x}
(
f
∗
g
)
(
y
)
=
∫
R
d
f
(
x
)
g
(
y
−
x
)
d
x
=
∫
R
d
f
(
y
−
x
)
g
(
x
)
d
x
=
(
g
∗
f
)
(
y
)
{\displaystyle (f*g)(y)=\int _{\mathbb {R} ^{d}}f(x)g(y-x)dx=\int _{\mathbb {R} ^{d}}f(y-x)g(x)dx=(g*f)(y)}
◻
{\displaystyle \Box }
Lemma 4.7 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
f
∈
L
1
(
R
d
)
{\displaystyle f\in L^{1}(\mathbb {R} ^{d})}
f
∗
η
δ
∈
C
∞
(
R
d
)
{\displaystyle f*\eta _{\delta }\in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
Proof :
Let
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
y
∈
R
d
{\displaystyle y\in \mathbb {R} ^{d}}
∫
R
d
|
f
(
x
)
∂
α
η
δ
(
y
−
x
)
|
d
x
≤
‖
∂
α
η
δ
‖
∞
∫
R
d
|
f
(
x
)
|
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}|f(x)\partial _{\alpha }\eta _{\delta }(y-x)|dx\leq \|\partial _{\alpha }\eta _{\delta }\|_{\infty }\int _{\mathbb {R} ^{d}}|f(x)|dx}
and further
|
f
(
x
)
∂
α
η
δ
(
y
−
x
)
|
≤
|
f
(
x
)
|
{\displaystyle |f(x)\partial _{\alpha }\eta _{\delta }(y-x)|\leq |f(x)|}
Leibniz' integral rule (theorem 2.2) is applicable, and by repeated application of Leibniz' integral rule we obtain
∂
α
f
∗
η
δ
=
f
∗
∂
α
η
δ
{\displaystyle \partial _{\alpha }f*\eta _{\delta }=f*\partial _{\alpha }\eta _{\delta }}
◻
{\displaystyle \Box }
In this section, we shortly study a class of distributions which we call regular distributions . In particular, we will see that for certain kinds of functions there exist corresponding distributions.
Two questions related to this definition could be asked: Given a function
f
:
R
d
→
R
{\displaystyle f:\mathbb {R} ^{d}\to \mathbb {R} }
T
f
:
D
(
O
)
→
R
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {D}}(O)\to \mathbb {R} }
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
T
f
(
φ
)
:=
∫
O
f
(
x
)
φ
(
x
)
d
x
{\displaystyle {\mathcal {T}}_{f}(\varphi ):=\int _{O}f(x)\varphi (x)dx}
well-defined and a distribution? Or is
T
f
:
S
(
R
d
)
→
R
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {S}}(\mathbb {R} ^{d})\to \mathbb {R} }
T
f
(
ϕ
)
:=
∫
R
d
f
(
x
)
ϕ
(
x
)
d
x
{\displaystyle {\mathcal {T}}_{f}(\phi ):=\int _{\mathbb {R} ^{d}}f(x)\phi (x)dx}
well-defined and a tempered distribution? In general, the answer to these two questions is no, but both questions can be answered with yes if the respective function
f
{\displaystyle f}
f
{\displaystyle f}
Now we are ready to give some sufficient conditions on
f
{\displaystyle f}
T
f
:
D
(
O
)
→
R
,
T
f
(
φ
)
:=
∫
O
f
(
x
)
φ
(
x
)
d
x
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {D}}(O)\to \mathbb {R} ,{\mathcal {T}}_{f}(\varphi ):=\int _{O}f(x)\varphi (x)dx}
or
T
f
:
S
(
R
d
)
→
R
,
T
f
(
ϕ
)
:=
∫
R
d
f
(
x
)
ϕ
(
x
)
d
x
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {S}}(\mathbb {R} ^{d})\to \mathbb {R} ,{\mathcal {T}}_{f}(\phi ):=\int _{\mathbb {R} ^{d}}f(x)\phi (x)dx}
Theorem 4.11 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
f
:
O
→
R
{\displaystyle f:O\to \mathbb {R} }
T
f
:
D
(
O
)
→
R
,
T
f
(
φ
)
:=
∫
O
f
(
x
)
φ
(
x
)
d
x
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {D}}(O)\to \mathbb {R} ,{\mathcal {T}}_{f}(\varphi ):=\int _{O}f(x)\varphi (x)dx}
is a regular distribution iff
f
∈
L
loc
1
(
O
)
{\displaystyle f\in L_{\text{loc}}^{1}(O)}
Proof :
1.
We show that if
f
∈
L
loc
1
(
O
)
{\displaystyle f\in L_{\text{loc}}^{1}(O)}
T
f
:
D
(
O
)
→
R
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {D}}(O)\to \mathbb {R} }
Well-definedness follows from the triangle inequality of the integral and the monotony of the integral:
|
∫
U
φ
(
x
)
f
(
x
)
d
x
|
≤
∫
U
|
φ
(
x
)
f
(
x
)
|
d
x
=
∫
supp
φ
|
φ
(
x
)
f
(
x
)
|
d
x
≤
∫
supp
φ
‖
φ
‖
∞
|
f
(
x
)
|
d
x
=
‖
φ
‖
∞
∫
supp
φ
|
f
(
x
)
|
d
x
<
∞
{\displaystyle {\begin{aligned}\left|\int _{U}\varphi (x)f(x)dx\right|\leq \int _{U}|\varphi (x)f(x)|dx=\int _{{\text{supp }}\varphi }|\varphi (x)f(x)|dx\\\leq \int _{{\text{supp }}\varphi }\|\varphi \|_{\infty }|f(x)|dx=\|\varphi \|_{\infty }\int _{{\text{supp }}\varphi }|f(x)|dx<\infty \end{aligned}}}
In order to have an absolute value strictly less than infinity, the first integral must have a well-defined value in the first place. Therefore,
T
f
{\displaystyle {\mathcal {T}}_{f}}
R
{\displaystyle \mathbb {R} }
Continuity follows similarly due to
|
T
f
φ
l
−
T
f
φ
|
=
|
∫
K
(
φ
l
−
φ
)
(
x
)
f
(
x
)
d
x
|
≤
‖
φ
l
−
φ
‖
∞
∫
K
|
f
(
x
)
|
d
x
⏟
independent of
l
→
0
,
l
→
∞
{\displaystyle |T_{f}\varphi _{l}-T_{f}\varphi |=\left|\int _{K}(\varphi _{l}-\varphi )(x)f(x)dx\right|\leq \|\varphi _{l}-\varphi \|_{\infty }\underbrace {\int _{K}|f(x)|dx} _{{\text{independent of }}l}\to 0,l\to \infty }
, where
K
{\displaystyle K}
φ
l
,
l
∈
N
{\displaystyle \varphi _{l},l\in \mathbb {N} }
φ
{\displaystyle \varphi }
φ
l
,
l
∈
N
{\displaystyle \varphi _{l},l\in \mathbb {N} }
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
φ
{\displaystyle \varphi }
K
{\displaystyle K}
Linearity follows due to the linearity of the integral.
2.
We show that
T
f
{\displaystyle {\mathcal {T}}_{f}}
f
∈
L
loc
1
(
O
)
{\displaystyle f\in L_{\text{loc}}^{1}(O)}
T
f
(
φ
)
{\displaystyle {\mathcal {T}}_{f}(\varphi )}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
f
∈
L
loc
1
(
O
)
{\displaystyle f\in L_{\text{loc}}^{1}(O)}
f
∈
L
loc
1
(
O
)
{\displaystyle f\in L_{\text{loc}}^{1}(O)}
T
f
{\displaystyle {\mathcal {T}}_{f}}
D
∗
(
O
)
{\displaystyle {\mathcal {D}}^{*}(O)}
T
f
(
φ
)
{\displaystyle {\mathcal {T}}_{f}(\varphi )}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
T
f
{\displaystyle {\mathcal {T}}_{f}}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
Let
K
⊂
U
{\displaystyle K\subset U}
μ
:
K
→
R
,
μ
(
ξ
)
:=
inf
x
∈
R
d
∖
O
‖
ξ
−
x
‖
{\displaystyle \mu :K\to \mathbb {R} ,\mu (\xi ):=\inf _{x\in \mathbb {R} ^{d}\setminus O}\|\xi -x\|}
μ
{\displaystyle \mu }
1
{\displaystyle 1}
ξ
,
ι
∈
R
d
{\displaystyle \xi ,\iota \in \mathbb {R} ^{d}}
∀
(
x
,
y
)
∈
R
2
:
‖
ξ
−
x
‖
≤
‖
ξ
−
ι
‖
+
‖
ι
−
y
‖
+
‖
y
−
x
‖
(
∗
)
{\displaystyle \forall (x,y)\in \mathbb {R} ^{2}:\|\xi -x\|\leq \|\xi -\iota \|+\|\iota -y\|+\|y-x\|~~~~~(*)}
and
∀
(
x
,
y
)
∈
R
2
:
‖
ι
−
y
‖
≤
‖
ι
−
ξ
‖
+
‖
ξ
−
x
‖
+
‖
x
−
y
‖
(
∗
∗
)
{\displaystyle \forall (x,y)\in \mathbb {R} ^{2}:\|\iota -y\|\leq \|\iota -\xi \|+\|\xi -x\|+\|x-y\|~~~~~(**)}
, which can be seen by applying the triangle inequality twice.
We choose sequences
(
x
l
)
l
∈
N
{\displaystyle (x_{l})_{l\in \mathbb {N} }}
(
y
m
)
m
∈
N
{\displaystyle (y_{m})_{m\in \mathbb {N} }}
R
d
∖
O
{\displaystyle \mathbb {R} ^{d}\setminus O}
lim
l
→
∞
‖
ξ
−
x
l
‖
=
μ
(
ξ
)
{\displaystyle \lim _{l\to \infty }\|\xi -x_{l}\|=\mu (\xi )}
lim
m
→
∞
‖
ι
−
y
m
‖
=
μ
(
ι
)
{\displaystyle \lim _{m\to \infty }\|\iota -y_{m}\|=\mu (\iota )}
μ
(
ξ
)
≥
μ
(
ι
)
{\displaystyle \mu (\xi )\geq \mu (\iota )}
|
μ
(
ξ
)
−
μ
(
ι
)
|
=
μ
(
ξ
)
−
μ
(
ι
)
=
inf
x
∈
R
d
∖
O
‖
ξ
−
x
‖
−
inf
y
∈
R
d
∖
O
‖
ι
−
y
‖
=
inf
x
∈
R
d
∖
O
‖
ξ
−
x
‖
−
lim
m
→
∞
‖
ι
−
y
m
‖
=
lim
m
→
∞
inf
x
∈
R
d
∖
O
(
‖
ξ
−
x
‖
−
‖
ι
−
y
m
‖
)
≤
lim
m
→
∞
inf
x
∈
R
d
∖
O
(
‖
ξ
−
ι
‖
+
‖
x
−
y
m
‖
)
(
∗
)
with
y
=
y
m
=
‖
ξ
−
ι
‖
{\displaystyle {\begin{aligned}|\mu (\xi )-\mu (\iota )|&=\mu (\xi )-\mu (\iota )&\\&=\inf _{x\in \mathbb {R} ^{d}\setminus O}\|\xi -x\|-\inf _{y\in \mathbb {R} ^{d}\setminus O}\|\iota -y\|&\\&=\inf _{x\in \mathbb {R} ^{d}\setminus O}\|\xi -x\|-\lim _{m\to \infty }\|\iota -y_{m}\|&\\&=\lim _{m\to \infty }\inf _{x\in \mathbb {R} ^{d}\setminus O}\left(\|\xi -x\|-\|\iota -y_{m}\|\right)&\\&\leq \lim _{m\to \infty }\inf _{x\in \mathbb {R} ^{d}\setminus O}\left(\|\xi -\iota \|+\|x-y_{m}\|\right)&(*){\text{ with }}y=y_{m}\\&=\|\xi -\iota \|&\end{aligned}}}
Second, we consider what happens if
μ
(
ξ
)
≤
μ
(
ι
)
{\displaystyle \mu (\xi )\leq \mu (\iota )}
|
μ
(
ξ
)
−
μ
(
ι
)
|
=
μ
(
ι
)
−
μ
(
ξ
)
=
inf
y
∈
R
d
∖
O
‖
ι
−
y
‖
−
inf
x
∈
R
d
∖
O
‖
ξ
−
x
‖
=
inf
y
∈
R
d
∖
O
‖
ι
−
y
‖
−
lim
l
→
∞
‖
ξ
−
x
l
‖
=
lim
l
→
∞
inf
y
∈
R
d
∖
O
(
‖
ι
−
y
‖
−
‖
ξ
−
x
l
‖
)
≤
lim
l
→
∞
inf
y
∈
R
d
∖
O
(
‖
ξ
−
ι
‖
+
‖
y
−
x
l
‖
)
(
∗
∗
)
with
x
=
x
l
=
‖
ξ
−
ι
‖
{\displaystyle {\begin{aligned}|\mu (\xi )-\mu (\iota )|&=\mu (\iota )-\mu (\xi )&\\&=\inf _{y\in \mathbb {R} ^{d}\setminus O}\|\iota -y\|-\inf _{x\in \mathbb {R} ^{d}\setminus O}\|\xi -x\|&\\&=\inf _{y\in \mathbb {R} ^{d}\setminus O}\|\iota -y\|-\lim _{l\to \infty }\|\xi -x_{l}\|&\\&=\lim _{l\to \infty }\inf _{y\in \mathbb {R} ^{d}\setminus O}\left(\|\iota -y\|-\|\xi -x_{l}\|\right)&\\&\leq \lim _{l\to \infty }\inf _{y\in \mathbb {R} ^{d}\setminus O}\left(\|\xi -\iota \|+\|y-x_{l}\|\right)&(**){\text{ with }}x=x_{l}\\&=\|\xi -\iota \|&\end{aligned}}}
Since always either
μ
(
ξ
)
≥
μ
(
ι
)
{\displaystyle \mu (\xi )\geq \mu (\iota )}
μ
(
ξ
)
≤
μ
(
ι
)
{\displaystyle \mu (\xi )\leq \mu (\iota )}
μ
{\displaystyle \mu }
κ
∈
R
d
{\displaystyle \kappa \in \mathbb {R} ^{d}}
μ
(
κ
)
=
0
{\displaystyle \mu (\kappa )=0}
‖
ξ
−
x
l
‖
→
0
,
l
→
∞
{\displaystyle \|\xi -x_{l}\|\to 0,l\to \infty }
(
x
l
)
l
∈
N
{\displaystyle (x_{l})_{l\in \mathbb {N} }}
R
d
∖
O
{\displaystyle \mathbb {R} ^{d}\setminus O}
R
d
∖
O
{\displaystyle \mathbb {R} ^{d}\setminus O}
κ
∈
K
⊂
O
{\displaystyle \kappa \in K\subset O}
μ
(
κ
)
>
0
{\displaystyle \mu (\kappa )>0}
Hence, if we define
δ
:=
μ
(
κ
)
{\displaystyle \delta :=\mu (\kappa )}
δ
>
0
{\displaystyle \delta >0}
ϑ
:
R
d
→
R
,
ϑ
(
x
)
:=
(
χ
K
+
B
δ
/
4
(
0
)
∗
η
δ
/
4
)
(
x
)
=
∫
R
d
η
δ
/
4
(
y
)
χ
K
+
B
δ
/
4
(
0
)
(
x
−
y
)
d
y
=
∫
B
δ
/
4
(
0
)
η
δ
/
4
(
y
)
χ
K
+
B
δ
/
4
(
0
)
(
x
−
y
)
d
y
{\displaystyle \vartheta :\mathbb {R} ^{d}\to \mathbb {R} ,\vartheta (x):=(\chi _{K+B_{\delta /4}(0)}*\eta _{\delta /4})(x)=\int _{\mathbb {R} ^{d}}\eta _{\delta /4}(y)\chi _{K+B_{\delta /4}(0)}(x-y)dy=\int _{B_{\delta /4}(0)}\eta _{\delta /4}(y)\chi _{K+B_{\delta /4}(0)}(x-y)dy}
has support contained in
O
{\displaystyle O}
1
{\displaystyle 1}
K
{\displaystyle K}
C
∞
(
R
d
)
{\displaystyle {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
∫
K
|
f
(
x
)
|
d
x
=
∫
O
|
f
(
x
)
|
χ
K
(
x
)
d
x
≤
∫
R
d
|
f
(
x
)
|
ϑ
(
x
)
d
x
{\displaystyle \int _{K}|f(x)|dx=\int _{O}|f(x)|\chi _{K}(x)dx\leq \int _{\mathbb {R} ^{d}}|f(x)|\vartheta (x)dx}
,
f
{\displaystyle f}
◻
{\displaystyle \Box }
Theorem 4.12 :
Let
f
∈
L
2
(
R
d
)
{\displaystyle f\in L^{2}(\mathbb {R} ^{d})}
∫
R
d
|
f
(
x
)
|
2
d
x
<
∞
{\displaystyle \int _{\mathbb {R} ^{d}}|f(x)|^{2}dx<\infty }
Then
T
f
:
S
(
R
d
)
→
R
,
T
f
(
ϕ
)
:=
∫
R
d
f
(
x
)
ϕ
(
x
)
d
x
{\displaystyle {\mathcal {T}}_{f}:{\mathcal {S}}(\mathbb {R} ^{d})\to \mathbb {R} ,{\mathcal {T}}_{f}(\phi ):=\int _{\mathbb {R} ^{d}}f(x)\phi (x)dx}
is a regular tempered distribution.
Proof :
From Hölder's inequality we obtain
∫
R
d
|
ϕ
(
x
)
|
|
f
(
x
)
|
d
x
≤
‖
ϕ
‖
L
2
‖
f
‖
L
2
<
∞
{\displaystyle \int _{\mathbb {R} ^{d}}|\phi (x)||f(x)|dx\leq \|\phi \|_{L^{2}}\|f\|_{L^{2}}<\infty }
Hence,
T
f
{\displaystyle {\mathcal {T}}_{f}}
Due to the triangle inequality for integrals and Hölder's inequality, we have
|
T
f
(
ϕ
l
)
−
T
f
(
ϕ
)
|
≤
∫
R
d
|
(
ϕ
l
−
ϕ
)
(
x
)
|
|
f
(
x
)
|
d
x
≤
‖
ϕ
l
−
ϕ
‖
L
2
‖
f
‖
L
2
{\displaystyle |T_{f}(\phi _{l})-T_{f}(\phi )|\leq \int _{\mathbb {R} ^{d}}|(\phi _{l}-\phi )(x)||f(x)|dx\leq \|\phi _{l}-\phi \|_{L^{2}}\|f\|_{L^{2}}}
Furthermore
‖
ϕ
l
−
ϕ
‖
L
2
2
≤
‖
ϕ
l
−
ϕ
‖
∞
∫
R
d
|
(
ϕ
l
−
ϕ
)
(
x
)
|
d
x
=
‖
ϕ
l
−
ϕ
‖
∞
∫
R
d
∏
j
=
1
d
(
1
+
x
j
2
)
|
(
ϕ
l
−
ϕ
)
(
x
)
|
1
∏
j
=
1
d
(
1
+
x
j
2
)
d
x
≤
‖
ϕ
l
−
ϕ
‖
∞
‖
∏
j
=
1
d
(
1
+
x
j
2
)
(
ϕ
l
−
ϕ
)
‖
∞
∫
R
d
1
∏
j
=
1
d
(
1
+
x
j
2
)
d
x
⏟
=
π
d
{\displaystyle {\begin{aligned}\|\phi _{l}-\phi \|_{L^{2}}^{2}&\leq \|\phi _{l}-\phi \|_{\infty }\int _{\mathbb {R} ^{d}}|(\phi _{l}-\phi )(x)|dx\\&=\|\phi _{l}-\phi \|_{\infty }\int _{\mathbb {R} ^{d}}\prod _{j=1}^{d}(1+x_{j}^{2})|(\phi _{l}-\phi )(x)|{\frac {1}{\prod _{j=1}^{d}(1+x_{j}^{2})}}dx\\&\leq \|\phi _{l}-\phi \|_{\infty }\left\|\prod _{j=1}^{d}(1+x_{j}^{2})(\phi _{l}-\phi )\right\|_{\infty }\underbrace {\int _{\mathbb {R} ^{d}}{\frac {1}{\prod _{j=1}^{d}(1+x_{j}^{2})}}dx} _{=\pi ^{d}}\end{aligned}}}
If
ϕ
l
→
ϕ
{\displaystyle \phi _{l}\to \phi }
Linearity follows from the linearity of the integral.
◻
{\displaystyle \Box }
We now introduce the concept of equicontinuity.
So equicontinuity is in fact defined for sets of continuous functions mapping from
X
{\displaystyle X}
R
{\displaystyle \mathbb {R} }
Proof :
In order to prove uniform convergence, by definition we must prove that for all
ϵ
>
0
{\displaystyle \epsilon >0}
N
∈
N
{\displaystyle N\in \mathbb {N} }
l
≥
N
:
∀
x
∈
Q
:
|
f
l
(
x
)
−
f
(
x
)
|
<
ϵ
{\displaystyle l\geq N:\forall x\in Q:|f_{l}(x)-f(x)|<\epsilon }
So let's assume the contrary, which equals by negating the logical statement
∃
ϵ
>
0
:
∀
N
∈
N
:
∃
l
≥
N
:
∃
x
∈
Q
:
|
f
l
(
x
)
−
f
(
x
)
|
≥
ϵ
{\displaystyle \exists \epsilon >0:\forall N\in \mathbb {N} :\exists l\geq N:\exists x\in Q:|f_{l}(x)-f(x)|\geq \epsilon }
We choose a sequence
(
x
m
)
m
∈
N
{\displaystyle (x_{m})_{m\in \mathbb {N} }}
Q
{\displaystyle Q}
x
1
{\displaystyle x_{1}}
Q
{\displaystyle Q}
|
f
l
1
(
x
1
)
−
f
(
x
1
)
|
≥
ϵ
{\displaystyle |f_{l_{1}}(x_{1})-f(x_{1})|\geq \epsilon }
l
1
∈
N
{\displaystyle l_{1}\in \mathbb {N} }
x
k
{\displaystyle x_{k}}
l
k
{\displaystyle l_{k}}
k
∈
{
1
,
…
,
m
}
{\displaystyle k\in \{1,\ldots ,m\}}
x
m
+
1
{\displaystyle x_{m+1}}
|
f
l
m
+
1
(
x
m
+
1
)
−
f
(
x
m
+
1
)
|
≥
ϵ
{\displaystyle |f_{l_{m+1}}(x_{m+1})-f(x_{m+1})|\geq \epsilon }
l
m
+
1
{\displaystyle l_{m+1}}
l
m
{\displaystyle l_{m}}
As
Q
{\displaystyle Q}
(
x
m
j
)
j
∈
N
{\displaystyle (x_{m_{j}})_{j\in \mathbb {N} }}
(
x
m
)
m
∈
N
{\displaystyle (x_{m})_{m\in \mathbb {N} }}
x
{\displaystyle x}
As
Q
{\displaystyle {\mathcal {Q}}}
δ
∈
R
>
0
{\displaystyle \delta \in \mathbb {R} _{>0}}
‖
x
−
y
‖
<
δ
⇒
∀
f
∈
Q
:
|
f
(
x
)
−
f
(
y
)
|
<
ϵ
4
{\displaystyle \|x-y\|<\delta \Rightarrow \forall f\in {\mathcal {Q}}:|f(x)-f(y)|<{\frac {\epsilon }{4}}}
Further, since
x
m
j
→
x
{\displaystyle x_{m_{j}}\to x}
j
→
∞
{\displaystyle j\to \infty }
J
∈
N
{\displaystyle J\in \mathbb {N} }
∀
j
≥
J
:
‖
x
m
j
−
x
‖
<
δ
{\displaystyle \forall j\geq J:\|x_{m_{j}}-x\|<\delta }
But then follows for
j
≥
J
{\displaystyle j\geq J}
reverse triangle inequality :
|
f
l
m
j
(
x
)
−
f
(
x
)
|
≥
|
|
f
l
m
j
(
x
)
−
f
(
x
m
j
)
|
−
|
f
(
x
m
j
)
−
f
(
x
)
|
|
{\displaystyle |f_{l_{m_{j}}}(x)-f(x)|\geq \left||f_{l_{m_{j}}}(x)-f(x_{m_{j}})|-|f(x_{m_{j}})-f(x)|\right|}
Since we had
|
f
(
x
m
j
)
−
f
(
x
)
|
<
ϵ
4
{\displaystyle |f(x_{m_{j}})-f(x)|<{\frac {\epsilon }{4}}}
|
f
l
m
j
(
x
)
−
f
(
x
m
j
)
|
≥
|
|
f
l
m
j
(
x
m
j
)
−
f
(
x
m
j
)
|
−
|
f
l
m
j
(
x
)
−
f
l
m
j
(
x
m
j
)
|
|
≥
ϵ
−
ϵ
4
{\displaystyle |f_{l_{m_{j}}}(x)-f(x_{m_{j}})|\geq \left||f_{l_{m_{j}}}(x_{m_{j}})-f(x_{m_{j}})|-|f_{l_{m_{j}}}(x)-f_{l_{m_{j}}}(x_{m_{j}})|\right|\geq \epsilon -{\frac {\epsilon }{4}}}
, we obtain:
|
f
l
m
j
(
x
)
−
f
(
x
)
|
≥
|
|
f
l
m
j
(
x
)
−
f
(
x
m
j
)
|
−
|
f
(
x
m
j
)
−
f
(
x
)
|
|
=
|
f
l
m
j
(
x
)
−
f
(
x
m
j
)
|
−
|
f
(
x
m
j
)
−
f
(
x
)
|
≥
ϵ
−
ϵ
4
−
ϵ
4
≥
ϵ
2
{\displaystyle {\begin{aligned}|f_{l_{m_{j}}}(x)-f(x)|&\geq \left||f_{l_{m_{j}}}(x)-f(x_{m_{j}})|-|f(x_{m_{j}})-f(x)|\right|\\&=|f_{l_{m_{j}}}(x)-f(x_{m_{j}})|-|f(x_{m_{j}})-f(x)|\\&\geq \epsilon -{\frac {\epsilon }{4}}-{\frac {\epsilon }{4}}\\&\geq {\frac {\epsilon }{2}}\end{aligned}}}
Thus we have a contradiction to
f
l
(
x
)
→
f
(
x
)
{\displaystyle f_{l}(x)\to f(x)}
◻
{\displaystyle \Box }
Proof : We have to prove equicontinuity, so we have to prove
∀
x
∈
X
:
∃
δ
∈
R
>
0
:
∀
y
∈
X
:
‖
x
−
y
‖
<
δ
⇒
∀
f
∈
Q
:
|
f
(
x
)
−
f
(
y
)
|
<
ϵ
{\displaystyle \forall x\in X:\exists \delta \in \mathbb {R} _{>0}:\forall y\in X:\|x-y\|<\delta \Rightarrow \forall f\in {\mathcal {Q}}:|f(x)-f(y)|<\epsilon }
Let
x
∈
X
{\displaystyle x\in X}
We choose
δ
:=
ϵ
b
{\displaystyle \delta :={\frac {\epsilon }{b}}}
Let
y
∈
X
{\displaystyle y\in X}
‖
x
−
y
‖
<
δ
{\displaystyle \|x-y\|<\delta }
f
∈
Q
{\displaystyle f\in {\mathcal {Q}}}
mean-value theorem in multiple dimensions , we obtain that there exists a
λ
∈
[
0
,
1
]
{\displaystyle \lambda \in [0,1]}
f
(
x
)
−
f
(
y
)
=
∇
f
(
λ
x
+
(
1
−
λ
)
y
)
⋅
(
x
−
y
)
{\displaystyle f(x)-f(y)=\nabla f(\lambda x+(1-\lambda )y)\cdot (x-y)}
The element
λ
x
+
(
1
−
λ
)
y
{\displaystyle \lambda x+(1-\lambda )y}
X
{\displaystyle X}
X
{\displaystyle X}
Cauchy-Schwarz inequality then follows:
|
f
(
x
)
−
f
(
y
)
|
=
|
∇
f
(
λ
x
+
(
1
−
λ
)
y
)
⋅
(
x
−
y
)
|
≤
‖
∇
f
(
λ
x
+
(
1
−
λ
)
y
)
‖
‖
x
−
y
‖
<
b
δ
=
b
b
ϵ
=
ϵ
{\displaystyle |f(x)-f(y)|=|\nabla f(\lambda x+(1-\lambda )y)\cdot (x-y)|\leq \|\nabla f(\lambda x+(1-\lambda )y)\|\|x-y\|<b\delta ={\frac {b}{b}}\epsilon =\epsilon }
◻
{\displaystyle \Box }
Definition 4.16 :
If
α
=
(
α
1
,
…
,
α
d
)
,
β
=
(
β
1
,
…
,
β
d
)
∈
N
0
d
{\displaystyle \alpha =(\alpha _{1},\ldots ,\alpha _{d}),\beta =(\beta _{1},\ldots ,\beta _{d})\in \mathbb {N} _{0}^{d}}
d
{\displaystyle d}
binomial coefficient of
α
{\displaystyle \alpha }
β
{\displaystyle \beta }
as
(
α
β
)
:=
(
α
1
β
1
)
(
α
2
β
2
)
⋯
(
α
d
β
d
)
{\displaystyle {\binom {\alpha }{\beta }}:={\binom {\alpha _{1}}{\beta _{1}}}{\binom {\alpha _{2}}{\beta _{2}}}\cdots {\binom {\alpha _{d}}{\beta _{d}}}}
We also define less or equal relation on the set of multi-indices.
Definition 4.17 :
Let
α
=
(
α
1
,
…
,
α
d
)
,
β
=
(
β
1
,
…
,
β
d
)
∈
N
0
d
{\displaystyle \alpha =(\alpha _{1},\ldots ,\alpha _{d}),\beta =(\beta _{1},\ldots ,\beta _{d})\in \mathbb {N} _{0}^{d}}
d
{\displaystyle d}
β
{\displaystyle \beta }
α
{\displaystyle \alpha }
β
≤
α
:⇔
∀
n
∈
{
1
,
…
,
d
}
:
β
n
≤
α
n
{\displaystyle \beta \leq \alpha :\Leftrightarrow \forall n\in \{1,\ldots ,d\}:\beta _{n}\leq \alpha _{n}}
For
d
≥
2
{\displaystyle d\geq 2}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
α
≤
β
{\displaystyle \alpha \leq \beta }
β
≤
α
{\displaystyle \beta \leq \alpha }
d
=
2
{\displaystyle d=2}
α
=
(
1
,
0
)
,
β
=
(
0
,
1
)
{\displaystyle \alpha =(1,0),\beta =(0,1)}
This example can be generalised to higher dimensions (see exercise 6).
With these multiindex definitions, we are able to write down a more general version of the product rule. But in order to prove it, we need another lemma.
Lemma 4.18 :
If
n
∈
{
1
,
…
,
d
}
{\displaystyle n\in \{1,\ldots ,d\}}
e
n
:=
(
0
,
…
,
0
,
1
,
0
,
…
,
0
)
{\displaystyle e_{n}:=(0,\ldots ,0,1,0,\ldots ,0)}
1
{\displaystyle 1}
n
{\displaystyle n}
(
α
−
e
n
β
−
e
n
)
+
(
α
−
e
n
β
)
=
(
α
β
)
{\displaystyle {\binom {\alpha -e_{n}}{\beta -e_{n}}}+{\binom {\alpha -e_{n}}{\beta }}={\binom {\alpha }{\beta }}}
for arbitrary multiindices
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
Proof :
For the ordinary binomial coefficients for natural numbers, we had the formula
(
n
−
1
k
−
1
)
+
(
n
−
1
k
)
=
(
n
k
)
{\displaystyle {\binom {n-1}{k-1}}+{\binom {n-1}{k}}={\binom {n}{k}}}
Therefore,
(
α
−
e
n
β
−
e
n
)
+
(
α
−
e
n
β
)
=
(
α
1
β
1
)
⋯
(
α
n
−
1
β
n
−
1
)
⋯
(
α
d
β
d
)
+
(
α
1
β
1
)
⋯
(
α
n
−
1
β
n
)
⋯
(
α
d
β
d
)
=
(
α
1
β
1
)
⋯
(
(
α
n
−
1
β
n
−
1
)
+
(
α
n
−
1
β
n
)
)
⋯
(
α
d
β
d
)
=
(
α
1
β
1
)
⋯
(
α
n
β
n
)
⋯
(
α
d
β
d
)
=
(
α
β
)
{\displaystyle {\begin{aligned}{\binom {\alpha -e_{n}}{\beta -e_{n}}}+{\binom {\alpha -e_{n}}{\beta }}&={\binom {\alpha _{1}}{\beta _{1}}}\cdots {\binom {\alpha _{n}-1}{\beta _{n}-1}}\cdots {\binom {\alpha _{d}}{\beta _{d}}}+{\binom {\alpha _{1}}{\beta _{1}}}\cdots {\binom {\alpha _{n}-1}{\beta _{n}}}\cdots {\binom {\alpha _{d}}{\beta _{d}}}\\&={\binom {\alpha _{1}}{\beta _{1}}}\cdots \left({\binom {\alpha _{n}-1}{\beta _{n}-1}}+{\binom {\alpha _{n}-1}{\beta _{n}}}\right)\cdots {\binom {\alpha _{d}}{\beta _{d}}}\\&={\binom {\alpha _{1}}{\beta _{1}}}\cdots {\binom {\alpha _{n}}{\beta _{n}}}\cdots {\binom {\alpha _{d}}{\beta _{d}}}={\binom {\alpha }{\beta }}\end{aligned}}}
◻
{\displaystyle \Box }
This is the general product rule:
Theorem 4.19 :
Let
f
∈
C
n
(
R
d
)
{\displaystyle f\in {\mathcal {C}}^{n}(\mathbb {R} ^{d})}
|
α
|
≤
n
{\displaystyle |\alpha |\leq n}
∂
α
(
f
g
)
=
∑
β
≤
α
(
α
β
)
∂
β
f
∂
α
−
β
g
{\displaystyle \partial _{\alpha }(fg)=\sum _{\beta \leq \alpha }{\binom {\alpha }{\beta }}\partial _{\beta }f\partial _{\alpha -\beta }g}
Proof :
We prove the claim by induction over
|
α
|
{\displaystyle |\alpha |}
1.
We start with the induction base
|
α
|
=
0
{\displaystyle |\alpha |=0}
f
(
x
)
g
(
x
)
=
f
(
x
)
g
(
x
)
{\displaystyle f(x)g(x)=f(x)g(x)}
, and this is true. Therefore, we have completed the induction base.
2.
Next, we do the induction step. Let's assume the claim is true for all
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
=
n
{\displaystyle |\alpha |=n}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
=
n
+
1
{\displaystyle |\alpha |=n+1}
k
∈
{
1
,
…
,
d
}
{\displaystyle k\in \{1,\ldots ,d\}}
α
k
>
0
{\displaystyle \alpha _{k}>0}
|
α
|
=
k
+
1
>
0
{\displaystyle |\alpha |=k+1>0}
e
k
=
(
0
,
…
,
0
,
1
,
0
,
…
,
0
)
{\displaystyle e_{k}=(0,\ldots ,0,1,0,\ldots ,0)}
1
{\displaystyle 1}
k
{\displaystyle k}
Schwarz' theorem and the ordinary product rule, we have
∂
α
f
g
=
∂
α
−
e
k
(
∂
x
k
f
g
)
=
∂
α
−
e
k
(
∂
x
k
f
g
+
f
∂
x
k
g
)
{\displaystyle \partial _{\alpha }fg=\partial _{\alpha -e_{k}}\left(\partial _{x_{k}}fg\right)=\partial _{\alpha -e_{k}}\left(\partial _{x_{k}}fg+f\partial _{x_{k}}g\right)}
By linearity of derivatives and induction hypothesis, we have
∂
α
−
e
k
(
∂
x
k
f
g
+
f
∂
x
k
g
)
=
∂
α
−
e
k
(
∂
x
k
f
g
)
+
∂
α
−
e
k
(
f
∂
x
k
g
)
=
∑
ς
≤
α
−
e
k
(
α
−
e
k
ς
)
∂
ς
∂
x
k
f
∂
α
−
e
k
−
ς
g
+
∑
ς
≤
α
−
e
k
(
α
−
e
k
ς
)
∂
ς
f
∂
α
−
e
k
−
ς
∂
x
k
g
{\displaystyle {\begin{aligned}\partial _{\alpha -e_{k}}\left(\partial _{x_{k}}fg+f\partial _{x_{k}}g\right)&=\partial _{\alpha -e_{k}}\left(\partial _{x_{k}}fg\right)+\partial _{\alpha -e_{k}}\left(f\partial _{x_{k}}g\right)\\&=\sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }\partial _{x_{k}}f\partial _{\alpha -e_{k}-\varsigma }g+\sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -e_{k}-\varsigma }\partial _{x_{k}}g\end{aligned}}}
Since
∂
α
−
e
k
−
ς
=
∂
α
−
(
ς
+
e
k
)
{\displaystyle \partial _{\alpha -e_{k}-\varsigma }=\partial _{\alpha -(\varsigma +e_{k})}}
and
{
ς
∈
N
0
d
|
0
≤
ς
≤
α
−
e
k
}
=
{
ς
−
e
k
∈
N
0
d
|
e
k
≤
ς
≤
α
}
{\displaystyle \{\varsigma \in \mathbb {N} _{0}^{d}|0\leq \varsigma \leq \alpha -e_{k}\}=\{\varsigma -e_{k}\in \mathbb {N} _{0}^{d}|e_{k}\leq \varsigma \leq \alpha \}}
we are allowed to shift indices in the first of the two above sums, and furthermore we have by definition
∂
ς
∂
x
k
=
∂
ς
+
e
k
{\displaystyle \partial _{\varsigma }\partial _{x_{k}}=\partial _{\varsigma +e_{k}}}
With this, we obtain
∑
ς
≤
α
−
e
k
(
α
−
e
k
ς
)
∂
ς
∂
x
k
f
∂
α
−
e
k
−
ς
g
+
∑
ς
≤
α
−
e
k
(
α
−
e
k
ς
)
∂
ς
f
∂
α
−
e
k
−
ς
∂
x
k
g
=
∑
e
k
≤
ς
≤
α
(
α
−
e
k
ς
−
e
k
)
∂
ς
f
⋅
∂
α
−
ς
g
+
∑
ς
≤
α
−
e
k
(
α
−
e
k
ς
)
∂
ς
f
∂
α
−
ς
g
{\displaystyle \sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }\partial _{x_{k}}f\partial _{\alpha -e_{k}-\varsigma }g+\sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -e_{k}-\varsigma }\partial _{x_{k}}g=\sum _{e_{k}\leq \varsigma \leq \alpha }{\binom {\alpha -e_{k}}{\varsigma -e_{k}}}\partial _{\varsigma }f\cdot \partial _{\alpha -\varsigma }g+\sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -\varsigma }g}
Due to lemma 4.18,
(
α
−
e
k
β
−
e
i
)
+
(
α
−
e
k
β
)
=
(
α
β
)
{\displaystyle {\binom {\alpha -e_{k}}{\beta -e_{i}}}+{\binom {\alpha -e_{k}}{\beta }}={\binom {\alpha }{\beta }}}
Further, we have
(
α
−
e
i
0
)
=
(
α
0
)
=
1
{\displaystyle {\binom {\alpha -e_{i}}{0}}={\binom {\alpha }{0}}=1}
0
=
(
0
,
…
,
0
)
{\displaystyle 0=(0,\ldots ,0)}
N
0
d
{\displaystyle \mathbb {N} _{0}^{d}}
and
(
α
−
e
k
α
−
e
k
)
=
(
α
α
)
=
1
{\displaystyle {\binom {\alpha -e_{k}}{\alpha -e_{k}}}={\binom {\alpha }{\alpha }}=1}
(these two rules may be checked from the definition of
(
α
β
)
{\displaystyle {\binom {\alpha }{\beta }}}
∂
α
(
f
g
)
=
∑
e
k
≤
ς
≤
α
(
α
−
e
k
ς
−
e
k
)
∂
ς
f
⋅
∂
α
−
ς
g
+
∑
ς
≤
α
−
e
k
(
α
−
e
k
ς
)
∂
ς
f
∂
α
−
ς
g
=
(
α
−
e
k
0
)
f
∂
α
g
+
∑
e
k
≤
ς
≤
α
−
e
k
[
(
α
−
e
k
ς
−
e
k
)
+
(
α
−
e
k
ς
)
]
∂
ς
f
∂
α
−
ς
g
+
(
α
−
e
k
α
−
e
k
)
f
∂
α
g
=
∑
ς
≤
α
(
α
ς
)
∂
ς
f
∂
α
−
ς
{\displaystyle {\begin{aligned}\partial _{\alpha }(fg)&=\sum _{e_{k}\leq \varsigma \leq \alpha }{\binom {\alpha -e_{k}}{\varsigma -e_{k}}}\partial _{\varsigma }f\cdot \partial _{\alpha -\varsigma }g+\sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -\varsigma }g\\&={\binom {\alpha -e_{k}}{0}}f\partial _{\alpha }g+\sum _{e_{k}\leq \varsigma \leq \alpha -e_{k}}\left[{\binom {\alpha -e_{k}}{\varsigma -e_{k}}}+{\binom {\alpha -e_{k}}{\varsigma }}\right]\partial _{\varsigma }f\partial _{\alpha -\varsigma }g+{\binom {\alpha -e_{k}}{\alpha -e_{k}}}f\partial _{\alpha }g\\&=\sum _{\varsigma \leq \alpha }{\binom {\alpha }{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -\varsigma }\end{aligned}}}
◻
{\displaystyle \Box }
For
φ
,
ϑ
∈
D
(
R
d
)
{\displaystyle \varphi ,\vartheta \in {\mathcal {D}}(\mathbb {R} ^{d})}
φ
{\displaystyle \varphi }
φ
{\displaystyle \varphi }
ϑ
{\displaystyle \vartheta }
φ
{\displaystyle \varphi }
ϑ
{\displaystyle \vartheta }
ϑ
{\displaystyle \vartheta }
ϑ
{\displaystyle \vartheta }
T
{\displaystyle {\mathcal {T}}}
φ
{\displaystyle \varphi }
Proof :
We have to prove two claims: First, that the function
φ
↦
T
(
L
(
φ
)
)
{\displaystyle \varphi \mapsto {\mathcal {T}}({\mathcal {L}}(\varphi ))}
Λ
{\displaystyle \Lambda }
∀
φ
∈
D
(
O
)
:
Λ
(
T
φ
)
=
T
L
(
φ
)
{\displaystyle \forall \varphi \in {\mathcal {D}}(O):\Lambda ({\mathcal {T}}_{\varphi })={\mathcal {T}}_{L(\varphi )}}
1.
We show that the function
φ
↦
T
(
L
(
φ
)
)
{\displaystyle \varphi \mapsto {\mathcal {T}}({\mathcal {L}}(\varphi ))}
T
(
L
(
φ
)
)
{\displaystyle {\mathcal {T}}({\mathcal {L}}(\varphi ))}
R
{\displaystyle \mathbb {R} }
L
{\displaystyle {\mathcal {L}}}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
T
{\displaystyle {\mathcal {T}}}
φ
↦
T
(
L
(
φ
)
)
{\displaystyle \varphi \mapsto {\mathcal {T}}({\mathcal {L}}(\varphi ))}
2.
We show that
Λ
{\displaystyle \Lambda }
∀
φ
∈
D
(
O
)
:
Λ
(
T
φ
)
=
T
L
(
φ
)
{\displaystyle \forall \varphi \in {\mathcal {D}}(O):\Lambda ({\mathcal {T}}_{\varphi })={\mathcal {T}}_{L(\varphi )}}
For every
ϑ
∈
D
(
U
)
{\displaystyle \vartheta \in {\mathcal {D}}(U)}
Λ
(
T
φ
)
(
ϑ
)
:=
(
T
φ
∘
L
)
(
ϑ
)
:=
∫
O
φ
(
x
)
L
(
ϑ
)
(
x
)
d
x
=
by assumption
∫
U
L
(
φ
)
(
x
)
ϑ
(
x
)
d
x
=:
T
L
(
φ
)
(
ϑ
)
{\displaystyle \Lambda ({\mathcal {T}}_{\varphi })(\vartheta ):=({\mathcal {T}}_{\varphi }\circ {\mathcal {L}})(\vartheta ):=\int _{O}\varphi (x){\mathcal {L}}(\vartheta )(x)dx{\overset {\text{by assumption}}{=}}\int _{U}L(\varphi )(x)\vartheta (x)dx=:{\mathcal {T}}_{L(\varphi )}(\vartheta )}
Since equality of two functions is equivalent to equality of these two functions evaluated at every point, this shows the desired property.
◻
{\displaystyle \Box }
We also have a similar lemma for Schwartz distributions:
The proof is exactly word-for-word the same as the one for lemma 4.20.
Noting that multiplication, differentiation and convolution are linear, we will define these operations for distributions by taking
L
{\displaystyle L}
Proof:
The product of two
C
∞
{\displaystyle {\mathcal {C}}^{\infty }}
C
∞
{\displaystyle {\mathcal {C}}^{\infty }}
φ
(
x
)
=
0
{\displaystyle \varphi (x)=0}
(
f
φ
)
(
x
)
=
f
(
x
)
φ
(
x
)
=
0
{\displaystyle (f\varphi )(x)=f(x)\varphi (x)=0}
f
φ
∈
D
(
O
)
{\displaystyle f\varphi \in {\mathcal {D}}(O)}
Also, if
φ
l
→
φ
{\displaystyle \varphi _{l}\to \varphi }
K
⊂
R
d
{\displaystyle K\subset \mathbb {R} ^{d}}
supp
φ
n
⊆
K
{\displaystyle {\text{supp }}\varphi _{n}\subseteq K}
n
∈
N
{\displaystyle n\in \mathbb {N} }
‖
∂
α
(
f
(
φ
l
−
φ
)
)
‖
∞
=
‖
∑
ς
≤
α
(
α
ς
)
∂
ς
f
∂
α
−
ς
(
φ
l
−
φ
)
‖
∞
≤
∑
ς
≤
α
‖
∂
ς
f
∂
α
−
ς
(
φ
l
−
φ
)
‖
∞
≤
∑
ς
≤
α
max
x
∈
K
|
∂
ς
f
|
‖
∂
α
−
ς
(
φ
l
−
φ
)
‖
∞
→
0
,
l
→
∞
{\displaystyle {\begin{aligned}\|\partial _{\alpha }(f(\varphi _{l}-\varphi ))\|_{\infty }&=\left\|\sum _{\varsigma \leq \alpha }{\binom {\alpha }{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -\varsigma }(\varphi _{l}-\varphi )\right\|_{\infty }\\&\leq \sum _{\varsigma \leq \alpha }\|\partial _{\varsigma }f\partial _{\alpha -\varsigma }(\varphi _{l}-\varphi )\|_{\infty }\\&\leq \sum _{\varsigma \leq \alpha }\max _{x\in K}|\partial _{\varsigma }f|\|\partial _{\alpha -\varsigma }(\varphi _{l}-\varphi )\|_{\infty }\to 0,l\to \infty \end{aligned}}}
Hence,
f
φ
l
→
f
φ
{\displaystyle f\varphi _{l}\to f\varphi }
Further, also
f
ϕ
∈
C
∞
(
R
d
)
{\displaystyle f\phi \in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
∂
β
f
ϕ
=
∑
ς
≤
β
(
β
ς
)
∂
ς
f
∂
β
−
ς
ϕ
{\displaystyle \partial _{\beta }f\phi =\sum _{\varsigma \leq \beta }{\binom {\beta }{\varsigma }}\partial _{\varsigma }f\partial _{\beta -\varsigma }\phi }
Since all the derivatives of
f
{\displaystyle f}
∀
x
∈
R
d
:
|
∂
ς
f
(
x
)
|
≤
|
p
ς
(
x
)
|
{\displaystyle \forall x\in \mathbb {R} ^{d}:|\partial _{\varsigma }f(x)|\leq |p_{\varsigma }(x)|}
, where
p
ς
,
ς
∈
N
0
d
{\displaystyle p_{\varsigma },\varsigma \in \mathbb {N} _{0}^{d}}
‖
x
α
∂
β
f
ϕ
‖
∞
≤
∑
ς
≤
β
‖
x
α
p
ς
∂
β
−
ς
ϕ
‖
∞
<
∞
{\displaystyle \|x^{\alpha }\partial _{\beta }f\phi \|_{\infty }\leq \sum _{\varsigma \leq \beta }\|x^{\alpha }p_{\varsigma }\partial _{\beta -\varsigma }\phi \|_{\infty }<\infty }
Similarly, if
ϕ
l
→
ϕ
{\displaystyle \phi _{l}\to \phi }
‖
x
α
∂
β
f
(
ϕ
−
ϕ
l
)
‖
∞
≤
∑
ς
≤
β
‖
x
α
p
ς
∂
β
−
ς
(
ϕ
−
ϕ
l
)
‖
∞
→
0
,
l
→
∞
{\displaystyle \|x^{\alpha }\partial _{\beta }f(\phi -\phi _{l})\|_{\infty }\leq \sum _{\varsigma \leq \beta }\|x^{\alpha }p_{\varsigma }\partial _{\beta -\varsigma }(\phi -\phi _{l})\|_{\infty }\to 0,l\to \infty }
and hence
f
ϕ
l
→
f
ϕ
{\displaystyle f\phi _{l}\to f\phi }
If we define
L
(
φ
)
:=
L
(
φ
)
:=
f
φ
{\displaystyle L(\varphi ):={\mathcal {L}}(\varphi ):=f\varphi }
◻
{\displaystyle \Box }
Theorem and definitions 4.23 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
L
:
S
(
R
d
)
→
C
∞
(
R
d
)
,
L
(
ϕ
)
:=
∑
α
∈
N
0
d
a
α
∂
α
ϕ
{\displaystyle L:{\mathcal {S}}(\mathbb {R} ^{d})\to {\mathcal {C}}^{\infty }(\mathbb {R} ^{d}),L(\phi ):=\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }\phi }
, where
a
α
∈
C
∞
(
R
d
)
{\displaystyle a_{\alpha }\in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
a
α
{\displaystyle a_{\alpha }}
linear partial differential operator ), and further we define
L
:
S
(
R
d
)
→
C
∞
(
R
d
)
,
L
(
ϕ
)
:=
∑
|
α
|
≤
k
(
−
1
)
|
α
|
∂
α
(
a
α
ϕ
)
{\displaystyle {\mathcal {L}}:{\mathcal {S}}(\mathbb {R} ^{d})\to {\mathcal {C}}^{\infty }(\mathbb {R} ^{d}),{\mathcal {L}}(\phi ):=\sum _{|\alpha |\leq k}(-1)^{|\alpha |}\partial _{\alpha }(a_{\alpha }\phi )}
Let
T
:
D
(
O
)
→
R
{\displaystyle {\mathcal {T}}:{\mathcal {D}}(O)\to \mathbb {R} }
∑
α
∈
N
0
d
a
α
∂
α
T
:
D
(
O
)
→
R
,
∑
α
∈
N
0
d
a
α
∂
α
T
(
φ
)
:=
T
(
L
(
φ
)
)
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}:{\mathcal {D}}(O)\to \mathbb {R} ,\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}(\varphi ):={\mathcal {T}}({\mathcal {L}}(\varphi ))}
then the expression on the right hand side is well-defined, for all
ϑ
∈
D
(
O
)
{\displaystyle \vartheta \in {\mathcal {D}}(O)}
∑
α
∈
N
0
d
a
α
∂
α
T
ϑ
=
T
L
(
ϑ
)
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}_{\vartheta }={\mathcal {T}}_{L(\vartheta )}}
and
∑
α
∈
N
0
d
a
α
∂
α
T
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}}
Assume that all
a
α
{\displaystyle a_{\alpha }}
T
:
S
(
R
d
)
→
R
{\displaystyle {\mathcal {T}}:{\mathcal {S}}(\mathbb {R} ^{d})\to \mathbb {R} }
∑
α
∈
N
0
d
a
α
∂
α
T
:
D
(
O
)
→
R
,
∑
α
∈
N
0
d
a
α
∂
α
T
(
φ
)
:=
T
(
L
(
φ
)
)
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}:{\mathcal {D}}(O)\to \mathbb {R} ,\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}(\varphi ):={\mathcal {T}}({\mathcal {L}}(\varphi ))}
then the expression on the right hand side is well-defined, for all
ϑ
∈
D
(
O
)
{\displaystyle \vartheta \in {\mathcal {D}}(O)}
∑
α
∈
N
0
d
a
α
∂
α
T
ϑ
=
T
L
(
ϑ
)
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}_{\vartheta }={\mathcal {T}}_{L(\vartheta )}}
and
∑
α
∈
N
0
d
a
α
∂
α
T
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}}
Proof :
We want to apply lemmas 4.20 and 4.21. Hence, we prove that the requirements of these lemmas are met.
Since the derivatives of bump functions are again bump functions, the derivatives of Schwartz functions are again Schwartz functions (see exercise 3.3 for both), and because of theorem 4.22, we have that
L
{\displaystyle L}
L
{\displaystyle {\mathcal {L}}}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
D
(
O
)
{\displaystyle {\mathcal {D}}(O)}
a
α
{\displaystyle a_{\alpha }}
L
{\displaystyle L}
L
{\displaystyle {\mathcal {L}}}
S
(
R
d
)
{\displaystyle {\mathcal {S}}(\mathbb {R} ^{d})}
S
(
R
d
)
{\displaystyle {\mathcal {S}}(\mathbb {R} ^{d})}
The sequential continuity of
L
{\displaystyle {\mathcal {L}}}
Further, for all
ϕ
,
θ
∈
S
(
R
d
)
{\displaystyle \phi ,\theta \in {\mathcal {S}}(\mathbb {R} ^{d})}
∫
R
d
ϕ
(
x
)
L
(
θ
)
(
x
)
d
x
=
∑
α
∈
N
0
d
(
−
1
)
|
α
|
∫
R
d
ϕ
(
x
)
∂
α
(
a
α
θ
)
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}\phi (x){\mathcal {L}}(\theta )(x)dx=\sum _{\alpha \in \mathbb {N} _{0}^{d}}(-1)^{|\alpha |}\int _{\mathbb {R} ^{d}}\phi (x)\partial _{\alpha }(a_{\alpha }\theta )(x)dx}
Further, if we single out an
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
Fubini's theorem and integration by parts we obtain
∫
R
d
ϕ
(
x
)
∂
α
(
a
α
θ
)
(
x
)
d
x
=
∫
R
d
−
1
∫
R
ϕ
(
x
)
∂
α
(
a
α
θ
)
(
x
)
d
x
1
d
(
x
2
,
…
,
x
d
)
=
∫
R
d
−
1
∫
R
ϕ
(
x
)
∂
α
(
a
α
θ
)
(
x
)
d
x
1
d
(
x
2
,
…
,
x
d
)
=
∫
R
d
−
1
(
−
1
)
α
1
∫
R
∂
(
α
1
,
0
,
…
,
0
)
ϕ
(
x
)
∂
α
−
(
α
1
,
0
,
…
,
0
)
(
a
α
θ
)
(
x
)
d
x
1
d
(
x
2
,
…
,
x
d
)
=
⋯
=
(
−
1
)
|
α
|
∫
R
d
∂
α
ϕ
(
x
)
a
α
(
x
)
θ
(
x
)
d
x
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}\phi (x)\partial _{\alpha }(a_{\alpha }\theta )(x)dx&=\int _{\mathbb {R} ^{d-1}}\int _{\mathbb {R} }\phi (x)\partial _{\alpha }(a_{\alpha }\theta )(x)dx_{1}d(x_{2},\ldots ,x_{d})\\&=\int _{\mathbb {R} ^{d-1}}\int _{\mathbb {R} }\phi (x)\partial _{\alpha }(a_{\alpha }\theta )(x)dx_{1}d(x_{2},\ldots ,x_{d})\\&=\int _{\mathbb {R} ^{d-1}}(-1)^{\alpha _{1}}\int _{\mathbb {R} }\partial _{(\alpha _{1},0,\ldots ,0)}\phi (x)\partial _{\alpha -(\alpha _{1},0,\ldots ,0)}(a_{\alpha }\theta )(x)dx_{1}d(x_{2},\ldots ,x_{d})\\&=\cdots =(-1)^{|\alpha |}\int _{\mathbb {R} ^{d}}\partial _{\alpha }\phi (x)a_{\alpha }(x)\theta (x)dx\end{aligned}}}
Hence,
∫
R
d
ϕ
(
x
)
L
(
θ
)
(
x
)
d
x
=
∫
R
d
L
(
ϕ
)
(
x
)
θ
(
x
)
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}\phi (x){\mathcal {L}}(\theta )(x)dx=\int _{\mathbb {R} ^{d}}L(\phi )(x)\theta (x)dx}
and the lemmas are applicable.
◻
{\displaystyle \Box }
Proof :
1.
Let
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
(
x
l
)
l
∈
N
{\displaystyle (x_{l})_{l\in \mathbb {N} }}
x
{\displaystyle x}
N
∈
N
{\displaystyle N\in \mathbb {N} }
∀
n
≥
N
:
‖
x
n
−
x
‖
≤
1
{\displaystyle \forall n\geq N:\|x_{n}-x\|\leq 1}
K
:=
⋃
n
≥
N
supp
φ
(
x
n
−
⋅
)
∪
⋃
n
<
N
supp
φ
(
x
n
−
⋅
)
¯
{\displaystyle K:={\overline {\bigcup _{n\geq N}{\text{supp }}\varphi (x_{n}-\cdot )\cup \bigcup _{n<N}{\text{supp }}\varphi (x_{n}-\cdot )}}}
is compact. Hence, if
β
∈
N
0
d
{\displaystyle \beta \in \mathbb {N} _{0}^{d}}
∂
β
φ
(
x
l
−
⋅
)
|
K
→
∂
β
φ
(
x
−
⋅
)
|
K
{\displaystyle \partial _{\beta }\varphi (x_{l}-\cdot )|_{K}\to \partial _{\beta }\varphi (x-\cdot )|_{K}}
K
{\displaystyle K}
∂
β
φ
(
x
l
−
⋅
)
−
∂
β
φ
(
x
−
⋅
)
=
0
{\displaystyle \partial _{\beta }\varphi (x_{l}-\cdot )-\partial _{\beta }\varphi (x-\cdot )=0}
∂
β
φ
(
x
l
−
⋅
)
→
∂
β
φ
(
x
−
⋅
)
{\displaystyle \partial _{\beta }\varphi (x_{l}-\cdot )\to \partial _{\beta }\varphi (x-\cdot )}
n
∈
N
{\displaystyle n\in \mathbb {N} }
supp
φ
(
x
n
−
⋅
)
⊆
K
{\displaystyle {\text{supp }}\varphi (x_{n}-\cdot )\subseteq K}
φ
(
x
l
−
⋅
)
→
φ
,
l
→
∞
{\displaystyle \varphi (x_{l}-\cdot )\to \varphi ,l\to \infty }
T
{\displaystyle {\mathcal {T}}}
(
T
∗
φ
)
(
x
l
)
=
T
(
φ
(
x
l
−
⋅
)
)
→
T
(
φ
(
x
−
⋅
)
)
=
(
T
∗
φ
)
(
x
)
,
l
→
∞
{\displaystyle ({\mathcal {T}}*\varphi )(x_{l})={\mathcal {T}}(\varphi (x_{l}-\cdot ))\to {\mathcal {T}}(\varphi (x-\cdot ))=({\mathcal {T}}*\varphi )(x),l\to \infty }
2.
We proceed by induction on
|
α
|
{\displaystyle |\alpha |}
The induction base
|
α
|
=
0
{\displaystyle |\alpha |=0}
∂
(
0
,
…
,
0
)
f
=
f
{\displaystyle \partial _{(0,\ldots ,0)}f=f}
f
:
R
d
→
R
{\displaystyle f:\mathbb {R} ^{d}\to \mathbb {R} }
Let the statement be true for all
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
=
n
{\displaystyle |\alpha |=n}
β
∈
N
0
d
{\displaystyle \beta \in \mathbb {N} _{0}^{d}}
|
β
|
=
n
+
1
{\displaystyle |\beta |=n+1}
k
∈
{
1
,
…
,
d
}
{\displaystyle k\in \{1,\ldots ,d\}}
β
k
>
0
{\displaystyle \beta _{k}>0}
β
=
0
{\displaystyle \beta =\mathbf {0} }
e
k
:=
(
0
,
…
,
0
,
1
⏞
k
th place
,
0
,
…
,
0
)
{\displaystyle e_{k}:=(0,\ldots ,0,\overbrace {1} ^{k{\text{th place}}},0,\ldots ,0)}
Then
|
β
−
e
k
|
=
n
{\displaystyle |\beta -e_{k}|=n}
∂
β
−
e
k
(
T
∗
φ
)
=
T
∗
(
∂
β
−
e
k
φ
)
{\displaystyle \partial _{\beta -e_{k}}({\mathcal {T}}*\varphi )={\mathcal {T}}*(\partial _{\beta -e_{k}}\varphi )}
Furthermore, for all
ϑ
∈
D
(
R
d
)
{\displaystyle \vartheta \in {\mathcal {D}}(\mathbb {R} ^{d})}
lim
λ
→
0
T
∗
ϑ
(
x
+
λ
e
k
)
−
T
∗
ϑ
(
x
)
λ
=
lim
λ
→
0
T
(
ϑ
(
x
+
λ
e
k
−
⋅
)
−
ϑ
(
x
−
⋅
)
λ
)
{\displaystyle \lim _{\lambda \to 0}{\frac {{\mathcal {T}}*\vartheta (x+\lambda e_{k})-{\mathcal {T}}*\vartheta (x)}{\lambda }}=\lim _{\lambda \to 0}{\mathcal {T}}\left({\frac {\vartheta (x+\lambda e_{k}-\cdot )-\vartheta (x-\cdot )}{\lambda }}\right)}
But due to Schwarz' theorem ,
ϑ
(
x
+
λ
e
k
−
⋅
)
−
ϑ
(
x
−
⋅
)
λ
→
∂
x
k
ϑ
,
λ
→
0
{\displaystyle {\frac {\vartheta (x+\lambda e_{k}-\cdot )-\vartheta (x-\cdot )}{\lambda }}\to \partial _{x_{k}}\vartheta ,\lambda \to 0}
lim
λ
→
0
T
(
ϑ
(
x
+
λ
e
k
−
⋅
)
−
ϑ
(
x
−
⋅
)
λ
)
=
T
(
ϑ
(
x
−
⋅
)
)
{\displaystyle \lim _{\lambda \to 0}{\mathcal {T}}\left({\frac {\vartheta (x+\lambda e_{k}-\cdot )-\vartheta (x-\cdot )}{\lambda }}\right)={\mathcal {T}}(\vartheta (x-\cdot ))}
Hence,
∂
β
(
T
∗
φ
)
=
∂
e
k
T
∗
(
∂
β
−
e
k
φ
)
=
T
∗
(
∂
β
φ
)
{\displaystyle \partial _{\beta }({\mathcal {T}}*\varphi )=\partial _{e_{k}}{\mathcal {T}}*(\partial _{\beta -e_{k}}\varphi )={\mathcal {T}}*(\partial _{\beta }\varphi )}
∂
β
−
e
k
φ
{\displaystyle \partial _{\beta -e_{k}}\varphi }
3.
This follows from 1. and 2., since
∂
β
φ
{\displaystyle \partial _{\beta }\varphi }
β
∈
N
0
d
{\displaystyle \beta \in \mathbb {N} _{0}^{d}}
◻
{\displaystyle \Box }
Let
T
1
,
…
,
T
n
{\displaystyle {\mathcal {T}}_{1},\ldots ,{\mathcal {T}}_{n}}
c
1
,
…
,
c
n
∈
R
{\displaystyle c_{1},\ldots ,c_{n}\in \mathbb {R} }
∑
j
=
1
n
c
j
T
j
{\displaystyle \sum _{j=1}^{n}c_{j}{\mathcal {T}}_{j}}
Let
f
:
R
d
→
R
{\displaystyle f:\mathbb {R} ^{d}\to \mathbb {R} }
T
f
{\displaystyle {\mathcal {T}}_{f}}
Prove that if
Q
{\displaystyle {\mathcal {Q}}}
[
0
,
1
]
d
{\displaystyle [0,1]^{d}}
R
{\displaystyle \mathbb {R} }
c
∈
R
>
0
{\displaystyle c\in \mathbb {R} _{>0}}
g
∈
Q
{\displaystyle g\in {\mathcal {Q}}}
∀
x
∈
R
d
:
‖
∇
g
(
x
)
‖
<
c
{\displaystyle \forall x\in \mathbb {R} ^{d}:\|\nabla g(x)\|<c}
(
f
l
)
l
∈
N
{\displaystyle (f_{l})_{l\in \mathbb {N} }}
Q
{\displaystyle {\mathcal {Q}}}
lim
l
→
∞
f
l
(
x
)
{\displaystyle \lim _{l\to \infty }f_{l}(x)}
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
f
l
{\displaystyle f_{l}}
[
0
,
1
]
d
{\displaystyle [0,1]^{d}}
[
0
,
1
]
d
{\displaystyle [0,1]^{d}}
Bolzano–Weierstrass theorem ).
Let
f
:
R
d
→
R
{\displaystyle f:\mathbb {R} ^{d}\to \mathbb {R} }
T
f
{\displaystyle {\mathcal {T}}_{f}}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
T
f
∗
φ
=
f
∗
φ
{\displaystyle {\mathcal {T}}_{f}*\varphi =f*\varphi }
Prove that for
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
δ
x
:
S
(
R
d
)
→
R
,
δ
(
ϕ
)
:=
ϕ
(
x
)
{\displaystyle \delta _{x}:{\mathcal {S}}(\mathbb {R} ^{d})\to \mathbb {R} ,\delta (\phi ):=\phi (x)}
Paul Dirac ).
For each
d
∈
N
{\displaystyle d\in \mathbb {N} }
α
d
,
β
d
∈
N
0
d
{\displaystyle \alpha _{d},\beta _{d}\in \mathbb {N} _{0}^{d}}
α
d
≤
β
d
{\displaystyle \alpha _{d}\leq \beta _{d}}
β
d
≤
α
d
{\displaystyle \beta _{d}\leq \alpha _{d}}
[ edit | edit source ] In the last two chapters, we have studied test function spaces and distributions. In this chapter we will demonstrate a method to obtain solutions to linear partial differential equations which uses test function spaces and distributions.
In the last chapter, we had defined multiplication of a distribution with a smooth function and derivatives of distributions. Therefore, for a distribution
T
{\displaystyle {\mathcal {T}}}
a
⋅
∂
α
T
{\displaystyle a\cdot \partial _{\alpha }{\mathcal {T}}}
for a smooth function
a
:
R
d
→
R
{\displaystyle a:\mathbb {R} ^{d}\to \mathbb {R} }
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u(x)=f(x)}
we could insert any distribution
T
{\displaystyle {\mathcal {T}}}
u
{\displaystyle u}
a
α
{\displaystyle a_{\alpha }}
T
f
{\displaystyle {\mathcal {T}}_{f}}
f
{\displaystyle f}
T
{\displaystyle {\mathcal {T}}}
distributional solution . Let's summarise this definition in a box.
Definition 5.2 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u(x)=f(x)}
be a linear partial differential equation. If
F
:
O
→
D
(
O
)
∗
{\displaystyle F:O\to {\mathcal {D}}(O)^{*}}
∀
φ
∈
D
(
O
)
:
x
↦
F
(
x
)
(
φ
)
{\displaystyle \forall \varphi \in {\mathcal {D}}(O):x\mapsto F(x)(\varphi )}
∀
x
∈
O
:
∀
φ
∈
D
(
O
)
:
∑
α
∈
N
0
d
a
α
∂
α
F
(
x
)
(
φ
)
=
δ
x
(
φ
)
{\displaystyle \forall x\in O:\forall \varphi \in {\mathcal {D}}(O):\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }F(x)(\varphi )=\delta _{x}(\varphi )}
we call
F
{\displaystyle F}
fundamental solution for that partial differential equation.
For the definition of
δ
x
{\displaystyle \delta _{x}}
Proof :
Let
C
⊂
R
d
{\displaystyle C\subset \mathbb {R} ^{d}}
f
{\displaystyle f}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
C
→
R
,
x
↦
T
x
(
φ
)
{\displaystyle C\to \mathbb {R} ,x\mapsto {\mathcal {T}}_{x}(\varphi )}
‖
T
⋅
(
φ
)
‖
∞
{\displaystyle \|{\mathcal {T}}_{\cdot }(\varphi )\|_{\infty }}
For
‖
f
‖
L
1
=
0
{\displaystyle \|f\|_{L_{1}}=0}
‖
T
⋅
(
φ
)
‖
∞
=
0
{\displaystyle \|{\mathcal {T}}_{\cdot }(\varphi )\|_{\infty }=0}
T
{\displaystyle {\mathcal {T}}}
‖
f
‖
L
1
≠
0
{\displaystyle \|f\|_{L_{1}}\neq 0}
‖
T
⋅
(
φ
)
‖
∞
≠
0
{\displaystyle \|{\mathcal {T}}_{\cdot }(\varphi )\|_{\infty }\neq 0}
For each
n
∈
N
{\displaystyle n\in \mathbb {N} }
B
n
(
0
)
¯
{\displaystyle {\overline {B_{n}(0)}}}
B
n
(
0
)
¯
∩
S
{\displaystyle {\overline {B_{n}(0)}}\cap S}
Q
n
,
1
,
…
,
Q
n
,
k
n
{\displaystyle Q_{n,1},\ldots ,Q_{n,k_{n}}}
1
/
n
{\displaystyle 1/n}
B
n
(
0
)
¯
∩
S
{\displaystyle {\overline {B_{n}(0)}}\cap S}
x
n
,
1
∈
Q
n
,
1
,
…
,
x
n
,
k
n
∈
Q
n
,
k
n
{\displaystyle x_{n,1}\in Q_{n,1},\ldots ,x_{n,k_{n}}\in Q_{n,k_{n}}}
For each
n
∈
N
{\displaystyle n\in \mathbb {N} }
T
n
(
φ
)
:=
∑
j
=
1
k
n
∫
Q
n
,
j
f
(
x
)
T
x
n
,
j
(
φ
)
d
x
{\displaystyle {\mathcal {T}}_{n}(\varphi ):=\sum _{j=1}^{k_{n}}\int _{Q_{n,j}}f(x){\mathcal {T}}_{x_{n,j}}(\varphi )dx}
, which is a finite linear combination of distributions and therefore a distribution (see exercise 4.1).
Let now
ϑ
∈
D
(
O
)
{\displaystyle \vartheta \in {\mathcal {D}}(O)}
ϵ
>
0
{\displaystyle \epsilon >0}
N
1
∈
N
{\displaystyle N_{1}\in \mathbb {N} }
n
≥
N
1
{\displaystyle n\geq N_{1}}
∀
x
∈
B
R
n
(
0
)
∩
S
:
y
∈
B
1
/
n
(
x
)
⇒
|
T
x
(
φ
)
−
T
y
(
φ
)
|
<
ϵ
2
‖
f
‖
L
1
{\displaystyle \forall x\in B_{R_{n}}(0)\cap S:y\in B_{1/n}(x)\Rightarrow |{\mathcal {T}}_{x}(\varphi )-{\mathcal {T}}_{y}(\varphi )|<{\frac {\epsilon }{2\|f\|_{L^{1}}}}}
This we may do because continuous functions are uniformly continuous on compact sets. Further, we choose
N
2
∈
N
{\displaystyle N_{2}\in \mathbb {N} }
∫
S
∖
B
n
(
0
)
|
f
(
x
)
|
d
x
<
ϵ
2
‖
T
⋅
(
φ
)
‖
∞
{\displaystyle \int _{S\setminus B_{n}(0)}|f(x)|dx<{\frac {\epsilon }{2\|{\mathcal {T}}_{\cdot }(\varphi )\|_{\infty }}}}
This we may do due to dominated convergence. Since for
n
≥
N
:=
max
{
N
1
,
N
2
}
{\displaystyle n\geq N:=\max\{N_{1},N_{2}\}}
|
T
n
(
φ
)
−
T
(
φ
)
|
<
∑
j
=
1
k
n
∫
Q
n
,
j
|
f
(
x
)
|
|
T
λ
x
n
,
j
(
φ
)
−
T
x
(
φ
)
|
d
x
+
ϵ
‖
T
⋅
(
φ
)
‖
∞
2
‖
T
⋅
(
φ
)
‖
∞
<
ϵ
{\displaystyle |{\mathcal {T}}_{n}(\varphi )-{\mathcal {T}}(\varphi )|<\sum _{j=1}^{k_{n}}\int _{Q{n,j}}|f(x)||{\mathcal {T}}_{\lambda _{x_{n,j}}}(\varphi )-{\mathcal {T}}_{x}(\varphi )|dx+{\frac {\epsilon \|{\mathcal {T}}_{\cdot }(\varphi )\|_{\infty }}{2\|T_{\cdot }(\varphi )\|_{\infty }}}<\epsilon }
∀
φ
∈
D
(
O
)
:
T
l
(
φ
)
→
T
(
φ
)
{\displaystyle \forall \varphi \in {\mathcal {D}}(O):{\mathcal {T}}_{l}(\varphi )\to {\mathcal {T}}(\varphi )}
◻
{\displaystyle \Box }
Theorem 5.4 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u(x)=f(x)}
be a linear partial differential equation such that
f
{\displaystyle f}
F
{\displaystyle F}
T
:
D
(
O
)
→
R
,
T
(
φ
)
:=
∫
R
d
f
(
x
)
F
(
x
)
(
φ
)
d
x
{\displaystyle {\mathcal {T}}:{\mathcal {D}}(O)\to \mathbb {R} ,{\mathcal {T}}(\varphi ):=\int _{\mathbb {R} ^{d}}f(x)F(x)(\varphi )dx}
is a distribution which is a distributional solution for the partial differential equation.
Proof : Since by the definition of fundamental solutions the function
x
↦
F
(
x
)
(
φ
)
{\displaystyle x\mapsto F(x)(\varphi )}
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
T
{\displaystyle {\mathcal {T}}}
Further, by definitions 4.16,
∑
α
∈
N
0
d
a
α
∂
α
T
(
φ
)
=
T
(
∑
α
∈
N
0
d
∂
α
(
a
α
φ
)
)
=
∫
R
d
f
(
x
)
F
(
x
)
(
∑
α
∈
N
0
d
∂
α
(
a
α
φ
)
)
d
x
=
∫
R
d
f
(
x
)
∑
α
∈
N
0
d
a
α
∂
α
F
(
x
)
(
φ
)
d
x
=
∫
R
d
f
(
x
)
δ
x
(
φ
)
d
x
=
∫
R
d
f
(
x
)
φ
(
x
)
d
x
=
T
f
(
φ
)
{\displaystyle {\begin{aligned}\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}(\varphi )&={\mathcal {T}}\left(\sum _{\alpha \in \mathbb {N} _{0}^{d}}\partial _{\alpha }(a_{\alpha }\varphi )\right)\\&=\int _{\mathbb {R} ^{d}}f(x)F(x)\left(\sum _{\alpha \in \mathbb {N} _{0}^{d}}\partial _{\alpha }(a_{\alpha }\varphi )\right)dx\\&=\int _{\mathbb {R} ^{d}}f(x)\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }F(x)(\varphi )dx\\&=\int _{\mathbb {R} ^{d}}f(x)\delta _{x}(\varphi )dx\\&=\int _{\mathbb {R} ^{d}}f(x)\varphi (x)dx\\&={\mathcal {T}}_{f}(\varphi )\end{aligned}}}
◻
{\displaystyle \Box }
Lemma 5.5 :
Let
φ
∈
D
(
R
d
)
{\displaystyle \varphi \in {\mathcal {D}}(\mathbb {R} ^{d})}
f
∈
C
∞
(
R
d
)
{\displaystyle f\in {\mathcal {C}}^{\infty }(\mathbb {R} ^{d})}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
T
∈
D
(
R
d
)
∗
{\displaystyle {\mathcal {T}}\in {\mathcal {D}}(\mathbb {R} ^{d})^{*}}
f
∂
α
(
T
∗
φ
)
=
(
f
∂
α
T
)
∗
φ
{\displaystyle f\partial _{\alpha }({\mathcal {T}}*\varphi )=(f\partial _{\alpha }{\mathcal {T}})*\varphi }
Proof :
By theorem 4.21 2., for all
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
f
∂
α
(
T
∗
φ
)
(
x
)
=
f
T
∗
(
∂
α
φ
)
(
x
)
=
f
T
(
(
∂
α
φ
)
(
x
−
⋅
)
)
=
f
T
(
(
−
1
)
|
α
|
∂
α
(
φ
(
x
−
⋅
)
)
)
=
f
(
∂
α
T
)
(
φ
(
x
−
⋅
)
)
=
(
∂
α
T
)
(
f
φ
(
x
−
⋅
)
)
=
(
f
∂
α
T
)
(
φ
(
x
−
⋅
)
)
=
(
f
∂
α
T
)
∗
φ
(
x
)
{\displaystyle {\begin{aligned}f\partial _{\alpha }({\mathcal {T}}*\varphi )(x)&=f{\mathcal {T}}*(\partial _{\alpha }\varphi )(x)\\&=f{\mathcal {T}}((\partial _{\alpha }\varphi )(x-\cdot ))\\&=f{\mathcal {T}}\left((-1)^{|\alpha |}\partial _{\alpha }(\varphi (x-\cdot ))\right)\\&=f(\partial _{\alpha }{\mathcal {T}})(\varphi (x-\cdot ))\\&=(\partial _{\alpha }{\mathcal {T}})(f\varphi (x-\cdot ))\\&=(f\partial _{\alpha }{\mathcal {T}})(\varphi (x-\cdot ))=(f\partial _{\alpha }{\mathcal {T}})*\varphi (x)\\\end{aligned}}}
◻
{\displaystyle \Box }
Proof :
By lemma 5.5, we have
∑
α
∈
N
0
d
a
α
∂
α
u
(
x
)
=
∑
α
∈
N
0
d
a
α
∂
α
(
T
∗
ϑ
)
(
x
)
=
∑
α
∈
N
0
d
a
α
(
∂
α
T
)
∗
ϑ
(
x
)
=
∑
α
∈
N
0
d
a
α
∂
α
T
(
ϑ
(
x
−
⋅
)
)
=
δ
0
(
ϑ
(
x
−
⋅
)
)
=
ϑ
(
x
)
{\displaystyle {\begin{aligned}\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }u(x)&=\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }({\mathcal {T}}*\vartheta )(x)\\&=\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(\partial _{\alpha }{\mathcal {T}})*\vartheta (x)\\&=\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}(\vartheta (x-\cdot ))\\&=\delta _{0}(\vartheta (x-\cdot ))=\vartheta (x)\end{aligned}}}
◻
{\displaystyle \Box }
In this section you will get to know a very important tool in mathematics, namely partitions of unity. We will use it in this chapter and also later in the book. In order to prove the existence of partitions of unity (we will soon define what this is), we need a few definitions first.
We also need definition 3.13 in the proof, which is why we restate it now:
Definition 3.13 :
For
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
η
R
:
R
d
→
R
,
η
R
(
x
)
=
η
(
x
R
)
/
R
d
{\displaystyle \eta _{R}:\mathbb {R} ^{d}\to \mathbb {R} ,\eta _{R}(x)=\eta \left({\frac {x}{R}}\right){\big /}R^{d}}
Proof : We will prove this by explicitly constructing such a sequence of functions.
1. First, we construct a sequence of open balls
(
B
l
)
l
∈
N
{\displaystyle (B_{l})_{l\in \mathbb {N} }}
∀
n
∈
N
:
∃
υ
∈
Υ
:
B
n
¯
⊆
U
υ
{\displaystyle \forall n\in \mathbb {N} :\exists \upsilon \in \Upsilon :{\overline {B_{n}}}\subseteq U_{\upsilon }}
∀
x
∈
O
:
|
{
n
∈
N
|
x
∈
B
n
¯
}
|
<
∞
{\displaystyle \forall x\in O:|\{n\in \mathbb {N} |x\in {\overline {B_{n}}}\}|<\infty }
⋃
j
∈
N
B
j
=
O
{\displaystyle \bigcup _{j\in \mathbb {N} }B_{j}=O}
In order to do this, we first start with the definition of a sequence compact sets; for each
n
∈
N
{\displaystyle n\in \mathbb {N} }
K
n
:=
{
x
∈
O
|
dist
(
∂
O
,
x
)
≥
1
n
,
‖
x
‖
≤
n
}
{\displaystyle K_{n}:=\left\{x\in O{\big |}{\text{dist}}(\partial O,x)\geq {\frac {1}{n}},\|x\|\leq n\right\}}
This sequence has the properties
⋃
j
∈
N
K
j
=
O
{\displaystyle \bigcup _{j\in \mathbb {N} }K_{j}=O}
∀
n
∈
N
:
K
n
⊂
K
n
+
1
∘
{\displaystyle \forall n\in \mathbb {N} :K_{n}\subset {\overset {\circ }{K_{n+1}}}}
We now construct
(
B
l
)
l
∈
N
{\displaystyle (B_{l})_{l\in \mathbb {N} }}
K
1
⊂
⋃
1
≤
j
≤
k
1
B
j
⊆
K
2
∘
{\displaystyle K_{1}\subset \bigcup _{1\leq j\leq k_{1}}B_{j}\subseteq {\overset {\circ }{K_{2}}}}
∀
n
∈
N
:
K
n
+
1
∖
K
n
∘
⊂
⋃
k
n
<
j
≤
k
n
+
1
B
j
⊆
K
n
+
2
∘
∖
K
n
−
1
{\displaystyle \forall n\in \mathbb {N} :K_{n+1}\setminus {\overset {\circ }{K_{n}}}\subset \bigcup _{k_{n}<j\leq k_{n+1}}B_{j}\subseteq {\overset {\circ }{K_{n+2}}}\setminus K_{n-1}}
for some
k
1
,
k
2
,
…
∈
N
{\displaystyle k_{1},k_{2},\ldots \in \mathbb {N} }
K
1
{\displaystyle K_{1}}
x
∈
K
1
{\displaystyle x\in K_{1}}
B
x
{\displaystyle B_{x}}
B
x
⊆
U
υ
∩
K
2
∘
{\displaystyle B_{x}\subseteq U_{\upsilon }\cap {\overset {\circ }{K_{2}}}}
υ
∈
Υ
{\displaystyle \upsilon \in \Upsilon }
K
1
{\displaystyle K_{1}}
K
1
{\displaystyle K_{1}}
B
1
,
…
B
k
1
{\displaystyle B_{1},\ldots B_{k_{1}}}
To meet the second condition, we proceed analogously, noting that for all
n
∈
N
≥
2
{\displaystyle n\in \mathbb {N} _{\geq 2}}
K
n
+
1
∖
K
n
∘
{\displaystyle K_{n+1}\setminus {\overset {\circ }{K_{n}}}}
K
n
+
2
∘
∖
K
n
−
1
{\displaystyle {\overset {\circ }{K_{n+2}}}\setminus K_{n-1}}
This sequence of open balls has the properties which we wished for.
2. We choose the respective functions. Since each
B
n
{\displaystyle B_{n}}
n
∈
N
{\displaystyle n\in \mathbb {N} }
B
n
=
B
R
n
(
x
n
)
{\displaystyle B_{n}=B_{R_{n}}(x_{n})}
where
R
n
∈
R
{\displaystyle R_{n}\in \mathbb {R} }
x
n
∈
R
d
{\displaystyle x_{n}\in \mathbb {R} ^{d}}
It is easy to prove that the function defined by
η
~
n
(
x
)
:=
η
R
n
(
x
−
x
n
)
{\displaystyle {\tilde {\eta }}_{n}(x):=\eta _{R_{n}}(x-x_{n})}
satisfies
η
~
n
(
x
)
=
0
{\displaystyle {\tilde {\eta }}_{n}(x)=0}
x
∈
B
n
{\displaystyle x\in B_{n}}
supp
η
~
n
=
B
n
¯
{\displaystyle {\text{supp }}{\tilde {\eta }}_{n}={\overline {B_{n}}}}
η
(
x
)
:=
∑
j
=
1
∞
η
~
j
(
x
)
{\displaystyle \eta (x):=\sum _{j=1}^{\infty }{\tilde {\eta }}_{j}(x)}
and, for each
n
∈
N
{\displaystyle n\in \mathbb {N} }
η
n
:=
η
~
n
η
{\displaystyle \eta _{n}:={\frac {{\tilde {\eta }}_{n}}{\eta }}}
Then, since
η
{\displaystyle \eta }
(
η
l
)
l
∈
N
{\displaystyle (\eta _{l})_{l\in \mathbb {N} }}
D
(
R
d
)
{\displaystyle {\mathcal {D}}(\mathbb {R} ^{d})}
◻
{\displaystyle \Box }
Definition 5.9 :
Let
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u(x)=f(x)}
be a linear partial differential equation. A function
G
:
R
d
×
R
d
→
R
{\displaystyle G:\mathbb {R} ^{d}\times \mathbb {R} ^{d}\to \mathbb {R} }
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
T
G
(
⋅
,
x
)
{\displaystyle {\mathcal {T}}_{G(\cdot ,x)}}
F
(
x
)
:=
T
G
(
⋅
,
x
)
{\displaystyle F(x):={\mathcal {T}}_{G(\cdot ,x)}}
is a fundamental solution of that partial differential equation is called a Green's function of that partial differential equation.
Definition 5.10 :
Let
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u(x)=f(x)}
be a linear partial differential equation. A function
K
:
R
d
→
R
{\displaystyle K:\mathbb {R} ^{d}\to \mathbb {R} }
G
(
y
,
x
)
:=
K
(
y
−
x
)
{\displaystyle G(y,x):=K(y-x)}
is a Greens function for that partial differential equation is called a Green's kernel of that partial differential equation.
Theorem 5.11 :
Let
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u(x)=f(x)}
be a linear partial differential equation (in the following, we will sometimes abbreviate PDE for partial differential equation) such that
f
∈
C
(
R
d
)
{\displaystyle f\in {\mathcal {C}}(\mathbb {R} ^{d})}
K
{\displaystyle K}
u
:=
f
∗
K
{\displaystyle u:=f*K}
exists and
∑
α
∈
N
0
d
a
α
∂
α
u
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }u}
u
{\displaystyle u}
Proof :
We choose
(
η
l
)
l
∈
N
{\displaystyle (\eta _{l})_{l\in \mathbb {N} }}
O
{\displaystyle O}
O
{\displaystyle O}
O
{\displaystyle O}
f
=
∑
j
∈
N
η
j
f
{\displaystyle f=\sum _{j\in \mathbb {N} }\eta _{j}f}
For each
n
∈
N
{\displaystyle n\in \mathbb {N} }
f
n
:=
η
n
f
{\displaystyle f_{n}:=\eta _{n}f}
and
u
n
:=
f
n
∗
K
{\displaystyle u_{n}:=f_{n}*K}
By Fubini's theorem , for all
φ
∈
D
(
R
d
)
{\displaystyle \varphi \in {\mathcal {D}}(\mathbb {R} ^{d})}
n
∈
N
{\displaystyle n\in \mathbb {N} }
∫
R
d
T
K
(
⋅
−
y
)
(
φ
)
f
n
(
y
)
d
y
=
∫
R
d
∫
R
d
K
(
x
−
y
)
φ
(
x
)
d
x
f
n
(
y
)
d
y
=
∫
R
d
∫
R
d
f
n
(
y
)
K
(
x
−
y
)
φ
(
x
)
d
y
d
x
=
∫
R
d
(
f
n
∗
K
)
(
x
)
φ
(
x
)
d
x
=
T
u
n
(
φ
)
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}T_{K(\cdot -y)}(\varphi )f_{n}(y)dy&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}K(x-y)\varphi (x)dxf_{n}(y)dy\\&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}f_{n}(y)K(x-y)\varphi (x)dydx\\&=\int _{\mathbb {R} ^{d}}(f_{n}*K)(x)\varphi (x)dx\\&={\mathcal {T}}_{u_{n}}(\varphi )\end{aligned}}}
Hence,
T
u
n
{\displaystyle {\mathcal {T}}_{u_{n}}}
Theorem 5.4 implies that
T
u
n
{\displaystyle {\mathcal {T}}_{u_{n}}}
∀
x
∈
O
:
∑
α
∈
N
0
d
a
α
(
x
)
∂
α
u
n
(
x
)
=
f
n
(
x
)
{\displaystyle \forall x\in O:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }(x)\partial _{\alpha }u_{n}(x)=f_{n}(x)}
Thus, for all
φ
∈
D
(
R
d
)
{\displaystyle \varphi \in {\mathcal {D}}(\mathbb {R} ^{d})}
∫
R
d
(
∑
α
∈
N
0
d
a
α
∂
α
u
n
)
(
x
)
φ
(
x
)
d
x
=
T
∑
α
∈
N
0
d
a
α
∂
α
u
n
(
φ
)
=
∑
α
∈
N
0
d
a
α
∂
α
T
u
n
(
φ
)
=
T
f
n
(
φ
)
=
∫
R
d
f
n
(
x
)
φ
(
x
)
d
x
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}\left(\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }u_{n}\right)(x)\varphi (x)dx&={\mathcal {T}}_{\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }u_{n}}(\varphi )\\&=\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}_{u_{n}}(\varphi )\\&=T_{f_{n}}(\varphi )=\int _{\mathbb {R} ^{d}}f_{n}(x)\varphi (x)dx\end{aligned}}}
Since
∑
α
∈
N
0
d
a
α
∂
α
u
n
{\displaystyle \sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }u_{n}}
f
n
{\displaystyle f_{n}}
n
{\displaystyle n}
◻
{\displaystyle \Box }
Proof :
If
x
l
→
x
,
l
→
∞
{\displaystyle x_{l}\to x,l\to \infty }
T
K
(
⋅
−
x
l
)
(
φ
)
−
T
K
(
⋅
−
x
)
(
φ
)
=
∫
R
d
K
(
y
−
x
l
)
φ
(
y
)
d
y
−
∫
R
d
K
(
y
−
x
)
ϕ
(
y
)
d
y
=
∫
R
d
K
(
y
)
(
φ
(
y
+
x
l
)
−
φ
(
y
+
x
)
)
d
y
≤
max
y
∈
R
d
|
φ
(
y
+
x
l
)
−
φ
(
y
+
x
)
|
∫
supp
φ
+
B
1
(
x
)
K
(
y
)
d
y
⏟
constant
{\displaystyle {\begin{aligned}{\mathcal {T}}_{K(\cdot -x_{l})}(\varphi )-{\mathcal {T}}_{K(\cdot -x)}(\varphi )&=\int _{\mathbb {R} ^{d}}K(y-x_{l})\varphi (y)dy-\int _{\mathbb {R} ^{d}}K(y-x)\phi (y)dy\\&=\int _{\mathbb {R} ^{d}}K(y)(\varphi (y+x_{l})-\varphi (y+x))dy\\&\leq \max _{y\in \mathbb {R} ^{d}}|\varphi (y+x_{l})-\varphi (y+x)|\underbrace {\int _{{\text{supp }}\varphi +B_{1}(x)}K(y)dy} _{\text{constant}}\end{aligned}}}
for sufficiently large
l
{\displaystyle l}
0
{\displaystyle 0}
l
→
∞
{\displaystyle l\to \infty }
φ
{\displaystyle \varphi }
φ
{\displaystyle \varphi }
Heine–Cantor theorem .
◻
{\displaystyle \Box }
The last theorem shows that if we have found a locally integrable function
K
{\displaystyle K}
∀
x
∈
R
d
:
∑
α
∈
N
0
d
a
α
∂
α
T
K
(
⋅
−
x
)
=
δ
x
{\displaystyle \forall x\in \mathbb {R} ^{d}:\sum _{\alpha \in \mathbb {N} _{0}^{d}}a_{\alpha }\partial _{\alpha }{\mathcal {T}}_{K(\cdot -x)}=\delta _{x}}
we have found a Green's kernel
K
{\displaystyle K}
This chapter is about the heat equation , which looks like this:
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
u
(
t
,
x
)
−
Δ
x
u
(
t
,
x
)
=
f
(
t
,
x
)
{\displaystyle \forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:\partial _{t}u(t,x)-\Delta _{x}u(t,x)=f(t,x)}
for some
f
:
R
×
R
d
→
R
{\displaystyle f:\mathbb {R} \times \mathbb {R} ^{d}\to \mathbb {R} }
f
{\displaystyle f}
Lemma 6.1 :
∫
R
e
−
x
2
d
x
=
π
{\displaystyle \int _{\mathbb {R} }e^{-x^{2}}dx={\sqrt {\pi }}}
Proof :
(
∫
R
e
−
x
2
)
2
=
(
∫
R
e
−
x
2
)
⋅
(
∫
R
e
−
y
2
)
=
∫
R
∫
R
e
−
(
x
2
+
y
2
)
d
x
d
y
=
∫
R
2
e
−
‖
(
x
,
y
)
‖
2
d
(
x
,
y
)
Fubini
=
∫
0
∞
∫
0
2
π
r
e
−
r
2
d
φ
d
r
integration by substitution using spherical coordinates
=
2
π
∫
0
∞
r
e
−
r
2
d
r
=
2
π
∫
0
∞
1
2
r
r
e
−
r
d
r
integration by substitution using
r
↦
r
=
π
{\displaystyle {\begin{aligned}\left(\int _{\mathbb {R} }e^{-x^{2}}\right)^{2}&=\left(\int _{\mathbb {R} }e^{-x^{2}}\right)\cdot \left(\int _{\mathbb {R} }e^{-y^{2}}\right)&\\&=\int _{\mathbb {R} }\int _{\mathbb {R} }e^{-(x^{2}+y^{2})}dxdy&\\&=\int _{\mathbb {R} ^{2}}e^{-\|(x,y)\|^{2}}d(x,y)&{\text{Fubini}}\\&=\int _{0}^{\infty }\int _{0}^{2\pi }re^{-r^{2}}d\varphi dr&{\text{ integration by substitution using spherical coordinates}}\\&=2\pi \int _{0}^{\infty }re^{-r^{2}}dr&\\&=2\pi \int _{0}^{\infty }{\frac {1}{2{\sqrt {r}}}}{\sqrt {r}}e^{-r}dr&{\text{integration by substitution using }}r\mapsto {\sqrt {r}}\\&=\pi &\end{aligned}}}
Taking the square root on both sides finishes the proof.
◻
{\displaystyle \Box }
Lemma 6.2 :
∫
R
d
e
−
‖
x
‖
2
/
2
d
x
=
2
π
d
{\displaystyle \int _{\mathbb {R} ^{d}}e^{-\|x\|^{2}/2}dx={\sqrt {2\pi }}^{d}}
Proof :
∫
R
d
e
−
‖
x
‖
2
2
d
x
=
∫
−
∞
∞
⋯
∫
−
∞
∞
⏞
d
times
e
−
x
1
2
+
⋯
+
x
d
2
2
d
x
1
⋯
d
x
d
Fubini's theorem
=
∫
−
∞
∞
e
−
x
d
2
2
⋯
∫
−
∞
∞
e
−
x
1
2
2
d
x
1
⋯
d
x
d
pulling the constants out of the integrals
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}e^{-{\frac {\|x\|^{2}}{2}}}dx&=\overbrace {\int _{-\infty }^{\infty }\cdots \int _{-\infty }^{\infty }} ^{d{\text{ times}}}e^{-{\frac {x_{1}^{2}+\cdots +x_{d}^{2}}{2}}}dx_{1}\cdots dx_{d}&{\text{Fubini's theorem}}\\&=\int _{-\infty }^{\infty }e^{-{\frac {x_{d}^{2}}{2}}}\cdots \int _{-\infty }^{\infty }e^{-{\frac {x_{1}^{2}}{2}}}dx_{1}\,\cdots dx_{d}&{\text{pulling the constants out of the integrals}}\end{aligned}}}
By lemma 6.1,
∫
R
e
−
x
2
d
x
=
π
{\displaystyle \int _{\mathbb {R} }e^{-x^{2}}dx={\sqrt {\pi }}}
If we apply to this integration by substitution (theorem 5.5) with the diffeomorphism
x
↦
x
2
{\displaystyle x\mapsto {\frac {x}{\sqrt {2}}}}
π
=
∫
R
1
2
e
−
x
2
2
d
x
{\displaystyle {\sqrt {\pi }}=\int _{\mathbb {R} }{\frac {1}{\sqrt {2}}}e^{-{\frac {x^{2}}{2}}}dx}
and multiplying with
2
{\displaystyle {\sqrt {2}}}
2
π
=
∫
R
e
−
x
2
2
d
x
{\displaystyle {\sqrt {2\pi }}=\int _{\mathbb {R} }e^{-{\frac {x^{2}}{2}}}dx}
Therefore, calculating the innermost integrals first and then pulling out the resulting constants,
∫
−
∞
∞
e
−
x
d
2
2
⋯
∫
−
∞
∞
e
−
x
1
2
2
⏞
d
times
d
x
1
⋯
d
x
d
=
2
π
d
{\displaystyle \overbrace {\int _{-\infty }^{\infty }e^{-{\frac {x_{d}^{2}}{2}}}\cdots \int _{-\infty }^{\infty }e^{-{\frac {x_{1}^{2}}{2}}}} ^{d{\text{ times}}}dx_{1}\cdots dx_{d}={\sqrt {2\pi }}^{d}}
◻
{\displaystyle \Box }
Theorem 6.3 :
The function
E
:
R
×
R
d
→
R
,
E
(
t
,
x
)
=
{
4
π
t
−
d
e
−
‖
x
‖
2
4
t
t
>
0
0
t
≤
0
{\displaystyle E:\mathbb {R} \times \mathbb {R} ^{d}\to \mathbb {R} ,E(t,x)={\begin{cases}{\sqrt {4\pi t}}^{-d}e^{-{\frac {\|x\|^{2}}{4t}}}&t>0\\0&t\leq 0\end{cases}}}
is a Green's kernel for the heat equation.
Proof :
1.
We show that
E
{\displaystyle E}
Let
K
⊂
R
×
R
d
{\displaystyle K\subset \mathbb {R} \times \mathbb {R} ^{d}}
T
>
0
{\displaystyle T>0}
K
⊂
(
−
T
,
T
)
×
R
d
{\displaystyle K\subset (-T,T)\times \mathbb {R} ^{d}}
∫
(
−
T
,
T
)
×
R
d
E
(
s
,
y
)
d
(
s
,
y
)
{\displaystyle \int _{(-T,T)\times \mathbb {R} ^{d}}E(s,y)d(s,y)}
exists:
∫
(
−
T
,
T
)
×
R
d
E
(
s
,
y
)
d
(
s
,
y
)
=
∫
(
0
,
T
)
×
R
d
E
(
s
,
y
)
d
(
s
,
y
)
∀
s
≤
0
:
E
(
s
,
y
)
=
0
=
∫
0
T
∫
R
d
1
4
π
s
d
e
−
‖
y
‖
2
4
s
d
y
d
s
Fubini's theorem
{\displaystyle {\begin{aligned}\int _{(-T,T)\times \mathbb {R} ^{d}}E(s,y)d(s,y)&=\int _{(0,T)\times \mathbb {R} ^{d}}E(s,y)d(s,y)&\forall s\leq 0:E(s,y)=0\\&=\int _{0}^{T}\int _{\mathbb {R} ^{d}}{\frac {1}{{\sqrt {4\pi s}}^{d}}}e^{-{\frac {\|y\|^{2}}{4s}}}dyds&{\text{Fubini's theorem}}\end{aligned}}}
By transformation of variables in the inner integral using the diffeomorphism
y
↦
2
s
y
{\displaystyle y\mapsto {\sqrt {2s}}y}
=
∫
0
T
∫
R
d
2
s
d
4
π
s
d
e
−
‖
y
‖
2
2
d
y
d
s
=
∫
0
T
1
d
s
=
T
{\displaystyle =\int _{0}^{T}\int _{\mathbb {R} ^{d}}{\frac {{\sqrt {2s}}^{d}}{{\sqrt {4\pi s}}^{d}}}e^{-{\frac {\|y\|^{2}}{2}}}dyds=\int _{0}^{T}1ds=T}
Therefore the integral
∫
(
−
T
,
T
)
×
R
d
E
(
s
,
y
)
d
(
s
,
y
)
{\displaystyle \int _{(-T,T)\times \mathbb {R} ^{d}}E(s,y)d(s,y)}
exists. But since
∀
(
s
,
y
)
∈
R
×
R
d
:
|
χ
K
(
s
,
y
)
E
(
s
,
y
)
|
≤
|
E
(
s
,
y
)
|
{\displaystyle \forall (s,y)\in \mathbb {R} \times \mathbb {R} ^{d}:|\chi _{K}(s,y)E(s,y)|\leq |E(s,y)|}
, where
χ
K
{\displaystyle \chi _{K}}
K
{\displaystyle K}
∫
(
−
T
,
T
)
×
R
d
χ
K
(
s
,
y
)
E
(
s
,
y
)
d
(
s
,
y
)
=
∫
K
E
(
s
,
y
)
d
(
s
,
y
)
{\displaystyle \int _{(-T,T)\times \mathbb {R} ^{d}}\chi _{K}(s,y)E(s,y)d(s,y)=\int _{K}E(s,y)d(s,y)}
exists. Since
K
{\displaystyle K}
2.
We calculate
∂
t
E
{\displaystyle \partial _{t}E}
Δ
x
E
{\displaystyle \Delta _{x}E}
∂
t
E
(
t
,
x
)
=
(
‖
x
‖
2
4
t
2
−
d
4
t
)
E
(
t
,
x
)
{\displaystyle \partial _{t}E(t,x)=\left({\frac {\|x\|^{2}}{4t^{2}}}-{\frac {d}{4t}}\right)E(t,x)}
Δ
x
E
(
t
,
x
)
=
(
‖
x
‖
2
4
t
2
−
d
4
t
)
E
(
t
,
x
)
{\displaystyle \Delta _{x}E(t,x)=\left({\frac {\|x\|^{2}}{4t^{2}}}-{\frac {d}{4t}}\right)E(t,x)}
3.
We show that
∀
φ
∈
D
(
R
×
R
d
)
,
(
t
,
x
)
∈
R
×
R
d
:
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
δ
(
t
,
x
)
(
φ
)
{\displaystyle \forall \varphi \in {\mathcal {D}}(\mathbb {R} \times \mathbb {R} ^{d}),(t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:(\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )=\delta _{(t,x)}(\varphi )}
Let
φ
∈
D
(
R
×
R
d
)
,
(
t
,
x
)
∈
R
×
R
d
{\displaystyle \varphi \in {\mathcal {D}}(\mathbb {R} \times \mathbb {R} ^{d}),(t,x)\in \mathbb {R} \times \mathbb {R} ^{d}}
In this last step of the proof, we will only manipulate the term
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
{\displaystyle (\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )}
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
T
E
(
⋅
−
(
t
,
x
)
)
(
(
−
∂
t
−
Δ
x
)
φ
)
by definition of distribution derivation
=
∫
R
×
R
d
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
=
∫
(
t
,
∞
)
×
R
d
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
∀
t
≤
0
:
E
(
t
,
x
)
=
0
{\displaystyle {\begin{aligned}(\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )&=T_{E(\cdot -(t,x))}((-\partial _{t}-\Delta _{x})\varphi )&{\text{by definition of distribution derivation}}\\&=\int _{\mathbb {R} \times \mathbb {R} ^{d}}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)&\\&=\int _{(t,\infty )\times \mathbb {R} ^{d}}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)&\forall t\leq 0:E(t,x)=0\\\end{aligned}}}
If we choose
R
>
0
{\displaystyle R>0}
T
>
0
{\displaystyle T>0}
supp
φ
⊂
(
−
∞
,
t
+
T
)
×
B
R
(
x
)
{\displaystyle {\text{supp }}\varphi \subset (-\infty ,t+T)\times B_{R}(x)}
, we have even
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
∫
(
t
,
t
+
T
)
×
B
R
(
x
)
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
{\displaystyle (\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )=\int _{(t,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)}
Using the dominated convergence theorem (theorem 5.1), we can rewrite the term again:
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
∫
(
t
,
t
+
T
)
×
B
R
(
x
)
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
=
lim
ϵ
↓
0
∫
(
t
,
t
+
T
)
×
B
R
(
x
)
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
(
1
−
χ
[
t
,
t
+
ϵ
]
(
s
)
)
d
(
s
,
y
)
=
lim
ϵ
↓
0
∫
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
{\displaystyle {\begin{aligned}(\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )&=\int _{(t,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)\\&=\lim _{\epsilon \downarrow 0}\int _{(t,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)(1-\chi _{[t,t+\epsilon ]}(s))d(s,y)\\&=\lim _{\epsilon \downarrow 0}\int _{(t+\epsilon ,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)\end{aligned}}}
, where
χ
[
t
,
t
+
ϵ
]
{\displaystyle \chi _{[t,t+\epsilon ]}}
[
t
,
t
+
ϵ
]
{\displaystyle [t,t+\epsilon ]}
We split the limit term in half to manipulate each summand separately:
∫
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
(
−
∂
t
−
Δ
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
=
−
∫
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
Δ
x
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
−
∫
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
∂
t
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
{\displaystyle {\begin{aligned}\int _{(t+\epsilon ,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)\\=-\int _{(t+\epsilon ,t+T)\times B_{R}(x)}\Delta _{x}\varphi (s,y)E(s-t,y-x)d(s,y)\\-\int _{(t+\epsilon ,t+T)\times B_{R}(x)}\partial _{t}\varphi (s,y)E(s-t,y-x)d(s,y)\\\end{aligned}}}
The last integrals are taken over
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
{\displaystyle (t+\epsilon ,t+T)\times B_{R}(x)}
ϵ
>
0
{\displaystyle \epsilon >0}
E
(
s
−
t
,
y
−
x
)
{\displaystyle E(s-t,y-x)}
∫
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
Δ
x
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
=
∫
t
+
ϵ
t
+
T
∫
B
R
(
x
)
Δ
x
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
y
d
s
Fubini
=
∫
t
+
ϵ
t
+
T
∫
∂
B
R
(
x
)
E
(
s
,
y
)
n
(
y
)
⋅
∇
x
φ
(
s
,
y
)
⏟
=
0
d
y
d
s
−
∫
t
+
ϵ
t
+
T
∫
B
R
(
x
)
∇
x
φ
(
s
,
y
)
⋅
∇
x
E
(
s
−
t
,
y
−
x
)
d
y
d
s
integration by parts in
y
=
∫
t
+
ϵ
t
+
T
∫
B
R
(
x
)
φ
(
s
,
y
)
Δ
x
E
(
s
−
t
,
y
−
x
)
d
y
d
s
−
∫
t
+
ϵ
t
+
T
∫
∂
B
R
(
x
)
φ
(
s
,
y
)
⏟
=
0
n
(
y
)
⋅
∇
x
E
(
s
−
t
,
y
−
x
)
d
y
d
s
integration by parts in
y
{\displaystyle {\begin{aligned}\int _{(t+\epsilon ,t+T)\times B_{R}(x)}\Delta _{x}\varphi (s,y)E(s-t,y-x)d(s,y)&=\int _{t+\epsilon }^{t+T}\int _{B_{R}(x)}\Delta _{x}\varphi (s,y)E(s-t,y-x)dyds&{\text{Fubini}}\\=\int _{t+\epsilon }^{t+T}\int _{\partial B_{R}(x)}E(s,y)n(y)\cdot \underbrace {\nabla _{x}\varphi (s,y)} _{=0}dyds&-\int _{t+\epsilon }^{t+T}\int _{B_{R}(x)}\nabla _{x}\varphi (s,y)\cdot \nabla _{x}E(s-t,y-x)dyds&{\text{integration by parts in }}y\\=\int _{t+\epsilon }^{t+T}\int _{B_{R}(x)}\varphi (s,y)\Delta _{x}E(s-t,y-x)dyds&-\int _{t+\epsilon }^{t+T}\int _{\partial B_{R}(x)}\underbrace {\varphi (s,y)} _{=0}n(y)\cdot \nabla _{x}E(s-t,y-x)dyds&{\text{integration by parts in }}y\end{aligned}}}
In the last two manipulations, we used integration by parts where
φ
{\displaystyle \varphi }
f
{\displaystyle f}
∇
x
f
{\displaystyle \nabla _{x}f}
∇
x
φ
{\displaystyle \nabla _{x}\varphi }
Let's also integrate the other integral by parts.
∫
(
t
+
ϵ
,
t
+
T
)
×
B
R
(
x
)
∂
t
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
(
s
,
y
)
=
∫
B
R
(
x
)
∫
t
+
ϵ
t
+
T
∂
t
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
d
s
d
y
Fubini
=
∫
B
R
(
x
)
φ
(
s
,
y
)
E
(
s
−
t
,
y
−
x
)
|
s
=
t
+
ϵ
s
=
t
+
T
⏟
=
−
φ
(
t
+
ϵ
,
y
)
E
(
ϵ
,
y
−
x
)
d
y
−
∫
B
R
(
x
)
∫
t
+
ϵ
t
+
T
φ
(
s
,
y
)
∂
t
E
(
s
−
t
,
y
−
x
)
d
s
d
y
integration by parts in
s
{\displaystyle {\begin{aligned}\int _{(t+\epsilon ,t+T)\times B_{R}(x)}\partial _{t}\varphi (s,y)E(s-t,y-x)d(s,y)&=\int _{B_{R}(x)}\int _{t+\epsilon }^{t+T}\partial _{t}\varphi (s,y)E(s-t,y-x)dsdy&{\text{Fubini}}\\=\int _{B_{R}(x)}\underbrace {\varphi (s,y)E(s-t,y-x){\big |}_{s=t+\epsilon }^{s=t+T}} _{=-\varphi (t+\epsilon ,y)E(\epsilon ,y-x)}dy&-\int _{B_{R}(x)}\int _{t+\epsilon }^{t+T}\varphi (s,y)\partial _{t}E(s-t,y-x)dsdy&{\text{integration by parts in }}s\end{aligned}}}
Now we add the two terms back together and see that
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
lim
ϵ
↓
0
−
∫
B
R
(
x
)
−
φ
(
t
+
ϵ
,
y
)
E
(
ϵ
,
y
−
x
)
d
y
+
∫
B
R
(
x
)
∫
t
+
ϵ
t
+
T
φ
(
s
,
y
)
∂
t
E
(
s
−
t
,
y
−
x
)
d
s
d
y
−
∫
t
+
ϵ
t
+
T
∫
B
R
(
x
)
φ
(
s
,
y
)
Δ
x
E
(
s
−
t
,
y
−
x
)
d
y
d
s
{\displaystyle {\begin{aligned}(\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )&=\lim _{\epsilon \downarrow 0}-\int _{B_{R}(x)}-\varphi (t+\epsilon ,y)E(\epsilon ,y-x)dy\\+\int _{B_{R}(x)}\int _{t+\epsilon }^{t+T}\varphi (s,y)\partial _{t}E(s-t,y-x)dsdy&-\int _{t+\epsilon }^{t+T}\int _{B_{R}(x)}\varphi (s,y)\Delta _{x}E(s-t,y-x)dyds\end{aligned}}}
The derivative calculations from above show that
∂
t
E
=
Δ
x
E
{\displaystyle \partial _{t}E=\Delta _{x}E}
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
lim
ϵ
↓
0
∫
B
R
(
x
)
φ
(
t
+
ϵ
,
y
)
E
(
ϵ
,
y
−
x
)
d
y
{\displaystyle (\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )=\lim _{\epsilon \downarrow 0}\int _{B_{R}(x)}\varphi (t+\epsilon ,y)E(\epsilon ,y-x)dy}
Using that
supp
φ
(
t
+
ϵ
,
⋅
)
⊂
B
R
(
x
)
{\displaystyle {\text{supp }}\varphi (t+\epsilon ,\cdot )\subset B_{R}(x)}
y
↦
x
+
2
ϵ
y
{\displaystyle y\mapsto x+{\sqrt {2\epsilon }}y}
∫
B
R
(
x
)
φ
(
t
+
ϵ
,
y
)
E
(
ϵ
,
y
−
x
)
d
y
=
∫
R
d
φ
(
t
+
ϵ
,
y
)
E
(
ϵ
,
y
−
x
)
d
y
{\displaystyle \int _{B_{R}(x)}\varphi (t+\epsilon ,y)E(\epsilon ,y-x)dy=\int _{\mathbb {R} ^{d}}\varphi (t+\epsilon ,y)E(\epsilon ,y-x)dy}
=
∫
R
d
φ
(
t
+
ϵ
,
y
)
1
4
π
ϵ
d
e
−
‖
y
−
x
‖
2
4
ϵ
d
y
{\displaystyle =\int _{\mathbb {R} ^{d}}\varphi (t+\epsilon ,y){\frac {1}{{\sqrt {4\pi \epsilon }}^{d}}}e^{-{\frac {\|y-x\|^{2}}{4\epsilon }}}dy}
=
∫
R
d
φ
(
t
+
ϵ
,
x
+
2
ϵ
y
)
2
ϵ
d
4
π
ϵ
d
e
−
‖
y
‖
2
2
d
y
=
1
2
π
d
∫
R
d
φ
(
t
+
ϵ
,
x
+
2
ϵ
y
)
e
−
‖
y
‖
2
2
d
y
{\displaystyle =\int _{\mathbb {R} ^{d}}\varphi (t+\epsilon ,x+{\sqrt {2\epsilon }}y){\frac {{\sqrt {2\epsilon }}^{d}}{{\sqrt {4\pi \epsilon }}^{d}}}e^{-{\frac {\|y\|^{2}}{2}}}dy={\frac {1}{{\sqrt {2\pi }}^{d}}}\int _{\mathbb {R} ^{d}}\varphi (t+\epsilon ,x+{\sqrt {2\epsilon }}y)e^{-{\frac {\|y\|^{2}}{2}}}dy}
Since
φ
{\displaystyle \varphi }
∀
x
∈
R
d
:
lim
ϵ
→
0
φ
(
t
+
ϵ
,
x
+
2
ϵ
y
)
=
φ
(
t
,
x
)
{\displaystyle \forall x\in \mathbb {R} ^{d}:\lim _{\epsilon \to 0}\varphi (t+\epsilon ,x+{\sqrt {2\epsilon }}y)=\varphi (t,x)}
Therefore
(
∂
t
−
Δ
x
)
T
E
(
⋅
−
(
t
,
x
)
)
(
φ
)
=
lim
ϵ
↓
0
1
2
π
d
∫
R
d
φ
(
t
+
ϵ
,
x
+
2
ϵ
y
)
e
−
‖
y
‖
2
2
d
y
=
1
2
π
d
∫
R
d
φ
(
t
,
x
)
e
−
‖
y
‖
2
2
d
y
dominated convergence
=
φ
(
t
,
x
)
lemma 6.2
=
δ
(
t
,
x
)
(
φ
)
{\displaystyle {\begin{aligned}(\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )&=\lim _{\epsilon \downarrow 0}{\frac {1}{{\sqrt {2\pi }}^{d}}}\int _{\mathbb {R} ^{d}}\varphi (t+\epsilon ,x+{\sqrt {2\epsilon }}y)e^{-{\frac {\|y\|^{2}}{2}}}dy&\\&={\frac {1}{{\sqrt {2\pi }}^{d}}}\int _{\mathbb {R} ^{d}}\varphi (t,x)e^{-{\frac {\|y\|^{2}}{2}}}dy&{\text{dominated convergence}}\\&=\varphi (t,x)&{\text{lemma 6.2}}\\&=\delta _{(t,x)}(\varphi )&\end{aligned}}}
◻
{\displaystyle \Box }
Theorem 6.4 :
If
f
:
R
×
R
d
{\displaystyle f:\mathbb {R} \times \mathbb {R} ^{d}}
t
{\displaystyle t}
x
{\displaystyle x}
u
(
t
,
x
)
:=
(
E
∗
f
)
(
t
,
x
)
{\displaystyle u(t,x):=(E*f)(t,x)}
solves the heat equation
∀
(
t
,
x
)
∈
R
×
R
d
:
∂
t
u
(
t
,
x
)
−
Δ
x
u
(
t
,
x
)
=
f
(
t
,
x
)
{\displaystyle \forall (t,x)\in \mathbb {R} \times \mathbb {R} ^{d}:\partial _{t}u(t,x)-\Delta _{x}u(t,x)=f(t,x)}
Proof :
1.
We show that
(
E
∗
f
)
(
t
,
x
)
{\displaystyle (E*f)(t,x)}
2.
We invoke theorem 5.?, which states exactly that a convolution with a Green's kernel is a solution, provided that the convolution is sufficiently often differentiable (which we showed in part 1 of the proof).
◻
{\displaystyle \Box }
Theorem and definition 6.6 :
Let
f
:
[
0
,
∞
)
×
R
d
→
R
{\displaystyle f:[0,\infty )\times \mathbb {R} ^{d}\to \mathbb {R} }
t
{\displaystyle t}
x
{\displaystyle x}
E
:
R
d
→
R
{\displaystyle E:\mathbb {R} ^{d}\to \mathbb {R} }
f
~
:
R
×
R
d
→
R
,
f
~
(
t
,
x
)
=
{
f
(
t
,
x
)
t
≥
0
0
t
<
0
{\displaystyle {\tilde {f}}:\mathbb {R} \times \mathbb {R} ^{d}\to \mathbb {R} ,{\tilde {f}}(t,x)={\begin{cases}f(t,x)&t\geq 0\\0&t<0\end{cases}}}
, then the function
u
:
[
0
,
∞
)
×
R
d
→
R
,
u
(
t
,
x
)
=
{
(
E
∗
x
g
)
(
t
,
x
)
+
(
f
~
∗
E
)
(
t
,
x
)
t
>
0
g
(
x
)
t
=
0
{\displaystyle u:[0,\infty )\times \mathbb {R} ^{d}\to \mathbb {R} ,u(t,x)={\begin{cases}(E*_{x}g)(t,x)+({\tilde {f}}*E)(t,x)&t>0\\g(x)&t=0\end{cases}}}
is a continuous solution of the initial value problem for the heat equation , that is
{
∀
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
:
∂
t
u
(
t
,
x
)
−
Δ
x
u
(
t
,
x
)
=
f
(
t
,
x
)
∀
x
∈
R
d
:
u
(
0
,
x
)
=
g
(
x
)
{\displaystyle {\begin{cases}\forall (t,x)\in (0,\infty )\times \mathbb {R} ^{d}:&\partial _{t}u(t,x)-\Delta _{x}u(t,x)=f(t,x)\\\forall x\in \mathbb {R} ^{d}:&u(0,x)=g(x)\end{cases}}}
Note that if we do not require the solution to be continuous, we may just take any solution and just set it to
g
{\displaystyle g}
t
=
0
{\displaystyle t=0}
Proof :
1.
We show
∀
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
:
∂
t
u
(
t
,
x
)
−
Δ
x
u
(
t
,
x
)
=
f
(
t
,
x
)
(
∗
)
{\displaystyle \forall (t,x)\in (0,\infty )\times \mathbb {R} ^{d}:\partial _{t}u(t,x)-\Delta _{x}u(t,x)=f(t,x)~~~~~(*)}
From theorem 7.4, we already know that
f
~
∗
E
{\displaystyle {\tilde {f}}*E}
∀
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
:
∂
t
(
f
~
∗
E
)
(
t
,
x
)
−
Δ
x
(
f
~
∗
E
)
(
t
,
x
)
=
f
~
(
t
,
x
)
=
t
>
0
f
(
t
,
x
)
{\displaystyle \forall (t,x)\in (0,\infty )\times \mathbb {R} ^{d}:\partial _{t}({\tilde {f}}*E)(t,x)-\Delta _{x}({\tilde {f}}*E)(t,x)={\tilde {f}}(t,x){\overset {t>0}{=}}f(t,x)}
Therefore, we have for
∀
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
{\displaystyle \forall (t,x)\in (0,\infty )\times \mathbb {R} ^{d}}
∂
t
u
(
t
,
x
)
−
Δ
x
u
(
t
,
x
)
=
∂
t
(
E
∗
x
g
)
(
t
,
x
)
+
∂
t
(
f
~
∗
E
)
(
t
,
x
)
−
Δ
x
(
E
∗
x
g
)
(
t
,
x
)
−
Δ
x
(
f
~
∗
E
)
(
t
,
x
)
=
f
(
t
,
x
)
+
∂
t
(
E
∗
x
g
)
(
t
,
x
)
−
Δ
x
(
E
∗
x
g
)
(
t
,
x
)
{\displaystyle {\begin{aligned}\partial _{t}u(t,x)-\Delta _{x}u(t,x)=&\partial _{t}(E*_{x}g)(t,x)+\partial _{t}({\tilde {f}}*E)(t,x)\\&-\Delta _{x}(E*_{x}g)(t,x)-\Delta _{x}({\tilde {f}}*E)(t,x)\\=&f(t,x)+\partial _{t}(E*_{x}g)(t,x)-\Delta _{x}(E*_{x}g)(t,x)\end{aligned}}}
which is why
(
∗
)
{\displaystyle (*)}
∀
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
:
∂
t
(
E
∗
x
g
)
(
t
,
x
)
−
Δ
x
(
E
∗
x
g
)
(
t
,
x
)
=
0
{\displaystyle \forall (t,x)\in (0,\infty )\times \mathbb {R} ^{d}:\partial _{t}(E*_{x}g)(t,x)-\Delta _{x}(E*_{x}g)(t,x)=0}
This we shall now check.
By definition of the spatial convolution, we have
∂
t
(
E
∗
x
g
)
(
t
,
x
)
=
∂
t
∫
R
d
E
(
t
,
x
−
y
)
g
(
y
)
d
y
{\displaystyle \partial _{t}(E*_{x}g)(t,x)=\partial _{t}\int _{\mathbb {R} ^{d}}E(t,x-y)g(y)dy}
and
Δ
x
(
E
∗
x
g
)
(
t
,
x
)
=
Δ
x
∫
R
d
E
(
t
,
x
−
y
)
g
(
y
)
d
y
{\displaystyle \Delta _{x}(E*_{x}g)(t,x)=\Delta _{x}\int _{\mathbb {R} ^{d}}E(t,x-y)g(y)dy}
By applying Leibniz' integral rule (see exercise 2) we find that
∂
t
(
E
∗
x
g
)
(
t
,
x
)
−
Δ
x
(
E
∗
x
g
)
(
t
,
x
)
=
∂
t
∫
R
d
E
(
t
,
x
−
y
)
g
(
y
)
d
y
−
Δ
x
∫
R
d
E
(
t
,
x
−
y
)
g
(
y
)
d
y
=
∫
R
d
∂
t
E
(
t
,
x
−
y
)
g
(
y
)
d
y
−
∫
R
d
Δ
x
E
(
t
,
x
−
y
)
g
(
y
)
d
y
Leibniz' integral rule
=
∫
R
d
(
∂
t
E
(
t
,
x
−
y
)
−
Δ
x
E
(
t
,
x
−
y
)
)
g
(
y
)
d
y
linearity of the integral
=
0
exercise 1
{\displaystyle {\begin{aligned}\partial _{t}(E*_{x}g)(t,x)-\Delta _{x}(E*_{x}g)(t,x)&=\partial _{t}\int _{\mathbb {R} ^{d}}E(t,x-y)g(y)dy-\Delta _{x}\int _{\mathbb {R} ^{d}}E(t,x-y)g(y)dy&\\&=\int _{\mathbb {R} ^{d}}\partial _{t}E(t,x-y)g(y)dy-\int _{\mathbb {R} ^{d}}\Delta _{x}E(t,x-y)g(y)dy&{\text{ Leibniz' integral rule}}\\&=\int _{\mathbb {R} ^{d}}\left(\partial _{t}E(t,x-y)-\Delta _{x}E(t,x-y)\right)g(y)dy&{\text{ linearity of the integral}}\\&=0&{\text{ exercise 1}}\end{aligned}}}
for all
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
{\displaystyle (t,x)\in (0,\infty )\times \mathbb {R} ^{d}}
2.
We show that
u
{\displaystyle u}
It is clear that
u
{\displaystyle u}
(
0
,
∞
)
×
R
d
{\displaystyle (0,\infty )\times \mathbb {R} ^{d}}
u
{\displaystyle u}
{
0
}
×
R
d
{\displaystyle \{0\}\times \mathbb {R} ^{d}}
To do so, we first note that for all
(
t
,
x
)
∈
(
0
,
∞
)
×
R
d
{\displaystyle (t,x)\in (0,\infty )\times \mathbb {R} ^{d}}
∫
R
d
E
(
t
,
x
−
y
)
d
y
=
∫
R
d
E
(
t
,
y
)
d
y
integration by substitution using
y
↦
x
−
y
=
∫
R
d
4
π
t
−
d
e
‖
y
‖
2
4
t
d
y
=
∫
R
d
2
π
−
d
e
‖
y
‖
2
2
d
y
integration by substitution using
y
↦
2
t
y
=
1
lemma 6.2
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}E(t,x-y)dy&=\int _{\mathbb {R} ^{d}}E(t,y)dy&{\text{ integration by substitution using }}y\mapsto x-y\\&=\int _{\mathbb {R} ^{d}}{\sqrt {4\pi t}}^{-d}e^{\frac {\|y\|^{2}}{4t}}dy&\\&=\int _{\mathbb {R} ^{d}}{\sqrt {2\pi }}^{-d}e^{\frac {\|y\|^{2}}{2}}dy&{\text{ integration by substitution using }}y\mapsto {\sqrt {2t}}y\\&=1&{\text{ lemma 6.2}}\end{aligned}}}
Furthermore, due to the continuity of
g
{\displaystyle g}
ϵ
>
0
{\displaystyle \epsilon >0}
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
δ
>
0
{\displaystyle \delta >0}
∀
y
∈
B
δ
(
x
)
:
|
g
(
y
)
−
g
(
x
)
|
<
ϵ
{\displaystyle \forall y\in B_{\delta }(x):|g(y)-g(x)|<\epsilon }
From these last two observations, we may conclude:
|
g
(
x
)
−
(
E
∗
x
g
)
(
t
,
x
)
|
=
|
1
⋅
g
(
x
)
−
∫
R
d
E
(
t
,
x
−
y
)
g
(
x
)
d
y
|
=
|
∫
R
d
E
(
t
,
x
−
y
)
g
(
x
)
d
y
−
∫
R
d
E
(
t
,
x
−
y
)
g
(
x
)
d
y
|
=
|
∫
B
δ
(
x
)
E
(
t
,
x
−
y
)
(
g
(
y
)
−
g
(
x
)
)
d
y
+
∫
R
d
∖
B
δ
(
x
)
E
(
t
,
x
−
y
)
(
g
(
y
)
−
g
(
x
)
)
d
y
|
≤
|
∫
B
δ
(
x
)
E
(
t
,
x
−
y
)
(
g
(
y
)
−
g
(
x
)
)
d
y
|
+
|
∫
R
d
∖
B
δ
(
x
)
E
(
t
,
x
−
y
)
(
g
(
y
)
−
g
(
x
)
)
d
y
|
triangle ineq. in
R
≤
∫
B
δ
(
x
)
|
E
(
t
,
x
−
y
)
|
|
g
(
y
)
−
g
(
x
)
|
⏟
<
ϵ
d
y
+
∫
R
d
∖
B
δ
(
x
)
|
E
(
t
,
x
−
y
)
(
g
(
y
)
−
g
(
x
)
)
|
d
y
triangle ineq. for
∫
<
∫
R
d
|
E
(
t
,
x
−
y
)
|
ϵ
d
y
+
∫
R
d
∖
B
δ
(
x
)
|
E
(
t
,
x
−
y
)
|
(
|
g
(
y
)
|
+
|
g
(
x
)
|
)
⏟
≤
2
‖
g
‖
∞
d
y
monotony of the
∫
=
ϵ
+
2
‖
g
‖
∞
|
∫
R
d
∖
B
δ
(
x
)
E
(
t
,
x
−
y
)
d
y
|
{\displaystyle {\begin{aligned}|g(x)-(E*_{x}g)(t,x)|&=\left|1\cdot g(x)-\int _{\mathbb {R} ^{d}}E(t,x-y)g(x)dy\right|&\\&=\left|\int _{\mathbb {R} ^{d}}E(t,x-y)g(x)dy-\int _{\mathbb {R} ^{d}}E(t,x-y)g(x)dy\right|\\&=\left|\int _{B_{\delta }(x)}E(t,x-y)(g(y)-g(x))dy+\int _{\mathbb {R} ^{d}\setminus B_{\delta }(x)}E(t,x-y)(g(y)-g(x))dy\right|&\\&\leq \left|\int _{B_{\delta }(x)}E(t,x-y)(g(y)-g(x))dy\right|+\left|\int _{\mathbb {R} ^{d}\setminus B_{\delta }(x)}E(t,x-y)(g(y)-g(x))dy\right|&{\text{triangle ineq. in }}\mathbb {R} \\&\leq \int _{B_{\delta }(x)}|E(t,x-y)|\underbrace {|g(y)-g(x)|} _{<\epsilon }dy+\int _{\mathbb {R} ^{d}\setminus B_{\delta }(x)}|E(t,x-y)(g(y)-g(x))|dy&{\text{ triangle ineq. for }}\int \\&<\int _{\mathbb {R} ^{d}}|E(t,x-y)|\epsilon dy+\int _{\mathbb {R} ^{d}\setminus B_{\delta }(x)}|E(t,x-y)|\underbrace {(|g(y)|+|g(x)|)} _{\leq 2\|g\|_{\infty }}dy&{\text{ monotony of the }}\int \\&=\epsilon +2\|g\|_{\infty }\left|\int _{\mathbb {R} ^{d}\setminus B_{\delta }(x)}E(t,x-y)dy\right|\end{aligned}}}
But due to integration by substitution using the diffeomorphism
x
↦
2
t
x
{\displaystyle x\mapsto {\sqrt {2t}}x}
∫
R
d
∖
B
δ
(
x
)
E
(
t
,
x
−
y
)
d
y
=
∫
R
d
∖
B
δ
(
0
)
E
(
t
,
x
)
d
y
=
∫
R
d
∖
B
δ
2
t
(
0
)
1
2
π
d
e
−
‖
x
‖
2
2
d
y
→
0
,
t
→
0
{\displaystyle \int _{\mathbb {R} ^{d}\setminus B_{\delta }(x)}E(t,x-y)dy=\int _{\mathbb {R} ^{d}\setminus B_{\delta }(0)}E(t,x)dy=\int _{\mathbb {R} ^{d}\setminus B_{\frac {\delta }{\sqrt {2t}}}(0)}{\frac {1}{{\sqrt {2\pi }}^{d}}}e^{-{\frac {\|x\|^{2}}{2}}}dy\to 0,t\to 0}
which is why
lim
t
→
0
|
g
(
x
)
−
(
E
∗
x
g
)
(
t
,
x
)
|
<
ϵ
{\displaystyle \lim _{t\to 0}|g(x)-(E*_{x}g)(t,x)|<\epsilon }
Since
ϵ
>
0
{\displaystyle \epsilon >0}
◻
{\displaystyle \Box }
This chapter deals with Poisson's equation
∀
x
∈
R
d
:
−
Δ
u
(
x
)
=
f
(
x
)
{\displaystyle \forall x\in \mathbb {R} ^{d}:-\Delta u(x)=f(x)}
Provided that
f
∈
C
2
(
R
d
)
{\displaystyle f\in {\mathcal {C}}^{2}(\mathbb {R} ^{d})}
∀
x
∈
R
d
:
−
Δ
u
(
x
)
=
0
{\displaystyle \forall x\in \mathbb {R} ^{d}:-\Delta u(x)=0}
The solutions to the homogenous Poisson's equation are called harmonic functions .
In section 2, we had seen Leibniz' integral rule, and in section 4, Fubini's theorem. In this section, we repeat the other theorems from multi-dimensional integration which we need in order to carry on with applying the theory of distributions to partial differential equations. Proofs will not be given, since understanding the proofs of these theorems is not very important for the understanding of this wikibook. The only exception will be theorem 6.3, which follows from theorem 6.2. The proof of this theorem is an exercise.
Theorem 6.2 : (Divergence theorem)
Let
K
⊂
R
d
{\displaystyle K\subset \mathbb {R} ^{d}}
V
:
K
→
R
d
{\displaystyle \mathbf {V} :K\to \mathbb {R} ^{d}}
∫
K
∇
⋅
V
(
x
)
d
x
=
∫
∂
K
ν
(
x
)
⋅
V
(
x
)
d
x
{\displaystyle \int _{K}\nabla \cdot \mathbf {V} (x)dx=\int _{\partial K}\nu (x)\cdot \mathbf {V} (x)dx}
, where
ν
:
∂
K
→
R
d
{\displaystyle \nu :\partial K\to \mathbb {R} ^{d}}
Proof : See exercise 1.
Definition 6.5 :
The Gamma function
Γ
:
R
>
0
→
R
{\displaystyle \Gamma :\mathbb {R} _{>0}\to \mathbb {R} }
Γ
(
x
)
:=
∫
0
∞
s
x
−
1
e
−
s
d
s
{\displaystyle \Gamma (x):=\int _{0}^{\infty }s^{x-1}e^{-s}ds}
The Gamma function satisfies the following equation:
Theorem 6.6 :
∀
x
∈
R
>
0
:
Γ
(
x
+
1
)
=
x
Γ
(
x
)
{\displaystyle \forall x\in \mathbb {R} _{>0}:\Gamma (x+1)=x\Gamma (x)}
Proof :
Γ
(
x
+
1
)
=
∫
0
∞
s
x
e
−
s
d
s
=
integration by parts
−
s
x
e
−
s
|
s
=
0
s
=
∞
⏟
=
0
−
∫
0
∞
−
x
s
x
−
1
e
−
s
d
s
=
x
Γ
(
x
)
{\displaystyle \Gamma (x+1)=\int _{0}^{\infty }s^{x}e^{-s}ds{\overset {\text{integration by parts}}{=}}\underbrace {-s^{x}e^{-s}{\big |}_{s=0}^{s=\infty }} _{=0}-\int _{0}^{\infty }-xs^{x-1}e^{-s}ds=x\Gamma (x)}
◻
{\displaystyle \Box }
If the Gamma function is shifted by 1, it is an interpolation of the factorial (see exercise 2):
As you can see, in the above plot the Gamma function also has values on negative numbers. This is because what is plotted above is some sort of a natural continuation of the Gamma function which one can construct using complex analysis.
Definition and theorem 6.7 :
The
d
{\displaystyle d}
Ψ
:
(
0
,
∞
)
×
(
0
,
2
π
)
×
(
−
π
/
2
,
π
/
2
)
d
−
2
→
R
d
∖
{
(
x
1
,
…
,
x
d
)
∈
R
d
:
x
1
≥
0
∧
x
2
=
0
}
{\displaystyle \Psi :(0,\infty )\times (0,2\pi )\times (-\pi /2,\pi /2)^{d-2}\to \mathbb {R} ^{d}\setminus \{(x_{1},\ldots ,x_{d})\in \mathbb {R} ^{d}:x_{1}\geq 0\wedge x_{2}=0\}}
Ψ
(
r
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
=
(
r
cos
(
Φ
)
cos
(
Θ
1
)
⋯
cos
(
Θ
d
−
2
)
r
sin
(
Φ
)
cos
(
Θ
1
)
⋯
cos
(
Θ
d
−
2
)
r
sin
(
Θ
1
)
cos
(
Θ
2
)
⋯
cos
(
Θ
d
−
2
)
⋮
r
sin
(
Θ
d
−
3
)
cos
(
Θ
d
−
2
)
r
sin
(
Θ
d
−
2
)
)
{\displaystyle \Psi (r,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2})={\begin{pmatrix}r\cos(\Phi )\cos(\Theta _{1})\cdots \cos(\Theta _{d-2})\\r\sin(\Phi )\cos(\Theta _{1})\cdots \cos(\Theta _{d-2})\\r\sin(\Theta _{1})\cos(\Theta _{2})\cdots \cos(\Theta _{d-2})\\\vdots \\r\sin(\Theta _{d-3})\cos(\Theta _{d-2})\\r\sin(\Theta _{d-2})\\\end{pmatrix}}}
are a diffeomorphism. The determinant of the Jacobian matrix of
Ψ
{\displaystyle \Psi }
det
J
Ψ
{\displaystyle \det J_{\Psi }}
det
J
Ψ
(
r
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
=
r
d
−
1
cos
(
Θ
1
)
cos
(
Θ
2
)
2
⋯
cos
(
Θ
d
−
2
)
d
−
2
{\displaystyle \det J_{\Psi }(r,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2})=r^{d-1}\cos(\Theta _{1})\cos(\Theta _{2})^{2}\cdots \cos(\Theta _{d-2})^{d-2}}
Proof :
Proof :
The surface area and the volume of the
d
{\displaystyle d}
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
Proof :
A
d
(
R
)
:=
d
π
d
/
2
Γ
(
d
/
2
+
1
)
R
d
−
1
=
d
R
V
d
(
R
)
=
d
R
∫
B
R
(
0
)
1
d
x
theorem 6.8
=
∫
B
R
(
0
)
1
R
∇
⋅
x
d
x
=
∫
∂
B
R
(
0
)
1
R
x
R
⋅
x
d
x
divergence theorem
=
∫
∂
B
R
(
0
)
1
d
x
{\displaystyle {\begin{aligned}A_{d}(R)&:={\frac {d\pi ^{d/2}}{\Gamma (d/2+1)}}R^{d-1}&\\&={\frac {d}{R}}V_{d}(R)&\\&={\frac {d}{R}}\int _{B_{R}(0)}1dx&{\text{ theorem 6.8}}\\&=\int _{B_{R}(0)}{\frac {1}{R}}\nabla \cdot xdx&\\&=\int _{\partial B_{R}(0)}{\frac {1}{R}}{\frac {x}{R}}\cdot xdx&{\text{ divergence theorem}}\\&=\int _{\partial B_{R}(0)}1dx&\end{aligned}}}
◻
{\displaystyle \Box }
We recall a fact from integration theory:
Lemma 6.11 :
f
{\displaystyle f}
⇔
{\displaystyle \Leftrightarrow }
|
f
|
{\displaystyle |f|}
We omit the proof.
Theorem 6.12 :
The function
P
:
R
d
→
R
{\displaystyle P:\mathbb {R} ^{d}\to \mathbb {R} }
P
(
x
)
:=
{
−
1
2
|
x
|
d
=
1
−
1
2
π
ln
(
‖
x
‖
)
d
=
2
1
(
d
−
2
)
A
d
(
1
)
‖
x
‖
d
−
2
d
≥
3
{\displaystyle P(x):={\begin{cases}-{\frac {1}{2}}|x|&d=1\\-{\frac {1}{2\pi }}\ln(\|x\|)&d=2\\{\frac {1}{(d-2)A_{d}(1)\|x\|^{d-2}}}&d\geq 3\end{cases}}}
is a Green's kernel for Poisson's equation.
We only prove the theorem for
d
≥
2
{\displaystyle d\geq 2}
d
=
1
{\displaystyle d=1}
Proof :
1.
We show that
P
{\displaystyle P}
K
⊆
R
d
{\displaystyle K\subseteq \mathbb {R} ^{d}}
∫
K
P
(
x
)
d
x
{\displaystyle \int _{K}P(x)dx}
is a real number, which by lemma 6.11 is equivalent to
∫
K
|
P
(
x
)
|
d
x
{\displaystyle \int _{K}|P(x)|dx}
is a real number. As compact in
R
d
{\displaystyle \mathbb {R} ^{d}}
R
>
0
{\displaystyle R>0}
K
⊂
B
R
(
0
)
{\displaystyle K\subset B_{R}(0)}
R
>
1
{\displaystyle R>1}
R
{\displaystyle R}
≤
1
{\displaystyle \leq 1}
R
>
1
{\displaystyle R>1}
∫
K
|
P
(
x
)
|
d
x
≤
∫
B
R
(
0
)
|
P
(
x
)
|
d
x
{\displaystyle \int _{K}|P(x)|dx\leq \int _{B_{R}(0)}|P(x)|dx}
For
d
=
2
{\displaystyle d=2}
∫
B
R
(
0
)
|
P
(
x
)
|
d
x
=
∫
B
1
(
0
)
−
1
2
π
ln
(
‖
x
‖
)
d
x
+
∫
B
R
(
0
)
∖
B
1
(
0
)
1
2
π
ln
(
‖
x
‖
)
d
x
=
∫
B
1
(
0
)
−
1
2
π
ln
(
‖
x
‖
)
d
x
+
∫
B
R
(
0
)
1
2
π
ln
(
‖
x
‖
)
d
x
−
∫
B
1
(
0
)
1
2
π
ln
(
‖
x
‖
)
d
x
=
∫
0
1
−
r
π
ln
(
r
)
d
r
+
∫
0
R
r
2
π
ln
(
r
)
d
r
int. by subst. using spherical coords.
=
1
4
π
+
R
2
4
π
(
ln
(
R
)
−
1
2
R
)
<
∞
{\displaystyle {\begin{aligned}\int _{B_{R}(0)}|P(x)|dx&=\int _{B_{1}(0)}-{\frac {1}{2\pi }}\ln(\|x\|)dx+\int _{B_{R}(0)\setminus B_{1}(0)}{\frac {1}{2\pi }}\ln(\|x\|)dx&\\&=\int _{B_{1}(0)}-{\frac {1}{2\pi }}\ln(\|x\|)dx+\int _{B_{R}(0)}{\frac {1}{2\pi }}\ln(\|x\|)dx-\int _{B_{1}(0)}{\frac {1}{2\pi }}\ln(\|x\|)dx&\\&=\int _{0}^{1}-{\frac {r}{\pi }}\ln(r)dr+\int _{0}^{R}{\frac {r}{2\pi }}\ln(r)dr&{\text{int. by subst. using spherical coords.}}\\&={\frac {1}{4\pi }}+{\frac {R^{2}}{4\pi }}\left(\ln(R)-{\frac {1}{2}}R\right)<\infty \end{aligned}}}
For
d
≥
3
{\displaystyle d\geq 3}
∫
B
R
(
0
)
|
P
(
x
)
|
d
x
=
∫
B
R
(
0
)
1
(
d
−
2
)
A
d
(
1
)
‖
x
‖
d
−
2
=
∫
0
R
∫
0
2
π
∫
−
π
2
π
2
⋯
∫
−
π
2
π
2
⏟
d
−
2
times
|
r
d
−
1
cos
(
Θ
1
)
⋯
cos
(
Θ
d
−
2
)
d
−
2
|
⏞
≤
r
d
−
1
1
(
d
−
2
)
A
d
(
1
)
r
d
−
2
d
Θ
1
⋯
d
Θ
d
−
2
d
Φ
d
r
≤
∫
0
R
2
r
d
−
1
(
d
−
2
)
A
d
(
1
)
r
d
−
2
π
d
−
1
d
r
=
π
d
−
1
(
d
−
2
)
A
d
(
1
)
R
2
{\displaystyle {\begin{aligned}\int _{B_{R}(0)}|P(x)|dx&=\int _{B_{R}(0)}{\frac {1}{(d-2)A_{d}(1)\|x\|^{d-2}}}\\&=\int _{0}^{R}\int _{0}^{2\pi }\underbrace {\int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}\cdots \int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}} _{d-2{\text{ times}}}\overbrace {|r^{d-1}\cos(\Theta _{1})\cdots \cos(\Theta _{d-2})^{d-2}|} ^{\leq r^{d-1}}{\frac {1}{(d-2)A_{d}(1)r^{d-2}}}d\Theta _{1}\cdots d\Theta _{d-2}d\Phi dr\\&\leq \int _{0}^{R}2{\frac {r^{d-1}}{(d-2)A_{d}(1)r^{d-2}}}\pi ^{d-1}dr\\&={\frac {\pi ^{d-1}}{(d-2)A_{d}(1)}}R^{2}\end{aligned}}}
, where we applied integration by substitution using spherical coordinates from the first to the second line.
2.
We calculate some derivatives of
P
{\displaystyle P}
For
d
=
2
{\displaystyle d=2}
∀
x
∈
R
d
∖
{
0
}
:
∇
P
(
x
)
=
−
x
2
π
‖
x
‖
2
{\displaystyle \forall x\in \mathbb {R} ^{d}\setminus \{0\}:\nabla P(x)=-{\frac {x}{2\pi \|x\|^{2}}}}
For
d
≥
3
{\displaystyle d\geq 3}
∀
x
∈
R
d
∖
{
0
}
:
∇
P
(
x
)
=
x
A
d
(
1
)
‖
x
‖
d
{\displaystyle \forall x\in \mathbb {R} ^{d}\setminus \{0\}:\nabla P(x)={\frac {x}{A_{d}(1)\|x\|^{d}}}}
For all
d
≥
2
{\displaystyle d\geq 2}
∀
x
∈
R
d
∖
{
0
}
:
Δ
P
(
x
)
=
0
{\displaystyle \forall x\in \mathbb {R} ^{d}\setminus \{0\}:\Delta P(x)=0}
3.
We show that
∀
x
∈
R
d
:
−
Δ
T
P
(
⋅
−
x
)
=
δ
x
{\displaystyle \forall x\in \mathbb {R} ^{d}:-\Delta {\mathcal {T}}_{P(\cdot -x)}=\delta _{x}}
Let
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
φ
∈
D
(
R
d
)
{\displaystyle \varphi \in {\mathcal {D}}(\mathbb {R} ^{d})}
−
Δ
T
P
(
⋅
−
x
)
(
φ
)
{\displaystyle -\Delta {\mathcal {T}}_{P(\cdot -x)}(\varphi )}
φ
∈
D
(
R
d
)
{\displaystyle \varphi \in {\mathcal {D}}(\mathbb {R} ^{d})}
φ
{\displaystyle \varphi }
K
:=
supp
φ
∪
B
1
(
x
)
¯
{\displaystyle K:={\text{supp }}\varphi \cup {\overline {B_{1}(x)}}}
Since the support of
−
Δ
T
P
(
⋅
−
x
)
(
φ
)
=
T
P
(
⋅
−
x
)
(
−
Δ
φ
)
=
∫
R
d
P
(
y
−
x
)
(
−
Δ
φ
(
y
)
)
d
y
=
∫
K
P
(
y
−
x
)
(
−
Δ
φ
(
y
)
)
d
y
=
lim
ϵ
↓
0
∫
K
P
(
y
−
x
)
(
−
Δ
φ
(
y
)
)
χ
K
∖
B
ϵ
(
x
)
(
y
)
d
y
dominated convergence
=
lim
ϵ
↓
0
∫
K
∖
B
ϵ
(
x
)
P
(
y
−
x
)
(
−
Δ
φ
(
y
)
)
d
y
{\displaystyle {\begin{aligned}-\Delta {\mathcal {T}}_{P(\cdot -x)}(\varphi )&={\mathcal {T}}_{P(\cdot -x)}(-\Delta \varphi )&\\&=\int _{\mathbb {R} ^{d}}P(y-x)(-\Delta \varphi (y))dy&\\&=\int _{K}P(y-x)(-\Delta \varphi (y))dy&\\&=\lim _{\epsilon \downarrow 0}\int _{K}P(y-x)(-\Delta \varphi (y))\chi _{K\setminus B_{\epsilon }(x)}(y)dy&{\text{dominated convergence}}\\&=\lim _{\epsilon \downarrow 0}\int _{K\setminus B_{\epsilon }(x)}P(y-x)(-\Delta \varphi (y))dy&\\\end{aligned}}}
, where
χ
K
∖
B
ϵ
(
0
)
{\displaystyle \chi _{K\setminus B_{\epsilon }(0)}}
K
∖
B
ϵ
(
0
)
{\displaystyle K\setminus B_{\epsilon }(0)}
The last integral is taken over
K
∖
B
ϵ
(
x
)
{\displaystyle K\setminus B_{\epsilon }(x)}
K
{\displaystyle K}
R
d
∖
B
ϵ
(
x
)
{\displaystyle \mathbb {R} ^{d}\setminus B_{\epsilon }(x)}
P
(
y
−
x
)
{\displaystyle P(y-x)}
x
−
y
‖
y
−
x
‖
{\displaystyle {\frac {x-y}{\|y-x\|}}}
y
∈
∂
B
ϵ
(
x
)
{\displaystyle y\in \partial B_{\epsilon }(x)}
K
∖
B
ϵ
(
x
)
{\displaystyle K\setminus B_{\epsilon }(x)}
∫
K
∖
B
ϵ
(
x
)
P
(
y
−
x
)
(
−
Δ
φ
(
y
)
)
⏞
=
−
∇
⋅
∇
φ
(
y
)
d
y
=
∫
∂
B
ϵ
(
x
)
P
(
y
−
x
)
∇
φ
(
y
)
⋅
x
−
y
‖
y
−
x
‖
d
y
−
∫
R
d
∖
B
ϵ
(
x
)
∇
φ
(
y
)
⋅
∇
P
(
y
−
x
)
d
y
{\displaystyle \int _{K\setminus B_{\epsilon }(x)}P(y-x)\overbrace {(-\Delta \varphi (y))} {=-\nabla \cdot \nabla \varphi (y)}dy=\int _{\partial B_{\epsilon }(x)}P(y-x)\nabla \varphi (y)\cdot {\frac {x-y}{\|y-x\|}}dy-\int _{\mathbb {R} ^{d}\setminus B_{\epsilon }(x)}\nabla \varphi (y)\cdot \nabla P(y-x)dy}
Let's furthermore choose
w
(
x
)
=
G
~
(
x
−
ξ
)
∇
φ
(
x
)
{\displaystyle w(x)={\tilde {G}}(x-\xi )\nabla \varphi (x)}
∇
⋅
w
(
x
)
=
Δ
φ
(
x
)
G
~
(
x
−
ξ
)
+
⟨
∇
G
~
(
x
−
ξ
)
,
∇
φ
(
x
)
⟩
{\displaystyle \nabla \cdot w(x)=\Delta \varphi (x){\tilde {G}}(x-\xi )+\langle \nabla {\tilde {G}}(x-\xi ),\nabla \varphi (x)\rangle }
From Gauß' theorem, we obtain
∫
R
d
∖
B
R
(
ξ
)
Δ
φ
(
x
)
G
~
(
x
−
ξ
)
+
⟨
∇
G
~
(
x
−
ξ
)
,
∇
φ
(
x
)
⟩
d
x
=
−
∫
∂
B
R
(
ξ
)
⟨
G
~
(
x
−
ξ
)
∇
φ
(
x
)
,
x
−
ξ
‖
x
−
ξ
‖
⟩
d
x
{\displaystyle \int _{\mathbb {R} ^{d}\setminus B_{R}(\xi )}\Delta \varphi (x){\tilde {G}}(x-\xi )+\langle \nabla {\tilde {G}}(x-\xi ),\nabla \varphi (x)\rangle dx=-\int _{\partial B_{R}(\xi )}\langle {\tilde {G}}(x-\xi )\nabla \varphi (x),{\frac {x-\xi }{\|x-\xi \|}}\rangle dx}
, where the minus in the right hand side occurs because we need the inward normal vector. From this follows immediately that
∫
R
d
∖
B
R
(
ξ
)
−
Δ
φ
(
x
)
G
~
(
x
−
ξ
)
=
∫
∂
B
R
(
ξ
)
⟨
G
~
(
x
−
ξ
)
∇
φ
(
x
)
,
x
−
ξ
‖
x
−
ξ
‖
⟩
d
x
⏟
:=
J
1
(
R
)
−
∫
R
d
∖
B
R
(
ξ
)
⟨
∇
G
~
(
x
−
ξ
)
,
∇
φ
(
x
)
⟩
d
x
⏟
:=
J
2
(
R
)
{\displaystyle \int _{\mathbb {R} ^{d}\setminus B_{R}(\xi )}-\Delta \varphi (x){\tilde {G}}(x-\xi )=\underbrace {\int _{\partial B_{R}(\xi )}\langle {\tilde {G}}(x-\xi )\nabla \varphi (x),{\frac {x-\xi }{\|x-\xi \|}}\rangle dx} _{:=J_{1}(R)}-\underbrace {\int _{\mathbb {R} ^{d}\setminus B_{R}(\xi )}\langle \nabla {\tilde {G}}(x-\xi ),\nabla \varphi (x)\rangle dx} _{:=J_{2}(R)}}
We can now calculate the following, using the Cauchy-Schwartz inequality:
|
J
1
(
R
)
|
≤
∫
∂
B
R
(
ξ
)
‖
G
~
(
x
−
ξ
)
∇
φ
(
x
)
‖
‖
x
−
ξ
‖
x
−
ξ
‖
‖
⏞
=
1
d
x
{\displaystyle |J_{1}(R)|\leq \int _{\partial B_{R}(\xi )}\|{\tilde {G}}(x-\xi )\nabla \varphi (x)\|\overbrace {\|{\frac {x-\xi }{\|x-\xi \|}}\|} ^{=1}dx}
=
{
∫
∂
B
R
(
ξ
)
−
1
2
π
ln
|
x
−
ξ
|
‖
∇
φ
(
x
)
‖
d
x
=
∫
∂
B
1
(
ξ
)
−
R
1
2
π
ln
‖
R
(
x
−
ξ
)
‖
‖
∇
φ
(
R
x
)
‖
d
x
d
=
2
∫
∂
B
R
(
ξ
)
1
(
d
−
2
)
c
1
|
x
−
ξ
|
d
−
2
‖
∇
φ
(
x
)
‖
d
x
=
∫
∂
B
1
(
ξ
)
R
d
−
1
1
(
d
−
2
)
c
1
|
R
(
x
−
ξ
)
|
d
−
2
d
≥
3
{\displaystyle ={\begin{cases}\displaystyle \int _{\partial B_{R}(\xi )}-{\frac {1}{2\pi }}\ln |x-\xi |\|\nabla \varphi (x)\|dx=\int _{\partial B_{1}(\xi )}-R{\frac {1}{2\pi }}\ln \|R(x-\xi )\|\|\nabla \varphi (Rx)\|dx&d=2\\\displaystyle \int _{\partial B_{R}(\xi )}{\frac {1}{(d-2)c}}{\frac {1}{|x-\xi |^{d-2}}}\|\nabla \varphi (x)\|dx=\int _{\partial B_{1}(\xi )}R^{d-1}{\frac {1}{(d-2)c}}{\frac {1}{|R(x-\xi )|^{d-2}}}&d\geq 3\end{cases}}}
≤
{
max
x
∈
R
d
‖
∇
φ
(
R
x
)
‖
∫
∂
B
1
(
ξ
)
−
R
1
2
π
ln
R
2
d
x
=
−
max
x
∈
R
d
‖
∇
φ
(
R
x
)
‖
c
2
π
R
ln
R
2
→
0
,
R
→
0
d
=
2
max
x
∈
R
d
‖
∇
φ
(
R
x
)
‖
∫
∂
B
1
(
ξ
)
1
(
d
−
2
)
c
R
d
x
=
max
x
∈
R
d
‖
∇
φ
(
R
x
)
‖
R
d
−
2
→
0
,
R
→
0
d
≥
3
{\displaystyle \leq {\begin{cases}\displaystyle \max \limits _{x\in \mathbb {R} ^{d}}\|\nabla \varphi (Rx)\|\int _{\partial B_{1}(\xi )}-R{\frac {1}{2\pi }}\ln R^{2}dx=-\max \limits _{x\in \mathbb {R} ^{d}}\|\nabla \varphi (Rx)\|{\frac {c}{2\pi }}R\ln R^{2}\to 0,R\to 0&d=2\\\displaystyle \max \limits _{x\in \mathbb {R} ^{d}}\|\nabla \varphi (Rx)\|\int _{\partial B_{1}(\xi )}{\frac {1}{(d-2)c}}Rdx=\max \limits _{x\in \mathbb {R} ^{d}}\|\nabla \varphi (Rx)\|{\frac {R}{d-2}}\to 0,R\to 0&d\geq 3\end{cases}}}
Now we define
v
(
x
)
=
φ
(
x
)
∇
G
~
(
x
−
ξ
)
{\displaystyle v(x)=\varphi (x)\nabla {\tilde {G}}(x-\xi )}
∇
⋅
v
(
x
)
=
φ
(
x
)
Δ
G
~
(
x
−
ξ
)
⏟
=
0
,
x
≠
ξ
+
⟨
∇
φ
(
x
)
,
∇
G
~
(
x
−
ξ
)
⟩
{\displaystyle \nabla \cdot v(x)=\varphi (x)\underbrace {\Delta {\tilde {G}}(x-\xi )} _{=0,x\neq \xi }+\langle \nabla \varphi (x),\nabla {\tilde {G}}(x-\xi )\rangle }
Applying Gauß' theorem on
v
{\displaystyle v}
J
2
(
R
)
=
∫
∂
B
R
(
ξ
)
φ
(
x
)
⟨
∇
G
~
(
x
−
ξ
)
,
x
−
ξ
‖
x
−
ξ
‖
⟩
d
x
{\displaystyle J_{2}(R)=\int _{\partial B_{R}(\xi )}\varphi (x)\langle \nabla {\tilde {G}}(x-\xi ),{\frac {x-\xi }{\|x-\xi \|}}\rangle dx}
=
∫
∂
B
R
(
ξ
)
φ
(
x
)
⟨
−
x
−
ξ
c
‖
x
−
ξ
‖
d
,
x
−
ξ
‖
x
−
ξ
‖
⟩
d
x
=
−
1
c
∫
∂
B
R
(
ξ
)
1
R
d
−
1
φ
(
x
)
d
x
{\displaystyle =\int _{\partial B_{R}(\xi )}\varphi (x)\langle -{\frac {x-\xi }{c\|x-\xi \|^{d}}},{\frac {x-\xi }{\|x-\xi \|}}\rangle dx=-{\frac {1}{c}}\int _{\partial B_{R}(\xi )}{\frac {1}{R^{d-1}}}\varphi (x)dx}
, noting that
d
=
2
⇒
c
=
2
π
{\displaystyle d=2\Rightarrow c=2\pi }
We furthermore note that
φ
(
ξ
)
=
1
c
∫
∂
B
1
(
ξ
)
φ
(
ξ
)
d
x
=
1
c
∫
∂
B
R
(
ξ
)
1
R
d
−
1
φ
(
ξ
)
d
x
{\displaystyle \varphi (\xi )={\frac {1}{c}}\int _{\partial B_{1}(\xi )}\varphi (\xi )dx={\frac {1}{c}}\int _{\partial B_{R}(\xi )}{\frac {1}{R^{d-1}}}\varphi (\xi )dx}
Therefore, we have
lim
R
→
0
|
−
J
2
(
R
)
−
φ
(
ξ
)
|
≤
1
c
lim
R
→
0
∫
∂
B
R
(
ξ
)
1
R
d
−
1
|
φ
(
ξ
)
−
φ
(
x
)
|
d
x
≤
lim
R
→
0
1
c
max
x
∈
B
R
(
ξ
)
|
φ
(
x
)
−
φ
(
ξ
)
|
∫
∂
B
1
(
ξ
)
1
d
x
{\displaystyle \lim _{R\to 0}|-J_{2}(R)-\varphi (\xi )|\leq {\frac {1}{c}}\lim _{R\to 0}\int _{\partial B_{R}(\xi )}{\frac {1}{R^{d-1}}}|\varphi (\xi )-\varphi (x)|dx\leq \lim _{R\to 0}{\frac {1}{c}}\max _{x\in B_{R}(\xi )}|\varphi (x)-\varphi (\xi )|\int _{\partial B_{1}(\xi )}1dx}
=
lim
R
→
0
max
x
∈
B
R
(
ξ
)
|
φ
(
x
)
−
φ
(
ξ
)
|
=
0
{\displaystyle =\lim _{R\to 0}\max _{x\in B_{R}(\xi )}|\varphi (x)-\varphi (\xi )|=0}
due to the continuity of
φ
{\displaystyle \varphi }
Thus we can conclude that
∀
Ω
domain of
R
d
:
∀
φ
∈
D
(
Ω
)
:
−
Δ
T
G
~
(
⋅
−
ξ
)
(
φ
)
=
lim
R
→
0
J
0
(
R
)
=
lim
R
→
0
J
1
(
R
)
−
J
2
(
R
)
=
0
+
φ
(
ξ
)
=
δ
ξ
(
φ
)
{\displaystyle \forall \Omega {\text{ domain of }}\mathbb {R} ^{d}:\forall \varphi \in {\mathcal {D}}(\Omega ):-\Delta T_{{\tilde {G}}(\cdot -\xi )}(\varphi )=\lim _{R\to 0}J_{0}(R)=\lim _{R\to 0}J_{1}(R)-J_{2}(R)=0+\varphi (\xi )=\delta _{\xi }(\varphi )}
Therefore,
G
~
{\displaystyle {\tilde {G}}}
d
≥
2
{\displaystyle d\geq 2}
QED.
Theorem 6.12 :
Let
f
:
R
d
→
R
{\displaystyle f:\mathbb {R} ^{d}\to \mathbb {R} }
∫
∂
B
R
(
0
)
f
(
x
)
d
x
=
R
d
−
1
∫
0
2
π
∫
−
π
2
π
2
⋯
∫
−
π
2
π
2
⏟
d
−
2
times
f
(
Ψ
(
r
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
)
cos
(
Θ
1
)
cos
(
Θ
2
)
2
⋯
cos
(
Θ
d
−
2
)
d
−
2
d
Θ
1
d
Θ
2
⋯
d
Θ
d
−
2
d
Φ
{\displaystyle \int _{\partial B_{R}(0)}f(x)dx=R^{d-1}\int _{0}^{2\pi }\underbrace {\int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}\cdots \int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}} _{d-2{\text{ times}}}f\left(\Psi (r,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2})\right)\cos(\Theta _{1})\cos(\Theta _{2})^{2}\cdots \cos(\Theta _{d-2})^{d-2}d\Theta _{1}d\Theta _{2}\cdots d\Theta _{d-2}d\Phi }
Proof : We choose as an orientation the border orientation of the sphere. We know that for
∂
B
r
(
0
)
{\displaystyle \partial B_{r}(0)}
ν
(
x
)
=
x
r
{\displaystyle \nu (x)={\frac {x}{r}}}
B
r
(
0
)
{\displaystyle B_{r}(0)}
B
r
(
0
)
{\displaystyle B_{r}(0)}
ω
B
r
(
0
)
(
x
)
=
e
1
∗
∧
⋯
∧
e
d
∗
{\displaystyle \omega _{B_{r}(0)}(x)=e_{1}^{*}\wedge \cdots \wedge e_{d}^{*}}
Now, we use the normal vector field to obtain the volume form of
∂
B
r
(
0
)
{\displaystyle \partial B_{r}(0)}
ω
∂
B
r
(
0
)
(
x
)
(
v
1
,
…
,
v
d
−
1
)
=
ω
B
r
(
0
)
(
x
)
(
ν
(
x
)
,
v
1
,
…
,
v
d
−
1
)
{\displaystyle \omega _{\partial B_{r}(0)}(x)(v_{1},\ldots ,v_{d-1})=\omega _{B_{r}(0)}(x)(\nu (x),v_{1},\ldots ,v_{d-1})}
We insert the formula for
ω
B
r
(
0
)
(
x
)
{\displaystyle \omega _{B_{r}(0)}(x)}
=
e
1
∗
∧
⋯
∧
e
d
∗
(
ν
(
x
)
,
v
1
,
…
,
v
d
−
1
)
=
1
r
∑
i
=
1
d
(
−
1
)
i
+
1
x
i
e
1
∗
⋯
∧
e
i
−
1
∗
∧
e
i
+
1
∗
∧
⋯
∧
e
d
∗
(
v
1
,
…
,
v
d
−
1
)
{\displaystyle =e_{1}^{*}\wedge \cdots \wedge e_{d}^{*}(\nu (x),v_{1},\ldots ,v_{d-1})={\frac {1}{r}}\sum _{i=1}^{d}(-1)^{i+1}x_{i}e_{1}^{*}\cdots \wedge e_{i-1}^{*}\wedge e_{i+1}^{*}\wedge \cdots \wedge e_{d}^{*}(v_{1},\ldots ,v_{d-1})}
As a parametrisation of
∂
B
r
(
x
)
{\displaystyle \partial B_{r}(x)}
r
{\displaystyle r}
We calculate the Jacobian matrix for the spherical coordinates:
J
=
(
cos
(
φ
)
cos
(
ϑ
1
)
⋯
cos
(
ϑ
d
−
2
)
r
−
sin
(
φ
)
cos
(
ϑ
1
)
⋯
cos
(
ϑ
d
−
2
)
−
r
cos
(
φ
)
sin
(
ϑ
1
)
⋯
cos
(
ϑ
d
−
2
)
⋯
⋯
−
r
cos
(
φ
)
cos
(
ϑ
1
)
⋯
sin
(
ϑ
d
−
2
)
sin
(
φ
)
cos
(
ϑ
1
)
⋯
cos
(
ϑ
d
−
2
)
r
cos
(
φ
)
cos
(
ϑ
1
)
⋯
cos
(
ϑ
d
−
2
)
−
r
sin
(
φ
)
sin
(
ϑ
1
)
⋯
cos
(
ϑ
d
−
2
)
⋯
⋯
−
r
sin
(
φ
)
cos
(
ϑ
1
)
⋯
sin
(
ϑ
d
−
2
)
⋮
0
⋱
⋱
⋱
⋮
⋮
⋮
⋱
⋱
⋱
sin
(
ϑ
d
−
3
)
cos
(
ϑ
d
−
2
)
0
⋯
0
r
cos
(
ϑ
d
−
3
)
cos
(
ϑ
d
−
2
)
r
sin
(
ϑ
d
−
3
)
cos
(
ϑ
d
−
2
)
sin
(
ϑ
d
−
2
)
0
⋯
⋯
0
r
cos
(
ϑ
d
−
2
)
)
{\displaystyle J=\left({\begin{smallmatrix}\cos(\varphi )\cos(\vartheta _{1})\cdots \cos(\vartheta _{d-2})&r-\sin(\varphi )\cos(\vartheta _{1})\cdots \cos(\vartheta _{d-2})&-r\cos(\varphi )\sin(\vartheta _{1})\cdots \cos(\vartheta _{d-2})&\cdots &\cdots &-r\cos(\varphi )\cos(\vartheta _{1})\cdots \sin(\vartheta _{d-2})\\\sin(\varphi )\cos(\vartheta _{1})\cdots \cos(\vartheta _{d-2})&r\cos(\varphi )\cos(\vartheta _{1})\cdots \cos(\vartheta _{d-2})&-r\sin(\varphi )\sin(\vartheta _{1})\cdots \cos(\vartheta _{d-2})&\cdots &\cdots &-r\sin(\varphi )\cos(\vartheta _{1})\cdots \sin(\vartheta _{d-2})\\\vdots &0&\ddots &\ddots &\ddots &\vdots \\\vdots &\vdots &\ddots &\ddots &\ddots &\\\sin(\vartheta _{d-3})\cos(\vartheta _{d-2})&0&\cdots &0&r\cos(\vartheta _{d-3})\cos(\vartheta _{d-2})&r\sin(\vartheta _{d-3})\cos(\vartheta _{d-2})\\\sin(\vartheta _{d-2})&0&\cdots &\cdots &0&r\cos(\vartheta _{d-2})\end{smallmatrix}}\right)}
We observe that in the first column, we have only the spherical coordinates divided by
r
{\displaystyle r}
r
{\displaystyle r}
J
′
{\displaystyle J'}
r
{\displaystyle r}
Ψ
{\displaystyle \Psi }
Ψ
∗
ω
∂
B
r
(
0
)
(
x
)
(
v
1
,
…
,
v
d
−
1
)
=
ω
∂
B
r
(
0
)
(
Ψ
(
x
)
)
(
J
′
v
1
,
…
,
J
′
v
d
−
1
)
{\displaystyle \Psi ^{*}\omega _{\partial B_{r}(0)}(x)(v_{1},\ldots ,v_{d-1})=\omega _{\partial B_{r}(0)}(\Psi (x))(J'v_{1},\ldots ,J'v_{d-1})}
=
1
r
∑
i
=
1
d
(
−
1
)
i
+
1
Ψ
(
x
)
i
e
1
∗
⋯
∧
e
i
−
1
∗
∧
e
i
+
1
∗
∧
⋯
∧
e
d
∗
(
J
′
v
1
,
…
,
J
′
v
d
−
1
)
{\displaystyle ={\frac {1}{r}}\sum _{i=1}^{d}(-1)^{i+1}\Psi (x)_{i}e_{1}^{*}\cdots \wedge e_{i-1}^{*}\wedge e_{i+1}^{*}\wedge \cdots \wedge e_{d}^{*}(J'v_{1},\ldots ,J'v_{d-1})}
=
1
r
∑
i
=
1
d
(
−
1
)
i
+
1
Ψ
(
x
)
i
det
(
e
j
∗
(
J
′
v
k
)
)
j
≠
i
=
det
J
⋅
det
(
v
1
,
…
,
v
d
−
1
)
{\displaystyle ={\frac {1}{r}}\sum _{i=1}^{d}(-1)^{i+1}\Psi (x)_{i}\det(e_{j}^{*}(J'v_{k}))_{j\neq i}=\det J\cdot \det(v_{1},\ldots ,v_{d-1})}
Recalling that
det
J
=
r
d
−
1
cos
(
ϕ
1
)
n
−
2
cos
(
ϕ
2
)
d
−
3
⋯
cos
(
ϕ
d
−
2
)
{\displaystyle \det J=r^{d-1}\cos(\phi _{1})^{n-2}\cos(\phi _{2})^{d-3}\cdots \cos(\phi _{d-2})}
, the claim follows using the definition of the surface integral.
Theorem 6.13 :
Let
f
:
R
d
→
R
{\displaystyle f:\mathbb {R} ^{d}\to \mathbb {R} }
∫
R
d
f
(
x
)
d
x
=
∫
0
∞
r
d
−
1
∫
∂
B
1
(
0
)
f
(
r
x
)
d
x
d
r
{\displaystyle \int _{\mathbb {R} ^{d}}f(x)dx=\int _{0}^{\infty }r^{d-1}\int _{\partial B_{1}(0)}f(rx)dxdr}
Proof :
We have
r
Ψ
(
1
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
=
Ψ
(
r
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
{\displaystyle r\Psi (1,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2})=\Psi (r,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2})}
Ψ
{\displaystyle \Psi }
∫
R
d
f
(
x
)
d
x
=
∫
(
0
,
∞
)
×
(
0
,
2
π
)
×
(
−
π
/
2
,
π
/
2
)
d
−
2
f
(
Ψ
(
x
)
)
|
det
J
Ψ
(
x
)
|
d
x
=
∫
0
∞
∫
0
2
π
∫
−
π
2
π
2
⋯
∫
−
π
2
π
2
⏟
d
−
2
times
f
(
Ψ
(
r
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
)
r
d
−
1
cos
(
Θ
1
)
⋯
cos
(
Θ
d
−
2
)
d
−
2
d
Θ
1
⋯
d
Θ
d
−
2
d
Φ
d
r
=
∫
0
∞
r
d
−
1
∫
0
2
π
∫
−
π
2
π
2
⋯
∫
−
π
2
π
2
⏟
d
−
2
times
f
(
r
Ψ
(
1
,
Φ
,
Θ
1
,
…
,
Θ
d
−
2
)
)
cos
(
Θ
1
)
⋯
cos
(
Θ
d
−
2
)
d
−
2
d
Θ
1
⋯
d
Θ
d
−
2
d
Φ
d
r
=
∫
0
∞
r
d
−
1
∫
∂
B
1
(
0
)
f
(
r
x
)
d
r
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}f(x)dx&=\int _{(0,\infty )\times (0,2\pi )\times (-\pi /2,\pi /2)^{d-2}}f(\Psi (x))|\det J_{\Psi }(x)|dx\\&=\int _{0}^{\infty }\int _{0}^{2\pi }\underbrace {\int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}\cdots \int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}} _{d-2{\text{ times}}}f(\Psi (r,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2}))r^{d-1}\cos(\Theta _{1})\cdots \cos(\Theta _{d-2})^{d-2}d\Theta _{1}\cdots d\Theta _{d-2}d\Phi dr\\&=\int _{0}^{\infty }r^{d-1}\int _{0}^{2\pi }\underbrace {\int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}\cdots \int _{-{\frac {\pi }{2}}}^{\frac {\pi }{2}}} _{d-2{\text{ times}}}f(r\Psi (1,\Phi ,\Theta _{1},\ldots ,\Theta _{d-2}))\cos(\Theta _{1})\cdots \cos(\Theta _{d-2})^{d-2}d\Theta _{1}\cdots d\Theta _{d-2}d\Phi dr\\&=\int _{0}^{\infty }r^{d-1}\int _{\partial B_{1}(0)}f(rx)dr\end{aligned}}}
◻
{\displaystyle \Box }
Proof : Let's define the following function:
ϕ
(
r
)
=
1
r
d
−
1
∫
∂
B
r
(
x
)
u
(
y
)
d
y
{\displaystyle \phi (r)={\frac {1}{r^{d-1}}}\int _{\partial B_{r}(x)}u(y)dy}
From first coordinate transformation with the diffeomorphism
y
↦
x
+
y
{\displaystyle y\mapsto x+y}
ϕ
(
r
)
=
1
r
d
−
1
∫
∂
B
r
(
0
)
u
(
y
+
x
)
d
y
=
∫
∂
B
1
(
0
)
u
(
x
+
r
y
)
d
y
{\displaystyle \phi (r)={\frac {1}{r^{d-1}}}\int _{\partial B_{r}(0)}u(y+x)dy=\int _{\partial B_{1}(0)}u(x+ry)dy}
From first differentiation under the integral sign and then Gauss' theorem, we know that
ϕ
′
(
r
)
=
∫
∂
B
1
(
0
)
⟨
∇
u
(
x
+
r
y
)
,
y
⟩
d
y
=
∫
B
1
(
0
)
Δ
u
(
x
+
r
y
)
d
y
=
0
{\displaystyle \phi '(r)=\int _{\partial B_{1}(0)}\langle \nabla u(x+ry),y\rangle dy=\int _{B_{1}(0)}\Delta u(x+ry)dy=0}
Case 1 :
If
u
{\displaystyle u}
∫
B
1
(
0
)
Δ
u
(
x
+
r
y
)
d
y
=
0
{\displaystyle \int _{B_{1}(0)}\Delta u(x+ry)dy=0}
, which is why
ϕ
{\displaystyle \phi }
lim
r
→
0
ϕ
(
r
)
=
∫
∂
B
1
(
0
)
lim
r
→
0
u
(
x
+
r
y
)
d
y
=
c
(
1
)
u
(
x
)
{\displaystyle \lim _{r\to 0}\phi (r)=\int _{\partial B_{1}(0)}\lim _{r\to 0}u(x+ry)dy=c(1)u(x)}
Therefore
ϕ
(
r
)
=
c
(
1
)
u
(
x
)
{\displaystyle \phi (r)=c(1)u(x)}
r
{\displaystyle r}
With the relationship
r
d
−
1
c
(
1
)
=
c
(
r
)
{\displaystyle r^{d-1}c(1)=c(r)}
, which is true because of our formula for
c
(
x
)
,
x
∈
R
>
0
{\displaystyle c(x),x\in \mathbb {R} _{>0}}
u
(
x
)
=
ϕ
(
r
)
c
(
1
)
=
1
c
(
1
)
1
r
d
−
1
∫
∂
B
r
(
x
)
u
(
y
)
d
y
=
1
c
(
r
)
∫
∂
B
r
(
x
)
u
(
y
)
d
y
{\displaystyle u(x)={\frac {\phi (r)}{c(1)}}={\frac {1}{c(1)}}{\frac {1}{r^{d-1}}}\int _{\partial B_{r}(x)}u(y)dy={\frac {1}{c(r)}}\int _{\partial B_{r}(x)}u(y)dy}
, which proves the first formula.
Furthermore, we can prove the second formula by first transformation of variables, then integrating by onion skins, then using the first formula of this theorem and then integration by onion skins again:
∫
B
r
(
x
)
u
(
y
)
d
y
=
∫
B
r
(
0
)
u
(
y
+
x
)
d
y
=
∫
0
r
s
d
−
1
∫
∂
B
1
(
0
)
u
(
y
+
s
x
)
d
x
d
s
=
∫
0
r
s
d
−
1
u
(
x
)
∫
∂
B
1
(
0
)
1
d
x
d
s
=
u
(
x
)
d
(
r
)
{\displaystyle \int _{B_{r}(x)}u(y)dy=\int _{B_{r}(0)}u(y+x)dy=\int _{0}^{r}s^{d-1}\int _{\partial B_{1}(0)}u(y+sx)dxds=\int _{0}^{r}s^{d-1}u(x)\int _{\partial B_{1}(0)}1dxds=u(x)d(r)}
This shows that if
u
{\displaystyle u}
u
{\displaystyle u}
Case 2 :
Suppose that
u
{\displaystyle u}
x
∈
Ω
{\displaystyle x\in \Omega }
−
Δ
u
(
x
)
≠
0
{\displaystyle -\Delta u(x)\neq 0}
−
Δ
u
(
x
)
>
0
{\displaystyle -\Delta u(x)>0}
−
Δ
u
(
x
)
<
0
{\displaystyle -\Delta u(x)<0}
lim
r
→
0
ϕ
′
(
r
)
=
∫
B
1
(
0
)
lim
r
→
0
Δ
u
(
x
+
r
y
)
d
y
>
0
{\displaystyle \lim _{r\to 0}\phi '(r)=\int _{B_{1}(0)}\lim _{r\to 0}\Delta u(x+ry)dy>0}
Since
ϕ
′
{\displaystyle \phi '}
ϕ
{\displaystyle \phi }
0
{\displaystyle 0}
The contradiction to the second formula can be obtained by observing that
ϕ
′
{\displaystyle \phi '}
σ
∈
R
>
0
{\displaystyle \sigma \in \mathbb {R} _{>0}}
∀
r
∈
[
0
,
σ
)
:
ϕ
′
(
r
)
>
0
{\displaystyle \forall r\in [0,\sigma ):\phi '(r)>0}
This means that since
lim
r
→
0
ϕ
(
r
)
=
∫
∂
B
1
(
0
)
lim
r
→
0
u
(
x
+
r
y
)
d
y
=
c
(
1
)
u
(
x
)
{\displaystyle \lim _{r\to 0}\phi (r)=\int _{\partial B_{1}(0)}\lim _{r\to 0}u(x+ry)dy=c(1)u(x)}
and therefore
ϕ
(
0
)
=
c
(
1
)
u
(
x
)
{\displaystyle \phi (0)=c(1)u(x)}
, that
∀
r
∈
(
0
,
σ
)
:
ϕ
(
r
)
>
c
(
1
)
u
(
x
)
{\displaystyle \forall r\in (0,\sigma ):\phi (r)>c(1)u(x)}
and therefore, by the same calculation as above,
∫
B
r
(
x
)
u
(
y
)
d
y
=
∫
B
r
(
0
)
u
(
y
+
x
)
d
y
=
∫
0
r
s
d
−
1
∫
∂
B
1
(
0
)
u
(
y
+
s
x
)
d
x
d
s
>
∫
0
r
s
d
−
1
u
(
x
)
∫
∂
B
1
(
0
)
1
d
x
d
s
=
u
(
x
)
d
(
r
)
{\displaystyle \int _{B_{r}(x)}u(y)dy=\int _{B_{r}(0)}u(y+x)dy=\int _{0}^{r}s^{d-1}\int _{\partial B_{1}(0)}u(y+sx)dxds>\int _{0}^{r}s^{d-1}u(x)\int _{\partial B_{1}(0)}1dxds=u(x)d(r)}
This shows (by proof with contradiction) that if one of the two formulas hold, then
u
∈
C
2
(
Ω
)
{\displaystyle u\in C^{2}(\Omega )}
Definition 6.16 :
A domain is an open and connected subset of
R
d
{\displaystyle \mathbb {R} ^{d}}
For the proof of the next theorem, we need two theorems from other subjects, the first from integration theory and the second from topology.
Theorem 6.17 :
Let
B
⊆
R
d
{\displaystyle B\subseteq \mathbb {R} ^{d}}
f
:
B
→
R
{\displaystyle f:B\to \mathbb {R} }
∫
B
|
f
(
x
)
|
d
x
=
0
{\displaystyle \int _{B}|f(x)|dx=0}
then
f
(
x
)
=
0
{\displaystyle f(x)=0}
x
∈
B
{\displaystyle x\in B}
Theorem 6.18 :
In a connected topological space, the only simultaneously open and closed sets are the whole space and the empty set.
We will omit the proofs.
Proof :
We choose
B
:=
{
x
∈
Ω
:
u
(
x
)
=
sup
y
∈
Ω
u
(
y
)
}
{\displaystyle B:=\left\{x\in \Omega :u(x)=\sup _{y\in \Omega }u(y)\right\}}
Since
Ω
{\displaystyle \Omega }
B
⊆
Ω
{\displaystyle B\subseteq \Omega }
x
∈
B
{\displaystyle x\in B}
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
B
R
(
x
)
¯
⊆
Ω
{\displaystyle {\overline {B_{R}(x)}}\subseteq \Omega }
By theorem 6.15, we obtain in this case:
sup
y
∈
Ω
u
(
y
)
=
u
(
x
)
=
1
V
d
(
R
)
∫
B
R
(
x
)
u
(
z
)
d
z
{\displaystyle \sup _{y\in \Omega }u(y)=u(x)={\frac {1}{V_{d}(R)}}\int _{B_{R}(x)}u(z)dz}
Further,
sup
y
∈
Ω
u
(
y
)
=
sup
y
∈
Ω
u
(
y
)
V
d
(
R
)
V
d
(
R
)
=
1
V
d
(
R
)
∫
B
r
(
x
)
sup
y
∈
Ω
u
(
y
)
d
z
{\displaystyle \sup _{y\in \Omega }u(y)=\sup _{y\in \Omega }u(y){\frac {V_{d}(R)}{V_{d}(R)}}={\frac {1}{V_{d}(R)}}\int _{B_{r}(x)}\sup _{y\in \Omega }u(y)dz}
, which is why
1
V
d
(
R
)
∫
B
R
(
x
)
u
(
z
)
d
z
=
∫
B
R
(
x
)
sup
y
∈
Ω
u
(
y
)
d
z
⇔
1
V
d
(
R
)
∫
B
R
(
x
)
(
u
(
z
)
−
sup
y
∈
Ω
u
(
y
)
)
d
z
=
0
{\displaystyle {\begin{aligned}{\frac {1}{V_{d}(R)}}\int _{B_{R}(x)}u(z)dz=\int _{B_{R}(x)}\sup _{y\in \Omega }u(y)dz\\\Leftrightarrow {\frac {1}{V_{d}(R)}}\int _{B_{R}(x)}(u(z)-\sup _{y\in \Omega }u(y))dz=0\end{aligned}}}
Since
∀
z
∈
Ω
:
sup
y
∈
Ω
u
(
y
)
≥
u
(
z
)
{\displaystyle \forall z\in \Omega :\sup _{y\in \Omega }u(y)\geq u(z)}
, we have even
0
=
1
V
d
(
R
)
∫
B
R
(
x
)
(
u
(
z
)
−
sup
y
∈
Ω
u
(
y
)
)
d
z
=
−
1
V
d
(
R
)
∫
B
R
(
x
)
|
u
(
z
)
−
sup
y
∈
Ω
u
(
y
)
|
d
z
{\displaystyle 0={\frac {1}{V_{d}(R)}}\int _{B_{R}(x)}(u(z)-\sup _{y\in \Omega }u(y))dz=-{\frac {1}{V_{d}(R)}}\int _{B_{R}(x)}|u(z)-\sup _{y\in \Omega }u(y)|dz}
By theorem 6.17 we conclude that
u
(
z
)
=
sup
y
∈
Ω
u
(
y
)
{\displaystyle u(z)=\sup _{y\in \Omega }u(y)}
almost everywhere in
B
R
(
x
)
{\displaystyle B_{R}(x)}
z
↦
u
(
z
)
−
sup
y
∈
Ω
u
(
y
)
{\displaystyle z\mapsto u(z)-\sup _{y\in \Omega }u(y)}
is continuous, even
u
(
z
)
=
sup
y
∈
Ω
u
(
y
)
{\displaystyle u(z)=\sup _{y\in \Omega }u(y)}
really everywhere in
B
R
(
x
)
{\displaystyle B_{R}(x)}
B
R
(
0
)
⊆
B
{\displaystyle B_{R}(0)\subseteq B}
x
∈
B
{\displaystyle x\in B}
B
{\displaystyle B}
Also,
B
=
u
−
1
(
{
sup
y
∈
Ω
u
(
y
)
}
)
{\displaystyle B=u^{-1}\left(\left\{\sup _{y\in \Omega }u(y)\right\}\right)}
and
u
{\displaystyle u}
B
{\displaystyle B}
Ω
{\displaystyle \Omega }
B
{\displaystyle B}
B
=
∅
{\displaystyle B=\emptyset }
B
=
Ω
{\displaystyle B=\Omega }
B
{\displaystyle B}
B
=
Ω
{\displaystyle B=\Omega }
◻
{\displaystyle \Box }
Proof : See exercise 7.
Proof :
Proof :
What we will do next is showing that every harmonic function
u
∈
C
2
(
O
)
{\displaystyle u\in {\mathcal {C}}^{2}(O)}
C
∞
(
O
)
{\displaystyle {\mathcal {C}}^{\infty }(O)}
Proof :
Proof :
Proof :
Theorem 6.31 :
Let
(
u
l
)
l
∈
N
{\displaystyle (u_{l})_{l\in \mathbb {N} }}
Proof :
The dirichlet problem for the Poisson equation is to find a solution for
{
−
Δ
u
(
x
)
=
f
(
x
)
x
∈
Ω
u
(
x
)
=
g
(
x
)
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta u(x)=f(x)&x\in \Omega \\u(x)=g(x)&x\in \partial \Omega \end{cases}}}
If
Ω
{\displaystyle \Omega }
{
−
Δ
u
(
x
)
=
f
(
x
)
x
∈
Ω
u
(
x
)
=
g
(
x
)
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta u(x)=f(x)&x\in \Omega \\u(x)=g(x)&x\in \partial \Omega \end{cases}}}
has a solution
u
1
{\displaystyle u_{1}}
Ω
{\displaystyle \Omega }
Proof :
Let
u
2
{\displaystyle u_{2}}
u
=
u
1
−
u
2
{\displaystyle u=u_{1}-u_{2}}
u
{\displaystyle u}
{
−
Δ
u
(
x
)
=
0
,
x
∈
Ω
u
(
x
)
=
0
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta u(x)=0&,x\in \Omega \\u(x)=0&x\in \partial \Omega \end{cases}}}
, since
−
Δ
(
u
1
(
x
)
−
u
2
(
x
)
)
=
−
Δ
u
1
(
x
)
−
(
−
Δ
u
2
(
x
)
)
=
f
(
x
)
−
f
(
x
)
=
0
{\displaystyle -\Delta (u_{1}(x)-u_{2}(x))=-\Delta u_{1}(x)-(-\Delta u_{2}(x))=f(x)-f(x)=0}
x
∈
Ω
{\displaystyle x\in \Omega }
u
1
(
x
)
−
u
2
(
x
)
=
g
(
x
)
−
g
(
x
)
=
0
{\displaystyle u_{1}(x)-u_{2}(x)=g(x)-g(x)=0}
x
∈
∂
Ω
{\displaystyle x\in \partial \Omega }
Due to the above corollary from the minimum and maximum principle, we obtain that
u
{\displaystyle u}
Ω
{\displaystyle \Omega }
u
1
(
x
)
−
u
2
(
x
)
=
0
⇔
u
1
(
x
)
=
u
2
(
x
)
{\displaystyle u_{1}(x)-u_{2}(x)=0\Leftrightarrow u_{1}(x)=u_{2}(x)}
Ω
{\displaystyle \Omega }
Let
Ω
⊆
R
d
{\displaystyle \Omega \subseteq \mathbb {R} ^{d}}
G
~
{\displaystyle {\tilde {G}}}
G
~
(
x
)
:=
{
−
1
2
|
x
|
d
=
1
−
1
2
π
ln
‖
x
‖
d
=
2
1
(
d
−
2
)
c
1
‖
x
‖
d
−
2
d
≥
3
{\displaystyle {\tilde {G}}(x):={\begin{cases}-{\frac {1}{2}}|x|&d=1\\-{\frac {1}{2\pi }}\ln \|x\|&d=2\\{\frac {1}{(d-2)c}}{\frac {1}{\|x\|^{d-2}}}&d\geq 3\end{cases}}}
, where
c
:=
∫
∂
B
1
(
0
)
1
d
z
{\displaystyle c:=\int _{\partial B_{1}(0)}1dz}
B
1
(
0
)
⊂
R
d
{\displaystyle B_{1}(0)\subset \mathbb {R} ^{d}}
Suppose there is a function
h
:
Ω
×
Ω
→
R
{\displaystyle h:\Omega \times \Omega \to \mathbb {R} }
{
−
Δ
h
(
x
,
ξ
)
=
0
x
∈
Ω
h
(
x
,
ξ
)
=
G
~
(
x
−
ξ
)
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta h(x,\xi )=0&x\in \Omega \\h(x,\xi )={\tilde {G}}(x-\xi )&x\in \partial \Omega \end{cases}}}
Then the Green's function of the first kind for
−
Δ
{\displaystyle -\Delta }
Ω
{\displaystyle \Omega }
G
~
Ω
(
x
,
ξ
)
:=
G
~
(
x
−
ξ
)
−
h
(
x
,
ξ
)
{\displaystyle {\tilde {G}}_{\Omega }(x,\xi ):={\tilde {G}}(x-\xi )-h(x,\xi )}
G
~
(
x
−
ξ
)
−
h
(
x
,
ξ
)
{\displaystyle {\tilde {G}}(x-\xi )-h(x,\xi )}
−
Δ
{\displaystyle -\Delta }
G
~
{\displaystyle {\tilde {G}}}
h
{\displaystyle h}
A property of this function is that it satisfies
{
−
Δ
G
~
Ω
(
x
,
ξ
)
=
0
x
∈
Ω
∖
{
ξ
}
G
~
Ω
(
x
,
ξ
)
=
0
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta {\tilde {G}}_{\Omega }(x,\xi )=0&x\in \Omega \setminus \{\xi \}\\{\tilde {G}}_{\Omega }(x,\xi )=0&x\in \partial \Omega \end{cases}}}
The second of these equations is clear from the definition, and the first follows recalling that we calculated above (where we calculated the Green's kernel), that
Δ
G
~
(
x
)
=
0
{\displaystyle \Delta {\tilde {G}}(x)=0}
x
≠
0
{\displaystyle x\neq 0}
Let
Ω
⊆
R
d
{\displaystyle \Omega \subseteq \mathbb {R} ^{d}}
u
∈
C
2
(
Ω
)
{\displaystyle u\in C^{2}(\Omega )}
{
−
Δ
u
(
x
)
=
f
(
x
)
x
∈
Ω
u
(
x
)
=
g
(
x
)
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta u(x)=f(x)&x\in \Omega \\u(x)=g(x)&x\in \partial \Omega \end{cases}}}
. Then the following representation formula for
u
{\displaystyle u}
u
(
ξ
)
=
∫
Ω
−
Δ
u
(
y
)
G
~
Ω
(
y
,
ξ
)
d
y
−
∫
∂
Ω
u
(
y
)
ν
(
y
)
∇
y
G
~
Ω
(
y
,
ξ
)
d
y
{\displaystyle u(\xi )=\int _{\Omega }-\Delta u(y){\tilde {G}}_{\Omega }(y,\xi )dy-\int _{\partial \Omega }u(y)\nu (y)\nabla _{y}{\tilde {G}}_{\Omega }(y,\xi )dy}
, where
G
~
Ω
{\displaystyle {\tilde {G}}_{\Omega }}
Ω
{\displaystyle \Omega }
Proof :
Let's define
J
(
ϵ
)
:=
∫
Ω
∖
B
ϵ
(
ξ
)
−
Δ
u
(
y
)
G
~
Ω
(
y
,
ξ
)
d
y
{\displaystyle J(\epsilon ):=\int _{\Omega \setminus B_{\epsilon }(\xi )}-\Delta u(y){\tilde {G}}_{\Omega }(y,\xi )dy}
. By the theorem of dominated convergence, we have that
lim
ϵ
→
0
J
(
ϵ
)
=
∫
Ω
−
Δ
u
(
y
)
G
~
Ω
(
y
,
ξ
)
d
y
{\displaystyle \lim _{\epsilon \to 0}J(\epsilon )=\int _{\Omega }-\Delta u(y){\tilde {G}}_{\Omega }(y,\xi )dy}
Using multi-dimensional integration by parts, it can be obtained that:
J
(
ϵ
)
=
−
∫
∂
Ω
G
~
Ω
(
y
,
ξ
)
⏟
=
0
⟨
∇
u
(
y
)
,
ν
(
y
)
⟩
d
y
+
∫
∂
B
ϵ
(
ξ
)
G
~
Ω
(
y
,
ξ
)
⟨
∇
u
(
y
)
,
y
−
ξ
‖
y
−
ξ
‖
⟩
d
y
+
∫
Ω
∖
B
ϵ
(
ξ
)
⟨
∇
u
(
y
)
,
∇
x
G
~
Ω
(
y
,
ξ
)
⟩
d
y
{\displaystyle J(\epsilon )=-\int _{\partial \Omega }\underbrace {{\tilde {G}}_{\Omega }(y,\xi )} _{=0}\langle \nabla u(y),\nu (y)\rangle dy+\int _{\partial B_{\epsilon }(\xi )}{\tilde {G}}_{\Omega }(y,\xi )\langle \nabla u(y),{\frac {y-\xi }{\|y-\xi \|}}\rangle dy+\int _{\Omega \setminus B_{\epsilon }(\xi )}\langle \nabla u(y),\nabla _{x}{\tilde {G}}_{\Omega }(y,\xi )\rangle dy}
=
∫
∂
B
ϵ
(
ξ
)
G
~
Ω
(
y
,
ξ
)
⟨
∇
u
(
y
)
,
y
−
ξ
‖
y
−
ξ
‖
⟩
d
y
⏟
:=
J
1
(
ϵ
)
−
∫
Ω
∖
B
ϵ
(
ξ
)
Δ
G
~
Ω
(
y
,
ξ
)
u
(
y
)
d
y
{\displaystyle =\underbrace {\int _{\partial B_{\epsilon }(\xi )}{\tilde {G}}_{\Omega }(y,\xi )\langle \nabla u(y),{\frac {y-\xi }{\|y-\xi \|}}\rangle dy} _{:=J_{1}(\epsilon )}-\int _{\Omega \setminus B_{\epsilon }(\xi )}\Delta {\tilde {G}}_{\Omega }(y,\xi )u(y)dy}
−
∫
∂
B
ϵ
(
ξ
)
u
(
y
)
⟨
∇
G
~
Ω
(
y
,
ξ
)
,
y
−
ξ
‖
y
−
ξ
‖
⟩
d
y
⏟
:=
J
2
(
ϵ
)
−
∫
∂
Ω
u
(
y
)
⟨
∇
G
~
Ω
(
y
,
ξ
)
,
ν
(
y
)
⟩
d
y
{\displaystyle -\underbrace {\int _{\partial B_{\epsilon }(\xi )}u(y)\langle \nabla {\tilde {G}}_{\Omega }(y,\xi ),{\frac {y-\xi }{\|y-\xi \|}}\rangle dy} _{:=J_{2}(\epsilon )}-\int _{\partial \Omega }u(y)\langle \nabla {\tilde {G}}_{\Omega }(y,\xi ),\nu (y)\rangle dy}
When we proved the formula for the Green's kernel of Poisson's equation, we had already shown that
lim
ϵ
→
0
−
J
2
(
ϵ
)
=
u
(
ξ
)
{\displaystyle \lim _{\epsilon \to 0}-J_{2}(\epsilon )=u(\xi )}
lim
ϵ
→
0
J
1
(
ϵ
)
=
0
{\displaystyle \lim _{\epsilon \to 0}J_{1}(\epsilon )=0}
The only additional thing which is needed to verify this is that
h
∈
C
∞
(
Ω
)
{\displaystyle h\in C^{\infty }(\Omega )}
G
~
{\displaystyle {\tilde {G}}}
ϵ
→
0
{\displaystyle \epsilon \to 0}
h
{\displaystyle h}
This proves the formula.
Harmonic functions on the ball: A special case of the Dirichlet problem [ edit | edit source ] Let's choose
h
(
x
,
ξ
)
=
G
~
(
‖
ξ
‖
r
(
x
−
r
2
‖
ξ
‖
2
ξ
)
)
{\displaystyle h(x,\xi )={\tilde {G}}\left({\frac {\|\xi \|}{r}}\left(x-{\frac {r^{2}}{\|\xi \|^{2}}}\xi \right)\right)}
Then
G
~
B
r
(
x
0
)
(
x
,
ξ
)
:=
G
~
(
x
−
ξ
)
−
h
(
x
−
x
0
,
ξ
−
x
0
)
{\displaystyle {\tilde {G}}_{B_{r}(x_{0})}(x,\xi ):={\tilde {G}}(x-\xi )-h(x-x_{0},\xi -x_{0})}
is a Green's function of the first kind for
B
r
(
x
0
)
{\displaystyle B_{r}(x_{0})}
Proof : Since
ξ
−
x
0
∈
B
r
(
0
)
⇒
r
2
‖
ξ
−
x
0
‖
2
(
ξ
−
x
0
)
∉
B
r
(
0
)
{\displaystyle \xi -x_{0}\in B_{r}(0)\Rightarrow {\frac {r^{2}}{\|\xi -x_{0}\|^{2}}}(\xi -x_{0})\notin B_{r}(0)}
∀
x
,
ξ
∈
B
r
(
0
)
:
−
Δ
x
h
(
x
−
x
0
,
ξ
−
x
0
)
=
0
{\displaystyle \forall x,\xi \in B_{r}(0):-\Delta _{x}h(x-x_{0},\xi -x_{0})=0}
Furthermore, we obtain:
∫
B
r
(
x
0
)
−
Δ
φ
(
x
)
G
~
Ω
(
x
,
ξ
)
d
x
=
∫
B
r
(
x
0
)
−
Δ
φ
(
x
)
G
~
(
x
−
ξ
)
d
x
+
∫
B
r
(
x
0
)
φ
(
x
)
−
Δ
h
(
x
,
ξ
)
d
x
=
φ
(
ξ
)
+
0
{\displaystyle \int _{B_{r}(x_{0})}-\Delta \varphi (x){\tilde {G}}_{\Omega }(x,\xi )dx=\int _{B_{r}(x_{0})}-\Delta \varphi (x){\tilde {G}}(x-\xi )dx+\int _{B_{r}(x_{0})}\varphi (x)-\Delta h(x,\xi )dx=\varphi (\xi )+0}
, which is why
G
~
Ω
(
x
,
ξ
)
{\displaystyle {\tilde {G}}_{\Omega }(x,\xi )}
The property for the boundary comes from the following calculation:
∀
x
∈
∂
B
r
(
0
)
:
‖
x
−
ξ
‖
2
=
⟨
x
−
ξ
,
x
−
ξ
⟩
=
r
2
+
‖
ξ
‖
2
−
2
⟨
x
,
ξ
⟩
=
‖
ξ
‖
2
r
2
(
⟨
x
−
r
2
‖
ξ
‖
2
ξ
,
x
−
r
2
‖
ξ
‖
2
ξ
⟩
)
=
‖
ξ
‖
2
r
2
‖
x
−
r
2
‖
ξ
‖
2
ξ
‖
2
{\displaystyle \forall x\in \partial B_{r}(0):\|x-\xi \|^{2}=\langle x-\xi ,x-\xi \rangle =r^{2}+\|\xi \|^{2}-2\langle x,\xi \rangle ={\frac {\|\xi \|^{2}}{r^{2}}}(\langle x-{\frac {r^{2}}{\|\xi \|^{2}}}\xi ,x-{\frac {r^{2}}{\|\xi \|^{2}}}\xi \rangle )={\frac {\|\xi \|^{2}}{r^{2}}}\|x-{\frac {r^{2}}{\|\xi \|^{2}}}\xi \|^{2}}
, which is why
x
∈
∂
B
r
(
0
)
⇒
h
(
x
,
ξ
)
=
G
~
(
x
,
ξ
)
{\displaystyle x\in \partial B_{r}(0)\Rightarrow h(x,\xi )={\tilde {G}}(x,\xi )}
G
~
{\displaystyle {\tilde {G}}}
Let's consider the following problem:
{
−
Δ
u
(
x
)
=
0
x
∈
B
r
(
0
)
u
(
x
)
=
φ
(
x
)
x
∈
∂
B
r
(
0
)
{\displaystyle {\begin{cases}-\Delta u(x)=0&x\in B_{r}(0)\\u(x)=\varphi (x)&x\in \partial B_{r}(0)\end{cases}}}
Here
φ
{\displaystyle \varphi }
∂
B
r
(
0
)
{\displaystyle \partial B_{r}(0)}
u
∈
C
(
B
r
(
0
)
¯
)
∩
C
2
(
B
r
(
0
)
)
{\displaystyle u\in C({\overline {B_{r}(0)}})\cap C^{2}(B_{r}(0))}
u
(
ξ
)
=
{
∫
∂
B
r
(
0
)
⟨
−
ν
(
y
)
,
∇
y
G
~
B
r
(
0
)
(
y
,
ξ
)
⟩
φ
(
y
)
d
y
ξ
∈
B
r
(
0
)
φ
(
ξ
)
ξ
∈
∂
B
r
(
0
)
{\displaystyle u(\xi )={\begin{cases}\int _{\partial B_{r}(0)}\langle -\nu (y),\nabla _{y}{\tilde {G}}_{B_{r}(0)}(y,\xi )\rangle \varphi (y)dy&\xi \in B_{r}(0)\\\varphi (\xi )&\xi \in \partial B_{r}(0)\end{cases}}}
Proof : Uniqueness we have already proven; we have shown that for all Dirichlet problems for
−
Δ
{\displaystyle -\Delta }
Therefore, it only remains to show that the above function is a solution to the problem. To do so, we note first that
−
Δ
∫
∂
B
r
(
0
)
⟨
−
ν
(
y
)
,
∇
~
y
G
B
r
(
0
)
(
y
,
ξ
)
⟩
φ
(
y
)
d
y
=
−
Δ
∫
∂
B
r
(
0
)
⟨
−
ν
(
y
)
,
∇
y
(
G
~
(
y
−
ξ
)
−
h
(
y
,
ξ
)
)
⟩
φ
(
y
)
d
y
{\displaystyle -\Delta \int _{\partial B_{r}(0)}\langle -\nu (y),{\tilde {\nabla }}_{y}G_{B_{r}(0)}(y,\xi )\rangle \varphi (y)dy=-\Delta \int _{\partial B_{r}(0)}\langle -\nu (y),\nabla _{y}({\tilde {G}}(y-\xi )-h(y,\xi ))\rangle \varphi (y)dy}
Let
0
<
s
<
r
{\displaystyle 0<s<r}
G
~
B
r
(
0
)
{\displaystyle {\tilde {G}}_{B_{r}(0)}}
B
s
(
0
)
{\displaystyle B_{s}(0)}
B
s
(
0
)
{\displaystyle B_{s}(0)}
B
s
(
0
)
{\displaystyle B_{s}(0)}
r
>
s
>
0
{\displaystyle r>s>0}
B
r
(
0
)
{\displaystyle B_{r}(0)}
−
Δ
u
(
ξ
)
=
∫
∂
B
r
(
0
)
−
Δ
(
⟨
−
ν
(
y
)
,
∇
~
y
G
~
(
x
−
ξ
)
−
h
(
x
,
ξ
)
⟩
φ
(
y
)
)
⏞
=
0
d
y
=
0
{\displaystyle -\Delta u(\xi )=\int _{\partial B_{r}(0)}\overbrace {-\Delta (\langle -\nu (y),{\tilde {\nabla }}_{y}{\tilde {G}}(x-\xi )-h(x,\xi )\rangle \varphi (y))} ^{=0}dy=0}
Furthermore, we have to show that
∀
x
∈
∂
B
r
(
0
)
:
lim
y
→
x
u
(
y
)
=
φ
(
x
)
{\displaystyle \forall x\in \partial B_{r}(0):\lim _{y\to x}u(y)=\varphi (x)}
u
{\displaystyle u}
To do this, we notice first that
∫
∂
B
r
(
0
)
⟨
−
ν
(
y
)
,
∇
y
G
~
B
r
(
0
)
(
y
,
ξ
)
⟩
d
y
=
1
{\displaystyle \int _{\partial B_{r}(0)}\langle -\nu (y),\nabla _{y}{\tilde {G}}_{B_{r}(0)}(y,\xi )\rangle dy=1}
This follows due to the fact that if
u
≡
1
{\displaystyle u\equiv 1}
u
{\displaystyle u}
{
−
Δ
u
(
x
)
=
0
x
∈
B
r
(
0
)
u
(
x
)
=
1
x
∈
∂
B
r
(
0
)
{\displaystyle {\begin{cases}-\Delta u(x)=0&x\in B_{r}(0)\\u(x)=1&x\in \partial B_{r}(0)\end{cases}}}
and the application of the representation formula.
Furthermore, if
‖
x
−
x
∗
‖
<
1
2
δ
{\displaystyle \|x-x^{*}\|<{\frac {1}{2}}\delta }
‖
y
−
x
∗
‖
≥
δ
{\displaystyle \|y-x^{*}\|\geq \delta }
‖
x
−
y
‖
≥
|
‖
y
−
x
∗
‖
−
‖
x
∗
−
x
‖
|
≥
1
2
δ
{\displaystyle \|x-y\|\geq |\|y-x^{*}\|-\|x^{*}-x\||\geq {\frac {1}{2}}\delta }
In addition, another application of the second triangle inequality gives:
(
r
2
−
‖
x
‖
2
)
=
(
r
+
‖
x
‖
)
(
r
−
‖
x
‖
)
=
(
r
+
‖
x
‖
)
(
‖
x
∗
‖
−
‖
x
‖
)
≤
2
r
‖
x
∗
−
x
‖
{\displaystyle (r^{2}-\|x\|^{2})=(r+\|x\|)(r-\|x\|)=(r+\|x\|)(\|x^{*}\|-\|x\|)\leq 2r\|x^{*}-x\|}
Let then
ϵ
>
0
{\displaystyle \epsilon >0}
x
∗
∈
∂
B
r
(
0
)
{\displaystyle x^{*}\in \partial B_{r}(0)}
φ
{\displaystyle \varphi }
δ
>
0
{\displaystyle \delta >0}
‖
x
−
x
∗
‖
<
δ
⇒
|
φ
(
x
)
−
φ
(
x
∗
)
|
<
ϵ
2
{\displaystyle \|x-x^{*}\|<\delta \Rightarrow |\varphi (x)-\varphi (x^{*})|<{\frac {\epsilon }{2}}}
In the end, with the help of all the previous estimations we have made, we may unleash the last chain of inequalities which shows that the representation formula is true:
|
u
(
x
)
−
u
(
x
∗
)
|
=
|
u
(
x
)
−
1
⋅
φ
(
x
∗
)
|
=
|
∫
∂
B
r
(
0
)
⟨
−
ν
(
y
)
,
∇
y
G
~
B
r
(
0
)
(
y
,
x
)
⟩
(
φ
(
x
)
−
φ
(
x
∗
)
)
d
y
|
{\displaystyle |u(x)-u(x^{*})|=|u(x)-1\cdot \varphi (x^{*})|=\left|\int _{\partial B_{r}(0)}\langle -\nu (y),\nabla _{y}{\tilde {G}}_{B_{r}(0)}(y,x)\rangle (\varphi (x)-\varphi (x^{*}))dy\right|}
≤
ϵ
2
∫
∂
B
r
(
0
)
∩
B
δ
(
x
∗
)
|
⟨
−
ν
(
y
)
,
∇
y
G
~
B
r
(
0
)
(
y
,
x
)
⟩
|
d
y
+
2
‖
φ
‖
∞
∫
∂
B
r
(
0
)
∖
B
δ
(
x
∗
)
|
⟨
−
ν
(
y
)
,
∇
y
G
~
B
r
(
0
)
(
y
,
x
)
⟩
|
d
y
{\displaystyle \leq {\frac {\epsilon }{2}}\int _{\partial B_{r}(0)\cap B_{\delta }(x^{*})}|\langle -\nu (y),\nabla _{y}{\tilde {G}}_{B_{r}(0)}(y,x)\rangle |dy+2\|\varphi \|_{\infty }\int _{\partial B_{r}(0)\setminus B_{\delta }(x^{*})}|\langle -\nu (y),\nabla _{y}{\tilde {G}}_{B_{r}(0)}(y,x)\rangle |dy}
≤
ϵ
2
+
2
‖
φ
‖
∞
∫
∂
B
r
(
0
)
∖
B
δ
(
x
∗
)
r
2
−
‖
x
‖
2
r
c
(
1
)
(
δ
2
)
d
d
y
≤
ϵ
2
+
2
‖
φ
‖
∞
r
d
−
2
r
2
−
‖
x
‖
2
(
δ
2
)
d
{\displaystyle \leq {\frac {\epsilon }{2}}+2\|\varphi \|_{\infty }\int _{\partial B_{r}(0)\setminus B_{\delta }(x^{*})}{\frac {r^{2}-\|x\|^{2}}{rc(1)\left({\frac {\delta }{2}}\right)^{d}}}dy\leq {\frac {\epsilon }{2}}+2\|\varphi \|_{\infty }r^{d-2}{\frac {r^{2}-\|x\|^{2}}{\left({\frac {\delta }{2}}\right)^{d}}}}
Since
x
→
x
∗
{\displaystyle x\to x^{*}}
r
2
−
‖
x
‖
2
→
0
{\displaystyle r^{2}-\|x\|^{2}\to 0}
x
{\displaystyle x}
x
∗
{\displaystyle x^{*}}
2
‖
φ
‖
∞
r
d
−
2
r
2
−
‖
x
‖
2
(
δ
2
)
d
<
ϵ
2
{\displaystyle 2\|\varphi \|_{\infty }r^{d-2}{\frac {r^{2}-\|x\|^{2}}{\left({\frac {\delta }{2}}\right)^{d}}}<{\frac {\epsilon }{2}}}
ϵ
>
0
{\displaystyle \epsilon >0}
Let
Ω
⊂
R
d
{\displaystyle \Omega \subset \mathbb {R} ^{d}}
b
:
R
d
→
R
{\displaystyle b:\mathbb {R} ^{d}\to \mathbb {R} }
barrier with respect to
y
∈
∂
Ω
{\displaystyle y\in \partial \Omega }
b
{\displaystyle b}
b
{\displaystyle b}
Ω
{\displaystyle \Omega }
b
(
y
)
=
0
{\displaystyle b(y)=0}
∀
x
∈
R
d
∖
Ω
:
b
(
x
)
>
0
{\displaystyle \forall x\in \mathbb {R} ^{d}\setminus \Omega :b(x)>0}
Let
Ω
⊆
R
d
{\displaystyle \Omega \subseteq \mathbb {R} ^{d}}
x
∈
∂
Ω
{\displaystyle x\in \partial \Omega }
B
r
(
z
)
⊆
R
d
∖
Ω
{\displaystyle B_{r}(z)\subseteq \mathbb {R} ^{d}\setminus \Omega }
x
∈
∂
B
r
(
z
)
{\displaystyle x\in \partial B_{r}(z)}
z
∈
R
d
∖
Ω
{\displaystyle z\in \mathbb {R} ^{d}\setminus \Omega }
r
∈
R
≥
0
{\displaystyle r\in \mathbb {R} _{\geq 0}}
Let
Ω
⊆
R
d
{\displaystyle \Omega \subseteq \mathbb {R} ^{d}}
v
∈
C
(
Ω
)
{\displaystyle v\in C(\Omega )}
We call
v
{\displaystyle v}
subharmonic if and only if:
v
(
x
)
≤
1
d
(
r
)
∫
B
r
(
x
)
v
(
y
)
d
y
{\displaystyle v(x)\leq {\frac {1}{d(r)}}\int _{B_{r}(x)}v(y)dy}
We call
v
{\displaystyle v}
superharmonic if and only if:
v
(
x
)
≥
1
d
(
r
)
∫
B
r
(
x
)
v
(
y
)
d
y
{\displaystyle v(x)\geq {\frac {1}{d(r)}}\int _{B_{r}(x)}v(y)dy}
From this definition we can see that a function is harmonic if and only if it is subharmonic and superharmonic.
A superharmonic function
u
{\displaystyle u}
Ω
{\displaystyle \Omega }
Ω
{\displaystyle \Omega }
∂
Ω
{\displaystyle \partial \Omega }
Proof : Almost the same as the proof of the minimum and maximum principle for harmonic functions. As an exercise, you might try to prove this minimum principle yourself.
Let
u
∈
S
φ
(
Ω
)
{\displaystyle u\in {\mathcal {S}}_{\varphi }(\Omega )}
B
r
(
x
0
)
⊂
Ω
{\displaystyle B_{r}(x_{0})\subset \Omega }
u
~
(
x
)
=
{
u
(
x
)
x
∉
B
r
(
x
0
)
∫
∂
B
r
(
0
)
⟨
−
ν
(
y
)
,
∇
y
G
~
B
r
(
0
)
(
y
,
x
)
⟩
φ
(
y
)
d
y
x
∈
B
r
(
x
0
)
{\displaystyle {\tilde {u}}(x)={\begin{cases}u(x)&x\notin B_{r}(x_{0})\\\int _{\partial B_{r}(0)}\langle -\nu (y),\nabla _{y}{\tilde {G}}_{B_{r}(0)}(y,x)\rangle \varphi (y)dy&x\in B_{r}(x_{0})\end{cases}}}
, then
u
~
∈
S
φ
(
Ω
)
{\displaystyle {\tilde {u}}\in {\mathcal {S}}_{\varphi }(\Omega )}
Proof : For this proof, the very important thing to notice is that the formula for
u
~
{\displaystyle {\tilde {u}}}
B
r
(
x
0
)
{\displaystyle B_{r}(x_{0})}
u
~
{\displaystyle {\tilde {u}}}
∂
Ω
{\displaystyle \partial \Omega }
u
~
∈
S
φ
(
Ω
)
{\displaystyle {\tilde {u}}\in {\mathcal {S}}_{\varphi }(\Omega )}
Let
φ
∈
C
(
∂
Ω
)
{\displaystyle \varphi \in C(\partial \Omega )}
S
φ
(
Ω
)
:=
{
u
∈
C
(
Ω
¯
)
:
u
superharmonic and
x
∈
∂
Ω
⇒
u
(
x
)
≥
φ
(
x
)
}
{\displaystyle {\mathcal {S}}_{\varphi }(\Omega ):=\{u\in C({\overline {\Omega }}):u{\text{ superharmonic and }}x\in \partial \Omega \Rightarrow u(x)\geq \varphi (x)\}}
S
φ
(
Ω
)
{\displaystyle {\mathcal {S}}_{\varphi }(\Omega )}
∀
u
∈
S
φ
(
Ω
)
:
∀
x
∈
Ω
:
u
(
x
)
≥
min
y
∈
∂
Ω
φ
(
y
)
{\displaystyle \forall u\in {\mathcal {S}}_{\varphi }(\Omega ):\forall x\in \Omega :u(x)\geq \min _{y\in \partial \Omega }\varphi (y)}
Proof : The first part follows by choosing the constant function
u
(
x
)
=
max
y
∈
∂
Ω
φ
(
y
)
{\displaystyle u(x)=\max _{y\in \partial \Omega }\varphi (y)}
Let
u
1
,
u
2
∈
S
φ
(
Ω
)
{\displaystyle u_{1},u_{2}\in {\mathcal {S}}_{\varphi }(\Omega )}
u
(
x
)
=
min
{
u
1
(
x
)
,
u
2
(
x
)
}
{\displaystyle u(x)=\min\{u_{1}(x),u_{2}(x)\}}
u
∈
S
φ
(
Ω
)
{\displaystyle u\in {\mathcal {S}}_{\varphi }(\Omega )}
Proof : The condition on the border is satisfied, because
∀
x
∈
∂
Ω
:
u
1
(
x
)
≥
φ
(
x
)
∧
u
2
(
x
)
≥
φ
(
x
)
{\displaystyle \forall x\in \partial \Omega :u_{1}(x)\geq \varphi (x)\wedge u_{2}(x)\geq \varphi (x)}
u
{\displaystyle u}
u
(
x
)
=
u
1
(
x
)
{\displaystyle u(x)=u_{1}(x)}
u
(
x
)
=
u
1
(
x
)
≥
1
d
(
r
)
∫
B
r
(
x
)
u
1
(
y
)
d
y
≥
1
d
(
r
)
∫
B
r
(
x
)
u
(
y
)
d
y
{\displaystyle u(x)=u_{1}(x)\geq {\frac {1}{d(r)}}\int _{B_{r}(x)}u_{1}(y)dy\geq {\frac {1}{d(r)}}\int _{B_{r}(x)}u(y)dy}
, due to the monotony of the integral. This argument is valid for all
x
∈
Ω
{\displaystyle x\in \Omega }
u
{\displaystyle u}
If
Ω
⊂
R
d
{\displaystyle \Omega \subset \mathbb {R} ^{d}}
φ
∈
C
(
∂
Ω
)
{\displaystyle \varphi \in C(\partial \Omega )}
u
(
x
)
=
inf
{
v
(
x
)
|
v
∈
S
φ
(
Ω
)
}
{\displaystyle u(x)=\inf\{v(x)|v\in {\mathcal {S}}_{\varphi }(\Omega )\}}
is harmonic.
Proof :
If
Ω
{\displaystyle \Omega }
y
∈
∂
Ω
{\displaystyle y\in \partial \Omega }
Let
Ω
⊂
R
d
{\displaystyle \Omega \subset \mathbb {R} ^{d}}
{
−
Δ
u
(
x
)
=
f
(
x
)
x
∈
Ω
u
(
x
)
=
g
(
x
)
x
∈
∂
Ω
{\displaystyle {\begin{cases}-\Delta u(x)=f(x)&x\in \Omega \\u(x)=g(x)&x\in \partial \Omega \end{cases}}}
has a solution
u
∈
C
∞
(
Ω
)
∩
C
(
Ω
¯
)
{\displaystyle u\in C^{\infty }(\Omega )\cap C({\overline {\Omega }})}
Proof :
Let's summarise the results of this section.
In the next chapter, we will have a look at the heat equation.
Prove theorem 6.3 using theorem 6.2 (Hint: Choose
V
(
x
)
=
W
(
x
)
f
(
x
)
{\displaystyle \mathbf {V} (x)=\mathbf {W} (x)f(x)}
Prove that
∀
n
∈
N
:
Γ
(
n
+
1
)
=
n
!
{\displaystyle \forall n\in \mathbb {N} :\Gamma (n+1)=n!}
n
!
{\displaystyle n!}
n
{\displaystyle n}
Calculate
V
d
′
(
R
)
{\displaystyle V_{d}'(R)}
Prove that for
d
=
1
{\displaystyle d=1}
P
d
{\displaystyle P_{d}}
For all
d
≥
2
{\displaystyle d\geq 2}
x
∈
R
d
∖
{
0
}
{\displaystyle x\in \mathbb {R} ^{d}\setminus \{0\}}
∇
P
d
(
x
)
{\displaystyle \nabla P_{d}(x)}
Δ
P
d
(
x
)
{\displaystyle \Delta P_{d}(x)}
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
f
:
O
→
R
d
{\displaystyle f:O\to \mathbb {R} ^{d}}
f
(
x
)
=
0
{\displaystyle f(x)=0}
O
{\displaystyle O}
f
(
x
)
=
0
{\displaystyle f(x)=0}
O
{\displaystyle O}
Prove theorem 6.20 by modelling your proof on the proof of theorem 6.19.
For all dimensions
d
≥
2
{\displaystyle d\geq 2}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
α
≤
β
{\displaystyle \alpha \leq \beta }
β
≤
α
{\displaystyle \beta \leq \alpha }
In this chapter, we introduce the Fourier transform. The Fourier transform transforms functions into other functions. It can be used to solve certain types of linear differential equations.
Definition 8.1 :
Let
f
∈
L
1
(
R
d
)
{\displaystyle f\in L^{1}(\mathbb {R} ^{d})}
Fourier transform of
f
{\displaystyle f}
f
^
:
R
d
→
R
,
f
^
(
y
)
:=
∫
R
d
f
(
x
)
e
−
2
π
i
x
⋅
y
d
x
{\displaystyle {\hat {f}}:\mathbb {R} ^{d}\to \mathbb {R} ,{\hat {f}}(y):=\int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ix\cdot y}dx}
We recall that
f
{\displaystyle f}
⇔
{\displaystyle \Leftrightarrow }
|
f
|
{\displaystyle |f|}
Now we're ready to prove the next theorem:
Theorem 8.2 : The Fourier transform of an integrable
f
{\displaystyle f}
Proof : Since
f
{\displaystyle f}
|
f
|
{\displaystyle |f|}
∀
x
,
y
∈
R
d
:
|
f
(
x
)
e
−
2
π
i
x
⋅
y
|
=
|
f
(
x
)
|
⋅
|
e
−
2
π
i
x
⋅
y
|
⏞
=
1
=
|
f
(
x
)
|
{\displaystyle \forall x,y\in \mathbb {R} ^{d}:|f(x)e^{-2\pi ix\cdot y}|=|f(x)|\cdot \overbrace {|e^{-2\pi ix\cdot y}|} ^{=1}=|f(x)|}
, and therefore
x
↦
|
f
(
x
)
e
−
2
π
i
x
⋅
y
|
{\displaystyle x\mapsto |f(x)e^{-2\pi ix\cdot y}|}
x
↦
f
(
x
)
e
−
2
π
i
x
⋅
y
{\displaystyle x\mapsto f(x)e^{-2\pi ix\cdot y}}
∫
R
d
f
(
x
)
e
−
2
π
i
x
⋅
y
d
x
=
f
^
(
y
)
{\displaystyle \int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ix\cdot y}dx={\hat {f}}(y)}
has a unique complex value, by definition of integrability.
◻
{\displaystyle \Box }
Theorem 8.3 : Let
f
∈
L
1
(
R
d
)
{\displaystyle f\in L^{1}(\mathbb {R} ^{d})}
f
{\displaystyle f}
f
^
{\displaystyle {\hat {f}}}
Proof :
|
∫
R
d
f
(
x
)
e
−
2
π
i
x
⋅
y
d
x
|
≤
∫
R
d
|
f
(
x
)
e
−
2
π
i
x
⋅
y
|
d
x
triangle ineq. for the
∫
=
∫
R
d
|
f
(
x
)
|
d
x
|
e
−
2
π
i
x
⋅
y
|
=
1
∈
R
f
∈
L
1
(
R
d
)
{\displaystyle {\begin{aligned}\left|\int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ix\cdot y}dx\right|&\leq \int _{\mathbb {R} ^{d}}\left|f(x)e^{-2\pi ix\cdot y}\right|dx&{\text{triangle ineq. for the }}\int \\&=\int _{\mathbb {R} ^{d}}|f(x)|dx&\left|e^{-2\pi ix\cdot y}\right|=1\\&\in \mathbb {R} &f\in L^{1}(\mathbb {R} ^{d})\end{aligned}}}
◻
{\displaystyle \Box }
Once we have calculated the Fourier transform
f
~
{\displaystyle {\tilde {f}}}
f
{\displaystyle f}
f
{\displaystyle f}
Definition 2.6 :
For a vector
x
=
(
x
1
,
…
,
x
d
)
∈
R
d
{\displaystyle x=(x_{1},\ldots ,x_{d})\in \mathbb {R} ^{d}}
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
x
α
{\displaystyle x^{\alpha }}
x
{\displaystyle x}
α
{\displaystyle \alpha }
x
α
:=
x
1
α
1
⋯
x
d
α
d
{\displaystyle x^{\alpha }:=x_{1}^{\alpha _{1}}\cdots x_{d}^{\alpha _{d}}}
Now we write down the calculation rules, using the following notation:
Notation 8.4 :
We write
f
(
x
)
→
g
(
y
)
{\displaystyle f(x)\rightarrow g(y)}
to mean the sentence 'the function
y
↦
g
(
y
)
{\displaystyle y\mapsto g(y)}
x
↦
f
(
x
)
{\displaystyle x\mapsto f(x)}
Proof : To prove the first rule, we only need one of the rules for the exponential function (and the symmetry of the standard dot product):
1.
f
(
x
)
e
−
2
π
i
h
⋅
x
→
∫
R
d
f
(
x
)
e
−
2
π
i
h
⋅
x
e
−
2
π
i
x
⋅
y
d
x
=
∫
R
d
f
(
x
)
e
−
2
π
i
x
⋅
(
y
+
h
)
d
x
=
f
^
(
y
+
h
)
{\displaystyle f(x)e^{-2\pi ih\cdot x}\rightarrow \int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ih\cdot x}e^{-2\pi ix\cdot y}dx=\int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ix\cdot (y+h)}dx={\hat {f}}(y+h)}
For the next two rules, we apply the general integration by substitution rule, using the diffeomorphisms
x
↦
x
−
h
{\displaystyle x\mapsto x-h}
x
↦
δ
−
1
x
{\displaystyle x\mapsto \delta ^{-1}x}
R
d
{\displaystyle \mathbb {R} ^{d}}
2.
f
(
x
+
h
)
→
∫
R
d
f
(
x
+
h
)
e
−
2
π
i
x
⋅
y
d
x
=
∫
R
d
f
(
x
)
e
−
2
π
i
(
x
−
h
)
⋅
y
d
x
=
e
2
π
i
h
⋅
y
∫
R
d
f
(
x
)
e
−
2
π
i
x
⋅
y
d
x
=
f
^
(
y
)
e
2
π
i
h
⋅
y
{\displaystyle f(x+h)\rightarrow \int _{\mathbb {R} ^{d}}f(x+h)e^{-2\pi ix\cdot y}dx=\int _{\mathbb {R} ^{d}}f(x)e^{-2\pi i(x-h)\cdot y}dx=e^{2\pi ih\cdot y}\int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ix\cdot y}dx={\hat {f}}(y)e^{2\pi ih\cdot y}}
3.
f
(
δ
x
)
→
∫
R
d
f
(
δ
x
)
e
−
2
π
i
x
⋅
y
d
x
=
∫
R
d
δ
−
d
f
(
x
)
e
−
2
π
i
(
δ
−
1
x
)
⋅
y
d
x
=
δ
−
d
∫
R
d
f
(
x
)
e
−
2
π
i
x
⋅
(
δ
−
1
y
)
d
x
=
δ
−
d
f
^
(
δ
−
1
y
)
{\displaystyle f(\delta x)\rightarrow \int _{\mathbb {R} ^{d}}f(\delta x)e^{-2\pi ix\cdot y}dx=\int _{\mathbb {R} ^{d}}\delta ^{-d}f(x)e^{-2\pi i(\delta ^{-1}x)\cdot y}dx=\delta ^{-d}\int _{\mathbb {R} ^{d}}f(x)e^{-2\pi ix\cdot (\delta ^{-1}y)}dx=\delta ^{-d}{\hat {f}}(\delta ^{-1}y)}
4.
∫
R
d
g
^
(
x
)
f
(
x
)
d
x
=
∫
R
d
∫
R
d
g
(
y
)
e
2
π
i
x
⋅
y
d
y
f
(
x
)
d
x
Def. of the Fourier transform
=
∫
R
d
∫
R
d
f
(
x
)
g
(
y
)
e
2
π
i
x
⋅
y
d
y
d
x
putting a constant inside the integral
=
∫
R
d
∫
R
d
f
(
x
)
g
(
y
)
e
2
π
i
x
⋅
y
d
x
d
y
Fubini
=
∫
R
d
g
(
y
)
∫
R
d
f
(
x
)
e
2
π
i
x
⋅
y
d
x
d
y
pulling a constant out of the integral
=
∫
R
d
g
(
y
)
f
^
(
y
)
d
y
Def. of the Fourier transform
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}{\hat {g}}(x)f(x)dx&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}g(y)e^{2\pi ix\cdot y}dyf(x)dx&{\text{Def. of the Fourier transform}}\\&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}f(x)g(y)e^{2\pi ix\cdot y}dydx&{\text{putting a constant inside the integral}}\\&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}f(x)g(y)e^{2\pi ix\cdot y}dxdy&{\text{Fubini}}\\&=\int _{\mathbb {R} ^{d}}g(y)\int _{\mathbb {R} ^{d}}f(x)e^{2\pi ix\cdot y}dxdy&{\text{pulling a constant out of the integral}}\\&=\int _{\mathbb {R} ^{d}}g(y){\hat {f}}(y)dy&{\text{Def. of the Fourier transform}}\\\end{aligned}}}
◻
{\displaystyle \Box }
In order to proceed with further rules for the Fourier transform which involve Schwartz functions, we first need some further properties of Schwartz functions.
Theorem 8.6 :
Let
ϕ
:
R
d
→
R
{\displaystyle \phi :\mathbb {R} ^{d}\to \mathbb {R} }
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
x
↦
x
α
∂
β
ϕ
(
x
)
{\displaystyle x\mapsto x^{\alpha }\partial _{\beta }\phi (x)}
is a Schwartz function as well.
Proof :
Let
ϱ
,
ς
∈
N
0
d
{\displaystyle \varrho ,\varsigma \in \mathbb {N} _{0}^{d}}
∂
ς
x
α
∂
β
ϕ
(
x
)
=
∑
ε
∈
N
0
d
ε
≤
ς
(
ς
ε
)
∂
ε
(
x
α
)
∂
ς
−
ε
∂
β
ϕ
(
x
)
{\displaystyle \partial _{\varsigma }x^{\alpha }\partial _{\beta }\phi (x)=\sum _{\varepsilon \in \mathbb {N} _{0}^{d} \atop \varepsilon \leq \varsigma }{\binom {\varsigma }{\varepsilon }}\partial _{\varepsilon }(x^{\alpha })\partial _{\varsigma -\varepsilon }\partial _{\beta }\phi (x)}
We note that for all
α
{\displaystyle \alpha }
ε
{\displaystyle \varepsilon }
∂
ε
(
x
α
)
{\displaystyle \partial _{\varepsilon }(x^{\alpha })}
x
{\displaystyle x}
ϕ
{\displaystyle \phi }
c
ε
{\displaystyle c_{\varepsilon }}
‖
x
ϱ
∂
ε
(
x
α
)
∂
ς
−
ε
∂
β
ϕ
‖
∞
≤
c
ε
{\displaystyle \|x^{\varrho }\partial _{\varepsilon }(x^{\alpha })\partial _{\varsigma -\varepsilon }\partial _{\beta }\phi \|_{\infty }\leq c_{\varepsilon }}
Hence, the triangle inequality for
‖
⋅
‖
∞
{\displaystyle \|\cdot \|_{\infty }}
‖
x
ϱ
∂
ς
x
α
∂
β
ϕ
‖
∞
≤
∑
ε
∈
N
0
d
ε
≤
ς
(
ς
ε
)
c
ε
{\displaystyle \|x^{\varrho }\partial _{\varsigma }x^{\alpha }\partial _{\beta }\phi \|_{\infty }\leq \sum _{\varepsilon \in \mathbb {N} _{0}^{d} \atop \varepsilon \leq \varsigma }{\binom {\varsigma }{\varepsilon }}c_{\varepsilon }}
◻
{\displaystyle \Box }
Theorem 8.7 :
Every Schwartz function is integrable.
Proof :
We use that if the absolute value of a function is almost everywhere smaller than the value of an integrable function, then the first function is integrable.
Let
ϕ
{\displaystyle \phi }
b
,
c
∈
R
>
0
{\displaystyle b,c\in \mathbb {R} _{>0}}
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
|
ϕ
(
x
)
|
≤
min
{
b
∏
j
=
1
d
|
x
j
|
−
2
,
c
}
{\displaystyle |\phi (x)|\leq \min \left\{b\prod _{j=1}^{d}|x_{j}|^{-2},c\right\}}
The latter function is integrable, and integrability of
ϕ
{\displaystyle \phi }
◻
{\displaystyle \Box }
Now we can prove all three of the following rules for the Fourier transform involving Schwartz functions.
Proof :
1.
For the first rule, we use induction over
|
α
|
{\displaystyle |\alpha |}
It is clear that the claim is true for
|
α
|
=
0
{\displaystyle |\alpha |=0}
ϕ
{\displaystyle \phi }
ϕ
{\displaystyle \phi }
We proceed to the induction step: Let
n
∈
N
0
{\displaystyle n\in \mathbb {N} _{0}}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
=
n
{\displaystyle |\alpha |=n}
β
∈
N
0
d
{\displaystyle \beta \in \mathbb {N} _{0}^{d}}
|
β
|
=
n
+
1
{\displaystyle |\beta |=n+1}
β
{\displaystyle \beta }
Remember that we have
∂
β
ϕ
:=
∂
x
1
β
1
⋯
∂
x
d
β
d
ϕ
{\displaystyle \partial _{\beta }\phi :=\partial _{x_{1}}^{\beta _{1}}\cdots \partial _{x_{d}}^{\beta _{d}}\phi }
k
∈
{
1
,
…
,
d
}
{\displaystyle k\in \{1,\ldots ,d\}}
β
k
>
0
{\displaystyle \beta _{k}>0}
|
β
|
=
0
{\displaystyle |\beta |=0}
e
k
:=
(
0
,
…
,
0
,
1
⏞
k
th entry
,
0
,
…
,
0
)
{\displaystyle e_{k}:=(0,\ldots ,0,\overbrace {1} ^{k{\text{th entry}}},0,\ldots ,0)}
α
:=
β
−
e
k
{\displaystyle \alpha :=\beta -e_{k}}
and obtain
∂
β
ϕ
=
∂
x
k
∂
α
ϕ
{\displaystyle \partial _{\beta }\phi =\partial _{x_{k}}\partial _{\alpha }\phi }
by Schwarz' theorem , which implies that one may interchange the order of partial derivation arbitrarily.
Let
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
∫
[
−
R
,
R
]
d
∂
x
k
∂
α
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
=
∫
[
−
R
,
R
]
d
−
1
∫
−
R
R
∂
x
k
∂
α
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
k
d
(
x
1
,
…
,
x
k
−
1
,
x
k
+
1
,
…
,
x
d
)
=
∫
[
−
R
,
R
]
d
−
1
(
(
∂
α
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
)
|
x
k
=
−
R
x
k
=
R
−
∫
−
R
R
∂
α
ϕ
(
x
)
(
−
2
π
i
y
k
)
e
−
2
π
i
x
⋅
y
d
x
k
)
d
(
x
1
,
…
,
x
k
−
1
,
x
k
+
1
,
…
,
x
d
)
{\displaystyle {\begin{aligned}\int _{[-R,R]^{d}}\partial _{x_{k}}\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx&=\int _{[-R,R]^{d-1}}\int _{-R}^{R}\partial _{x_{k}}\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx_{k}d(x_{1},\ldots ,x_{k-1},x_{k+1},\ldots ,x_{d})\\&=\int _{[-R,R]^{d-1}}\left(\left(\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}\right){\big |}_{x_{k}=-R}^{x_{k}=R}-\int _{-R}^{R}\partial _{\alpha }\phi (x)(-2\pi iy_{k})e^{-2\pi ix\cdot y}dx_{k}\right)d(x_{1},\ldots ,x_{k-1},x_{k+1},\ldots ,x_{d})\end{aligned}}}
Due to the dominated convergence theorem (with dominating function
x
↦
∂
x
k
∂
α
ϕ
(
x
)
{\displaystyle x\mapsto \partial _{x_{k}}\partial _{\alpha }\phi (x)}
∫
R
d
∂
x
k
∂
α
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
{\displaystyle \int _{\mathbb {R} ^{d}}\partial _{x_{k}}\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx}
as
R
→
∞
{\displaystyle R\to \infty }
ϕ
{\displaystyle \phi }
b
,
c
∈
R
>
0
{\displaystyle b,c\in \mathbb {R} _{>0}}
|
∂
α
ϕ
(
x
)
|
<
min
{
b
∏
j
=
1
j
≠
k
d
|
x
j
|
−
2
,
c
}
{\displaystyle |\partial _{\alpha }\phi (x)|<\min \left\{b\prod _{j=1 \atop j\neq k}^{d}|x_{j}|^{-2},c\right\}}
Hence, the function within the large parentheses in the right hand sinde of the last line of the last equation is dominated by the
L
1
(
R
d
−
1
)
{\displaystyle L^{1}(\mathbb {R} ^{d-1})}
(
x
1
,
…
,
x
k
−
1
,
x
k
+
1
,
…
,
x
d
)
↦
min
{
b
∏
j
=
1
j
≠
k
d
|
x
j
|
−
2
,
c
}
+
∫
−
∞
∞
|
∂
α
ϕ
(
x
)
(
−
2
π
i
y
k
)
|
d
x
k
{\displaystyle (x_{1},\ldots ,x_{k-1},x_{k+1},\ldots ,x_{d})\mapsto \min \left\{b\prod _{j=1 \atop j\neq k}^{d}|x_{j}|^{-2},c\right\}+\int _{-\infty }^{\infty }|\partial _{\alpha }\phi (x)(-2\pi iy_{k})|dx_{k}}
and hence, by the dominated convergence theorem, the integral over that function converges, as
R
→
∞
{\displaystyle R\to \infty }
∫
R
d
−
1
(
∫
−
∞
∞
∂
α
ϕ
(
x
)
(
2
π
i
y
k
)
e
−
2
π
i
x
⋅
y
d
x
k
)
d
(
x
1
,
…
,
x
k
−
1
,
x
k
+
1
,
…
,
x
d
)
=
∫
R
d
∂
α
ϕ
(
x
)
(
2
π
i
y
k
)
e
−
2
π
i
x
⋅
y
d
x
Fubini
=
∫
R
d
(
2
π
i
y
β
)
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
induction hypothesis
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d-1}}\left(\int _{-\infty }^{\infty }\partial _{\alpha }\phi (x)(2\pi iy_{k})e^{-2\pi ix\cdot y}dx_{k}\right)d(x_{1},\ldots ,x_{k-1},x_{k+1},\ldots ,x_{d})&=\int _{\mathbb {R} ^{d}}\partial _{\alpha }\phi (x)(2\pi iy_{k})e^{-2\pi ix\cdot y}dx&{\text{Fubini}}\\&=\int _{\mathbb {R} ^{d}}(2\pi iy^{\beta })\phi (x)e^{-2\pi ix\cdot y}dx&{\text{induction hypothesis}}\end{aligned}}}
From the uniqueness of limits of real sequences we obtain 1.
2.
We use again induction on
|
α
|
{\displaystyle |\alpha |}
|
α
|
=
0
{\displaystyle |\alpha |=0}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
=
n
{\displaystyle |\alpha |=n}
β
∈
N
0
d
{\displaystyle \beta \in \mathbb {N} _{0}^{d}}
|
β
|
=
n
+
1
{\displaystyle |\beta |=n+1}
k
∈
{
1
,
…
,
d
}
{\displaystyle k\in \{1,\ldots ,d\}}
β
k
>
0
{\displaystyle \beta _{k}>0}
α
:=
β
−
e
k
{\displaystyle \alpha :=\beta -e_{k}}
Theorems 8.6 and 8.7 imply that
for all
y
∈
R
d
{\displaystyle y\in \mathbb {R} ^{d}}
∫
R
d
|
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
|
d
x
<
∞
{\displaystyle \int _{\mathbb {R} ^{d}}|\phi (x)e^{-2\pi ix\cdot y}|dx<\infty }
for all
y
∈
R
d
{\displaystyle y\in \mathbb {R} ^{d}}
∫
R
d
|
ϕ
(
x
)
∂
y
k
e
−
2
π
i
x
⋅
y
|
d
x
<
∞
{\displaystyle \int _{\mathbb {R} ^{d}}|\phi (x)\partial _{y_{k}}e^{-2\pi ix\cdot y}|dx<\infty }
Further,
∂
y
k
(
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
)
{\displaystyle \partial _{y_{k}}(\phi (x)e^{-2\pi ix\cdot y})}
(
x
,
y
)
∈
R
d
×
R
d
{\displaystyle (x,y)\in \mathbb {R} ^{d}\times \mathbb {R} ^{d}}
Hence, Leibniz' integral rule implies:
∂
β
ϕ
^
(
y
)
=
∂
x
k
∂
α
ϕ
^
(
y
)
=
∂
y
k
∫
R
d
(
2
π
i
x
)
α
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
induction hypothesis
=
∫
R
d
∂
y
k
(
2
π
i
x
)
α
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
=
∫
R
d
(
2
π
i
x
)
β
ϕ
(
x
)
e
−
2
π
i
x
⋅
y
d
x
{\displaystyle {\begin{aligned}\partial _{\beta }{\hat {\phi }}(y)&=\partial _{x_{k}}\partial _{\alpha }{\hat {\phi }}(y)&\\&=\partial _{y_{k}}\int _{\mathbb {R} ^{d}}(2\pi ix)^{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx&{\text{induction hypothesis}}\\&=\int _{\mathbb {R} ^{d}}\partial _{y_{k}}(2\pi ix)^{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx&\\&=\int _{\mathbb {R} ^{d}}(2\pi ix)^{\beta }\phi (x)e^{-2\pi ix\cdot y}dx\end{aligned}}}
3.
ϕ
∗
θ
^
(
y
)
:=
∫
R
d
(
ϕ
∗
θ
)
(
x
)
e
−
2
π
i
x
⋅
y
d
x
Def. of Fourier transform
:=
∫
R
d
∫
R
d
ϕ
(
z
)
θ
(
x
−
z
)
d
z
e
−
2
π
i
x
⋅
y
d
x
Def. of convolution
=
∫
R
d
∫
R
d
e
−
2
π
i
x
⋅
y
ϕ
(
z
)
θ
(
x
−
z
)
d
z
d
x
linearity of the integral
=
∫
R
d
∫
R
d
e
−
2
π
i
x
⋅
y
ϕ
(
z
)
θ
(
x
−
z
)
d
x
d
z
Fubini
=
∫
R
d
∫
R
d
e
−
2
π
i
x
⋅
y
e
−
2
π
i
z
⋅
y
ϕ
(
z
)
θ
(
x
)
d
x
d
z
Integration by substitution using
x
↦
x
+
z
and
∀
b
,
c
∈
R
:
e
b
+
c
=
e
b
e
c
=
∫
R
d
∫
R
d
e
−
2
π
i
x
⋅
y
θ
(
x
)
d
x
⏞
=
θ
^
(
y
)
ϕ
(
z
)
e
−
2
π
i
z
⋅
y
d
z
pulling a constant out of the integral
=
θ
^
(
y
)
∫
R
d
ϕ
(
z
)
e
−
2
π
i
z
⋅
y
d
z
⏞
=
ϕ
^
(
y
)
pulling a constant out of the integral
{\displaystyle {\begin{aligned}{\widehat {\phi *\theta }}(y)&:=\int _{\mathbb {R} ^{d}}(\phi *\theta )(x)e^{-2\pi ix\cdot y}dx&{\text{Def. of Fourier transform}}\\&:=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}\phi (z)\theta (x-z)dze^{-2\pi ix\cdot y}dx&{\text{Def. of convolution}}\\&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}e^{-2\pi ix\cdot y}\phi (z)\theta (x-z)dzdx&{\text{linearity of the integral}}\\&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}e^{-2\pi ix\cdot y}\phi (z)\theta (x-z)dxdz&{\text{Fubini}}\\&=\int _{\mathbb {R} ^{d}}\int _{\mathbb {R} ^{d}}e^{-2\pi ix\cdot y}e^{-2\pi iz\cdot y}\phi (z)\theta (x)dxdz&{\text{Integration by substitution using }}x\mapsto x+z{\text{ and }}\forall b,c\in \mathbb {R} :e^{b+c}=e^{b}e^{c}\\&=\int _{\mathbb {R} ^{d}}\overbrace {\int _{\mathbb {R} ^{d}}e^{-2\pi ix\cdot y}\theta (x)dx} ^{={\hat {\theta }}(y)}\phi (z)e^{-2\pi iz\cdot y}dz&{\text{pulling a constant out of the integral}}\\&={\hat {\theta }}(y)\overbrace {\int _{\mathbb {R} ^{d}}\phi (z)e^{-2\pi iz\cdot y}dz} ^{={\hat {\phi }}(y)}&{\text{pulling a constant out of the integral}}\end{aligned}}}
◻
{\displaystyle \Box }
Proof :
Let
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
d
{\displaystyle d}
ϕ
∈
S
(
R
d
)
{\displaystyle \phi \in {\mathcal {S}}(\mathbb {R} ^{d})}
∂
α
(
(
−
2
π
i
x
)
β
ϕ
)
{\displaystyle \partial _{\alpha }((-2\pi \mathrm {i} x)^{\beta }\phi )}
x
α
∂
β
ϕ
^
=
∂
α
(
(
−
2
π
i
x
)
β
ϕ
)
^
{\displaystyle x^{\alpha }\partial _{\beta }{\hat {\phi }}={\widehat {\partial _{\alpha }((-2\pi \mathrm {i} x)^{\beta }\phi )}}}
By theorem 8.3,
∂
α
(
(
−
2
π
i
x
)
β
ϕ
)
^
{\displaystyle {\widehat {\partial _{\alpha }((-2\pi \mathrm {i} x)^{\beta }\phi )}}}
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
ϕ
^
∈
S
(
R
d
)
{\displaystyle {\hat {\phi }}\in {\mathcal {S}}(\mathbb {R} ^{d})}
◻
{\displaystyle \Box }
Definitions 8.10 :
We define the Fourier transform on the Schwartz space to be the function
F
:
S
(
R
d
)
→
S
(
R
d
)
,
F
(
ϕ
)
:=
ϕ
^
{\displaystyle {\mathcal {F}}:{\mathcal {S}}(\mathbb {R} ^{d})\to {\mathcal {S}}(\mathbb {R} ^{d}),{\mathcal {F}}(\phi ):={\hat {\phi }}}
Theorem 8.9 assures that this function really maps to
S
(
R
d
)
{\displaystyle {\mathcal {S}}(\mathbb {R} ^{d})}
inverse Fourier transform on the Schwartz space to be the function
F
−
1
:
S
(
R
d
)
→
S
(
R
d
)
,
F
(
ϕ
)
:=
x
↦
∫
R
d
ϕ
(
y
)
e
2
π
i
x
⋅
y
d
y
{\displaystyle {\mathcal {F}}^{-1}:{\mathcal {S}}(\mathbb {R} ^{d})\to {\mathcal {S}}(\mathbb {R} ^{d}),{\mathcal {F}}(\phi ):=x\mapsto \int _{\mathbb {R} ^{d}}\phi (y)e^{2\pi ix\cdot y}dy}
This function maps to
S
(
R
d
)
{\displaystyle {\mathcal {S}}(\mathbb {R} ^{d})}
F
−
1
(
ϕ
)
(
x
)
=
ϕ
^
(
−
x
)
{\displaystyle {\mathcal {F}}^{-1}(\phi )(x)={\hat {\phi }}(-x)}
Both the Fourier transform and the inverse Fourier transform are sequentially continuous:
Proof :
1. We prove
F
(
ϕ
l
)
→
F
(
ϕ
)
,
l
→
∞
{\displaystyle {\mathcal {F}}(\phi _{l})\to {\mathcal {F}}(\phi ),l\to \infty }
Let
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
x
α
∂
β
(
F
(
ϕ
l
)
(
x
)
−
F
(
ϕ
)
(
x
)
)
=
∫
R
d
∂
α
(
(
−
2
π
i
y
)
β
(
ϕ
l
(
y
)
−
ϕ
(
y
)
)
)
e
−
2
π
i
x
⋅
y
d
y
{\displaystyle x^{\alpha }\partial _{\beta }({\mathcal {F}}(\phi _{l})(x)-{\mathcal {F}}(\phi )(x))=\int _{\mathbb {R} ^{d}}\partial _{\alpha }((-2\pi iy)^{\beta }(\phi _{l}(y)-\phi (y)))e^{-2\pi ix\cdot y}dy}
As in the proof of theorem 8.3, we hence obtain
|
x
α
∂
β
(
F
(
ϕ
l
)
(
x
)
−
F
(
ϕ
)
(
x
)
)
|
≤
‖
∂
α
(
(
−
2
π
i
x
)
β
(
ϕ
l
−
ϕ
)
)
)
‖
L
1
{\displaystyle \left|x^{\alpha }\partial _{\beta }({\mathcal {F}}(\phi _{l})(x)-{\mathcal {F}}(\phi )(x))\right|\leq \|\partial _{\alpha }((-2\pi ix)^{\beta }(\phi _{l}-\phi )))\|_{L^{1}}}
Due to the multi-dimensional product rule,
∂
α
(
(
−
2
π
i
x
)
β
(
ϕ
l
(
x
)
−
ϕ
(
x
)
)
)
=
∑
ς
∈
N
0
d
ς
≤
α
(
ς
α
)
∂
ς
(
(
−
2
π
i
x
)
β
)
∂
α
−
ς
(
ϕ
l
(
x
)
−
ϕ
(
x
)
)
{\displaystyle \partial _{\alpha }((-2\pi ix)^{\beta }(\phi _{l}(x)-\phi (x)))=\sum _{\varsigma \in \mathbb {N} _{0}^{d} \atop \varsigma \leq \alpha }{\binom {\varsigma }{\alpha }}\partial _{\varsigma }((-2\pi ix)^{\beta })\partial _{\alpha -\varsigma }(\phi _{l}(x)-\phi (x))}
Let now
ϵ
>
0
{\displaystyle \epsilon >0}
ϕ
l
→
ϕ
{\displaystyle \phi _{l}\to \phi }
n
∈
{
1
,
…
,
d
}
{\displaystyle n\in \{1,\ldots ,d\}}
N
1
∈
N
{\displaystyle N_{1}\in \mathbb {N} }
∀
k
≥
N
1
:
∑
ς
∈
N
0
d
ς
≤
α
(
ς
α
)
‖
x
n
2
∂
ς
(
(
−
2
π
i
x
)
β
)
∂
α
−
ς
(
ϕ
l
(
x
)
−
ϕ
(
x
)
)
‖
∞
<
ϵ
{\displaystyle \forall k\geq N_{1}:\sum _{\varsigma \in \mathbb {N} _{0}^{d} \atop \varsigma \leq \alpha }{\binom {\varsigma }{\alpha }}\|x_{n}^{2}\partial _{\varsigma }((-2\pi ix)^{\beta })\partial _{\alpha -\varsigma }(\phi _{l}(x)-\phi (x))\|_{\infty }<\epsilon }
Further, we may choose
N
2
∈
N
{\displaystyle N_{2}\in \mathbb {N} }
∀
k
≥
N
2
:
∑
ς
∈
N
0
d
ς
≤
α
(
ς
α
)
‖
∂
ς
(
(
−
2
π
i
x
)
β
)
∂
α
−
ς
(
ϕ
l
(
x
)
−
ϕ
(
x
)
)
‖
∞
<
ϵ
{\displaystyle \forall k\geq N_{2}:\sum _{\varsigma \in \mathbb {N} _{0}^{d} \atop \varsigma \leq \alpha }{\binom {\varsigma }{\alpha }}\|\partial _{\varsigma }((-2\pi ix)^{\beta })\partial _{\alpha -\varsigma }(\phi _{l}(x)-\phi (x))\|_{\infty }<\epsilon }
Hence follows for
k
≥
N
:=
max
{
N
1
,
N
2
}
{\displaystyle k\geq N:=\max\{N_{1},N_{2}\}}
‖
∂
α
(
(
−
2
π
i
x
)
β
(
ϕ
l
−
ϕ
)
)
)
‖
L
1
:=
∫
R
d
|
∂
α
(
(
−
2
π
i
x
)
β
(
ϕ
l
(
x
)
−
ϕ
(
x
)
)
)
|
d
x
≤
∫
R
d
ϵ
min
{
x
1
−
2
,
…
,
x
d
−
2
,
1
}
d
x
=
ϵ
∫
R
d
min
{
x
1
−
2
,
…
,
x
d
−
2
,
1
}
d
x
{\displaystyle {\begin{aligned}\|\partial _{\alpha }((-2\pi ix)^{\beta }(\phi _{l}-\phi )))\|_{L^{1}}&:=\int _{\mathbb {R} ^{d}}|\partial _{\alpha }((-2\pi ix)^{\beta }(\phi _{l}(x)-\phi (x)))|dx\\&\leq \int _{\mathbb {R} ^{d}}\epsilon \min\{x_{1}^{-2},\ldots ,x_{d}^{-2},1\}dx\\&=\epsilon \int _{\mathbb {R} ^{d}}\min\{x_{1}^{-2},\ldots ,x_{d}^{-2},1\}dx\end{aligned}}}
Since
ϵ
>
0
{\displaystyle \epsilon >0}
F
(
ϕ
l
)
→
F
(
ϕ
)
,
l
→
∞
{\displaystyle {\mathcal {F}}(\phi _{l})\to {\mathcal {F}}(\phi ),l\to \infty }
2. From 1., we deduce
F
−
1
(
ϕ
l
)
→
F
−
1
(
ϕ
)
,
l
→
∞
{\displaystyle {\mathcal {F}}^{-1}(\phi _{l})\to {\mathcal {F}}^{-1}(\phi ),l\to \infty }
If
ϕ
l
→
ϕ
{\displaystyle \phi _{l}\to \phi }
θ
l
→
θ
{\displaystyle \theta _{l}\to \theta }
θ
l
(
x
)
:=
ϕ
l
(
−
x
)
{\displaystyle \theta _{l}(x):=\phi _{l}(-x)}
θ
(
x
)
:=
ϕ
(
−
x
)
{\displaystyle \theta (x):=\phi (-x)}
Therefore, by 1. and integration by substitution using the diffeomorphism
x
↦
−
x
{\displaystyle x\mapsto -x}
F
−
1
(
ϕ
l
)
=
F
(
θ
l
)
→
F
(
θ
)
=
F
−
1
(
ϕ
)
{\displaystyle {\mathcal {F}}^{-1}(\phi _{l})={\mathcal {F}}(\theta _{l})\to {\mathcal {F}}(\theta )={\mathcal {F}}^{-1}(\phi )}
◻
{\displaystyle \Box }
In the next theorem, we prove that
F
−
1
{\displaystyle {\mathcal {F}}^{-1}}
Lemma 8.12 :
If we define the function
G
:
R
d
→
R
,
G
(
x
)
:=
e
−
π
‖
x
‖
2
{\displaystyle G:\mathbb {R} ^{d}\to \mathbb {R} ,G(x):=e^{-\pi \|x\|^{2}}}
then
F
(
G
)
=
G
{\displaystyle {\mathcal {F}}(G)=G}
F
−
1
(
G
)
=
G
{\displaystyle {\mathcal {F}}^{-1}(G)=G}
Proof :
1.
F
−
1
(
G
)
=
G
{\displaystyle {\mathcal {F}}^{-1}(G)=G}
We define
μ
:
R
d
→
R
,
μ
(
ξ
)
:=
e
π
‖
ξ
‖
2
G
^
(
ξ
)
{\displaystyle \mu :\mathbb {R} ^{d}\to \mathbb {R} ,\mu (\xi ):=e^{\pi \|\xi \|^{2}}{\hat {G}}(\xi )}
By the product rule, we have for all
n
∈
{
1
,
…
,
d
}
{\displaystyle n\in \{1,\ldots ,d\}}
∂
ξ
n
μ
(
ξ
)
=
2
π
ξ
n
e
π
‖
ξ
‖
2
G
^
(
ξ
)
+
e
π
‖
ξ
‖
2
∂
ξ
n
G
^
(
ξ
)
{\displaystyle \partial _{\xi _{n}}\mu (\xi )=2\pi \xi _{n}e^{\pi \|\xi \|^{2}}{\hat {G}}(\xi )+e^{\pi \|\xi \|^{2}}\partial _{\xi _{n}}{\hat {G}}(\xi )}
Due to 1. of theorem 8.8, we have
2
π
ξ
n
G
^
(
ξ
)
=
−
i
∂
x
n
G
^
(
ξ
)
=
−
i
∫
R
d
(
2
π
x
n
)
e
−
π
‖
x
‖
2
e
2
π
i
ξ
⋅
x
d
x
{\displaystyle 2\pi \xi _{n}{\hat {G}}(\xi )=-i{\widehat {\partial _{x_{n}}G}}(\xi )=-i\int _{\mathbb {R} ^{d}}(2\pi x_{n})e^{-\pi \|x\|^{2}}e^{2\pi i\xi \cdot x}dx}
from 2. of theorem 8.8 we further obtain
∂
ξ
n
G
^
(
ξ
)
=
∫
R
d
(
−
2
π
i
x
n
)
e
−
π
‖
x
‖
2
e
2
π
i
ξ
⋅
x
d
x
{\displaystyle \partial _{\xi _{n}}{\hat {G}}(\xi )=\int _{\mathbb {R} ^{d}}(-2\pi ix_{n})e^{-\pi \|x\|^{2}}e^{2\pi i\xi \cdot x}dx}
Hence,
μ
{\displaystyle \mu }
μ
(
0
)
=
∫
R
d
e
−
π
‖
x
‖
2
d
x
=
∫
R
d
1
2
π
d
e
−
‖
x
‖
2
/
2
d
x
substitution using
x
↦
1
2
π
d
x
=
1
lemma 6.2
{\displaystyle {\begin{aligned}\mu (0)&=\int _{\mathbb {R} ^{d}}e^{-\pi \|x\|^{2}}dx&\\&=\int _{\mathbb {R} ^{d}}{\frac {1}{{\sqrt {2\pi }}^{d}}}e^{-\|x\|^{2}/2}dx&{\text{substitution using }}x\mapsto {\frac {1}{{\sqrt {2\pi }}^{d}}}x\\&=1&{\text{lemma 6.2}}\end{aligned}}}
2.
F
−
1
(
G
)
=
G
{\displaystyle {\mathcal {F}}^{-1}(G)=G}
By substitution using the diffeomorphism
x
↦
−
x
{\displaystyle x\mapsto -x}
∀
x
∈
R
d
:
F
−
1
(
G
)
(
x
)
=
F
(
G
)
(
x
)
=
G
(
x
)
{\displaystyle \forall x\in \mathbb {R} ^{d}:{\mathcal {F}}^{-1}(G)(x)={\mathcal {F}}(G)(x)=G(x)}
◻
{\displaystyle \Box }
For the next lemma, we need example 3.4 again, which is why we restate it:
Example 3.4: The standard mollifier
η
{\displaystyle \eta }
η
:
R
d
→
R
,
η
(
x
)
=
1
c
{
e
−
1
1
−
‖
x
‖
2
if
‖
x
‖
2
<
1
0
if
‖
x
‖
2
≥
1
{\displaystyle \eta :\mathbb {R} ^{d}\to \mathbb {R} ,\eta (x)={\frac {1}{c}}{\begin{cases}e^{-{\frac {1}{1-\|x\|^{2}}}}&{\text{ if }}\|x\|_{2}<1\\0&{\text{ if }}\|x\|_{2}\geq 1\end{cases}}}
, where
c
:=
∫
B
1
(
0
)
e
−
1
1
−
‖
x
‖
2
d
x
{\displaystyle c:=\int _{B_{1}(0)}e^{-{\frac {1}{1-\|x\|^{2}}}}dx}
Proof :
Let
α
,
β
∈
N
0
d
{\displaystyle \alpha ,\beta \in \mathbb {N} _{0}^{d}}
∂
β
(
ϕ
n
−
ϕ
)
=
∑
ς
∈
N
0
d
ς
≤
β
(
β
ς
)
1
n
|
β
−
ς
|
∂
β
−
ς
η
(
x
/
n
)
∂
ς
ϕ
(
x
)
−
∂
β
ϕ
(
x
)
{\displaystyle \partial _{\beta }(\phi _{n}-\phi )=\sum _{\varsigma \in \mathbb {N} _{0}^{d} \atop \varsigma \leq \beta }{\binom {\beta }{\varsigma }}{\frac {1}{n^{|\beta -\varsigma |}}}\partial _{\beta -\varsigma }\eta (x/n)\partial _{\varsigma }\phi (x)-\partial _{\beta }\phi (x)}
By the triangle inequality, we may hence deduce
|
x
α
∂
β
(
ϕ
n
−
ϕ
)
|
≤
1
n
∑
ς
∈
N
0
d
ς
<
β
(
β
ς
)
1
n
|
β
−
ς
|
−
1
|
x
α
∂
ς
ϕ
(
x
)
|
|
∂
β
−
ς
η
(
x
/
n
)
|
+
|
x
α
∂
β
ϕ
(
x
)
|
|
1
−
η
(
x
/
n
)
|
{\displaystyle |x^{\alpha }\partial _{\beta }(\phi _{n}-\phi )|\leq {\frac {1}{n}}\sum _{\varsigma \in \mathbb {N} _{0}^{d} \atop \varsigma <\beta }{\binom {\beta }{\varsigma }}{\frac {1}{n^{|\beta -\varsigma |-1}}}|x^{\alpha }\partial _{\varsigma }\phi (x)||\partial _{\beta -\varsigma }\eta (x/n)|+|x^{\alpha }\partial _{\beta }\phi (x)||1-\eta (x/n)|}
Since both
ϕ
{\displaystyle \phi }
η
{\displaystyle \eta }
ϱ
∈
N
0
d
{\displaystyle \varrho \in \mathbb {N} _{0}^{d}}
b
ϱ
,
c
ϱ
∈
R
{\displaystyle b_{\varrho },c_{\varrho }\in \mathbb {R} }
‖
∂
β
−
ϱ
η
‖
∞
<
b
ϱ
{\displaystyle \|\partial _{\beta -\varrho }\eta \|_{\infty }<b_{\varrho }}
‖
x
α
∂
ϱ
ϕ
‖
∞
<
c
ϱ
{\displaystyle \|x^{\alpha }\partial _{\varrho }\phi \|_{\infty }<c_{\varrho }}
Further, for each
k
∈
{
1
,
…
,
d
}
{\displaystyle k\in \{1,\ldots ,d\}}
c
k
∈
R
d
{\displaystyle c_{k}\in \mathbb {R} ^{d}}
‖
x
k
x
α
∂
β
ϕ
‖
∞
<
c
k
{\displaystyle \|x_{k}x^{\alpha }\partial _{\beta }\phi \|_{\infty }<c_{k}}
Let now
ϵ
>
0
{\displaystyle \epsilon >0}
N
1
∈
N
{\displaystyle N_{1}\in \mathbb {N} }
n
≥
N
1
{\displaystyle n\geq N_{1}}
1
n
∑
ς
∈
N
0
d
ς
<
β
(
β
ς
)
1
n
|
β
−
ς
|
−
1
b
ς
c
ς
<
ϵ
/
2
{\displaystyle {\frac {1}{n}}\sum _{\varsigma \in \mathbb {N} _{0}^{d} \atop \varsigma <\beta }{\binom {\beta }{\varsigma }}{\frac {1}{n^{|\beta -\varsigma |-1}}}b_{\varsigma }c_{\varsigma }<\epsilon /2}
Further, we choose
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
‖
x
‖
>
R
⇒
|
x
α
∂
β
ϕ
(
x
)
|
<
ϵ
/
2
{\displaystyle \|x\|>R\Rightarrow |x^{\alpha }\partial _{\beta }\phi (x)|<\epsilon /2}
This is possible since
|
x
α
∂
β
ϕ
(
x
)
|
≤
min
{
c
1
|
x
1
|
,
…
,
c
d
|
x
d
|
}
{\displaystyle |x^{\alpha }\partial _{\beta }\phi (x)|\leq \min \left\{{\frac {c_{1}}{|x_{1}|}},\ldots ,{\frac {c_{d}}{|x_{d}|}}\right\}}
due to our choice of
c
1
,
…
,
c
d
{\displaystyle c_{1},\ldots ,c_{d}}
Then we choose
N
2
∈
N
{\displaystyle N_{2}\in \mathbb {N} }
n
≥
N
2
{\displaystyle n\geq N_{2}}
∀
x
∈
B
R
(
0
)
:
|
1
−
ϕ
(
x
/
n
)
|
<
1
/
c
β
{\displaystyle \forall x\in B_{R}(0):|1-\phi (x/n)|<1/c_{\beta }}
Inserting all this in the above equation gives
|
x
α
∂
β
(
ϕ
n
−
ϕ
)
|
<
ϵ
{\displaystyle |x^{\alpha }\partial _{\beta }(\phi _{n}-\phi )|<\epsilon }
n
≥
N
:=
max
{
N
1
,
N
2
}
{\displaystyle n\geq N:=\max\{N_{1},N_{2}\}}
α
{\displaystyle \alpha }
β
{\displaystyle \beta }
ϵ
{\displaystyle \epsilon }
ϕ
n
→
ϕ
{\displaystyle \phi _{n}\to \phi }
◻
{\displaystyle \Box }
Theorem 8.14 :
Let
ϕ
∈
S
(
R
d
)
{\displaystyle \phi \in {\mathcal {S}}(\mathbb {R} ^{d})}
F
(
F
−
1
(
ϕ
)
)
=
ϕ
{\displaystyle {\mathcal {F}}({\mathcal {F}}^{-1}(\phi ))=\phi }
F
−
1
(
F
(
ϕ
)
)
=
ϕ
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))=\phi }
Proof :
1. We prove that if
ϕ
{\displaystyle \phi }
ϕ
(
0
)
=
0
{\displaystyle \phi (0)=0}
F
−
1
(
F
(
ϕ
)
)
(
0
)
=
0
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))(0)=0}
So let
ϕ
{\displaystyle \phi }
ϕ
(
x
)
=
ϕ
(
x
)
−
ϕ
(
0
)
=
∫
0
1
d
d
t
ϕ
(
t
x
)
d
x
=
∑
j
=
1
d
x
j
∫
0
1
∂
x
j
ϕ
(
t
x
)
d
t
{\displaystyle \phi (x)=\phi (x)-\phi (0)=\int _{0}^{1}{\frac {d}{dt}}\phi (tx)dx=\sum _{j=1}^{d}x_{j}\int _{0}^{1}\partial _{x_{j}}\phi (tx)dt}
Defining
ϕ
n
(
x
)
:=
η
(
x
/
n
)
ϕ
(
x
)
{\displaystyle \phi _{n}(x):=\eta (x/n)\phi (x)}
θ
j
,
n
(
x
)
:=
η
(
x
/
n
)
∫
0
1
∂
x
j
ϕ
(
t
x
)
d
t
{\displaystyle \theta _{j,n}(x):=\eta (x/n)\int _{0}^{1}\partial _{x_{j}}\phi (tx)dt}
and multiplying both sides of the above equation by
η
(
x
/
n
)
{\displaystyle \eta (x/n)}
ϕ
n
(
x
)
=
∑
j
=
1
d
x
j
θ
j
,
n
(
x
)
{\displaystyle \phi _{n}(x)=\sum _{j=1}^{d}x_{j}\theta _{j,n}(x)}
Since by repeated application of Leibniz' integral rule for all
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
∂
α
θ
j
,
n
(
x
)
=
∑
ς
≤
α
(
α
ς
)
1
n
|
ς
|
∂
ς
η
(
x
/
n
)
∫
0
1
∂
α
−
ς
∂
x
j
ϕ
(
t
x
)
d
t
{\displaystyle \partial _{\alpha }\theta _{j,n}(x)=\sum _{\varsigma \leq \alpha }{\binom {\alpha }{\varsigma }}{\frac {1}{n^{|\varsigma |}}}\partial _{\varsigma }\eta (x/n)\int _{0}^{1}\partial _{\alpha -\varsigma }\partial _{x_{j}}\phi (tx)dt}
all the
θ
j
,
n
{\displaystyle \theta _{j,n}}
F
(
ϕ
n
)
(
y
)
=
∑
j
=
1
d
F
(
x
j
θ
j
,
n
)
(
y
)
=
1
−
2
π
i
∑
j
=
1
d
∂
y
j
F
(
θ
j
,
n
)
(
y
)
{\displaystyle {\mathcal {F}}(\phi _{n})(y)=\sum _{j=1}^{d}{\mathcal {F}}(x_{j}\theta _{j,n})(y)={\frac {1}{-2\pi i}}\sum _{j=1}^{d}\partial _{y_{j}}{\mathcal {F}}(\theta _{j,n})(y)}
Hence,
F
−
1
(
F
(
ϕ
n
)
)
(
0
)
=
∫
R
d
F
(
ϕ
n
)
(
y
)
e
−
2
π
i
0
⋅
y
⏞
=
1
d
y
=
1
−
2
π
i
∑
j
=
1
d
∫
R
d
∂
y
j
F
(
θ
j
,
n
)
(
y
)
d
y
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi _{n}))(0)=\int _{\mathbb {R} ^{d}}{\mathcal {F}}(\phi _{n})(y)\overbrace {e^{-2\pi i0\cdot y}} ^{=1}dy={\frac {1}{-2\pi i}}\sum _{j=1}^{d}\int _{\mathbb {R} ^{d}}\partial _{y_{j}}{\mathcal {F}}(\theta _{j,n})(y)dy}
Let
k
∈
{
1
,
…
,
d
}
{\displaystyle k\in \{1,\ldots ,d\}}
θ
k
,
n
{\displaystyle \theta _{k,n}}
∫
R
d
∂
y
k
F
(
θ
k
,
n
)
(
y
)
d
y
=
∫
R
d
−
1
∫
−
∞
∞
∂
y
k
F
(
θ
k
,
n
)
(
y
)
d
y
k
d
(
y
1
,
…
,
y
k
−
1
,
y
k
+
1
,
…
,
y
d
)
=
0
{\displaystyle \int _{\mathbb {R} ^{d}}\partial _{y_{k}}{\mathcal {F}}(\theta _{k,n})(y)dy=\int _{\mathbb {R} ^{d-1}}\int _{-\infty }^{\infty }\partial _{y_{k}}{\mathcal {F}}(\theta _{k,n})(y)dy_{k}d(y_{1},\ldots ,y_{k-1},y_{k+1},\ldots ,y_{d})=0}
If we let
n
→
∞
{\displaystyle n\to \infty }
2. We deduce from 1. that if
ϕ
{\displaystyle \phi }
F
−
1
(
F
(
ϕ
)
)
(
0
)
=
ϕ
(
0
)
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))(0)=\phi (0)}
As in lemma 8.12, we define
G
:
R
d
→
R
,
G
(
x
)
:=
e
−
π
‖
x
‖
2
{\displaystyle G:\mathbb {R} ^{d}\to \mathbb {R} ,G(x):=e^{-\pi \|x\|^{2}}}
Let now
ϕ
{\displaystyle \phi }
ϕ
−
ϕ
(
0
)
G
{\displaystyle \phi -\phi (0)G}
G
(
0
)
=
1
{\displaystyle G(0)=1}
F
−
1
(
F
(
ϕ
−
ϕ
(
0
)
G
)
)
(
0
)
=
0
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi -\phi (0)G))(0)=0}
Further, due to lemma 8.12 and the linearity of the Fourier transform,
0
=
F
−
1
(
F
(
ϕ
−
ϕ
(
0
)
G
)
)
(
0
)
=
F
−
1
(
F
(
ϕ
)
)
−
ϕ
(
0
)
G
(
0
)
{\displaystyle 0={\mathcal {F}}^{-1}({\mathcal {F}}(\phi -\phi (0)G))(0)={\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))-\phi (0)G(0)}
3. We deduce from 2. that if
ϕ
{\displaystyle \phi }
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
F
−
1
(
F
(
ϕ
)
)
(
x
)
=
ϕ
(
x
)
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))(x)=\phi (x)}
F
−
1
(
F
(
ϕ
)
)
=
ϕ
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))=\phi }
Let
ϕ
∈
S
(
R
d
)
{\displaystyle \phi \in {\mathcal {S}}(\mathbb {R} ^{d})}
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
F
−
1
{\displaystyle {\mathcal {F}}^{-1}}
F
−
1
(
F
(
ϕ
)
)
(
x
)
=
∫
R
d
F
(
ϕ
)
(
y
)
e
2
π
i
x
⋅
y
d
y
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))(x)=\int _{\mathbb {R} ^{d}}{\mathcal {F}}(\phi )(y)e^{2\pi ix\cdot y}dy}
Further, if we define
θ
(
z
)
:=
ϕ
(
z
+
x
)
{\displaystyle \theta (z):=\phi (z+x)}
F
(
ϕ
)
(
y
)
e
2
π
i
x
⋅
y
=
∫
R
d
ϕ
(
z
)
e
−
2
π
i
z
⋅
y
e
2
π
i
x
⋅
y
d
z
=
∫
R
d
θ
(
z
)
d
z
e
−
2
π
i
z
⋅
y
=
F
(
θ
)
(
y
)
{\displaystyle {\mathcal {F}}(\phi )(y)e^{2\pi ix\cdot y}=\int _{\mathbb {R} ^{d}}\phi (z)e^{-2\pi iz\cdot y}e^{2\pi ix\cdot y}dz=\int _{\mathbb {R} ^{d}}\theta (z)dze^{-2\pi iz\cdot y}={\mathcal {F}}(\theta )(y)}
Hence, by 2.,
F
−
1
(
F
(
ϕ
)
)
(
x
)
=
∫
R
d
F
(
θ
)
(
y
)
d
y
=
F
−
1
(
F
(
θ
)
)
(
0
)
=
θ
(
0
)
=
ϕ
(
x
)
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))(x)=\int _{\mathbb {R} ^{d}}{\mathcal {F}}(\theta )(y)dy={\mathcal {F}}^{-1}({\mathcal {F}}(\theta ))(0)=\theta (0)=\phi (x)}
4. We deduce from 3. that for any Schwartz function
ϕ
{\displaystyle \phi }
F
(
F
−
1
(
ϕ
)
)
=
ϕ
{\displaystyle {\mathcal {F}}({\mathcal {F}}^{-1}(\phi ))=\phi }
Let
ϕ
∈
S
(
R
d
)
{\displaystyle \phi \in {\mathcal {S}}(\mathbb {R} ^{d})}
x
∈
R
d
{\displaystyle x\in \mathbb {R} ^{d}}
F
(
F
−
1
(
ϕ
)
)
(
y
)
=
F
−
1
(
F
−
1
(
ϕ
)
)
(
−
y
)
=
∫
R
d
F
−
1
(
ϕ
)
(
x
)
e
−
2
π
i
x
⋅
y
d
x
=
∫
R
d
F
(
ϕ
)
(
−
x
)
e
−
2
π
i
x
⋅
y
d
x
=
F
−
1
(
F
(
ϕ
)
)
(
y
)
=
ϕ
(
y
)
{\displaystyle {\mathcal {F}}({\mathcal {F}}^{-1}(\phi ))(y)={\mathcal {F}}^{-1}({\mathcal {F}}^{-1}(\phi ))(-y)=\int _{\mathbb {R} ^{d}}{\mathcal {F}}^{-1}(\phi )(x)e^{-2\pi ix\cdot y}dx=\int _{\mathbb {R} ^{d}}{\mathcal {F}}(\phi )(-x)e^{-2\pi ix\cdot y}dx={\mathcal {F}}^{-1}({\mathcal {F}}(\phi ))(y)=\phi (y)}
◻
{\displaystyle \Box }
Definition 8.15 :
Let
T
{\displaystyle {\mathcal {T}}}
F
(
T
)
(
ϕ
)
:=
T
(
F
(
ϕ
)
)
{\displaystyle {\mathcal {F}}({\mathcal {T}})(\phi ):={\mathcal {T}}({\mathcal {F}}(\phi ))}
Theorem 8.16 :
F
(
T
)
{\displaystyle {\mathcal {F}}({\mathcal {T}})}
Proof :
1. Sequential continuity follows from the sequential continuity of
T
{\displaystyle {\mathcal {T}}}
F
{\displaystyle {\mathcal {F}}}
2. Linearity follows from the linearity of
T
{\displaystyle {\mathcal {T}}}
F
{\displaystyle {\mathcal {F}}}
◻
{\displaystyle \Box }
Definition 8.17 :
Let
T
{\displaystyle {\mathcal {T}}}
F
−
1
(
T
)
(
ϕ
)
:=
T
(
F
−
1
(
ϕ
)
)
{\displaystyle {\mathcal {F}}^{-1}({\mathcal {T}})(\phi ):={\mathcal {T}}({\mathcal {F}}^{-1}(\phi ))}
For
x
1
,
…
,
x
n
{\displaystyle x_{1},\ldots ,x_{n}}
x
k
≠
x
m
{\displaystyle x_{k}\neq x_{m}}
k
≠
m
{\displaystyle k\neq m}
Theorem 10.2 :
Let
A
{\displaystyle \mathbf {A} }
x
1
,
…
,
x
n
{\displaystyle x_{1},\ldots ,x_{n}}
B
{\displaystyle \mathbf {B} }
k
,
m
{\displaystyle k,m}
b
k
,
m
:=
{
∑
1
≤
l
1
<
⋯
<
l
n
−
m
≤
n
l
1
,
…
,
l
n
−
m
≠
k
(
−
1
)
m
−
1
x
l
1
⋯
x
l
n
−
m
x
k
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
k
)
m
<
n
1
x
k
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
k
)
m
=
n
{\displaystyle \mathbf {b} _{k,m}:={\begin{cases}{\frac {\sum _{1\leq l_{1}<\cdots <l_{n-m}\leq n \atop l_{1},\ldots ,l_{n-m}\neq k}(-1)^{m-1}x_{l_{1}}\cdots x_{l_{n-m}}}{x_{k}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{k})}}&m<n\\{\frac {1}{x_{k}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{k})}}&m=n\end{cases}}}
is the inverse matrix of
A
{\displaystyle \mathbf {A} }
Proof :
We prove that
B
A
=
I
n
{\displaystyle \mathbf {B} \mathbf {A} =\mathbf {I} _{n}}
I
n
{\displaystyle \mathbf {I} _{n}}
n
×
n
{\displaystyle n\times n}
Let
1
≤
k
,
m
≤
n
{\displaystyle 1\leq k,m\leq n}
x
m
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
m
)
=
∑
j
=
1
n
x
m
j
{
∑
1
≤
l
1
<
⋯
<
l
n
−
j
≤
n
l
1
,
…
,
l
n
−
j
≠
k
(
−
1
)
j
−
1
x
l
1
⋯
x
l
n
−
j
j
<
n
1
j
=
n
{\displaystyle x_{m}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{m})=\sum _{j=1}^{n}x_{m}^{j}{\begin{cases}\sum _{1\leq l_{1}<\cdots <l_{n-j}\leq n \atop l_{1},\ldots ,l_{n-j}\neq k}(-1)^{j-1}x_{l_{1}}\cdots x_{l_{n-j}}&j<n\\1&j=n\end{cases}}}
Therefore, if
c
k
,
m
{\displaystyle \mathbf {c} _{k,m}}
k
,
m
{\displaystyle k,m}
B
A
{\displaystyle \mathbf {B} \mathbf {A} }
c
k
,
m
=
∑
j
=
1
n
x
m
j
{
∑
1
≤
l
1
<
⋯
<
l
n
−
j
≤
n
l
1
,
…
,
l
n
−
j
≠
k
(
−
1
)
j
−
1
x
l
1
⋯
x
l
n
−
j
j
<
n
1
j
=
n
x
k
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
k
)
=
x
m
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
m
)
x
k
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
k
)
=
{
1
k
=
m
0
k
≠
m
{\displaystyle \mathbf {c} _{k,m}=\sum _{j=1}^{n}{\frac {x_{m}^{j}{\begin{cases}\sum _{1\leq l_{1}<\cdots <l_{n-j}\leq n \atop l_{1},\ldots ,l_{n-j}\neq k}(-1)^{j-1}x_{l_{1}}\cdots x_{l_{n-j}}&j<n\\1&j=n\end{cases}}}{x_{k}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{k})}}={\frac {x_{m}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{m})}{x_{k}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{k})}}={\begin{cases}1&k=m\\0&k\neq m\end{cases}}}
◻
{\displaystyle \Box }
Lemma 10.3 :
Let
x
1
,
…
,
x
n
∈
R
{\displaystyle x_{1},\ldots ,x_{n}\in \mathbb {R} }
(
x
1
⋯
x
n
x
1
2
⋯
x
n
2
⋮
⋱
⋮
x
1
n
⋯
x
n
n
)
(
y
1
⋮
y
n
)
=
(
0
⋮
0
1
)
{\displaystyle {\begin{pmatrix}x_{1}&\cdots &x_{n}\\x_{1}^{2}&\cdots &x_{n}^{2}\\\vdots &\ddots &\vdots \\x_{1}^{n}&\cdots &x_{n}^{n}\end{pmatrix}}{\begin{pmatrix}y_{1}\\\vdots \\y_{n}\end{pmatrix}}={\begin{pmatrix}0\\\vdots \\0\\1\end{pmatrix}}}
is given by
y
k
=
1
x
k
∏
1
≤
l
≤
n
l
≠
k
(
x
l
−
x
k
)
{\displaystyle y_{k}={\frac {1}{x_{k}\prod _{1\leq l\leq n \atop l\neq k}(x_{l}-x_{k})}}}
k
∈
{
1
,
…
,
n
}
{\displaystyle k\in \{1,\ldots ,n\}}
Proof :
We multiply both sides of the equation by
B
{\displaystyle \mathbf {B} }
B
{\displaystyle \mathbf {B} }
B
{\displaystyle \mathbf {B} }
(
x
1
⋯
x
n
x
1
2
⋯
x
n
2
⋮
⋱
⋮
x
1
n
⋯
x
n
n
)
{\displaystyle {\begin{pmatrix}x_{1}&\cdots &x_{n}\\x_{1}^{2}&\cdots &x_{n}^{2}\\\vdots &\ddots &\vdots \\x_{1}^{n}&\cdots &x_{n}^{n}\end{pmatrix}}}
we end up with the equation
(
y
1
⋮
y
n
)
=
B
(
0
⋮
0
1
)
{\displaystyle {\begin{pmatrix}y_{1}\\\vdots \\y_{n}\end{pmatrix}}=\mathbf {B} {\begin{pmatrix}0\\\vdots \\0\\1\end{pmatrix}}}
Calculating the last expression directly leads to the desired formula.
◻
{\displaystyle \Box }
There are some partial differential equations which have no solution. However, some of them have something like ‘almost a solution’, which we call a weak solution . Among these there are partial differential equations whose weak solutions model processes in nature, just like solutions of partial differential equations which have a solution.
These weak solutions will be elements of the so-called Sobolev spaces . By proving properties which elements of Sobolev spaces in general have, we will thus obtain properties of weak solutions to partial differential equations, which therefore are properties of some processes in nature.
In this chapter we do show some properties of elements of Sobolev spaces. Furthermore, we will show that Sobolev spaces are Banach spaces (this will help us in the next section, where we investigate existence and uniqueness of weak solutions).
But first we shall repeat the definition of the standard mollifier defined in chapter 3.
Example 3.4: The standard mollifier
η
{\displaystyle \eta }
η
:
R
d
→
R
,
η
(
x
)
=
1
c
{
e
−
1
1
−
‖
x
‖
2
if
‖
x
‖
2
<
1
0
if
‖
x
‖
2
≥
1
{\displaystyle \eta :\mathbb {R} ^{d}\to \mathbb {R} ,\eta (x)={\frac {1}{c}}{\begin{cases}e^{-{\frac {1}{1-\|x\|^{2}}}}&{\text{ if }}\|x\|_{2}<1\\0&{\text{ if }}\|x\|_{2}\geq 1\end{cases}}}
, where
c
:=
∫
B
1
(
0
)
e
−
1
1
−
‖
x
‖
2
d
x
{\displaystyle c:=\int _{B_{1}(0)}e^{-{\frac {1}{1-\|x\|^{2}}}}dx}
Definition 3.13 :
For
R
∈
R
>
0
{\displaystyle R\in \mathbb {R} _{>0}}
η
R
:
R
d
→
R
,
η
R
(
x
)
=
η
(
x
R
)
/
R
d
{\displaystyle \eta _{R}:\mathbb {R} ^{d}\to \mathbb {R} ,\eta _{R}(x)=\eta \left({\frac {x}{R}}\right){\big /}R^{d}}
Lemma 12.1 : (to be replaced by characteristic function version)
Let
g
∈
L
p
{\displaystyle g\in L^{p}}
simple function , i. e.
g
=
∑
j
=
1
n
b
j
χ
I
j
{\displaystyle g=\sum _{j=1}^{n}b_{j}\chi _{I_{j}}}
where
I
j
{\displaystyle I_{j}}
χ
{\displaystyle \chi }
indicator function . If
ϵ
<
1
/
2
diam
(
I
j
)
{\displaystyle \epsilon <1/2{\text{diam}}(I_{j})}
then
‖
g
∗
η
ϵ
−
g
‖
p
≤
2
ϵ
max
k
∈
{
1
,
…
,
n
}
b
k
{\displaystyle \|g*\eta _{\epsilon }-g\|_{p}\leq 2\epsilon \max _{k\in \{1,\ldots ,n\}}b_{k}}
The following lemma, which is important for some theorems about Sobolev spaces, is known as the fundamental lemma of the calculus of variations:
Lemma 12.2 :
Let
S
⊆
R
d
{\displaystyle S\subseteq \mathbb {R} ^{d}}
f
,
g
:
S
→
R
{\displaystyle f,g:S\to \mathbb {R} }
f
,
g
∈
L
loc
1
(
S
)
{\displaystyle f,g\in L_{\text{loc}}^{1}(S)}
T
f
=
T
g
{\displaystyle {\mathcal {T}}_{f}={\mathcal {T}}_{g}}
f
=
g
{\displaystyle f=g}
Proof :
We define
h
:
R
d
→
R
,
h
(
x
)
:=
{
f
(
x
)
−
g
(
x
)
x
∈
S
0
x
∉
S
{\displaystyle h:\mathbb {R} ^{d}\to \mathbb {R} ,h(x):={\begin{cases}f(x)-g(x)&x\in S\\0&x\notin S\end{cases}}}
Remarks 12.2 : If
f
∈
L
p
(
S
)
{\displaystyle f\in L^{p}(S)}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
d
{\displaystyle d}
α
{\displaystyle \alpha }
f
{\displaystyle f}
∂
α
f
{\displaystyle \partial _{\alpha }f}
α
{\displaystyle \alpha }
f
{\displaystyle f}
Proof :
1. We prove that any two
α
{\displaystyle \alpha }
Let
g
,
h
∈
L
p
(
S
)
{\displaystyle g,h\in L^{p}(S)}
α
{\displaystyle \alpha }
f
{\displaystyle f}
T
g
=
∂
α
T
f
=
T
h
{\displaystyle {\mathcal {T}}_{g}=\partial _{\alpha }{\mathcal {T}}_{f}={\mathcal {T}}_{h}}
Notation 12.3 If it exists, we denote the
α
{\displaystyle \alpha }
f
{\displaystyle f}
∂
α
f
{\displaystyle \partial _{\alpha }f}
Theorem 12.4 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
p
∈
[
1
,
∞
]
{\displaystyle p\in [1,\infty ]}
f
,
g
∈
L
p
(
O
)
{\displaystyle f,g\in L^{p}(O)}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
f
,
g
{\displaystyle f,g}
α
{\displaystyle \alpha }
∂
α
f
{\displaystyle \partial _{\alpha }f}
∂
α
g
{\displaystyle \partial _{\alpha }g}
b
,
c
∈
R
{\displaystyle b,c\in \mathbb {R} }
∂
α
(
b
f
+
c
g
)
=
b
∂
α
f
+
c
∂
α
g
{\displaystyle \partial _{\alpha }(bf+cg)=b\partial _{\alpha }f+c\partial _{\alpha }g}
Proof :
Definition and theorem 12.6 :
Let
O
⊆
R
d
{\displaystyle O\subseteq \mathbb {R} ^{d}}
p
∈
[
1
,
∞
]
{\displaystyle p\in [1,\infty ]}
f
,
g
∈
L
p
(
O
)
{\displaystyle f,g\in L^{p}(O)}
n
∈
N
0
{\displaystyle n\in \mathbb {N} _{0}}
Sobolev space
W
n
,
p
(
O
)
{\displaystyle {\mathcal {W}}^{n,p}(O)}
is defined as follows:
W
n
,
p
(
O
)
:=
{
f
∈
L
p
(
O
)
:
∀
α
∈
N
0
d
such that
|
α
|
≤
n
:
∂
α
f
exists
}
{\displaystyle {\mathcal {W}}^{n,p}(O):=\{f\in L^{p}(O):\forall \alpha \in \mathbb {N} _{0}^{d}{\text{ such that }}|\alpha |\leq n:\partial _{\alpha }f{\text{ exists}}\}}
A norm on
W
n
,
p
(
O
)
{\displaystyle {\mathcal {W}}^{n,p}(O)}
‖
f
‖
W
n
,
p
(
O
)
:=
∑
|
α
|
≤
n
‖
∂
α
f
‖
L
p
(
O
)
{\displaystyle \|f\|_{{\mathcal {W}}^{n,p}(O)}:=\sum _{|\alpha |\leq n}\left\|\partial _{\alpha }f\right\|_{L^{p}(O)}}
With respect to this norm,
W
n
,
p
(
O
)
{\displaystyle {\mathcal {W}}^{n,p}(O)}
In the above definition,
∂
α
f
{\displaystyle \partial _{\alpha }f}
α
{\displaystyle \alpha }
f
{\displaystyle f}
Proof :
1.
We show that
‖
f
‖
W
n
,
p
(
O
)
=
∑
|
α
|
≤
n
‖
∂
α
f
‖
L
p
(
O
)
{\displaystyle \|f\|_{{\mathcal {W}}^{n,p}(O)}=\sum _{|\alpha |\leq n}\left\|\partial _{\alpha }f\right\|_{L^{p}(O)}}
is a norm.
We have to check the three defining properties for a norm:
‖
f
‖
W
n
,
p
(
O
)
=
0
⇔
f
=
0
{\displaystyle \|f\|_{{\mathcal {W}}^{n,p}(O)}=0\Leftrightarrow f=0}
‖
c
f
‖
W
n
,
p
(
O
)
=
|
c
|
‖
f
‖
W
n
,
p
(
O
)
{\displaystyle \|cf\|_{{\mathcal {W}}^{n,p}(O)}=|c|\|f\|_{{\mathcal {W}}^{n,p}(O)}}
c
∈
R
{\displaystyle c\in \mathbb {R} }
‖
f
+
g
‖
W
n
,
p
(
O
)
≤
‖
f
‖
W
n
,
p
(
O
)
+
‖
g
‖
W
n
,
p
(
O
)
{\displaystyle \|f+g\|_{{\mathcal {W}}^{n,p}(O)}\leq \|f\|_{{\mathcal {W}}^{n,p}(O)}+\|g\|_{{\mathcal {W}}^{n,p}(O)}}
We start with definiteness: If
f
=
0
{\displaystyle f=0}
‖
f
‖
W
n
,
p
(
O
)
=
0
{\displaystyle \|f\|_{{\mathcal {W}}^{n,p}(O)}=0}
‖
f
‖
W
n
,
p
(
O
)
=
0
{\displaystyle \|f\|_{{\mathcal {W}}^{n,p}(O)}=0}
‖
f
‖
L
p
(
O
)
=
0
{\displaystyle \|f\|_{L^{p}(O)}=0}
f
=
0
{\displaystyle f=0}
‖
f
‖
L
p
(
O
)
{\displaystyle \|f\|_{L^{p}(O)}}
We proceed to absolute homogeneity. Let
c
∈
R
{\displaystyle c\in \mathbb {R} }
‖
c
f
‖
W
n
,
p
(
O
)
:=
∑
|
α
|
≤
n
‖
∂
α
c
f
‖
L
p
(
O
)
=
∑
|
α
|
≤
n
‖
c
∂
α
f
‖
L
p
(
O
)
theorem 12.4
=
∑
|
α
|
≤
n
|
c
|
‖
∂
α
f
‖
L
p
(
O
)
by absolute homogeneity of
‖
⋅
‖
L
p
(
O
)
=
|
c
|
∑
|
α
|
≤
n
‖
∂
α
f
‖
L
p
(
O
)
=:
|
c
|
‖
f
‖
W
n
,
p
(
O
)
{\displaystyle {\begin{aligned}\|cf\|_{{\mathcal {W}}^{n,p}(O)}&:=\sum _{|\alpha |\leq n}\left\|\partial _{\alpha }cf\right\|_{L^{p}(O)}&\\&=\sum _{|\alpha |\leq n}\left\|c\partial _{\alpha }f\right\|_{L^{p}(O)}&{\text{ theorem 12.4}}\\&=\sum _{|\alpha |\leq n}|c|\left\|\partial _{\alpha }f\right\|_{L^{p}(O)}&{\text{ by absolute homogeneity of }}\|\cdot \|_{L^{p}(O)}\\&=|c|\sum _{|\alpha |\leq n}\left\|\partial _{\alpha }f\right\|_{L^{p}(O)}&\\&=:|c|\|f\|_{{\mathcal {W}}^{n,p}(O)}\end{aligned}}}
And the triangle inequality has to be shown:
‖
f
+
g
‖
W
n
,
p
(
O
)
:=
∑
|
α
|
≤
n
‖
∂
α
(
f
+
g
)
‖
L
p
(
O
)
=
∑
|
α
|
≤
n
‖
∂
α
f
+
∂
α
g
‖
L
p
(
O
)
theorem 12.4
≤
∑
|
α
|
≤
n
(
‖
∂
α
f
‖
L
p
(
O
)
+
‖
∂
α
g
‖
L
p
(
O
)
)
by triangle inequality of
‖
⋅
‖
L
p
(
O
)
=
‖
f
‖
W
n
,
p
(
O
)
+
‖
g
‖
W
n
,
p
(
O
)
{\displaystyle {\begin{aligned}\|f+g\|_{{\mathcal {W}}^{n,p}(O)}&:=\sum _{|\alpha |\leq n}\left\|\partial _{\alpha }(f+g)\right\|_{L^{p}(O)}&\\&=\sum _{|\alpha |\leq n}\left\|\partial _{\alpha }f+\partial _{\alpha }g\right\|_{L^{p}(O)}&{\text{ theorem 12.4}}\\&\leq \sum _{|\alpha |\leq n}\left(\left\|\partial _{\alpha }f\right\|_{L^{p}(O)}+\left\|\partial _{\alpha }g\right\|_{L^{p}(O)}\right)&{\text{ by triangle inequality of }}\|\cdot \|_{L^{p}(O)}\\&=\|f\|_{{\mathcal {W}}^{n,p}(O)}+\|g\|_{{\mathcal {W}}^{n,p}(O)}\end{aligned}}}
2.
We prove that
W
n
,
p
(
O
)
{\displaystyle {\mathcal {W}}^{n,p}(O)}
Let
(
f
l
)
l
∈
N
{\displaystyle (f_{l})_{l\in \mathbb {N} }}
W
n
,
p
(
O
)
{\displaystyle {\mathcal {W}}^{n,p}(O)}
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
≤
n
{\displaystyle |\alpha |\leq n}
m
,
l
∈
N
{\displaystyle m,l\in \mathbb {N} }
‖
∂
α
f
l
−
∂
α
f
m
)
‖
L
p
(
O
)
=
‖
∂
α
(
f
l
−
f
m
)
‖
L
p
(
O
)
≤
∑
|
α
|
≤
n
‖
∂
α
(
f
l
−
f
m
)
‖
L
p
(
O
)
{\displaystyle \|\partial _{\alpha }f_{l}-\partial _{\alpha }f_{m})\|_{L^{p}(O)}=\|\partial _{\alpha }(f_{l}-f_{m})\|_{L^{p}(O)}\leq \sum _{|\alpha |\leq n}\left\|\partial _{\alpha }(f_{l}-f_{m})\right\|_{L^{p}(O)}}
since we only added non-negative terms, we obtain that for all
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
≤
n
{\displaystyle |\alpha |\leq n}
(
∂
α
f
l
)
l
∈
N
{\displaystyle (\partial _{\alpha }f_{l})_{l\in \mathbb {N} }}
L
p
(
O
)
{\displaystyle L^{p}(O)}
L
p
(
O
)
{\displaystyle L^{p}(O)}
L
p
(
O
)
{\displaystyle L^{p}(O)}
f
α
{\displaystyle f_{\alpha }}
We show now that
f
:=
f
(
0
,
…
,
0
)
∈
W
n
,
p
(
O
)
{\displaystyle f:=f_{(0,\ldots ,0)}\in {\mathcal {W}}^{n,p}(O)}
f
l
→
f
,
l
→
∞
{\displaystyle f_{l}\to f,l\to \infty }
‖
⋅
‖
W
n
,
p
(
O
)
{\displaystyle \|\cdot \|_{{\mathcal {W}}^{n,p}(O)}}
W
n
,
p
(
O
)
{\displaystyle {\mathcal {W}}^{n,p}(O)}
To do so, we show that for all
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
≤
n
{\displaystyle |\alpha |\leq n}
α
{\displaystyle \alpha }
f
{\displaystyle f}
f
α
{\displaystyle f_{\alpha }}
f
l
→
f
,
l
→
∞
⇔
‖
f
l
−
f
‖
W
n
,
p
(
O
)
→
0
,
l
→
∞
⇔
∑
|
α
|
≤
n
‖
∂
α
(
f
l
−
f
)
‖
L
p
(
O
)
→
0
,
l
→
∞
⇔
∑
|
α
|
≤
n
‖
∂
α
f
l
−
∂
α
f
‖
L
p
(
O
)
→
0
,
l
→
∞
by theorem 12.4
{\displaystyle {\begin{aligned}f_{l}\to f,l\to \infty &\Leftrightarrow \|f_{l}-f\|_{{\mathcal {W}}^{n,p}(O)}\to 0,l\to \infty &\\&\Leftrightarrow \sum _{|\alpha |\leq n}\left\|\partial _{\alpha }(f_{l}-f)\right\|_{L^{p}(O)}\to 0,l\to \infty &\\&\Leftrightarrow \sum _{|\alpha |\leq n}\left\|\partial _{\alpha }f_{l}-\partial _{\alpha }f\right\|_{L^{p}(O)}\to 0,l\to \infty &{\text{by theorem 12.4}}\\\end{aligned}}}
where in the last line all the summands converge to zero provided that
∂
α
f
=
f
α
{\displaystyle \partial _{\alpha }f=f_{\alpha }}
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
≤
n
{\displaystyle |\alpha |\leq n}
Let
φ
∈
D
(
O
)
{\displaystyle \varphi \in {\mathcal {D}}(O)}
∂
α
f
l
→
f
α
{\displaystyle \partial _{\alpha }f_{l}\to f_{\alpha }}
‖
∂
α
f
−
f
α
‖
≥
|
‖
∂
α
f
‖
−
‖
f
α
‖
|
{\displaystyle \|\partial _{\alpha }f-f_{\alpha }\|\geq |\|\partial _{\alpha }f\|-\|f_{\alpha }\||}
, the sequence
(
φ
∂
α
f
l
)
l
∈
N
{\displaystyle (\varphi \partial _{\alpha }f_{l})_{l\in \mathbb {N} }}
l
{\displaystyle l}
2
‖
φ
‖
∞
f
α
{\displaystyle 2\|\varphi \|_{\infty }f_{\alpha }}
(
∂
α
φ
f
l
)
l
∈
N
{\displaystyle (\partial _{\alpha }\varphi f_{l})_{l\in \mathbb {N} }}
2
‖
∂
α
φ
‖
∞
f
{\displaystyle 2\|\partial _{\alpha }\varphi \|_{\infty }f}
incomplete: Why are the dominating functions L1?
Therefore
∫
R
d
∂
α
φ
(
x
)
f
(
x
)
d
x
=
lim
l
→
∞
∫
R
d
∂
α
φ
(
x
)
f
l
(
x
)
d
x
dominated convergence
=
lim
l
→
∞
(
−
1
)
|
α
|
∫
R
d
φ
(
x
)
∂
α
f
l
(
x
)
d
x
=
(
−
1
)
|
α
|
∫
R
d
φ
(
x
)
f
α
(
x
)
d
x
dominated convergence
{\displaystyle {\begin{aligned}\int _{\mathbb {R} ^{d}}\partial _{\alpha }\varphi (x)f(x)dx=&\lim _{l\to \infty }\int _{\mathbb {R} ^{d}}\partial _{\alpha }\varphi (x)f_{l}(x)dx&{\text{ dominated convergence}}\\&=\lim _{l\to \infty }(-1)^{|\alpha |}\int _{\mathbb {R} ^{d}}\varphi (x)\partial _{\alpha }f_{l}(x)dx&\\&=(-1)^{|\alpha |}\int _{\mathbb {R} ^{d}}\varphi (x)f_{\alpha }(x)dx&{\text{ dominated convergence}}\end{aligned}}}
, which is why
f
α
{\displaystyle f_{\alpha }}
α
{\displaystyle \alpha }
f
{\displaystyle f}
d
{\displaystyle d}
α
∈
N
0
d
{\displaystyle \alpha \in \mathbb {N} _{0}^{d}}
|
α
|
≤
n
{\displaystyle |\alpha |\leq n}
◻
{\displaystyle \Box }
We shall now prove that for any
L
p
{\displaystyle L^{p}}
L
p
{\displaystyle L^{p}}
approximation by simple functions and lemma 12.1, ||f_eps-f|| le ||f_eps - g_eps|| + ||g_eps - g|| + ||g - f||
Let
Ω
⊂
R
d
{\displaystyle \Omega \subset \mathbb {R} ^{d}}
r
>
0
{\displaystyle r>0}
U
⊂
Ω
{\displaystyle U\subset \Omega }
U
+
B
r
(
0
)
⊆
Ω
{\displaystyle U+B_{r}(0)\subseteq \Omega }
u
∈
W
m
,
p
(
U
)
{\displaystyle u\in {\mathcal {W}}^{m,p}(U)}
μ
ϵ
∗
f
{\displaystyle \mu _{\epsilon }*f}
C
∞
(
U
)
{\displaystyle C^{\infty }(U)}
ϵ
<
r
{\displaystyle \epsilon <r}
lim
ϵ
→
0
‖
μ
ϵ
∗
f
−
f
‖
W
m
,
p
(
U
)
=
0
{\displaystyle \lim _{\epsilon \to 0}\|\mu _{\epsilon }*f-f\|_{W^{m,p}(U)}=0}
Proof : The first claim, that
μ
ϵ
∗
f
∈
C
∞
(
U
)
{\displaystyle \mu _{\epsilon }*f\in C^{\infty }(U)}
f
~
(
x
)
=
{
f
(
x
)
x
∈
U
0
x
∉
U
{\displaystyle {\tilde {f}}(x)={\begin{cases}f(x)&x\in U\\0&x\notin U\end{cases}}}
Then, due to the above section about mollifying
L
p
{\displaystyle L^{p}}
The second claim follows from the following calculation, using the one-dimensional chain rule:
∂
α
∂
x
α
(
μ
ϵ
∗
f
)
(
y
)
=
∫
R
d
∂
α
∂
x
α
μ
ϵ
(
y
−
x
)
f
(
x
)
d
x
=
(
−
1
)
|
α
|
∫
R
d
∂
α
∂
y
α
μ
ϵ
(
y
−
x
)
f
(
x
)
d
x
{\displaystyle {\frac {\partial ^{\alpha }}{\partial x^{\alpha }}}(\mu _{\epsilon }*f)(y)=\int _{\mathbb {R} ^{d}}{\frac {\partial ^{\alpha }}{\partial x^{\alpha }}}\mu _{\epsilon }(y-x)f(x)dx=(-1)^{|\alpha |}\int _{\mathbb {R} ^{d}}{\frac {\partial ^{\alpha }}{\partial y^{\alpha }}}\mu _{\epsilon }(y-x)f(x)dx}
=
∫
R
d
μ
ϵ
(
y
−
x
)
∂
α
∂
y
α
f
(
x
)
d
x
=
(
μ
ϵ
∗
∂
α
∂
y
α
f
)
(
y
)
{\displaystyle =\int _{\mathbb {R} ^{d}}\mu _{\epsilon }(y-x){\frac {\partial ^{\alpha }}{\partial y^{\alpha }}}f(x)dx=(\mu _{\epsilon }*{\frac {\partial ^{\alpha }}{\partial y^{\alpha }}}f)(y)}
Due to the above secion about mollifying
L
p
{\displaystyle L^{p}}
lim
ϵ
→
0
‖
μ
ϵ
∗
∂
α
∂
y
α
f
−
f
‖
=
0
{\displaystyle \lim _{\epsilon \to 0}\|\mu _{\epsilon }*{\frac {\partial ^{\alpha }}{\partial y^{\alpha }}}f-f\|=0}
W
m
,
p
(
U
)
{\displaystyle W^{m,p}(U)}
Let
Ω
⊆
R
d
{\displaystyle \Omega \subseteq \mathbb {R} ^{d}}
v
∈
W
m
,
p
(
Ω
)
{\displaystyle v\in W^{m,p}(\Omega )}
C
∞
(
Ω
)
∩
W
m
,
p
(
Ω
)
{\displaystyle C^{\infty }(\Omega )\cap W^{m,p}(\Omega )}
Proof :
Let's choose
U
i
:=
{
x
∈
Ω
:
dist
(
∂
Ω
,
x
)
>
1
i
∧
‖
x
‖
<
i
}
{\displaystyle U_{i}:=\{x\in \Omega :{\text{dist}}(\partial \Omega ,x)>{\frac {1}{i}}\wedge \|x\|<i\}}
and
V
i
=
{
U
3
i
=
0
U
i
+
3
∖
U
i
+
1
¯
i
>
0
{\displaystyle V_{i}={\begin{cases}U_{3}&i=0\\U_{i+3}\setminus {\overline {U_{i+1}}}&i>0\end{cases}}}
One sees that the
V
i
{\displaystyle V_{i}}
Ω
{\displaystyle \Omega }
(
η
~
i
)
i
∈
N
{\displaystyle ({\tilde {\eta }}_{i})_{i\in \mathbb {N} }}
∀
i
∈
N
:
∀
x
∈
Ω
:
0
≤
η
~
i
(
x
)
≤
1
{\displaystyle \forall i\in \mathbb {N} :\forall x\in \Omega :0\leq {\tilde {\eta }}_{i}(x)\leq 1}
∀
x
∈
Ω
:
∃
only finitely many
i
∈
N
:
η
~
i
(
x
)
≠
0
{\displaystyle \forall x\in \Omega :\exists {\text{ only finitely many }}i\in \mathbb {N} :{\tilde {\eta }}_{i}(x)\neq 0}
∀
i
∈
N
:
∃
j
∈
N
:
supp
η
~
i
⊆
V
j
{\displaystyle \forall i\in \mathbb {N} :\exists j\in \mathbb {N} :{\text{supp }}{\tilde {\eta }}_{i}\subseteq V_{j}}
∀
x
∈
Ω
:
∑
i
=
0
∞
η
~
i
(
x
)
=
1
{\displaystyle \forall x\in \Omega :\sum _{i=0}^{\infty }{\tilde {\eta }}_{i}(x)=1}
By defining
H
i
:=
{
η
~
j
∈
{
η
~
m
}
m
∈
N
:
supp
η
~
j
⊆
V
i
}
{\displaystyle \mathrm {H} _{i}:=\{{\tilde {\eta }}_{j}\in \{{\tilde {\eta }}_{m}\}_{m\in \mathbb {N} }:{\text{supp }}{\tilde {\eta }}_{j}\subseteq V_{i}\}}
η
i
(
x
)
:=
∑
η
∈
H
i
η
(
x
)
{\displaystyle \eta _{i}(x):=\sum _{\eta \in \mathrm {H} _{i}}\eta (x)}
∀
i
∈
N
:
∀
x
∈
Ω
:
0
≤
η
i
(
x
)
≤
1
{\displaystyle \forall i\in \mathbb {N} :\forall x\in \Omega :0\leq \eta _{i}(x)\leq 1}
∀
x
∈
Ω
:
∃
only finitely many
i
∈
N
:
η
i
(
x
)
≠
0
{\displaystyle \forall x\in \Omega :\exists {\text{ only finitely many }}i\in \mathbb {N} :\eta _{i}(x)\neq 0}
∀
i
∈
N
:
supp
η
i
⊆
V
i
{\displaystyle \forall i\in \mathbb {N} :{\text{supp }}\eta _{i}\subseteq V_{i}}
∀
x
∈
Ω
:
∑
i
=
0
∞
η
~
i
(
x
)
=
1
{\displaystyle \forall x\in \Omega :\sum _{i=0}^{\infty }{\tilde {\eta }}_{i}(x)=1}
where the properties are the same as before except the third property, which changed.
Let
|
α
|
=
1
{\displaystyle |\alpha |=1}
φ
{\displaystyle \varphi }
(
v
j
)
j
∈
N
{\displaystyle (v_{j})_{j\in \mathbb {N} }}
v
{\displaystyle v}
L
p
(
Ω
)
{\displaystyle L^{p}(\Omega )}
∫
Ω
η
i
(
x
)
v
j
(
x
)
∂
α
∂
x
α
φ
(
x
)
d
x
=
−
∫
Ω
(
∂
α
∂
x
α
η
i
(
x
)
v
j
(
x
)
+
η
i
(
x
)
∂
α
∂
x
α
v
j
(
x
)
)
φ
(
x
)
d
x
{\displaystyle \int _{\Omega }\eta _{i}(x)v_{j}(x){\frac {\partial ^{\alpha }}{\partial x^{\alpha }}}\varphi (x)dx=-\int _{\Omega }\left({\frac {\partial ^{\alpha }}{\partial x^{\alpha }}}\eta _{i}(x)v_{j}(x)+\eta _{i}(x){\frac {\partial ^{\alpha }}{\partial x^{\alpha }}}v_{j}(x)\right)\varphi (x)dx}
reveals that, by taking the limit
j
→
∞
{\displaystyle j\to \infty }
v
∈
W
m
,
p
(
Ω
)
{\displaystyle v\in W^{m,p}(\Omega )}
η
i
v
∈
W
m
,
p
(
Ω
)
{\displaystyle \eta _{i}v\in W^{m,p}(\Omega )}
η
i
(
x
)
∂
α
∂
x
α
v
j
(
x
)
{\displaystyle \eta _{i}(x){\frac {\partial ^{\alpha }}{\partial x^{\alpha }}}v_{j}(x)}
L
p
(
Ω
)
{\displaystyle L^{p}(\Omega )}
φ
k
{\displaystyle \varphi _{k}}
Let's choose now
W
i
=
{
U
i
+
4
∖
U
i
¯
i
≥
1
U
4
i
=
0
{\displaystyle W_{i}={\begin{cases}U_{i+4}\setminus {\overline {U_{i}}}&i\geq 1\\U_{4}&i=0\end{cases}}}
We may choose now an arbitrary
δ
>
0
{\displaystyle \delta >0}
ϵ
i
{\displaystyle \epsilon _{i}}
‖
η
ϵ
i
∗
(
η
i
v
)
−
η
i
v
‖
W
m
,
p
(
Ω
)
<
δ
⋅
2
−
(
j
+
1
)
{\displaystyle \|\eta _{\epsilon _{i}}*(\eta _{i}v)-\eta _{i}v\|_{W^{m,p}(\Omega )}<\delta \cdot 2^{-(j+1)}}
supp
(
η
ϵ
i
∗
(
η
i
v
)
)
⊂
W
i
{\displaystyle {\text{supp }}(\eta _{\epsilon _{i}}*(\eta _{i}v))\subset W_{i}}
Let's now define
w
(
x
)
:=
∑
i
=
0
∞
η
ϵ
i
∗
(
η
i
v
)
(
x
)
{\displaystyle w(x):=\sum _{i=0}^{\infty }\eta _{\epsilon _{i}}*(\eta _{i}v)(x)}
This function is infinitely often differentiable, since by construction there are only finitely many elements of the sum which do not vanish on each
W
i
{\displaystyle W_{i}}
‖
w
−
v
‖
W
m
,
p
(
Ω
)
=
‖
∑
i
=
0
∞
η
ϵ
i
∗
(
η
i
v
)
−
∑
i
=
0
∞
(
η
i
v
)
‖
W
m
,
p
(
Ω
)
≤
∑
i
=
0
∞
‖
η
ϵ
i
∗
(
η
i
v
)
−
η
i
v
‖
W
m
,
p
(
Ω
)
<
δ
∑
i
=
0
∞
2
−
(
j
+
1
)
=
δ
{\displaystyle \|w-v\|_{W^{m,p}(\Omega )}=\left\|\sum _{i=0}^{\infty }\eta _{\epsilon _{i}}*(\eta _{i}v)-\sum _{i=0}^{\infty }(\eta _{i}v)\right\|_{W^{m,p}(\Omega )}\leq \sum _{i=0}^{\infty }\|\eta _{\epsilon _{i}}*(\eta _{i}v)-\eta _{i}v\|_{W^{m,p}(\Omega )}<\delta \sum _{i=0}^{\infty }2^{-(j+1)}=\delta }
Since
δ
{\displaystyle \delta }
Let
Ω
{\displaystyle \Omega }
∂
Ω
{\displaystyle \partial \Omega }
x
∈
∂
Ω
{\displaystyle x\in \partial \Omega }
U
x
{\displaystyle {\mathcal {U}}_{x}}
Ω
∩
U
x
=
{
(
x
1
,
…
,
x
d
)
∈
R
d
:
x
i
<
f
(
x
1
,
…
,
x
i
−
1
,
x
i
+
1
,
…
,
x
d
−
1
)
}
{\displaystyle \Omega \cap {\mathcal {U}}_{x}=\{(x_{1},\ldots ,x_{d})\in \mathbb {R} ^{d}:x_{i}<f(x_{1},\ldots ,x_{i-1},x_{i+1},\ldots ,x_{d-1})\}}
for a continuous function
f
{\displaystyle f}
W
m
,
p
(
Ω
)
{\displaystyle W^{m,p}(\Omega )}
C
∞
(
Ω
¯
)
{\displaystyle C^{\infty }({\overline {\Omega }})}
W
m
,
p
(
Ω
)
{\displaystyle W^{m,p}(\Omega )}
Proof :
to follow






































































































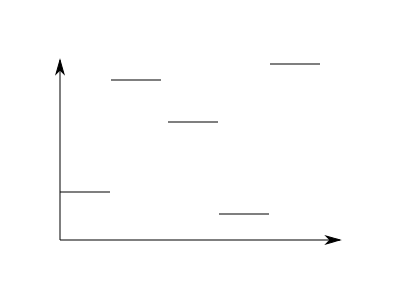







































































































































![{\displaystyle p,q\in [1,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/68ee142bd759e6d2fa264a449ed5d40d60edede7)




















































































































![{\displaystyle \lambda \in [0,1]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/010c0ee88963a09590dd07393d288edd83786b91)







































![{\displaystyle {\begin{aligned}\partial _{\alpha }(fg)&=\sum _{e_{k}\leq \varsigma \leq \alpha }{\binom {\alpha -e_{k}}{\varsigma -e_{k}}}\partial _{\varsigma }f\cdot \partial _{\alpha -\varsigma }g+\sum _{\varsigma \leq \alpha -e_{k}}{\binom {\alpha -e_{k}}{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -\varsigma }g\\&={\binom {\alpha -e_{k}}{0}}f\partial _{\alpha }g+\sum _{e_{k}\leq \varsigma \leq \alpha -e_{k}}\left[{\binom {\alpha -e_{k}}{\varsigma -e_{k}}}+{\binom {\alpha -e_{k}}{\varsigma }}\right]\partial _{\varsigma }f\partial _{\alpha -\varsigma }g+{\binom {\alpha -e_{k}}{\alpha -e_{k}}}f\partial _{\alpha }g\\&=\sum _{\varsigma \leq \alpha }{\binom {\alpha }{\varsigma }}\partial _{\varsigma }f\partial _{\alpha -\varsigma }\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/34162777bda40c4b8dd81c7607faf4502e630358)























































































![{\displaystyle [0,1]^{d}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e13ae4917276744b214714a20b3cb8ee305e309d)


























































































































































![{\displaystyle {\begin{aligned}(\partial _{t}-\Delta _{x})T_{E(\cdot -(t,x))}(\varphi )&=\int _{(t,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)\\&=\lim _{\epsilon \downarrow 0}\int _{(t,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)(1-\chi _{[t,t+\epsilon ]}(s))d(s,y)\\&=\lim _{\epsilon \downarrow 0}\int _{(t+\epsilon ,t+T)\times B_{R}(x)}(-\partial _{t}-\Delta _{x})\varphi (s,y)E(s-t,y-x)d(s,y)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/e913301ee6126b390a02d6c41e7ad31a5f5c6014)
![{\displaystyle \chi _{[t,t+\epsilon ]}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/8800dd57105ad4550f2b238bbc5085837486da39)
![{\displaystyle [t,t+\epsilon ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cf6deeb954f11533df953e306b788cd9874d77a0)




































































































































































































































































































































































![{\displaystyle {\begin{aligned}\int _{[-R,R]^{d}}\partial _{x_{k}}\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx&=\int _{[-R,R]^{d-1}}\int _{-R}^{R}\partial _{x_{k}}\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}dx_{k}d(x_{1},\ldots ,x_{k-1},x_{k+1},\ldots ,x_{d})\\&=\int _{[-R,R]^{d-1}}\left(\left(\partial _{\alpha }\phi (x)e^{-2\pi ix\cdot y}\right){\big |}_{x_{k}=-R}^{x_{k}=R}-\int _{-R}^{R}\partial _{\alpha }\phi (x)(-2\pi iy_{k})e^{-2\pi ix\cdot y}dx_{k}\right)d(x_{1},\ldots ,x_{k-1},x_{k+1},\ldots ,x_{d})\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/aa188b1fa41913feed107e4293e93ac4ce43d051)






























































































































![{\displaystyle p\in [1,\infty ]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/97b602ff0617b1b60e63d22200cad684dc5b26a0)























































































