This section shows how to form the equation describing the position of a mass on a spring.
For a simple oscillator consisting of a mass m attached to one end of a spring with a spring constant s , the restoring force, f , can be expressed by the equation
f
=
−
s
x
{\displaystyle f=-sx\,}
where x is the displacement of the mass from its rest position. Substituting the expression for f into the linear momentum equation,
f
=
m
a
=
m
d
2
x
d
t
2
{\displaystyle f=ma=m{d^{2}x \over dt^{2}}\,}
where a is the acceleration of the mass, we can get
m
d
2
x
d
t
2
=
−
s
x
{\displaystyle m{\frac {d^{2}x}{dt^{2}}}=-sx}
or,
d
2
x
d
t
2
+
s
m
x
=
0
{\displaystyle {\frac {d^{2}x}{dt^{2}}}+{\frac {s}{m}}x=0}
Note that the frequency of oscillation
ω
0
{\displaystyle \omega _{0}}
ω
0
2
=
s
m
{\displaystyle \omega _{0}^{2}={s \over m}\,}
To solve the equation, we can assume
x
(
t
)
=
A
e
λ
t
{\displaystyle x(t)=Ae^{\lambda t}\,}
The force equation then becomes
(
λ
2
+
ω
0
2
)
A
e
λ
t
=
0
,
{\displaystyle (\lambda ^{2}+\omega _{0}^{2})Ae^{\lambda t}=0,}
Giving the equation
λ
2
+
ω
0
2
=
0
,
{\displaystyle \lambda ^{2}+\omega _{0}^{2}=0,}
Solving for
λ
{\displaystyle \lambda }
λ
=
±
j
ω
0
{\displaystyle \lambda =\pm j\omega _{0}\,}
This gives the equation of x to be
x
=
C
1
e
j
ω
0
t
+
C
2
e
−
j
ω
0
t
{\displaystyle x=C_{1}e^{j\omega _{0}t}+C_{2}e^{-j\omega _{0}t}\,}
Note that
j
=
(
−
1
)
1
/
2
{\displaystyle j=(-1)^{1/2}\,}
and that C1 and C2 are constants given by the initial conditions of the system
If the position of the mass at t = 0 is denoted as x0 , then
C
1
+
C
2
=
x
0
{\displaystyle C_{1}+C_{2}=x_{0}\,}
and if the velocity of the mass at t = 0 is denoted as u0 , then
−
j
(
u
0
/
ω
0
)
=
C
1
−
C
2
{\displaystyle -j(u_{0}/\omega _{0})=C_{1}-C_{2}\,}
Solving the two boundary condition equations gives
C
1
=
1
2
(
x
0
−
j
(
u
0
/
ω
0
)
)
{\displaystyle C_{1}={\frac {1}{2}}(x_{0}-j(u_{0}/\omega _{0}))}
C
2
=
1
2
(
x
0
+
j
(
u
0
/
ω
0
)
)
{\displaystyle C_{2}={\frac {1}{2}}(x_{0}+j(u_{0}/\omega _{0}))}
The position is then given by
x
(
t
)
=
x
0
c
o
s
(
ω
0
t
)
+
(
u
0
/
ω
0
)
s
i
n
(
ω
0
t
)
{\displaystyle x(t)=x_{0}cos(\omega _{0}t)+(u_{0}/\omega _{0})sin(\omega _{0}t)\,}
This equation can also be found by assuming that x is of the form
x
(
t
)
=
A
1
c
o
s
(
ω
0
t
)
+
A
2
s
i
n
(
ω
0
t
)
{\displaystyle x(t)=A_{1}cos(\omega _{0}t)+A_{2}sin(\omega _{0}t)\,}
And by applying the same initial conditions,
A
1
=
x
0
{\displaystyle A_{1}=x_{0}\,}
A
2
=
u
0
ω
0
{\displaystyle A_{2}={\frac {u_{0}}{\omega _{0}}}\,}
This gives rise to the same position equation
x
(
t
)
=
x
0
c
o
s
(
ω
0
t
)
+
(
u
0
/
ω
0
)
s
i
n
(
ω
0
t
)
{\displaystyle x(t)=x_{0}cos(\omega _{0}t)+(u_{0}/\omega _{0})sin(\omega _{0}t)\,}
Back to Main page
If A1 and A2 are of the form
A
1
=
A
c
o
s
(
ϕ
)
{\displaystyle A_{1}=Acos(\phi )\,}
A
2
=
A
s
i
n
(
ϕ
)
{\displaystyle A_{2}=Asin(\phi )\,}
Then the position equation can be written
x
(
t
)
=
A
c
o
s
(
ω
0
t
−
ϕ
)
{\displaystyle x(t)=Acos(\omega _{0}t-\phi )\,}
By applying the initial conditions (x(0)=x0 , u(0)=u0 ) it is found that
x
0
=
A
c
o
s
(
ϕ
)
{\displaystyle x_{0}=Acos(\phi )\,}
u
0
ω
0
=
A
s
i
n
(
ϕ
)
{\displaystyle {\frac {u_{0}}{\omega _{0}}}=Asin(\phi )\,}
If these two equations are squared and summed, then it is found that
A
=
x
0
2
+
(
u
0
ω
0
)
2
{\displaystyle A={\sqrt {x_{0}^{2}+({\frac {u_{0}}{\omega _{0}}})^{2}}}\,}
And if the difference of the same two equations is found, the result is that
ϕ
=
t
a
n
−
1
(
u
0
x
0
ω
0
)
{\displaystyle \phi =tan^{-1}({\frac {u_{0}}{x_{0}\omega _{0}}})\,}
The position equation can also be written as the Real part of the imaginary position equation
R
e
[
x
(
t
)
]
=
x
(
t
)
=
A
c
o
s
(
ω
0
t
−
ϕ
)
{\displaystyle \mathbf {Re} [x(t)]=x(t)=Acos(\omega _{0}t-\phi )\,}
Due to euler's rule (ejφ = cosφ + jsinφ), x (t) is of the form
x
(
t
)
=
A
e
j
(
ω
0
t
−
ϕ
)
{\displaystyle x(t)=Ae^{j(\omega _{0}t-\phi )}\,}
Example 1.1
GIVEN: Two springs of stiffness,
s
{\displaystyle s}
M
{\displaystyle M}
FIND: The natural frequencies of the systems sketched below
Simple Oscillator-1.2.1.a
s
T
O
T
A
L
=
s
+
s
(springs are in parallel)
{\displaystyle s_{TOTAL}=s+s{\text{ (springs are in parallel)}}}
ω
0
=
s
T
O
T
A
L
m
T
O
T
A
L
=
2
s
M
{\displaystyle \omega _{0}={\sqrt {\frac {s_{TOTAL}}{m_{TOTAL}}}}={\sqrt {\frac {2s}{M}}}}
f
0
=
ω
0
2
π
=
1
2
π
2
s
M
{\displaystyle \mathbf {f_{0}} ={\frac {\omega _{0}}{2\pi }}=\mathbf {{\frac {1}{2\pi }}{\sqrt {\frac {2s}{M}}}} }
Simple Oscillator-1.2.1.b
ω
0
=
s
T
O
T
A
L
m
T
O
T
A
L
=
s
2
M
{\displaystyle \omega _{0}={\sqrt {\frac {s_{TOTAL}}{m_{TOTAL}}}}={\sqrt {\frac {s}{2M}}}}
f
0
=
ω
0
2
π
=
1
2
π
s
2
M
{\displaystyle \mathbf {f_{0}} ={\frac {\omega _{0}}{2\pi }}=\mathbf {{\frac {1}{2\pi }}{\sqrt {\frac {s}{2M}}}} }
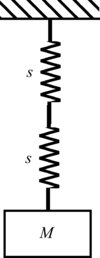
Simple Oscillator-1.2.1.c
1.
s
(
x
1
−
x
2
)
=
s
x
2
{\displaystyle \mathbf {1.} {\text{ }}s(x_{1}-x_{2})=sx_{2}}
2.
−
s
(
x
1
−
x
2
)
=
m
d
2
x
d
t
2
{\displaystyle \mathbf {2.} {\text{ }}-s(x_{1}-x_{2})=m{\frac {d^{2}x}{dt^{2}}}}
d
2
x
1
d
t
2
+
s
2
m
x
1
=
0
{\displaystyle {\frac {d^{2}x_{1}}{dt^{2}}}+{\frac {s}{2m}}x_{1}=0}
ω
0
=
s
2
m
{\displaystyle \omega _{0}={\sqrt {\frac {s}{2m}}}}
f
0
=
1
2
π
s
2
m
{\displaystyle \mathbf {f_{0}={\frac {1}{2\pi }}{\sqrt {\frac {s}{2m}}}} }
Simple Oscillator-1.2.1.d
ω
0
=
2
s
m
{\displaystyle \omega _{0}={\sqrt {\frac {2s}{m}}}}
f
0
=
1
2
π
2
s
m
{\displaystyle \mathbf {f_{0}={\frac {1}{2\pi }}{\sqrt {\frac {2s}{m}}}} }
Back to Main page





























![{\displaystyle \mathbf {Re} [x(t)]=x(t)=Acos(\omega _{0}t-\phi )\,}](https://wikimedia.org/api/rest_v1/media/math/render/svg/15c427c7cb9287e21e410751fb6ab22afe553eb7)