Visual physics and mathematics/Fluid dynamics

The Venturi Effect

The gradation from white to blue represents an increase in pressure. The pressure is lowest where the pipe is narrowest. This isn't very intuitive, especially if we think of a crowd passing through a narrow door. But we explain it by reasoning about the forces experienced by an element of the fluid. To pass through the constriction, it is first accelerated, as if pushed from behind. The pressure behind it is therefore higher than the pressure in front of it. After passing through the constriction, it is braked. The pressure in front of it is therefore higher than the pressure behind it.

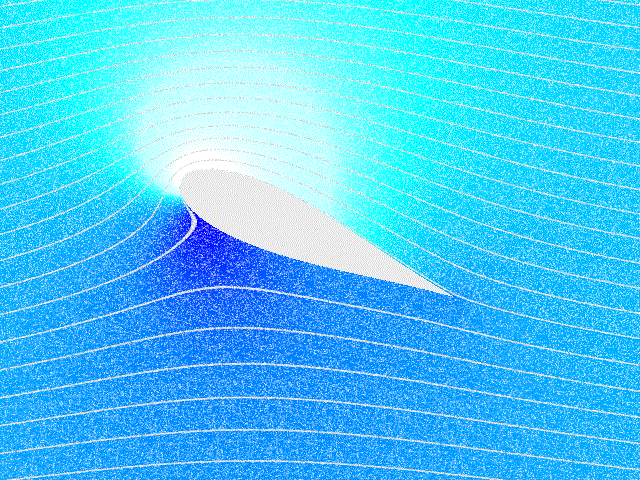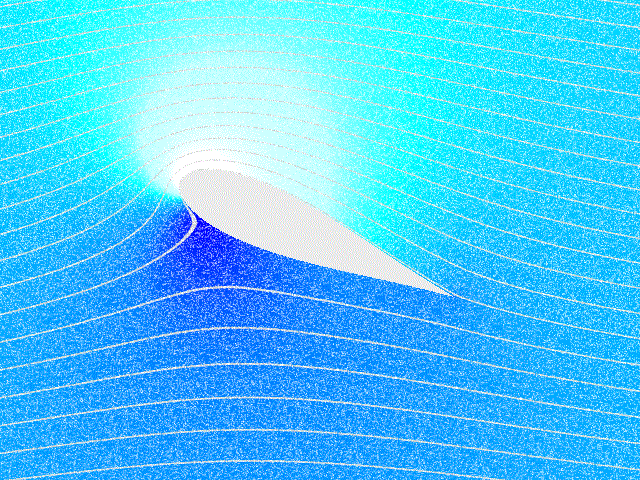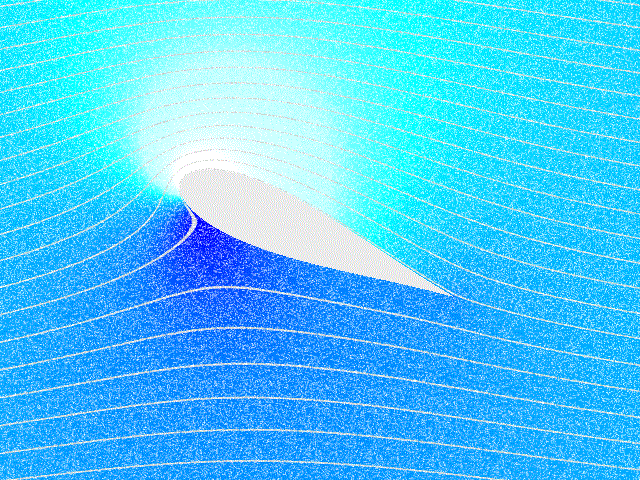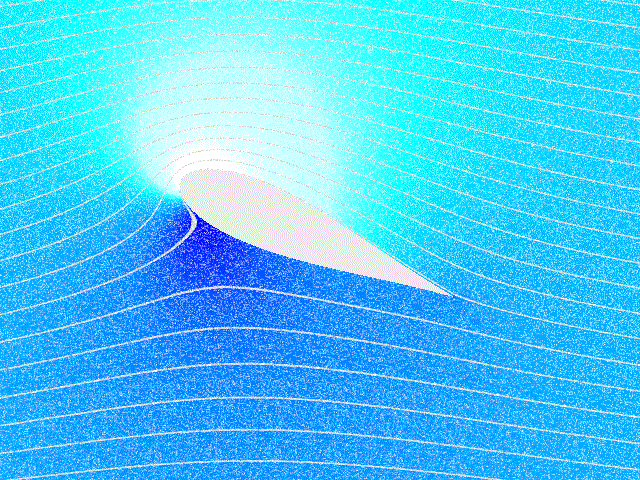
Why do planes have wings?

The angle of attack (the inclination of the wing relative to the horizontal, here greatly exaggerated compared to a realistic situation) requires the air to flow more quickly above the wing than below. As with the Venturi effect, the acceleration of the air imposes a reduction in pressure. The pressure below the wing is therefore higher than the pressure above it. This is why planes are carried by their wings.

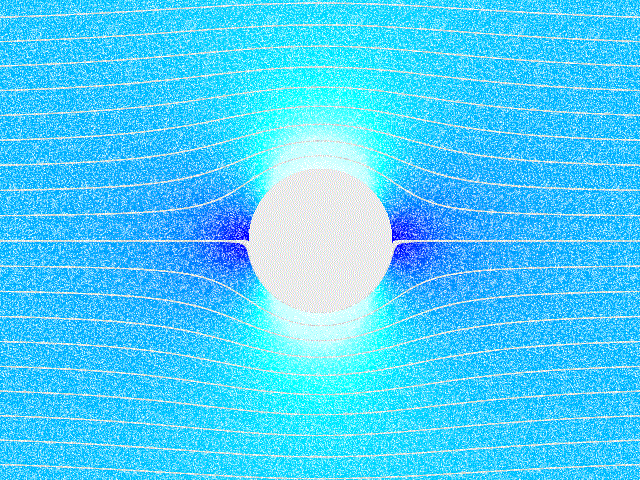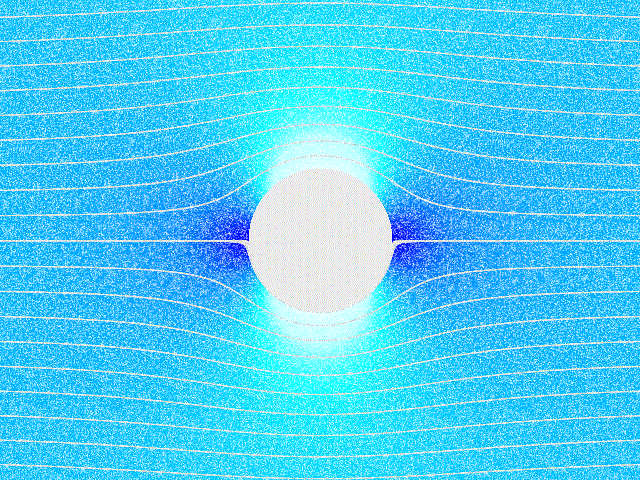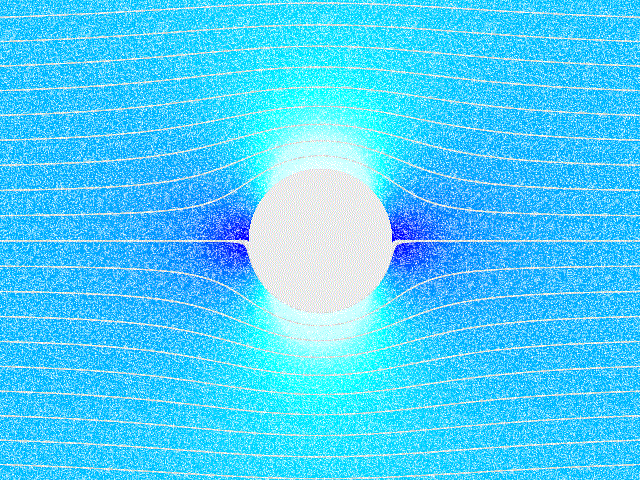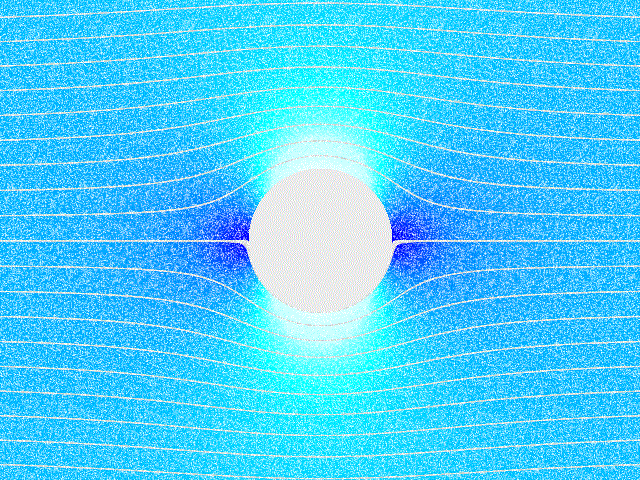
Perfect flow around a cylinder

Just in front of the cylinder, the fluid is almost still. So it was slowed down. The pressure on the front of the cylinder is therefore higher than the pressure upstream, as expected. Just behind the cylinder, the fluid is also almost still. It is therefore accelerated to join the flow downstream. The pressure on the rear of the cylinder is therefore higher than the pressure downstream, as one would not expect. If the fluid is without viscosity, the pressures on the rear and on the front of the cylinder compensate each other exactly and the cylinder is therefore not dragged by the flow, as if one could put one's legs in a torrent without being dragged towards downstream, or if a projectile could pass through the air without being slowed down. To explain the drag force, viscosity (the force of fluid friction) and turbulence must be taken into account.

A Karman vortex street

Half a million hard disks bounce off each other and an obstacle to their flow.

The Magnus effect

How to surprise an opponent? By sending him a spinning ball.

Demixing

A million hard disks bounce off each other. There are no forces of attraction, only repulsion. The two colors represent molecules of two species. Between two molecules of different species, the rebound distance is greater than between two molecules of the same species. This is enough to cause molecules of the same species to come nearer, the two fluids to separate and a line tension to appear between the two phases. The line tension in this two-dimensional fluid is the equivalent of the surface tension (the tension that makes drops, bubbles, and flat liquid surfaces) in three-dimensional fluids. Intermolecular forces of attraction are therefore not necessary to cause surface tension to appear.

Pressure and altitude

A 2D gas of hard disks bouncing on each other, initially at rest, collapses under the effect of gravity and reaches an equilibrium situation where the pressure decreases with altitude.

Archimedes' thrust

Hard disks subject to gravity bounce on each other. The large yellow disk experiences greater pressure at its base than at its top. This is why it is carried by the fluid like a hot air balloon.

Archimedes' thrust is the sum of the pressure forces exerted on a solid immersed in a fluid. In a fluid in equilibrium under the effect of gravity, it is directed upwards. If the solid is also in equilibrium, the Archimedes' thrust is exactly equal to its weight, which is also the weight of the volume of fluid occupied by the solid.