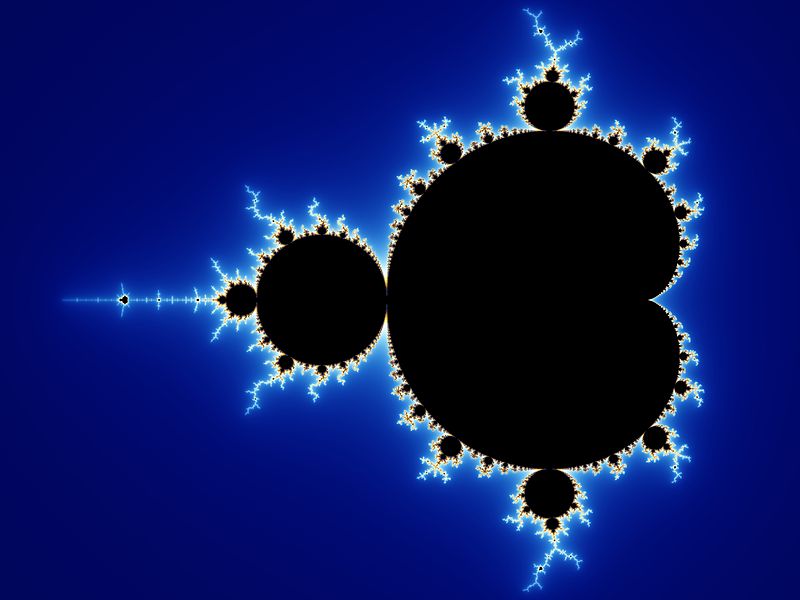
Dynamical Systems/The Mandelbrot set

Definition (Mandelbrot set):

For $c\in \mathbb {C}$, define $f_{c}(z):=z^{2}+c$. The Mandelbrot set is the subset $M$ of the complex plane $\mathbb {C}$ of points $c$ which are such that the set

$\{f_{c}(0),f_{c}(f_{c}(0)),f_{c}(f_{c}(f_{c}(0))),\ldots \}\subset \mathbb {C}$

is bounded.

Here is a picture of the Mandelbrot set:

This set motivates the following more precise definition:

Definition (Mandelbrot-type set):

A Mandelbrot-type set we define to be as the set of points $c$ of the complex plane such that

$\{f(c,0),f(c,f(c,0)),f(c,f(c,f(c,0))),\ldots \}$

is a bounded subset of $\mathbb {C}$, where $f:\mathbb {C} \times \mathbb {C} \to \mathbb {C}$ is a function holomorphic in two variables, and such that

$\{f(c,0),f(c,f(c,0)),f(c,f(c,f(c,0))),\ldots \}$

will be unbounded once the modulus of any of its elements passes a certain threshold $C>0$.

Proposition (mandelbrot-type set is bounded):

Proposition (mandelbrot-type set is closed):

Proposition (mandelbrot-type set is compact):

Proposition (mandelbrot-type set is simply connected):

Let $M$ be a Mandelbrot-type set. Then $M$ is simply connected.

Proof: Suppose not. Then the complement of $M$ would have a bounded component $A\subset \mathbb {C}$. Take any point $c_{0}\in {\overset {\circ }{A}}$. Then define inductively $\phi _{n}(c):=f(c,\phi _{n-1}(c))$, with $\phi _{0}(c)=0$; this is the iterated function, and it is holomorphic. By assumption, there exists $n$ such that $|\phi _{n}(c_{0})|>C$. But by the maximum principle, $|\phi _{n}(c_{1})|>C$ for some $c_{1}\in \partial A$, so that $c_{1}\in \partial A\cap M^{c}$. But $M$ was closed. $\Box$