Transportation Economics/Negative externalities
An externality is a cost or benefit incurred by a party's decision or purchase on another, who neither consents, nor is considered in the decision. One example of a negative externality we will consider is pollution
Introduction
[edit | edit source]There has been a long-standing interest in the issue of the social or external costs of transportation (see for instance: Keeler et al. 1975 [1], Fuller et al. 1983 [2], Mackenzie et al. 1992[3], INRETS 1993 [4], Miller and Moffet 1993 [5], IWW/INFRAS 1995 [6], IBI 1995 [7]). The passions surrounding social costs and transportation, in particular those related to the environment, have evoked far more shadow than light. At the center of this debate is the question of whether various modes of transportation are implicitly subsidized because they generate externalities, and to what extent this biases investment and usage decisions. On the one hand, exaggerations of environmental damages as well as environmental standards formulated without consideration of costs and benefits are used to stop new infrastructure. On the other hand, the real social costs are typically ignored in financing projects or charging for their use.
Associated with the interest in social and external cost has been a continual definition and re-definition of externalities in transportation systems. Verhoef (1994)[8] states “An external effect exists when an actor’s (the receptor’s) utility (or profit) function contains a real variable whose actual value depends on the behavior of another actor (the supplier) who does not take these effects of his behavior into account in this decision making process.” This definition eliminates pecuniary externalities (for instance, an increase in consumer surplus), and does not include criminal activities or altruism as producers of external benefits or costs. Rothengatter (1994) [9] presents a similar definition: “an externality is a relevant cost or benefit that individuals fail to consider when making rational decisions.” Verhoef (1994) divides external cost into social, ecological, and intra-sectoral categories, which are caused by vehicles (in-motion or non-in-motion) and infrastructure. To the externalities we consider (noise, congestion, crashes, pollution), he adds the use of space (e.g. parking) and the use of matter and energy (e.g. the production and disposal of vehicles and facilities). Button (1994) [10] classes externalities spatially, considering them to be local (noise, lead, pollution), transboundary (acid rain, oil spills), and global (greenhouse gases, ozone depletion). Gwilliam and Geerlings (1994) [11] combines Verhoef’s and Button’s schemes, looking at a Global, Local, Quality of Life (Social), and Resource Utilization (air, land, water, space, materials) classification.
Rothengatter (1994) views externalities as occurring at three levels: individual, partial market, total market, and argues that only the total market level is relevant for checking the need of public interventions. This excludes pecuniary effects (consumer and producer surplus), activities concerning risk management, activities concerning transaction costs. Externalities are thus public goods and effects that cannot be internalized by private arrangements.
Rietveld (1994) [12] identifies temporary effects and non-temporary effects occurring at the demand side and supply side. Maggi (1994) divides the world by mode (road and rail) and medium (air, water, land) and considers noise, crashes, and community and ecosystem severance. Though not mentioned among the effects above, to all of this might be added the heat output of transportation. This leads to the “urban heat island” effect -- with its own inestimable damage rate and difficulty of prevention.
Coase (1992) [13] argues that the problem is that of actions of firms (and individuals) which have harmful effects on others. His theorem is restated from Stigler (1966) [14] as “... under perfect competition, private and social costs will be equal.” This analysis extends and controverts the argument of Pigou (1920) [15], who argued that the creator of the externality should pay a tax or be liable. Coase suggests the problem is lack of property rights, and notes that the externality is caused by both parties, the polluter and the receiver of pollution. In this reciprocal relationship, there would be no noise pollution externality if no-one was around to hear. This theory echoes the Zen question “If a tree falls in the woods and no-one is around to hear, does it make a sound?”. Moreover, the allocation of property rights to either the polluter or pollutee results in a socially optimal level of production, because in theory the individuals or firms could merge and the external cost would become internal. However, this analysis assumes zero transaction costs. If the transaction costs exceed the gains from a rearrangement of activities to maximize production value, then the switch in behavior won’t be made.
There are several means for internalizing these external costs. Pigou identifies the imposition of taxes and transfers, Coase suggests assigning property rights, while our government most frequently uses regulation. To some extent all have been tried in various places and times. In dealing with air pollution, transferable pollution rights have been created for some pollutants. Fuel taxes are used in some countries to deter the amount of travel, with an added rationale being compensation for the air pollution created by cars. The US government establishes pollution and noise standards for vehicles, and requires noise walls be installed along highways in some areas. Therefore, a consensus definition might be, “Externalities are costs or benefits generated by a system (in this case transportation, including infrastructure and vehicle/carrier operations,) and borne in part or in whole by parties outside the system.”
Definitions
[edit | edit source]An externality is that situation in which the actions of one agent imposes a benefit or cost on another economic agent who is not party to a transaction.
Externalities are the difference between what parties to a transaction pay and what society pays
- A pecuniary externality, increases the price of a resource and therefore involves only transfers,
- A technical externality exhibits a real resource effect. A technical externality can be an external benefit (positive) or an external disbenefit (negative).
Examples
[edit | edit source]Negative externalities (external disbenefits) are air pollution, water pollution, noise, congestion.
Positive externalities (external benefits) include examples such as bees from apiary pollinating fruit trees and orchards supplying bees with nectar for honey.
Cause
[edit | edit source]The source of externalities is the poorly defined property rights for an asset which is scarce. For example, no one owns the environment and yet everyone does. Since no one has property rights to it, no one will use it efficiently and price it. Without prices people treat it as a free good and do not cost it in their decision making. Overfishing can be explained in the same way.
We want that amount of the externality which is only worth what it costs. Efficiency requires that we set the price of any asset >0 so the externality is internalized. If the price is set equal to the marginal social damages, we will get a socially efficient amount of the good or bad. Economic agents will voluntarily abate if the price is non-zero.
The Coase Theorem states that in the absence of transaction costs, all allocations of property are equally efficient, because interested parties will bargain privately to correct any externality. As a corollary, the theorem also implies that in the presence of transaction costs, government may minimize inefficiency by allocating property initially to the party assigning it the greatest utility.
Pareto Optimality
[edit | edit source]A change that can make at least one individual better off, without making any other individual worse off is called a Pareto improvement: an allocation of resources is Pareto efficient when no further Pareto improvements can be made.
Thought Question
[edit | edit source]What is the Optimal Amount of Externality?
(Is it zero? Why or why not?)
Damages vs. Protection
[edit | edit source]

The tradeoff between benefits and costs is central to most economic analyses. Costs and benefits are both measurable and immeasurable, and a complete analysis must consider transaction and information costs as well as market costs. Individuals strive to maximize net benefits (benefits after considering costs), society might apply this to social costs as well. Reducing damages requires increasing protection (defense, abatement, or mitigation) to attenuate the damage. At some point, the cost of protection outweighs the benefit of reducing residual damages. This is illustrated in the figure. Whether this point is at zero damages (no damage is acceptable), zero protection (the damage is so insignificant as to be irrelevant), or somewhere in between is an empirical question. Total social costs are minimized where the marginal cost of additional damages equals the cost of additional protection. Whether the marginal costs of damage and of protection are fixed, rising or declining with output, and by how much will be another important empirical question.
The notion of damages and protection is compatible with the idea of supply and demand, as illustrated in the figure to the right. Here, the change in damages with output () is the demand curve (the marginal willingness to pay to avoid damage), and the change in protection (attenuation) with output is the supply curve (marginal cost) and represented as (). Again, the slopes of the curves are speculative:
In the next Figure, area A represents the consumer surplus, or the benefit which the community receives from production, and is maximized by producing at qo (marginal cost of protection or attenuation equals the marginal cost of defense). The shaded area B represents production costs, and is the amount of social cost at the optimal level of production. Area C is non-satisfied demand, and does not result in any social costs so long as production remains at .
Systems
[edit | edit source]
Central to the definition and valuation of externalities is the definition of the system in question. The intercity transportation system is open, dynamic, and constantly changing. Some of the more permanent elements include airports, intercity highways , and railroad tracks within the state. The system also includes the vehicles using those tracks (roads, rails, or airways) at any given time. Other components are less clear cut - are the roads which access the airports, freeways, or train stations part of the system? The energy to propel vehicles is part of the system, but is the extraction of resources from the ground (e.g. oil wells) part of the system? DeLuchi (1991) [17] analyzes them as part of his life-cycle analysis, but should we? Where in the energy production cycle does it enter the transportation system?
Any open system influences the world in many ways. Some influences are direct, some are indirect. The transportation system is no exception. Three examples may illustrate the point:
- Cars on roads create noise—this we consider a direct effect.
- Roads reduce the travel time between two places, which increases the amount of land development along the corridor—this is a less direct effect, not as immediate or obvious as the first. Other factors may intervene to cause or prevent this consequence.
- The new land development along the corridor results in increased demand for public schools and libraries—this is clearly an indirect effect of transportation.
As can be seen almost immediately, there is no end to the number or extent of indirect effects. While recognizing that the economy is dynamic and interlinked in an enormous number of ways, we also recognize that it is almost impossible to quantify anything other than proximate, first order, direct effects of the transportation system. If the degree to which “cause” (transportation) and “effect” (negative externality) are correlated is sufficiently high, then we consider the effect direct; the lower the probability of effect following from cause, the less direct is the effect. The question of degree of correlation is fundamentally empirical.
On the other hand, this raises some problems. Automobiles burn fuel that causes pollution directly. Electric powered high speed rail uses energy from fuel burned in a remote power plant. If the electricity is fully priced, including social costs, then there is no problem in excluding the power plant. But, if the social costs of burning fuel in a power plant are not properly priced, then to ignore these costs would be biased. This is the problem of the first best and second best. The idea of the first best solution suggests that we optimize the system under question as if all other sectors were optimal. The second best solution recognizes that other systems are also suboptimal. Clearly, other systems are suboptimal to some extent or another. However, if we make our system suboptimal in response, we lessen the pressure to change the other systems. In so doing we effectively condemn all other solutions to being second best.
Button (1994) develops a model relating ultimate economic causes to negative externalities and their consequences as summarized in the following graphic. Users and suppliers do not take full account of environmental impacts, leading to excessive use of transport. Button argues that policy tools are best aimed at economic causes, but in reality measures are aimed at any of four stages. Here we are considering the middle stage, physical causes and symptoms, and are ignoring feedback effects.
Another view has the “externalities” as inputs to the production of transportation, along with typical inputs as construction of transportation and the operation and maintenance of the system. There are multiple outputs, simplified to person trips and freight trips, although of course each person trip is in some respects a different commodity. This view comports with Becker’s (1965) [18] view that households use time in the production of commodities -- of which travel might be one.
Theory
[edit | edit source]
To establish optimal emission levels for pollution, congestion or any other externality consider the following framework. All emissions, damages, and costs are from one or more sources , subscripts are omitted for clarity.
where:
- is the Cost of Abatement function with
- and
and
where:
- is the Cost of Damages function due to emissions .
- is the actual emission from source.
- is the amount of emission at source in an uncontrolled state.
- is the amount of abatement at source.
Note if , and thus the actual emissions equal the maximum amount possible.
The solution to the problem is to minimize the sum of damage costs and abatement costs or
which indicates a constant marginal demand function.
The following is true:
This states that the optimal amount of any externality is established by minimizing the sum of damage and abatement costs so we end up with E* amount of aggregate pollution.
If a profit maximizing firm were faced with an abatement charge they would internalize the externality or abate until the marginal cost of abatement were equal to the price of pollution or the change.
Government Standards
[edit | edit source]If the government wanted to establish a 'standard' it would require knowledge of:
- level of marginal damages
- Marginal Cost function of polluters
It would therefore appear that there is an informational advantage to pricing.
The solution which has been illustrated above also applies with:
- spatially differentiated damages
- non-linear damage functions
- non-competitive market settings
Standards dominate charged because
- Uncertainty with respect to the marginal damage function.
- Uncertainty with respect to the marginal abatement costs.
Uncertainty with respect to marginal damage function
[edit | edit source]Now consider the situation where the MC of abatement has been underestimated so the true MC of abatement lies above the estimated MC of abatement function. Consider a standards scheme. Using the estimated MC of abatement the emission level is set at e instead of e*. Thus, the emission level is too low relative to the optimum. With the level of abatement too high, the damages reduced due to having this lower level of emissions is eAce* but at the cost of much higher abatement costs of eBCe*. The net social loss will be ABC.
Alternatively, suppose the authority set a sub-optimal emission standard of e because it is using the erroneous MD function. With emissions at e rather than e*, we again end up with a net social loss of ABC. Therefore, uncertainty with respect to the marginal damage function provides NO ADVANTAGE to either scheme; pricing or standards.
Uncertainty with respect to marginal abatement costs
[edit | edit source]Now consider the situation where the MC of abatement has been underestimated so the true MC of abatement lies above the estimated MC of abatement function. Consider a standards scheme. Using the estimated MC of abatement the emission level is set at e instead of e*. Thus, the emission level is too low relative to the optimum. With the level of abatement too high, the damages reduced due to having this lower level of emissions is eAce* but at the cost of much higher abatement costs of eBCe*. The net social loss will be ABC
Now consider a pricing scheme. The authority would set the emission charge at EC by setting the MD function equal to the MC of abatement function. This would result in a level of emission of e; thinking this is the correct amount. But with a true MC of abatement at MCT the level of emissions which the charge EC will generate will be e'.
e' > e* so we have too high a level of emissions. Pollution damages will increase by the amount e*CDe' but the abatement costs will be reduced (because of higher allowed emissions) by e*CEe'. Therefore, the net social loss will be CDE. Generally, there is no reason to expect CDE = ABC but it has been shown that
where:
- is the welfare loss from pricing
- is the welfare loss from standards
- is
- is the elasticity of the marginal damage function
- is the elasticity of the marginal cost of abatement function
The welfare loss from pricing and standards will be equal if 1. = in absolute value or 2. or
Standards will be preferred to charges when which occurs when
.
If charges are preferred while if standards are preferred.
Rationale
[edit | edit source]The rationale for this is:
- if the MD function is steep (e.g. with very toxic pollution) even a slight error in will generate large damages. With uncertainty about costs, the chances of such errors is greater with a charging scheme.
- if the MD function is flat, a charge will better approximate marginal damages. If the damage function is linear, the optimal result is independent of any knowledge about costs.
- if the MC is steep, an ambitious standard could result in excessive costs to abators. A charge places an upper limit on costs.
Therefore, the KEY in this is charges set an upper limit on costs while standards set an upper limit on discharges.
Prices
[edit | edit source]Externality prices can take three forms:
1. use to optimize social surplus
2. use to achieve a predetermined standard at least cost]
3. use to induce compliance to a particular standard
Perhaps the best know 'cure' for the congestion externality facing most major cities has been advocated by economists; road pricing. Standards are achieved in this instance by continuing to build roads.
Measurement
[edit | edit source]The cost of an externality is a function of two equations. The first relates the physical production of the externality to the amount of transportation output. The second computes the economic cost per unit of externality. The amount of an externality produced by transportation is the result of the technology of the transportation, as well as the amount of defense and abatement measures undertaken. There are several issues of general concern in the physical production of externalities. They are classified as: fungibility, geography, life cycle, technology, and point of view. Each are addressed in turn.
Fungibility
[edit | edit source]“Is the externality fungible?” In other words, does the externality which is physically produced by the system under question have to be eliminated or paid for, or can something substitute for it. For example, a car may produce X amount of Carbon Dioxide. If carbon dioxide were not fungible, then that X would need to be eliminated, or a tax assessed based on the damage that X causes. However, if it were fungible, then an equivalent amount X could be eliminated through some other means (for instance, by installing pollution control on a factory or by planting trees). The second option may be cheaper, and this may influence the economic effects of the pollution generated.
Geography
[edit | edit source]“Over what area are the externalities considered?” “Is a cost generated by a project in California which is borne by those outside California relevant?” This is particularly important in estimating environmental costs, many of which are global in nature. If we try to estimate damages (rather than the protection costs of defense, abatement, and mitigation), this becomes particularly slippery. However, if we can assume fungibility, and use the cost of mitigation techniques, the measurement problem becomes much simpler. Ideally, we would obtain estimates for both protection and damages in order to determine the tradeoffs.
Life Cycle
[edit | edit source]In some respects we would like to view the life-cycle of the transportation system. But it becomes more difficult to consider the life-cycle of every input to the transportation system. The stages which may be considered include: Pre-production, construction, utilization, refurbishing, destruction, and disposal. Ignoring the life-cycle of all inputs may create some difficulties. Electric power will produce pollution externalities at production in a power plant, before it enters the transportation system. Thus, modes using electric power (rail, electric cars), would be at an advantage using this decision rule over modes which burn fuel during the transport process (airplanes, gasoline powered cars, diesel trains). This is true, though to a lesser extent, with other inputs as well.
Technology
[edit | edit source]The technology involved in transportation is constantly changing. The automobile fleet on the ground in 2000 will have very different characteristic than that in the year 1900 regarding the number of externalities produced. Hopefully, cars will be safer, cleaner, and quieter. Similar progress will no doubt be made in aircraft and trains. While the analysis will initially assume current technology, sensitivity tests should consider the effect that an improved fleet will have on minimizing externality production.
Macro vs. Micro Analysis Scale
[edit | edit source]Estimates for externalities typically come in two forms macro and micro levels of analysis. Macroscopic analysis uses national (or global) estimates of costs as share of gross domestic product (GDP), such as Kanafani (1983), Quinet (1990), and Button (1994). The data for microscopic analysis is far more dispersed. It relies on numerous engineering and empirical cost-benefit and micro-economic studies. By and large, this study is a microscopic analysis, though, on occasion, the macroscopic numbers will be used as benchmarks for comparison and estimates of data where not otherwise available. This will be true for both the physical production of externalities as well as their economic costs through damages borne or protection/attenuation measures. Once cost estimates are produced, they can be expanded to estimate the state-wide social costs of transport as a share of state product (California GDP), which can be compared with other national estimates.
Issues
[edit | edit source]Two important issues of concern in measuring the economic cost of externalities are: the basis over which the output is measured and the consistency of the measurement . When estimating the full cost of externalities, the amount of externality is not simply the amount of traffic on the road multiplied by some externality rate. Rather, it must be measured as the difference between what is generated systemwide with and without the facility. For instance, a new freeway lane will have several effects: diverting existing traffic from current facilities, inducing new traffic on the new facility, and inducing new/different traffic on the old facility. The amount of this change must be accurately determined with a general equilibrium approach to estimate demand. In a general equilibrium approach, the travel time/cost used to estimate the amount of demand is equal to the travel time/cost resulting from that demand. Switching traffic from an older facility to a newer facility may in fact reduce the amount of negative externalities generated. For instance, the number of accidents or their severity may decline if the new facility is safer than the old. On the other hand, the induced traffic, while certainly a benefit in that it increases commerce, also imposes new additional costs, more accidents, pollution and noise. It is the net change which must be considered.
When addressing the costs of externalities, the estimates used across all externalities should be consistent. Cost estimates contain implicit assumptions, particularly concerning the value of time, life, and safety. Key questions can be asked of any study:
- Is the value of life and health used in estimating the cost of accidents the same as used in estimating the human effects of pollution?
- Is the value of time used consistent between congestion costs and accidents? With congestion, many are delayed a small time, crashes (ignoring congestion implications), a few are delayed a long time.
Cost-Function Estimation Methods
[edit | edit source]Many approaches have been undertaken to estimate the costs of externalities. The first class of approaches we call “Damage” based methods, the second can be called “Protection” based methods. The damage based methods begin with the presumption that there is an externality and it causes X amount of damage through lower property values, quality of life, and health levels.
The protection methods estimate the cost to protect against a certain amount of the externality through abatement, defense, or mitigation. One example of a defense measure is thicker windows in a house to reduce noise from the road. An abatement measure would have the highway authority construct noise walls to reduce noise or require better mufflers on vehicles. A mitigation measure may only be applicable for certain types of externalities; e.g. increased safety measures that reduce accidents on one facility also offset the increased number of accidents on another facility.
Rising marginal costs are expected of protection measures. The first quantity of externality abated /defended/mitigated is cheaper than the second and so on because the most cost-effective measures are undertaken first. This is not to say there are no economies of scale in mitigating externalities within a given mitigation technology. It merely suggests that between technologies, costs will probably rise. The mitigation approach can be applied if we consider the externality fungible. Air pollution from the road may cause as much damage as an equivalent amount of pollution from nearby factories. The most cost effective approach to eliminating the amount of pollution produced by the road may come from additional scrubbers on the factory. While it may be prohibitively expensive to eliminate 100% of roadway pollution from the roadway alone, it may be quite reasonable to eliminate the same amount of pollution from the system. Determining the most effective method of mitigating each system-wide externality requires understanding the nature of its fungibility.
Neither of these two approaches (Damages or Protection) will necessarily produce a single value for the cost of a facility. It is more likely that each approach will produce a number of different cost estimates based on how it is undertaken and what assumptions are made. This reinforces the need for sensitivity analyses and a well-defined “systems” approach. We divide the techniques of costing into three main categories: revealed preference, stated preference, and implied preference. Revealed preference is based on observed conditions and how individuals subject to the externality behave, stated preference comes from surveys of individuals in hypothetical situations, while implied preference looks at the cost which is implied based on legislative, executive, or judicial decisions.
Revealed Preference
[edit | edit source]The revealed preference approach attempts to determine the cost of an externality by determining how much damage reduces the price of a good.
Revealed preference can also be used to estimate the price people pay for various protection (defense/ abatement) measures and the effectiveness of those measures. For instance, insulation costs a certain amount of money and provides a certain amount of effectiveness in reducing noise. The extent to which individuals then purchase insulation or double-glazed windows may suggest how much they value quiet. However, individuals may be willing to spend some money (but less than the cost of insulation) if they could ensure quiet by some other means which they do not control - but which may be technically feasible.
Hedonic Models: The most widely used estimates of the cost of noise are derived from hedonic models. These assume that the price of a good (for instance a home) is composed of a number of factors: square footage, accessibility, lot area, age of home, pollution, noise, etc. Using a regression analysis, the parameters for each of these factors are estimated. From this, the decline in the value of housing with the increase in the amount of noise can be estimated. This has been done widely for estimating the social cost of road noise and airport noise on individual homes. In theory, the value of commercial real estate may be similarly influenced by noise. In our literature review thus far, no study of this sort has been found. Furthermore, although noise impacts public buildings, this method cannot be used as a measure since public buildings are not sold. Similarly, when determining some of the costs of noise, one could investigate how much individuals might be willing to pay for vehicles which are quieter. Like a home, a hedonic model of vehicle attributes could be estimated. A vehicle is a bundle of attributes (room, acceleration, MPG, smooth ride, quiet, quality of workmanship, accessories) which influence its price, also an attribute.
Unit/Cost Approach: A simple method, the “unit cost (Rate) approach” is used often for allocating costs in transit. This method assigns each cost element, somewhat arbitrarily, to a single output measure or cost center (for instance, Vehicle Miles Travel, Vehicle Hours Travel, Number of Vehicles, Number of Passengers) based on the highest statistical correlation of the cost with output.
Wage/Risk Study: A means for determining the economic cost of risk to life or health or general discomfort is by analyzing wage/salary differentials based on job characteristics, including risk as a factor.
Time Use Study: This approach measures the time used to reduce some risk by a certain amount. For instance, seatbelts reduce the risk of injury or using pedestrian overpass may reduce the risk of being hit by a car. The time saved has a value, which may inform estimates of risk aversion.
Years Lost plus Direct Cost: This method estimates the number of years lost to an accident due to death and years lost from non-fatal injuries. It also the monetary costs of non-life damages. However, it defines life in monetary terms. While it may have some humanistic advantages in that it does not place a dollar value on life, defining life through dollars and sense may have some practical value. Defining life through dollars and sense may help us assess whether an improvement, with a certain construction cost and life-saving potential, is economically worthwhile.
Comprehensive: This accident costing method extends the Years Lost plus Direct Cost method by placing a value on human life. The value is assessed looking at the tradeoffs people make when choosing to conduct an activity a certain risk level versus another activity at a different risk, but different cost/time. Studies are based both on what people actually pay and what are willing to pay, and use a variety of revealed preference techniques. This is the preferred method of the US Federal Highway Administration.
Human Capital: The Human Capital approach is an accounting approach which focuses on the accident victim’s productive capacity or potential output, using the discounted present value of future earnings. To this are added costs such as property damage and medical costs. Pain and suffering can added as well. The Human Capital approach can be used for accidents, environmental health, and possibly congestion costs . It is used in the Australian study Social Cost of Road Accidents (1990) [19]. However, Miller (1992) [20] and others discount the method because the only effect of injury that counts is the out-of-pocket cost plus lost work and housework. By extension, it places low value on children and perhaps even a negative value on the elderly. While measuring human capital is a necessary input to the costs of accidents, it cannot be the only input.
Stated Preference
[edit | edit source]Stated preference involves using hypothetical questions to determine individual preferences regarding the economic costs of a facility. There are two primary classes of stated preference studies: Contingent Valuation and Conjoint Analysis.
Contingent Valuation: Perhaps the most straight-forward way of determining the cost of an externality is asking the hypothetical questions, “How much you would a person pay to reduce externality by a certain amount” or “How would a person pay to avoid the imposition of a certain increment of externality”. Jones-Lee (1990) [21] has been the foremost investigator into this method for determining the cost of noise. This method can, in theory, be applied to any recipient of noise, although it has generally been asked of the neighbors (or potential neighbors) of a transportation facility.There are several difficulties with this approach. The first difficulty with any stated preference approach is that people give hypothetical answers to hypothetical questions. Therefore, the method should be calibrated to a revealed preference approach (with actual results for similar situations) before being relied upon as a sole source of information. The second regards the question of “rights”. For instance, someone who believes he has the right to quiet will not answer this question in the same way as someone who doesn’t. The third involves individuals who may claim infinite value to some commodity, which imposes difficulties for economic analysis.
Conjoint Analysis: To overcome the problems with contingent valuation, conjoint analysis has been used. Conjoint analysis requires individuals to tradeoffs between one good (e.g. quiet) and another (e.g. accessibility) has been used to better measure the cost of noise, as in Toronto by Gillen (1990) [22].
Implied Preference
[edit | edit source]There are methods for measuring the costs of externalities which are neither revealed from individual decisions nor stated by individuals on a survey. These are called implied preference because they are derived from regulatory or court-derived costs.
Regulatory Cost: Through government regulation, costs are imposed society with the aim of reducing the amount of noise or pollution or hazard is produced. These regulations include vehicle standards (e.g. mufflers) roadway abatement measures such as noise walls, as well as the many environmental regulations. By determining the costs and benefits of these regulations, the implicit cost of each externality can be estimated. This measure assumes that government is behaving consistently and rationally when imposing various standards or undertaking different projects.
Judicial Opinion and Negotiated Compensation: Similar to the implicit cost measure, one can look at how courts (judges and juries) weigh costs and benefits in cases which come before them. The cost per unit of noise or life from these judgments can be determined. This method is probably more viable in accident cases.
Incidence, Cost Allocation, and Compensation
[edit | edit source]This final set of topics deal with incidence (who causes the externality), cost allocation (who suffers from the externality), and compensation (how can the costs be appropriated and compensation paid fairly).
Incidence
[edit | edit source]The general model is that the costs can be generated by one of several parties and fall on one of several parties. The parties in this case are: the vehicle operators and carriers; the road, track, and airport operators; and the rest of society.
- Vehicle Operators and Carriers: bus company, truck company, driver of a car, railroad, airline
- Road/Track/Airport Operator: Department of Transportation, railroad, airport authority
- Society: the citizenry, government, citizens of other states/countries, the environment
This conceptual model is not concerned with anything smaller than the level of a vehicle. How costs on a vehicle are attributed to passengers in the vehicle, or the costs of freight carriage to the shipper, is not our concern. Similarly, ownership is not an issue, the operator of a vehicle may not be the owner, in the case, for instance, of a rented car. Obviously there is some overlap here between vehicle operators and road and track operators. In the case of American railroads, the firm which operates trains usually owns the track, although often a train will ride on tracks owned by a different railroad. Moreover, for some means of transportation, but not those considered here, there may be no vehicles (for example pipelines and conveyor belts.)
Costs can be imposed by any party on any party. As an illustration of how this works, we look at noise. Transportation noise is generated by vehicles in motion, and can affect any of the following classes: self, other vehicle users, and local society. There is noise generated by the roadway or the rail during construction, but this is ignored, and the noise does not actually hurt the road and track operators (except indirectly where they are held responsible for noise generated by vehicles and must build noise walls or other abatement measures.) A similar situation occurs with airports. Technically the planes make almost all of the noise, but the airport is held responsible. That noise is generated by wheels on pavement and thus depends in some respects on the roadway operator is also ignored.
- Vehicle operator on self, on other vehicles. For instance, one of the attributes of a vehicle (an auto say) is its quietness, this is reflected in the price of the vehicle. Quietness has two aspects: insulation, which protects the cab from noise generated by the car and other vehicles; and noise generation, which is how noisy the car is to itself and others. The noise generated by the vehicle and heard within the cab are internal costs, while those generated by the vehicle and heard by others is external to the vehicle operator, but internal to the transportation system.
- Vehicle operator on society. The noise generated by a vehicle negatively impacts the usefulness and flexibility of land uses nearby, where the impact declines with distance. The decline in utility is reflected in land values. The costs are clearly external to both the operator and the transportation system.
Cost Allocation
[edit | edit source]Clearly there are external costs, but it is not always clear who should bear them. This issue brings about questions of cost allocation. These include: objectives - for what reason are we allocating costs, methodology - how are we allocating costs, structure - how do we break down costs, and problems - how do we deal with the thorny issues of common and joint costs and cross-subsidies.
The first question that must be asked is what are the objectives of cost allocation. There are several contenders, which unfortunately are not entirely compatible. These include equity, efficiency, effectiveness, and acceptability.
The first consideration is equity or fairness. This concept raises a series of question summarized as “equity for whom”. Depending on how you slice it, different “fair” solutions are possible. The classic divisions are vertical vs. horizontal equity. Horizontal equity is a fair allocation of costs between users in the same sector, vertical equity is fairness across sectors. Are the costs allocated “fairly” between users, between facilities, between modes, between economic sectors? Is the burden for the project shared fairly between the economy and the environment? The second consideration is efficiency. Somewhat clearer than equity, efficiency still raises the same questions of “for whom.” Is the allocation efficient for the user, the operator, the state, the country? Does it consider inefficiencies, subsidies and taxes in other sectors of the economy, or other components of the transportation system? Efficiency can also be stratified into two categories: theoretical and practical.. The first ignores implementation (information and transaction) costs that rise with the number of charges imposed. Moreover, economists identify three kinds of efficiency: Allocative, which aims for the optimal mix of goods; Productive, which attempts to attain the minimum average cost; and Dynamic, which seeks long term optimal investment or capital rationing. Allocative efficiency may be thought of as congestion pricing, to ensure the optimal use of a transportation facility. Productive efficiency will attempt to raise enough money to operate and maintain the physical plant at the lowest cost. Dynamic efficiency will attempt to raise money to finance the facility, proactively or retroactively. To what extent these goals coincide is unclear.
Contrasted with efficiency is effectiveness. While the test of efficiency asks if the system is achieving its goals with minimum effort, the test of effectiveness asks if the system’s goals or output measures are consistent with broader societal goals. For instance, an efficient road may move traffic through a neighborhood at a high rate of speed, but this may be ineffective in meeting the broader social goal of a higher quality of life in the neighborhood, which the traffic disrupts. Costs can be allocated which achieve an efficient use of resources, but result in an ineffective or counter-productive system.
Added to this, we will consider the profit motive. If the facility is constructed by a profit seeking firm, prices will reflect an attempt at profit maximization in either a competitive, monopolistic, or oligopolistic environment.
A last consideration is acceptability. A system, which may have desirable attributes, if unimplemented, serves no-one. In the political world, tradeoffs and compromises must be made to achieve progress.
Costs can be allocated based on who causes them or by who receives benefit from them. There are pricing schemes reflecting both. There is a dichotomy between the methods of cost allocation suggested by economists and the approaches taken by engineers (as well as the official policy of the US government through modal cost allocation studies).
At least three economic approaches can be taken for allocating costs. The economic top-down approaches take equations of cost and allocate the results to users, these are: average total cost per user, average variable cost per user, and marginal cost (short run and long run), the last of which is favored by economists.
On the other hand, engineers working from the bottom-up break the system into components, which are assigned to users. Each mode or carrier has somewhat different methods for cost allocation. These are summarized below:
- Fixed Allocation - a set fee is charged based on some previous study
- Industry Agreed Upon (e.g. General Managers Associations Rules - rules allocating costs of freight cars on foreign rails, a pre-established agreement)
- Zero Allocation - user gets free ride on common costs and pays only attributable costs
- Proportional (New Investment/Long Range Pricing) - divides variable and fixed costs to users in proportion to use
- Minimum Cost of Service: Avoidable Cost Allocation (hierarchy costs/avoidable costs/separable costs/remaining benefits) - assigns to a beneficiary only the costs which could be avoided if the beneficiary did not use the service
- Minimum Cost of Service: Attributable Cost Allocation - assigns as cost allocation + share of common costs based on use.
- Minimum Cost of Service: Priority of Use Cost Allocation - assigns attributable cost allocation, but charges extra if priority is given to user or discounts if priority is taken from user (e.g. queue jumping)
In addition to the centralized cost allocation methods described above, there are other methods of allocation to users:
- Negotiated contracts - the parties negotiate the charge based on individual circumstances. This is often used in the rail industry where the trains of one carrier use the tracks of another.
- Arbitration - like a negotiated contract, but where a third party makes ultimate decision on the charge.
- Regulatory finding - A regulatory agency such as the former Interstate Commerce Commission gathers information and makes a decision as to appropriate rate. This is now most widely used in cases of monopoly oligopoly practice.
- Legislative finding - A legislature assumes the role of regulatory agency and prices and/or conditions of the cost allocation. An example of this is the adoption of taxes supporting the highway system, where gas taxes, vehicle licenses, and truck charges as well as tolls have to be approved by the state legislature.
- Judicial finding - After some dispute between parties (carrier vs. carrier, carrier vs. government or government vs. government) a court may be called on to make a final decision.
- Ramsey Pricing Rule - This rule would charge based on the customer’s elasticity of demand. The more elastic the customer (the more options he has the lower his price. So long as the short run marginal cost is covered, it may worthwhile for one firm to use this pricing rule to keep customers using their service rather than a competitors.
- Discriminating Monopolist/Oligopolist An unregulated monopoly discriminate among customers to obtain higher revenues (capture the consumer surplus). There are three classes of monopolistic discrimination: (1st degree, degree, 3rd degree).
The engineering and economic cost allocation discussed above allocate the costs to users. But there are alternative approaches:
- General Revenue: If transportation is to be subsidized, then the general public (including both users and non-users) can be charged a certain percentage of costs. This is seen when using general tax revenue for transportation.
- Value Capture: Similarly, another transfer occasionally used is a “value capture” approach, whereby nearby landowners are taxed based on the increase property value owing to a new transportation facility, this has been used in Angeles around new transit stations. In practice, some of each approach may be used.
Compensation
[edit | edit source]If individuals and organizations who cause externalities are to be charged, those who receive the unwanted noise, pollution, etc. should be compensated. To the extent that the recipients are amorphous, such as the environment, the collected funds should be expended in that sector for remediation of damages or their mitigation ahead of time. Also, the health damages from environmental damage are typically diffuse. On the other hand, it is fairly clear who suffers from noise. But the externality gets buried in the land price immediately after the opening (or perhaps announcement) of a facility. Therefore only the land owner at that time should receive compensation. Crashes result in damages to several classes of parties: those involved in crashes (and their families and insurance companies), commuters delayed by crashes (though this may be better treated in the congestion section), and society at large. Those involved are largely covered privately through the insurance sector, and care must be taken to avoid double-counting.
Congestion is typically divided into two classes: recurring and non-recurring. Non-recurring congestion is most often caused by incidents (traffic accidents, inclement weather). The value of time for these may be different, as recurring congestion probably entails less schedule delay since it is already accounted for by most commuters. Money raised from congestion pricing, in addition to reducing traffic volumes, can be used to expand capacity further to alleviate congestion. But this does not compensate those who now take a slower (but cheaper mode of transport) after road pricing is in effect. A question arises as to whether those individuals have some right to free travel which is being eliminated through pricing, or whether some general subsidies for travel are warranted. Congestion has further issues concerning pricing, for instance the peak vs. off-peak. When there is more traffic, each additional vehicle has more and more impact, suggesting higher tolls in the peak. However, the tolls will reduce demand, so an equilibrium solution to the problem is essential.
Social severance and visual impact are also amorphous. They will be difficult to price. To some extent for visual impact, the neighbors of a project can be identified and damages defined in terms of lower property values. In terms of the aesthetic quality of a trip, it may be conceptually possible to compare to parallel routes (a parkway vs. a freeway), one prettier than the other, and see if there is a difference in traffic volumes other than that explained by a route choice model. The difference in volume gives an implied choice of the value of the route in terms of additional time (and thus money), which may be significant in tourist areas. There is also a risk aspect to travel, drivers may choose certain roads which are through good areas, because they do not want to break down in isolated areas or perceived bad neighborhoods.
The social aspects of disruption of community (after taking into account net change in property value before and after infrastructure accounting for all of accessibility (increase or decrease), noise, and visual impact) is extremely difficult to determine. A political solution may need to be found to pricing and arranging for compensation.
Evidence on Pollution
[edit | edit source]Transportation sources in North America contribute approximately
- 47% of nitrogen oxide emissions (NOx)
- 71% of carbon monoxide emissions (CO)
- 39% of hydrocarbon emissions (HC)
Standards vs. Prices
[edit | edit source]To control most pollutants we have opted for standards rather than pricing. This is reflected in the 'level of allowed emissions' with catalytic converters on our vehicles.
Noise is another example where the U.S. has opted for a technological fix to achieve a standard. Europeans have, however, introduced noise charges at some airports for aircraft which exceed a particular noise level.
Private Cost v. Social Cost
[edit | edit source]The purpose of distinguishing private and social cost is to correct for real resource misallocation from economic agents actions which impose a cost (or benefit) on others in the market. The market provides no incentive for agents to take account of their actions.
The difference between private and social cost is that in making a decision a private individual will take account of the costs they face but will not consider the impact of their decision on others which may, in fact. impose a cost upon them. If this occurs an externality will misallocate resources since the economic agents are not forced to pay the cost they impose or does not receive any compensation for the benefits which they confer.
Full Cost Model
[edit | edit source]Based on: [23]
An essential first step in examining transportation issues and in making sound decisions on transportation systems is to understand the full cost of transportation today, including the social costs of crashes, air pollution, noise, and congestion as well as the internal costs of providing and operating the infrastructure. Furthermore, if cross subsidies between modes, user groups, or areas of the country or states are to be avoided, and if users are to pay the full cost of providing and maintaining the transportation system, then it is important to know what proportion of total costs users currently pay and what proportion is borne by others. Such a complete assessment of the full cost of the different modes of transportation for intercity travel has been lacking. The development of cost models and estimates of the type presented in this research are essential to gauging the true costs of transportation in the different modes, and is a prerequisite to sound investment decisions.
The full cost calculation includes the cost of building, operating, and maintaining infrastructure, as well as carrier, user, and social costs. Social costs include noise, air pollution, and accident costs, as well as congestion costs. User costs include the cost of purchasing, maintaining and operating a vehicle such as a car, and the cost of travel time.
We begin by developing a taxonomy for representing the full costs of transportation, independent of mode:
- Infrastructure Costs - including capital costs of construction and debt service, and costs of maintenance and operating costs as well as service costs to government or private sector;
- Carrier Costs - aggregate of all payments by carriers in capital costs to purchase a vehicle fleet), and maintain and operate a vehicle fleet (COC), minus those costs (such as usage charges) which are transfers to infrastructure, which we label Carrier Transfers.
- User Money Costs - aggregate of all fees, fares and tariffs paid by users in capital costs to purchase a vehicle, and money spent to maintain and operate the vehicle or to ride on a carrier (UOC); less those costs (such as fares) which are transfers to carriers or infrastructure, and accident insurance, which is considered under social costs, which we label User Transfers.
- User Travel Time Costs - the amount of time spent traveling under uncongested conditions multiplied by the monetary value of time.
- User Delay Costs- the amount of time spent traveling under congested conditions minus the amount of time spent traveling in uncongested conditions multiplied by the monetary value of time.
- Social Costs - additional net external costs to society due to emissions , crashes , and noise and are true resource costs used in making and using transportation services;
The method used to estimate the full cost of intercity travel will combine elements from a number of sources. Adding and subtracting the above factors, thereby avoiding double-counting, we have the following equation, the components of which will be dealt with in turn in the paper:
Key Issues
[edit | edit source]“Externalities” are Inputs to Production System. Clean Air, Quiet, Safety, Freeflow Time are used to produce a trip. The System has boundaries: Direct effects vs. Indirect effects Double Counting must be avoided
Selection of Externalities
[edit | edit source]Criteria: Direct Effects
Not Internalized in Capital or Operating Costs
External to User (not necessarily to system)
Result: Noise, Air Pollution, Congestion, Crashes
Not: Water Pollution, Parking, Defense ...
Approach
[edit | edit source]Noise
[edit | edit source]Measurement
[edit | edit source]Noise: Unwanted Sound
dB(A) = 10 log (P2/Pref)
P: Pressure, Pref: queitest audible sound
NEF: Noise Exposure Forecast is a function of number (frequency) of events and their loudness.
Generation
[edit | edit source]Amount of noise generated is a function of traffic flow, speed, types of traffic.
Additional vehicles have non-linear effect: e.g. 1 truck = 80 db, 2 trucks = 83 db, but sensitivity to loudness also rises
Noise decays with distance
Valuation
[edit | edit source]Hedonic Models: Decline of Property Values with Increase in Noise --> Noise Depreciation Index (NDI). Average NDI from many highway and airport studies is 0.62. For each unit increase in dB(A), there is a 0.62% decline in the price of a house
Integration
[edit | edit source]Noise Cost Functions ($/pkt) : f(Quantity of Noise, House Values, Housing Density, Interest Rates)
Using “reasonable” assumption, this ranges from $0.0001/vkt - $0.0060/vkt for highway. Best guess = $0.0045/pkt. For air, about the same, $0.0043/pkt.
Air Pollution
[edit | edit source]Measurement
[edit | edit source]Air Pollution Problems: Smog, Acid Rain, Ozone Depletion, Global Climate Change.
EPA “Criteria” Pollutants: HC (a.k.a. VOC, ROG), NOx, CO, SOx, PM10
Other Pollutants: CO2
Generation
[edit | edit source]| Mode | Pax km | HC kg, M | CO kg,M | NOx kg, M | C,Ton |
| (gm/pkt) | (gm/pkt) | (gm/pkt) | M | ||
| (gm/pkt) | |||||
| Highways | 5.4 x1012 | 5,118 | 32,690 | 5,945 | 263.2 |
| -0.95 | -6.053 | -1.11 | -46 | ||
| Jets | 5.8 x1011 | 54 | 163 | 72.7 | 59.2 |
| -0.093 | -0.28 | -0.13 | -100 | ||
| Total | 6,409 | 39,972 | 7,918 | ||
| Transport | |||||
| Total All | 18,536 | 60,863 | 19,890 | ||
| Sources | |||||
Valuation
[edit | edit source]Local Health Effects, Material and Vegetation Effects, Global Effects Greatest Uncertainty in Global Effects, Proposed “Carbon Tax” have 2 orders of magnitude differences
Integration
[edit | edit source]| Pollutant | Air Transportation Cost | Highway Transportation Costs |
| ($/pkt) | ($/vkt) | |
| PM10 | --- | $0.000066 |
| SOx | --- | $0.00024 |
| HC | $0.00012 | $0.0030 |
| CO | $0.0000018 | $0.000049 |
| NOx | $0.00017 | $0.0010 |
| Carbon | $0.00058 | $0.00026 |
| TOTAL | $0.00087 | $0.0046 |
Congestion
[edit | edit source]Measurement
[edit | edit source]Time: Congested, Uncongested
Congested Time Increases as Flow Approaches, Exceeds “Capacity”
Uncongested Time: Freeflow Time + Schedule Delay
Generation
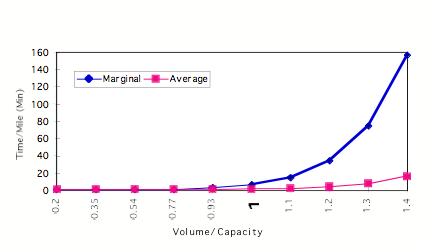
[edit | edit source]Air Transportation: Delay vs. Usage
Valuation
[edit | edit source]The value of time depends on a number of factors (Hensher 1995). Among them are the mode of travel, the time of day, the purpose (business, non-business) of the trip, the quality or level of service of the trip (including speed), and the specific characteristics of the trip-maker, including income. Furthermore, the value of time saved probably depends on the amount of time saved - 60 people saving 1 minute may not be worth the same as 1 person saving 60 minutes. Time in motion is valued differently than time spent waiting. Similarly schedule delay, the amount of time between when one wants to depart and the next scheduled service (bus, train, plane) also has a value associated with it. Unexpected delays are more costly than the expected, since those are built into decisions. All of these factors need to be considered in a detailed operational analysis of the costs of travel time and congestion.
Value of Time is a function of mode, time of day, purpose, quality of service, trip-maker.
Wide range, typically $50/hr air, $30/hr car. (Business Trips more valuble than Personal Trips).
On other hand, average hourly PCI rate (40 hour week) gives $10/hr
Integration
[edit | edit source]Time Cost Functions:
TC = VoT Qh ( Lf/ Vf + a (Qh / Qho)b)
highway: a=0.32, b=10
air: a=2.33, b=6
Crashes
[edit | edit source]Measurement
[edit | edit source]Number of Crashes by Severity Multiple Databases (NASS, FARS) Multiple Agencies (NHTSA, NTSB), + states and insurance agencies Inconsistent Classification Non-reporting
Generation
[edit | edit source]Crash Rates, Functions Highway: Crash Rate = f(urban/rural, onramps, auxiliarly lanes, flow, queueing) Air: Crash Rate = f( type of aircraft)
Valuation
[edit | edit source]The principal means for estimating the cost of crashes is to estimate their damage costs. The method presented here uses a comprehensive approach which includes valuing years lost to the accident as well as direct costs. Several steps must be undertaken: converting injuries to years of life, developing a value of life, and estimating other costs. Placing a value on injury requires measuring its severity. Miller (1993) describes a year of functional capacity (365 days/year, 24 hours/day) as consisting of several dimensions: Mobility, Cognitive, Self Care, Sensory, Cosmetic, Pain, Ability to perform household responsibilities, and Ability to perform wage work. The following Tables show the percent of hours lost by degree of injury, and the functional years lost by degree of injury.
Percentage of Hours Lost to Injuries by Degree of Injury
| Type of Activity | Modest | Major | Fatal | Total |
| Functioning | 18.0 | 40.7 | 41.3 | 100.0 |
| HH Production | 25.2 | 22.1 | 52.7 | 100.0 |
| Work | 21.7 | 19.1 | 59.2 | 100.0 |
source Miller (1991) p.26
Functional Years lost by Degree of Injury
| Degree of Injury | Per Injury | Percent of Lifespan | Per Year | Percent of Annual Total |
| 1. Minor | 0.07 | 0.15 | 316,600 | 10.7 |
| 2. Moderate | 1.1 | 2.3 | 587,700 | 20.0 |
| 3.Serious | 6.5 | 13.8 | 1,176,700 | 40.0 |
| 4. Severe | 16.5 | 35.0 | 446,700 | 15.2 |
| 5. Critical | 33.1 | 70.0 | 413,800 | 14.1 |
| Avg. Nonfatal | 0.7 | 1.5 | 2,941,500 | 100.0 |
| Fatal | 42.7 | 100.0 | 2.007,000 | |
source Miller (1991) p29 note: expected lifespan for nonfatally injured averages 47.2 years
Federal Highway Administration uses the following:
| MAIS Level | Severity | Fraction of VSL |
| MAIS 1 | Minor | 0.0020 |
| MAIS 2 | Moderate | 0.0155 |
| MAIS 3 | Serious | 0.0575 |
| MAIS 4 | Severe | 0.1875 |
| MAIS 5 | Critical | 0.7625 |
| MAIS 6 | Fatal | 1.0000 |
source:[24]
Central to the estimation of costs is an estimate of the value of life (or value of a statistical life). Numerous studies have approached this question from various angles. Jones-Lee (1988) provides one summary, with an emphasis on British values from revealed and stated preference studies. The FAA (1989) provides another summary. He finds the range of value of life to vary by up to two orders of magnitude (a factor of 100). Miller’s (1991) summary is reproduced below, with numbers updated to 1995 dollars.
Estimated Value of Life by Type of Study
| Type of Study | Value of Life ($) | |
| (1988 dollars) | Value of Life ($) | |
| (1995 dollars) | ||
| Average of 49 studies | 2.2 M | 2.9 M |
| Average of 11 auto safety studies | 2.1 M | 2.7 M |
| Study Type | ||
| Extra wages for risky jobs (30 studies) | 1.9-3.4 M | 2.5 - 4.4 M |
| Market demand vs. price | ||
| safer cars | 2.6 M | 3.4 M |
| smoke detectors | 1.2 M | 1.6 M |
| houses in less polluted areas | 2.6 M | 3.4 M |
| life insurance | 3.0 M | 3.9 M |
| wages | 2.1 M | 2.7 M |
| Safety behavior | ||
| pedestrian tunnel use | 2.1 M | 2.7 M |
| safety belt use (2 studies) | 2.0 - 3.1 M | 2.6 - 4.0 M |
| speed choice (2 studies) | 1.3 -2.2 M | 1.7 - 2.9 M |
| smoking | 1.0 M | 1.3 M |
| Surveys | ||
| Auto safety (5 studies) | 1.2-2.8 M | 1.6 - 3.6 M |
| Cancer | 2.6 M | 3.4 M |
| Safer Job | 2.2 M | 2.9 M |
| Fire Safety | 3.6 M | 4.7 M |
Source: Miller (1990), Note: in millions (M) of after-tax dollars ($1995 = $1988 * 1.3).
Currently (as of 2008 $) FHWA uses $5.8 M[25], which is the average of several recent studies.
Integration
[edit | edit source]Highway Accident Costs estimates range from $0.002 - $0.09/pkt. Our estimate is $0.02/pkt. Urban / rural tradeoff. Urban more but less severe crashes. Air Accident Costs $0.0005/pkt.
Summary
[edit | edit source]Costs in $ per passenger km traveled.
| Cost Category | Air System | Highway System |
| Noise | $0.0043 | $0.0045 |
| Air Pollution | $0.0009 | $0.0031 |
| Crashes | $0.0004 | $0.0200 |
| Congestion | $0.0017 | $0.0046 |
| TOTAL | $0.01 | $0.03 |
High Uncertainty About Valuation
Costs Vary with Usage
Accounting, Difficult, but necessary to avoid double counting.
Thought Question: Value of Life
[edit | edit source]Suppose there is a road improvement which will save 1 life per year, reducing the number of fatalities from 2 to 1 per year (out of 1000 people using the road). Assume all travelers are identical. What value of life should be used in the analysis?
Normally, we would do the equivalent of trying to compute for each traveler what is the willingness to pay for a 50% reduction in the chance of death by driving (from 2 in 1000 to 1 in 1000), and multiply that by the 1000 people whose chance of dying is reduced.
An alternative approach is to figure out the willingness to pay for the driver whose life is saved. So how much would you pay to avoid dying (with certainty) (i.e. what is your Willingness to Pay)? The answer to the first question is usually taken to be all of your resources (you would pay you everything so I won't kill you).
Alternatively how much can I pay you to allow you to let me kill you (Willingness to Accept)? The answer to this second question is: I would have to pay you an infinite amount of money in order for you to let me kill you.
Both of those sums of money (everything or infinity) likely exceed the willingness to pay to reduce the likelihood of dying with some probability, multiplied by the number of people experiencing it.
In economic terms, we are comparing the area under the demand curve (the consumer's surplus) for life (which has a value asymptotically approaching infinity as the amount of life approaches 0 (death approaches certainty) for a single individual, with the marginal change in the likelihood of survival multiplied by all individuals (i.e. the quadrilateral between the y-axis of price and the same demand curve, between Pb and Pa) which describes the change in price for a change in survival).
On the one hand, using the marginal change for everyone rather than total change for the one person whose life is saved, we will give a lower value to safety improvements. On the other hand, the value of life to the individual himself is much higher than the value of life of that individual to society at large.
References
[edit | edit source]- ↑ Keeler, T.E., K. Small and Associates (1975), The Full Costs of Urban Transport, Part III: Automobile Costs and Final Intermodal Cost Comparisons, Monograph No. 212, Institute of Urban and Regional Development, University of California, Berkeley.
- ↑ Fuller, John W. et al (1983) Measurements of Highway User Interference Costs and Air Pollution Costs and Noise Damage Costs: Final Report 34 University of Iowa Institute of Urban and Regional Research
- ↑ Mackenzie, James, Roger C. Dower, Donald D.T. Chen (1992) The Going Rate: What it Really Costs to Drive World Resources Institute Washington, DC
- ↑ INRETS (1993) Impact des Transport Terrestres sur L’Environment: Methodes d’evaluation et couts sociaux. Synthese Inrets No. 23.
- ↑ Miller, Peter and John Moffet (1993) The Price of Mobility: Uncovering the Hidden Costs of Transportation, National Resources Defense Council
- ↑ IWW/INFRAS (1995) The External Effects of Transport for International Union of Railways, Zurich and Karlsruhe
- ↑ IBI Group (1995) Full Cost Transportation Pricing Study: Final Report to Transportation and Climate Change Collaborative.
- ↑ Verhoef, Erik External Effects and Social Costs of Road Transport Transportation Research A Vol. 28A No. 4 pp. 273-387, 1994
- ↑ Rothengatter, Werner Do External Benefits Compensate for External Costs of Transport Transportation Research A Vol. 28A No. 4 pp. 321-328, 1994
- ↑ Button, Kenneth Alternative Approaches Toward Containing Transport Externalities: An International Comparison Transportation Research A Vol. 28A No. 4 PP. 289-305, 1994
- ↑ Gwilliam, Kenneth M. and Harry Geerlings New Technologies and Their Potential to Reduce the Environmental Impact of Transportation Transportation Research A Vol. 28A No. 4 pp. 307-319, 1994
- ↑ Rietveld, Piet Spatial Economic Impacts of Transport Infrastructure Supply Transportation Research A Vol. 28A No. 4 pp. 329-341, 1994
- ↑ Coase, R.H. The Problem of Social Cost, and Notes on the Problem of Social Cost reprinted in The Firm, The Market and the Law University of Chicago Press (1992)
- ↑ Stigler, George 1966 The Theory of Price, 3rd ed. (New York: Macmillan and Col. 1966), 113
- ↑ Pigou, A.C. 1920 orig. The Economics of Welfare, 4th ed. (London, Macmillan and Co. 1932)
- ↑ Button, Kenneth Alternative Approaches Toward Containing Transport Externalities: An International Comparison Transportation Research A Vol. 28A No. 4 PP. 289-305, 1994
- ↑ DeLuchi, M.A. (1991) Emissions of Greenhouse Gases from the Use of Transportation Fuels and Electricity: Volume 1 Main Text. Department of Energy: Argonne National Laboratory, Center for Transportation Research, Energy Systems Division.
- ↑ Becker, Gary, A Theory of the Allocation of Time - The economic journal, 1965 pp. 493--517
- ↑ Australian Bureau of Transport and Communications (1992) Social Cost of Road Accidents in Australia, Economics Report 79, Australian Government Publishing Service, Canberra, Australia
- ↑ Miller, Ted (1992) The Costs of Highway Crashes, Federal Highway Administration (FHWA-RD-91-055)
- ↑ Jones-Lee, Michael The Value of Transport Safety. Oxford Review of Economic Policy, Vol. 6, No. 2, Summer 1990 pp. 39-60
- ↑ Gillen, David (1990) The Management of Airport Noise, DWG Research Associates for Transport Development Centre, Transport Canada, July 1990
- ↑ Levinson, David, David Gillen, and Adib Kanafani (1998) A Comparison of the Social Costs of Air and Highway. Transport Reviews 18:3 215-240.)
- ↑ Duvall, Tyler (2008) Treatment of the Economic Value of a Statistical Life in Departmental Analyses http://ostpxweb.dot.gov/policy/reports/080205.htm
- ↑ http://www.fhwa.dot.gov/policy/2008cpr/appb.htm