Geometry/Print version
| This is the print version of Geometry You won't see this message or any elements not part of the book's content when you print or preview this page. |
The current, editable version of this book is available in Wikibooks, the open-content textbooks collection, at
https://en.wikibooks.org/wiki/Geometry
Chapter 1: Points, Lines, Line Segments and Rays
[edit | edit source]Points and lines are two of the most fundamental concepts in Geometry, but they are also the most difficult to define. We can describe intuitively their characteristics, but there is no set definition for them: they, along with the plane, are the undefined terms of geometry. All other geometric definitions and concepts are built on the undefined ideas of the point, line and plane. Nevertheless, we shall try to define them.
Point
[edit | edit source]A point is an exact location in space. A point is denoted by a dot. A point has no size.
Line
[edit | edit source]As for a line segment, we specify a line with two endpoints. Starting with the corresponding line segment, we find other line segments that share at least two points with the original line segment. In this way we extend the original line segment indefinitely. The set of all possible line segments findable in this way constitutes a line. A line extends indefinitely in a single dimension. Its length, having no limit, is infinite. Like the line segments that constitute it, it has no width or height. You may specify a line by specifying any two points within the line. For any two points, only one line passes through both points. On the other hand, an unlimited number of lines pass through any single point.
Ray
[edit | edit source]We construct a ray similarly to the way we constructed a line, but we extend the line segment beyond only one of the original two points. A ray extends indefinitely in one direction, but ends at a single point in the other direction. That point is called the end-point of the ray. Note that a line segment has two end-points, a ray one, and a line none. An angle can be formed when two rays meet at a common point. The rays are the sides of the angle. The point of the end of two rays is called the vertex.
Plane
[edit | edit source]A point exists in zero dimensions. A line exists in one dimension, and we specify a line with two points. A plane exists in two dimensions. We specify a plane with three points. Any two of the points specify a line. All possible lines that pass through the third point and any point in the line make up a plane. In more obvious language, a plane is a flat surface that extends indefinitely in its two dimensions, length and width. A plane has no height.
Space
[edit | edit source]Space exists in three dimensions. Space is made up of all possible planes, lines, and points. It extends indefinitely in all directions.
N-dimensional Space
[edit | edit source]Mathematics can extend space beyond the three dimensions of length, width, and height. We then refer to "normal" space as 3-dimensional space. A 4-dimensional space consists of an infinite number of 3-dimensional spaces. Etc.
Chapter 2: Angles
[edit | edit source]An angle is the union of two rays with a common endpoint, called the vertex. The angles formed by vertical and horizontal lines are called right angles; lines, segments, or rays that intersect in right angles are said to be perpendicular.
Angles, for our purposes, can be measured in either degrees (from 0 to 360) or radians (from 0 to ). Angles length can be determined by measuring along the arc they map out on a circle. In radians we consider the length of the arc of the circle mapped out by the angle. Since the circumference of a circle is , a right angle is radians. In degrees, the circle is 360 degrees, and so a right angle would be 90 degrees.
Naming Conventions
[edit | edit source]Angles are named in several ways.
- By naming the vertex of the angle (only if there is only one angle formed at that vertex; the name must be non-ambiguous)
- By naming a point on each side of the angle with the vertex in between.
- By placing a small number on the interior of the angle near the vertex.
Classification of Angles by Degree Measure
[edit | edit source]Acute Angle
- an angle is said to be acute if it measures between 0 and 90 degrees, exclusive.
Right Angle
- an angle is said to be right if it measures 90 degrees.
- notice the small box placed in the corner of a right angle, unless the box is present it is not assumed the angle is 90 degrees.
- all right angles are congruent
Obtuse Angle
- an angle is said to be obtuse if it measures between 90 and 180 degrees, exclusive.
Special Pairs of Angles
[edit | edit source]- adjacent angles
- adjacent angles are angles with a common vertex and a common side.
- adjacent angles have no interior points in common.
- complementary angles
- complementary angles are two angles whose sum is 90 degrees.
- complementary angles may or may not be adjacent.
- if two complementary angles are adjacent, then their exterior sides are perpendicular.
- supplementary angles
- two angles are said to be supplementary if their sum is 180 degrees.
- supplementary angles need not be adjacent.
- if supplementary angles are adjacent, then the sides they do not share form a line.
- linear pair
- if a pair of angles is both adjacent and supplementary, they are said to form a linear pair.
- vertical angles
- angles with a common vertex whose sides form opposite rays are called vertical angles.
- vertical angles are congruent.
Chapter 3: Properties
[edit | edit source]Triangle Properties
[edit | edit source]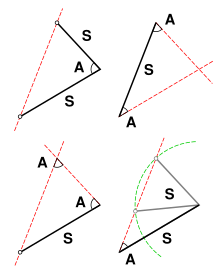
Side-Side-Side (SSS) (Postulate 12) If three sides of one triangle are congruent to three sides of a second triangle, then the two triangles are congruent.
Side-Angle-Side (SAS) (Postulate 13)
If two sides and the included angle of a second triangle, then the two triangles are congruent.
'Angle-Side-Angle' (ASA)
If two angles and the included side of one triangle are congruent to two angles and the included side of a second triangle, then two triangles are congruent.
'Angle-Angle-Side' (AAS)
If two angles and a non-included side of one triangle are congruent to two angles and the corresponding non-included side of a second triangle, then the two triangles are congruent.
NO - Angle-Side-Side (ASS)
The "ASS" postulate does not work, unlike the other ones. A way that students can remember this is that "ass" is not a nice word, so we don't use it in geometry (since it does not work).
Chapter 4. Inductive and Deductive Reasoning
[edit | edit source]There are two approaches to furthering knowledge: reasoning from known ideas and synthesizing observations. In inductive reasoning you observe the world, and attempt to explain based on your observations. You start with no prior assumptions. Deductive reasoning consists of logical assertions from known facts.
Basic Terms
[edit | edit source]Before one can start to understand logic, and thereby begin to prove geometric theorems, one must first know a few vocabulary words and symbols.
Conditional: a conditional is something which states that one statement implies another. A conditional contains two parts: the condition and the conclusion, where the former implies the latter. A conditional is always in the form "If statement 1, then statement 2." In most mathematical notation, a conditional is often written in the form p ⇒ q, which is read as "If p, then q" where p and q are statements.
Converse: the converse of a logical statement is when the conclusion becomes the condition and vice versa; i.e., p ⇒ q becomes q ⇒ p. For example, the converse of the statement "If someone is a woman, then they are a human" would be "If someone is a human, then they are a woman." The converse of a conditional does not necessarily have the same truth value as the original, though it sometimes does, as will become apparent later.
AND: And is a logical operator which is true only when both statements are true. For example, the statement "Diamond is the hardest substance known to man AND a diamond is a metal" is false. While the former statement is true, the latter is not. However, the statement "Diamond is the hardest substance known to man AND diamonds are made of carbon" would be true, because both parts are true.
OR: If two statements are joined together by "or," then the truth of the "or" statement is dependent upon whether one or both of the statements from which it is composed is true. For example, the statement "Tuesday is the day after Monday OR Thursday is the day after Saturday" would have a truth value of "true," because even though the latter statement is false, the former is true.
NOT: If a statement is preceded by "NOT," then it is evaluating the opposite truth value of that statement. The symbol for "NOT" is For example, if the statement p is "Elvis is dead," then ¬p would be "Elvis is not dead." The concept of "NOT" can cause some confusion when it relates to statements which contain the word "all." For example, if r is "¬". "All men have hair," then ¬r would be "All men do not have hair" or "No men have hair." Do not confuse this with "Not all men have hair" or "Some men have hair." The "NOT" should apply to the verb in the statement: in this case, "have." ¬p can also be written as NOT p or ~p. NOT p may also be referred to as the "negation of p."
Inverse: The inverse of a conditional says that the negation of the condition implies the negation of the conclusion. For example, the inverse of p ⇒ q is ¬p ⇒ ¬q. Like a converse, an inverse does not necessarily have the same truth value as the original conditional.
Biconditional: A biconditional is conditional where the condition and the conclusion imply one another. A biconditional starts with the words "if and only if." For example, "If and only if p, then q" means both that p implies q and that q implies p.
Premise: A premise is a statement whose truth value is known initially. For example, if one were to say "If today is Thursday, then the cafeteria will serve burritos," and one knew that what day it was, then the premise would be "Today is Thursday" or "Today is not Thursday."
⇒: The symbol which denotes a conditional. p ⇒ q is read as "if p, then q."
Iff: Iff is a shortened form of "if and only if." It is read as "if and only if."
⇔: The symbol which denotes a biconditonal. p ⇔ q is read as "If and only if p, then q."
∴: The symbol for "therefore." p ∴ q means that one knows that p is true (p is true is the premise), and has logically concluded that q must also be true.
∧: The symbol for "and."
∨: The symbol for "or."
Deductive Reasoning
[edit | edit source]There are a few forms of deductive logic. One of the most common deductive logical arguments is modus ponens, which states that:
- p ⇒ q
- p ∴ q
- (If p, then q)
- (p, therefore q)
An example of modus ponens:
- If I stub my toe, then I will be in pain.
- I stub my toe.
- Therefore, I am in pain.
Another form of deductive logic is modus tollens, which states the following.
- p ⇒ q
- ¬q ∴ ¬p
- (If p, then q)
- (not q, therefore not p)
Modus tollens is just as valid a form of logic as modus ponens. The following is an example which uses modus tollens.
- If today is Thursday, then the cafeteria will be serving burritos.
- The cafeteria is not serving burritos, therefore today is not Thursday.
Another form of deductive logic is known as the If-Then Transitive Property. Simply put, it means that there can be chains of logic where one thing implies another thing. The If-Then Transitive Property states:
- p ⇒ q
- (q ⇒ r) ∴ (p ⇒ r)
- (If p, then q)
- ((If q, then r), therefore (if p, then r))
For example, consider the following chain of if-then statements.
- If today is Thursday, then the cafeteria will be serving burritos.
- If the cafeteria will be serving burritos, then I will be happy.
- Therefore, if today is Thursday, then I will be happy.
Inductive Reasoning
[edit | edit source]Inductive reasoning is a logical argument which does not definitely prove a statement, but rather assumes it. Inductive reasoning is used often in life. Polling is an example of the use of inductive reasoning. If one were to poll one thousand people, and 300 of those people selected choice A, then one would infer that 30% of any population might also select choice A. This would be using inductive logic, because it does not definitively prove that 30% of any population would select choice A.
Because of this factor of uncertainty, inductive reasoning should be avoided when possible when attempting to prove geometric properties.
Truth Tables
[edit | edit source]Truth tables are a way that one can display all the possibilities that a logical system may have when given certain premises. The following is a truth table with two premises (p and q), which shows the truth value of some basic logical statements. (NOTE: T = true; F = false)
| p | q | ¬p | ¬q | p ⇒ q | p ⇔ q | p ∧ q | p ∨ q |
| T | T | F | F | T | T | T | T |
| T | F | F | T | F | F | F | T |
| F | T | T | F | T | F | F | T |
| F | F | T | T | T | T | F | F |
See also
[edit | edit source]Chapter 5: Proof
[edit | edit source]Introduction
[edit | edit source]Unlike science which has theories, mathematics has a definite notion of proof. Mathematics applies deductive reasoning to create a series of logical statements which show that one thing implies another.
Consider a triangle, which we define as a shape with three vertices joined by three lines. We know that we can arbitrarily pick some point on a page, and make that into a vertex. We repeat that process and pick a second point. Using a ruler, we can connect these two points. We now make a third point, not on the line through the first two points, and using the ruler connect it to each of the other points. We have constructed a triangle.
In mathematics we formalize this process into axioms, and carefully lay out the sequence of statements to show what follows. All definitions are clearly defined. In modern mathematics, we are always working within some system where various axioms hold.
Two-Column Proof
[edit | edit source]The most common form of explicit proof in high school geometry is a two column proof consists of five parts: the given, the proposition, the statement column, the reason column, and the diagram (if one is given).
Example of a Two-Column Proof
[edit | edit source]Now, suppose a problem tells you to solve for , showing all steps made to get to the answer. A proof shows how this is done:
Given:
Prove: x = 1
| Statement | Reason |
| Given | |
| Property of subtraction |
We use "Given" as the first reason, because it is "given" to us in the problem.
Written Proof
[edit | edit source]Written proofs (also known as informal proofs, paragraph proofs, or 'plans for proof') are written in paragraph form. Other than this formatting difference, they are similar to two-column proofs.
Sometimes it is helpful to start with a written proof, before formalizing the proof in two-column form. If you're having trouble putting your proof into two column form, try "talking it out" in a written proof first.
Example of a Written Proof
[edit | edit source]We are given that x + 1 = 2, so if we subtract one from each side of the equation (x + 1 - 1 = 2 - 1), then we can see that x = 1 by the definition of subtraction.
Flowchart Proof
[edit | edit source]A flowchart proof or more simply a flow proof is a graphical representation of a two-column proof. Each set of statement and reasons are recorded in a box and then arrows are drawn from one step to another. This method shows how different ideas come together to formulate the proof.
Chapter 6. Five Postulates of Euclidean Geometry
[edit | edit source]Postulates in geometry is very similar to axioms, self-evident truths, and beliefs in logic, political philosophy, and personal decision-making. The five postulates of Euclidean Geometry define the basic rules governing the creation and extension of geometric figures with ruler and compass. Together with the five axioms (or "common notions") and twenty-three definitions at the beginning of Euclid's Elements, they form the basis for the extensive proofs given in this masterful compilation of ancient Greek geometric knowledge. They are as follows:
- A straight line segment may be drawn from any given point to any other.
- A straight line may be extended to any finite length.
- A circle may be described with any given point as its center and any distance as its radius.
- All right angles are congruent.
- If a straight line intersects two other straight lines, and so makes the two interior angles on one side of it together less than two right angles, then the other straight lines will meet at a point if extended far enough on the side on which the angles are less than two right angles.
Postulate 5, the so-called Parallel Postulate was the source of much annoyance, probably even to Euclid, as it is not a simple, concise statement, as are the other four. Mathematicians, and really most of us, value simplicity arising from simplicity, with the long complicated proofs, equations, and calculations needed for rigorous certainty done behind the scenes, and to have such a long sentence amidst such other straightforward, intuitive statements seems awkward. As a result, many mathematicians over the centuries have tried to prove the results of the Elements without using the Parallel Postulate, but to no avail. However, in the past two centuries, assorted non-Euclidean geometries have been derived based on using the first four Euclidean postulates together with various negations of the fifth.
Chapter 7. Vertical Angles
[edit | edit source]Vertical angles are a pair of angles with a common vertex whose sides form opposite rays. An extensively useful fact about vertical angles is that they are congruent. Aside from saying that any pair of vertical angles "obviously" have the same measure by inspection, we can prove this fact with some simple algebra and an observation about supplementary angles. Let two lines intersect at a point, and angles A1 and A2 be a pair of vertical angles thus formed. At the point of intersection, two other angles are also formed, and we'll call either one of them B1 without loss of generality. Since B1 and A1 are supplementary, we can say that the measure of B1 plus the measure of A1 is 180. Similarly, the measure of B1 plus the measure of A2 is 180. Thus the measure of A1 plus the measure of B1 equals the measure of A2 plus the measure of B1, by substitution. Then by subracting the measure of B1 from each side of this equality, we have that the measure of A1 equals the measure of A2.
Chapter 8. Parallel and Perpendicular Lines and Planes
[edit | edit source]Parallel Lines in a Plane
[edit | edit source]Two coplanar lines are said to be parallel if they never intersect. For any given point on the first line, its distance to the second line is equal to the distance between any other point on the first line and the second line. The common notation for parallel lines is "||" (a double pipe); it is not unusual to see "//" as well. If line m is parallel to line n, we write "m || n". Lines in a plane either coincide, intersect in a point, or are parallel. Controversies surrounding the Parallel Postulate lead to the development of non-Euclidean geometries.
Parallel Lines and Special Pairs of Angles
[edit | edit source]When two (or more) parallel lines are cut by a transversal, the following angle relationships hold:
- corresponding angles are congruent
- alternate exterior angles are congruent
- same-side interior angles are supplementary
Theorems Involving Parallel Lines
[edit | edit source]- If a line in a plane is perpendicular to one of two parallel lines, it is perpendicular to the other line as well.
- If a line in a plane is parallel to one of two parallel lines, it is parallel to both parallel lines.
- If three or more parallel lines are intersected by two or more transversals, then they divide the transversals proportionally.
Chapter 9. Congruency and Similarity
[edit | edit source]Congruency
[edit | edit source]Congruent shapes are the same size with corresponding lengths and angles equal. In other words, they are exactly the same size and shape. They will fit on top of each other perfectly. Therefore if you know the size and shape of one you know the size and shape of the others. For example:
Each of the above shapes is congruent to each other. The only difference is in their orientation, or the way they are rotated. If you traced them onto paper and cut them out, you could see that they fit over each other exactly.
Having done this, right away we can see that, though the angles correspond in size and position, the sides do not. Therefore it is proved the triangles are not congruent.
Similarity
[edit | edit source]Similar shapes are like congruent shapes in that they must be the same shape, but they don't have to be the same size. Their corresponding angles are congruent and their corresponding sides are in proportion.
Chapter 10. Congruent Triangles
[edit | edit source]Methods of Determining Congruence
[edit | edit source]Two triangles are congruent if:
- each pair of corresponding sides is congruent
- two pairs of corresponding angles are congruent and a pair of corresponding sides are congruent
- two pairs of corresponding sides and the angles included between them are congruent
Tips for Proofs
[edit | edit source]Commonly used prerequisite knowledge in determining the congruence of two triangles includes:
- by the reflexive property, a segment is congruent to itself
- vertical angles are congruent
- when parallel lines are cut by a transversal corresponding angles are congruent
- when parallel lines are cut by a transversal alternate interior angles are congruent
- midpoints and bisectors divide segments and angles into two congruent parts
Chapter 11. Similar Triangles
[edit | edit source]
For two triangles to be similar, all 3 corresponding angles must be congruent, and all three sides must be proportionally equal. Two triangles are similar if...
- Two angles of each triangle are congruent.
- The acute angle of a right triangle is congruent to the acute angle of another right triangle.
- The two triangles are congruent. Note here that congruency implies similarity.
Chapter 12. Quadrilaterals
[edit | edit source]A quadrilateral is a polygon that has four sides.
Special Types of Quadrilaterals
[edit | edit source]- Parallelogram
- A parallelogram is a quadrilateral having two pairs of parallel sides.
- The two opposite sides are equal.
- Opposite angles are equal.
- Rhombus
- A rhombus is a quadrilateral of which all four sides are the same length.
- Rectangle
- A rectangle is a parallelogram of which all four angles are 90 degrees.
- Square
- A square is a quadrilateral of which all four sides are of the same length, and all four angles are 90 degrees.
- Trapezoid
- A trapezoid is a quadrilateral which has two parallel sides (U.S.)
- Trapezium
- U.S. usage: A trapezium is a quadrilateral which has no parallel sides.
- U.K usage: A trapezium is a quadrilateral with two parallel sides (same as US trapezoid definition).
- Kite
- A kite is an quadrilateral with two pairs of congruent adjacent sides.
One of the most important properties used in proofs is that the sum of the angles of the quadrilateral is always 360 degrees. This can easily be proven too:
If you draw a random quadrilateral, and one of its diagonals, you'll split it up into two triangles. Given that the sum of the angles of a triangle is 180 degrees, you can sum them up, and it'll give 360 degrees.
Chapter 13. Parallelograms
[edit | edit source]Parallelograms
[edit | edit source]A parallelogram is a geometric figure with two
pairs of parallel sides. Parallelograms are a special type of quadrilateral.
The opposite sides are equal in length and the opposite angles are also equal.
The area is equal to the product of any side and the distance between that
side and the line containing the opposite side.

Properties of Parallelograms
[edit | edit source]The following properties are common to all parallelograms (parallelogram, rhombus, rectangle, square)
- both pairs of opposite sides are parallel
- both pairs of opposite sides are congruent
- both pairs of opposite angles are congruent
- the diagonals bisect each other
- consecutive angles are supplementary
Special Parallelograms
[edit | edit source]Rhombus
[edit | edit source]- A rhombus is a parallelogram with four congruent sides.
- The diagonals of a rhombus are perpendicular.
- Each diagonal of a rhombus bisects two angles the rhombus.
- A rhombus may or may not be a square.
Square
[edit | edit source]- A square is a parallelogram with four right angles and four congruent sides.
- A square is both a rectangle and a rhombus and inherits all of their properties.
Chapter 14. Trapezoids
[edit | edit source]A Trapezoid (American English) or Trapezium (British English) is a quadrilateral that has two parallel sides and two non parallel sides.
Some properties of trapezoids:
- The interior angles sum to 360° as in any quadrilateral.
- The parallel sides are unequal.
- Each of the parallel sides is called a base (b) of the trapezoid. The two angles that join one base are called 'base angles'.
- If the two non-parallel sides are equal, the trapezoid is called an isosceles trapezoid.
- In an isosceles trapezoid, each pair of base angles are equal.
- If one pair of base angles of a trapezoid are equal, the trapezoid is isosceles.
- A line segment connecting the midpoints of the non-parallel sides is called the median (m) of the trapeziod.
- The median of a trapezoid is equal to one half the sum of the bases (called b1 and b2).
- A line segment perpendicular to the bases is called an altitude (h) of the trapezoid.
The area (A) of a trapezoid is equal to the product of an altitude and the median.
Recall though that the median is half of the sum of the bases.
Substituting for m, we get:
Chapter 15. Circles/Radii, Chords and Diameters
[edit | edit source]A circle is a set of all points in a plane that are equidistant from a single point; that single point is called the centre of the circle and the distance between any point on circle and the centre is called radius of the circle.
http://en.wikipedia.org/upload/d/dd/Circle-2.png
Chord
a chord is an internal segment of a circle that has both of its endpoints on the circumference of the circle.
- the diameter of a circle is the largest chord possible
Secant
a secant of a circle is any line that intersects a circle in two places.
- a secant contains any chord of the circle
Tangent
a tangent to a circle is a line that intersects a circle in exactly one point, called the point of tangency.
- at the point of tangency the tangent line and the radius of the circle are perpendicular
Chapter 16. Circles/Arcs
[edit | edit source]An arc is a segment of the perimeter of a given circle. The measure of an arc is measured as an angle, this could be in radians or degrees (more on radians later). The exact measure of the arc is determined by the measure of the angle formed when a line is drawn from the center of the circle to each end point. As an example the circle below has an arc cut out of it with a measure of 30 degrees.
Radians:
An arc can be measured in degrees or radians. A radian is merely a different method for measuring an angle. If we take a unit circle (which has a radius of 1 unit), then if we take an arc with the length equal to 1 unit, and draw line from each endpoint to the center of the circle the angle formed is equal to 1 radian. this concept is displayed below, in this circle an arc has been cut off by an angle of 1 radian, and therefore the length of the arc is equal to because the radius is 1.
From this definition we can say that on the unit circle a single radian is equal to radians because the perimeter of a unit circle is equal to . Another useful property of this definition that will be extremely useful to anyone who studies arcs is that the length of an arc is equal to its measure in radians multiplied by the radius of the circle.
Converting to and from radians is a fairly simple process. 2 facts are required to do so, first a circle is equal to 360 degrees, and it is also equal to . using these 2 facts we can form the following formula:
, thus 1 degree is equal to radians.
From here we can simply multiply by the number of degrees to convert to radians. for example if we have 20 degrees and want to convert to radians then we proceed as follows:
radians.
The same sort of argument can be used to show the formula for getting 1 radian.
, thus 1 radian is equal to
Chapter 17. Circles/Tangents and Secants
[edit | edit source]A tangent is a line in the same plane as a given circle that meets that circle in exactly one point. That point is called the point of tangency. A tangent cannot pass through a circle; if it does, it is classified as a chord. A secant is a line containing a chord.

A common tangent is a line tangent to two circles in the same plane. If the tangent does not intersect the line containing and connecting the centers of the circles, it is an external tangent. If it does, it is an internal tangent.
Two circles are tangent to one another if in a plane they intersect the same tangent in the same point.

Chapter 18. Circles/Sectors
[edit | edit source]Sector of a circle
[edit | edit source]A sector of a circle can be thought of as a pie piece. In the picture below, a sector of the circle is shaded yellow.
To find the area of a sector, find the area of the whole circle and then multiply by the angle of the sector over 360 degrees.
A more intuitive approach can be used when the sector is half the circle. In this case the area of the sector would just be the area of the circle divided by 2.
Appendix A. Postulates & Definitions
[edit | edit source]A
[edit | edit source]Acute Angle
[edit | edit source]- See Angle
Algebraic Properties of Equality
[edit | edit source]For real numbers a, b, and c
Addition Property of Equality: if a=b , then a+c=b+c
Subtraction Property of Equality: if a=b , then a-c+=b-c
Multiplication Property of Equality: is a=b, then ac=bc
Division Property of Equality: if a=b and c≠0 , then (a/c)=(b/c)
Symmetric Property of Equality: if a=b, then b=a
Transitive Property of Equality: if a=b and b=c , then a=c
Reflexive Property of Equality: if a=a , then a=a
Substitution Property of Equality: if a=b, then a can be substituted for b
Distributive Property of Equality: a(b+c)=ab+ac
Angle
[edit | edit source]
A figure is an angle if and only if it is composed of two rays which share a common endpoint. Each of these rays (or segments, as the case may be) is known as a side of the angle (For example, in the illustration at right), and the common point is known as the angle's vertex (point B in the illustration). Angles are measured by the difference of their slopes. The units for angle measure are radians and degrees. Angles may be classified by their degree measure.
- Acute Angle: an angle is an acute angle if and only if it has a measure of less than 90°
- Right Angle: an angle is an right angle if and only if it has a measure of exactly 90°
- Obtuse Angle: an angle is an obtuse angle if and only if it has a measure of greater than 90°
- Straight Angle: an angle is a straight angle if and only if it has a measure of exactly 180°
Using the Right Angle Congruence Theorem and the Straight Angle Congruence Theorem, all right angles and all straight angles are congruent
Angle Addition Postulate
[edit | edit source]If P is in the interior of an angle , then
Arithmetic mean
[edit | edit source]The arithmetic mean of 2 numbers a and b can be calculated as: arithmetic mean=(a+b)/2
B
[edit | edit source]Bisector
[edit | edit source]A figure bisects another figure if and only if it splits the figure it intersects into two equal parts
C
[edit | edit source]Center of a circle
[edit | edit source]Point P is the center of circle C if and only if all points in circle C are equidistant from point P and point P is contained in the same plane as circle C.
Circle
[edit | edit source]The set of all points in a plane that are equidistant from a given point (called the center of the circle).
Circumference
[edit | edit source]The distance around a circle.
It is calculated as:
C=2πr (where r is the radius of the circle)
Complementary Angles
[edit | edit source]Two angles are complimentary if and only if the sum of their measures equals up to 90 degrees.
Concave
[edit | edit source]A polygon is said to be concave if and only if it contains at least one interior angle with a measure greater than 180° exclusively and less than 360° exclusively.
Congruency
[edit | edit source]Two figures are congruent if and only if they have the same measure. It is designated by "≅".
Corresponding angles
[edit | edit source]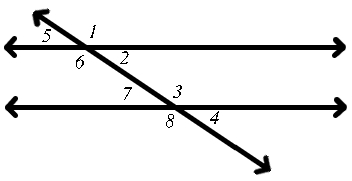
Two angles formed by a transversal intersecting with two lines are corresponding angles if and only if one is on the inside of the two lines, the other is on the outside of the two lines, and both are on the same side of the transversal.
Corresponding Angles Postulate
[edit | edit source]If two lines cut by a transversal are parallel, then their corresponding angles are congruent.
Corresponding Parts of Congruent Triangles are Congruent Postulate
[edit | edit source]The Corresponding Parts of Congruent Triangles are Congruent Postulate (CPCTC) states:
- If ∆ABC ≅ ∆XYZ, then all parts of ∆ABC are congruent to their corresponding parts in ∆XYZ. For example:
- ≅
- ≅
- ≅
- ∠ABC ≅ ∠XYZ
- ∠BCA ≅ ∠YZX
- ∠CAB ≅ ∠ZXY
CPCTC also applies to all other parts of the triangles, such as a triangle's altitude, median, circumcenter, et al.
Cosine
[edit | edit source]A trigonometric ratio, abbreviated as cos.
cos(θ)=adjacent/hypotenuse
D
[edit | edit source]Diameter
[edit | edit source]A line segment is the diameter of a circle if and only if it is a chord of the circle which contains the circle's center.
- See Circle
Distance
[edit | edit source]Distance between 2 points can be calculated as the absolute value of the difference of the coordinates of the points.
In a coordinate plane, distance for points A(x1,y1) and B(x2,y2) can be calculated as:
d(AB)=√((y2-y1)2+(x2-x1)2)
The distance between a point and a line is measured by the perpendicular segments connecting the 2 (using the Perpendicular postulate).
G
[edit | edit source]Geometric Mean
[edit | edit source]For 2 positive numbers a and b, the geometric mean of a and b is the positive number x that satisfies (a/x)=(x/b). So, x2=√(ab)
geometric mean of a and b = (a/x)=(x/b) : x2=√(ab)
H
[edit | edit source]Height of a paralellogram
[edit | edit source]The perpendicular distance bewtween 2 bases of a paralellogram
Hypotenuse
[edit | edit source]In a right triangle, the side opposite of the right angle.
Using the Pythagorean Theorem, the measure of the hypotenuse can be calculated as:
c2=a2=b2 (where c is the hypotenuse and a and b are legs of the right triangle)
L
[edit | edit source]Line
[edit | edit source]A collection of points is a line if and only if the collection of points is perfectly straight (aligned), is infinitely long, and is infinitely thin. Between any two points on a line, there exists an infinite number of points which are also contained by the line. Lines are usually written by two points in the line, such as line AB, or
Line segment
[edit | edit source]
A collection of points is a line segment if and only if it is perfectly straight, is infinitely thin, and has a finite length. A line segment is measured by the shortest distance between the two extreme points on the line segment, known as endpoints. Between any two points on a line segment, there exists an infinite number of points which are also contained by the line segment.
I
[edit | edit source]Interior angle of a regular polygon
[edit | edit source]The interior angles of a regular polygon are all congruent. As such, the measure of one interior angle of a regular polygon with n number of sides can be calculated as:
int angle = ((n-2)180)/n
Isosceles Trapezoid
[edit | edit source]A trapezoid in which the legs are congruent.
Isosceles Triangle
[edit | edit source]A triangle with at least 2 congruent sides.
Using the Base Angles theorem, the angles opposite of the congruent sides are also congruent.
L
[edit | edit source]Linear pair
[edit | edit source]The adjacent angles whose noncommon sides are opposite rays.
Using the Linear Pair Postulate, the angles in a linear pair are also supplementary.
If 2 angles forming a linear pair are congruent, then both the angles are right angles and the lines containing the angles are perpendicular.
M
[edit | edit source]Major arc
[edit | edit source]An arc whose measure is greater than 180 degrees. It must be named by 3 points.
Midsegment of a Trapezoid
[edit | edit source]A segment that connects the midpoints of the legs of a trapezoid.
It is parallel to the bases and the length of it is the arithmetic mean of the measures of the bases.
Minor arc
[edit | edit source]An arc whose measure is less than 180 degrees.
P
[edit | edit source]Parallel lines
[edit | edit source]Two lines or line segments are said to be parallel if and only if the lines are contained by the same plane and have no points in common if continued infinitely.
In a coordinate plane, two lines are parallel if and only if they share the same slope.
Parallel planes
[edit | edit source]Two planes are said to be parallel if and only if the planes have no points in common when continued infinitely.
Perpendicular lines
[edit | edit source]Two lines that intersect at a 90° angle.
In a coordinate plane, two lines are perpendicular if and only if their slopes' products are equal to -1 (or if the slopes are negative reciprocals).
Perpendicular Postulate
[edit | edit source]Given a line, and a point P not in line , then there is one and only one line that goes through point P perpendicular to
Plane
[edit | edit source]An object is a plane if and only if it is a two-dimensional object which has no thickness or curvature and continues infinitely. A plane can be defined by three points. A plane may be considered to be analogous to a piece of paper[1].
Point
[edit | edit source]A point is a zero-dimensional mathematical object representing a location in one or more dimensions[2]. A point has no size; it has only location.
Polygon
[edit | edit source]A polygon is a closed plane figure composed of at least 3 straight lines. Each side has to intersect another side at their respective endpoints, and that the lines intersecting are not collinear.
R
[edit | edit source]Radius
[edit | edit source]The radius of a circle is the distance between any given point on the circle and the circle's center.
All radii in the same circle (or congruent circles) have the same measure.
- See Circle
Ray
[edit | edit source]A ray is a straight collection of points which continues infinitely in one direction. The point at which the ray stops is known as the ray's endpoint. Between any two points on a ray, there exists an infinite number of points which are also contained by the ray.
Regular Polygon
[edit | edit source]A regular polygon is a polygon that is equilateral and equiangular.
Ruler Postulate
[edit | edit source]The points on a line can be matched one to one with the real numbers. The real number that corresponds to a point is the point's coordinate. The distance between two points is the absolute value of the difference between the two coordinates of the two points.
S
[edit | edit source]Semicircle
[edit | edit source]An arc whose measure is 180 degrees.
Sum of the interior angles of a polygon
[edit | edit source]The sum of the interior angles of a polygon with n number of sides is calculated as:
sum int angles = (n-2)180
Supplementary Angles
[edit | edit source]Two angles are supplementary if and only if the sum of their measures equal up to 180 degrees.
- Part II- Coordinate Geometry:
Synthetic versus analytic geometry
[edit | edit source]Geometry/Synthetic versus analytic geometry
- Two and Three-Dimensional Geometry and Other Geometric Figures
Perimeter and Arclength
[edit | edit source]Perimeter of Circle
[edit | edit source]The circles perimeter can be calculated using the following formula
where the radius of the circle.
Perimeter of Polygons
[edit | edit source]The perimeter of a polygon with number of sides abbreviated can be calculated using the following formula
- .
Arclength of Circles
[edit | edit source]The arclength of a given circle with radius can be calculated using
where is the angle given in radians.
Arclength of Curves
[edit | edit source]If a curve in has the parametric form for , then the arclength can be calculated using the following fomula
Derivation of formula can be found using differential geometry on infinitely small triangles.
Area
[edit | edit source]Area of Circles
[edit | edit source]The method for finding the area of a circle is
Where is the radius of the circle; a line drawn from any point on the circle to its center.
Area of triangles
[edit | edit source]Three ways of calculating the area inside of a Triangle are mentioned here.
First method
[edit | edit source]If one of the sides of the triangle is chosen as a base, then a height for the triangle and that particular base can be defined. The height is a line segment perpendicular to the base or the line formed by extending the base and the endpoints of the height are the corner point not on the base and a point on the base or line extending the base. Let B = the length of the side chosen as the base. Let
h = the distance between the endpoints of the height segment which is perpendicular to the base. Then the area of the triangle is given by:
This method of calculating the area is good if the value of a base and its corresponding height in the triangle is easily determined. This is particularly true if the triangle is a right triangle, and the lengths of the two sides sharing the angle can be determined.
Second method
[edit | edit source], also known as Heron's Formula
If the lengths of all three sides of a triangle are known, Hero's formula may be used to calculate the area of the triangle. First, the semiperimeter, s, must be calculated by dividing the sum of the lengths of all three sides by 2. For a triangle having side lengths :
Then the triangle's area is given by:
If the triangle is needle shaped, that is, one of the sides is very much shorter than the other two then it can be difficult to compute the area because the precision needed is greater than that available in the calculator or computer that is used. In otherwords Heron's formula is numerically unstable. Another formula that is much more stable is:
where have been sorted so that .
See also Heron's Formula at MathWorld and How JAVA's Floating-Point Hurts Everyone Everywhere
Third method
[edit | edit source]In a triangle with sides length and angles opposite them,
This formula is true because in the formula . It is useful because you don't need to find the height from an angle in a separate step, and is also used to prove the law of sines (divide all terms in the above equation by and you'll get it directly!)
Area of rectangles
[edit | edit source]The area calculation of a rectangle is simple and easy to understand. One of the sides is chosen as the base, with a length . An adjacent side is then the height, with a length , because in a rectangle the adjacent sides are perpendicular to the side chosen as the base. The rectangle's area is given by:
Sometimes, the baselength may be referred to as the length of the rectangle, l, and the height as the width of the rectangle, w. Then the area formula becomes:
Regardless of the labels used for the sides, it is apparent that the two formulas are equivalent.
Of course, the area of a square with sides having length would be:
Area of parallelograms
[edit | edit source]Parallelograms are described in their own chapter.
The area of a parallelogram can be determined using the equation for the area of a rectangle. The formula is:
- is the area of a parallelogram.
- is the base.
- is the height.
The height is a perpendicular line segment that connects one of the vertices to its opposite side (the base).
Area of a rhombus
[edit | edit source]Remember in a rombus all sides are equal in length.
where represent the diagonals.
Area of trapezoids
[edit | edit source]The area of a trapezoid is derived from taking the arithmetic mean of its two parallel sides to form a rectangle of equal area. Where are the lengths of the two parallel bases.
Area of kites
[edit | edit source]
The area of a kite is based on splitting the kite into four pieces by halving it along each diagonal and using these pieces to form a rectangle of equal area.
Where are the diagonals of the kite.
Alternatively, the kite may be divided into two halves, each of which is a triangle, by the longer of its diagonals, . The area of each triangle is thus
Where is the other (shorter) diagonal of the kite.
And the total area of the kite (which is composed of two identical such triangles) is
For more details see w:Kite (geometry)#Properties.
Areas of other quadrilaterals
[edit | edit source]The areas of other Quadrilaterals are slightly more complex to calculate, but can still be found if the quadrilateral is well-defined. For example, a quadrilateral can be divided into two triangles, or some combination of triangles and rectangles. The areas of the constituent polygons can be found and added up with arithmetic.
Areas defining angles
[edit | edit source]The area of a circular sector is a fraction of the area of the whole circle. When the circle has radius that is the square root of two, the circle has area 2 π, and the radian measure of the sector corresponds to the fraction of the total circular area. In calculus, another type of angle called hyperbolic is related to the exponential function. This type of angle also corresponds to the area of a sector of the hyperbola xy=1. Use of area measure provides a means to unify these angle types. See Unified Angles.
Volume
[edit | edit source]Volume
[edit | edit source]Volume is like area expanded out into 3 dimensions. Area deals with only 2 dimensions. For volume we have to consider another dimension. Area can be thought of as how much space some drawing takes up on a flat piece of paper. Volume can be thought of as how much space an object takes up.
Volume formulae
[edit | edit source]| Common equations for volume: | ||
|---|---|---|
| Shape | Equation | Variables |
| A cube: | s = length of a side | |
| A rectangular prism: | l = length, w = width, h = height | |
| A cylinder (circular prism): | r = radius of circular face, h = height | |
| Any prism that has a constant cross sectional area along the height: | A = area of the base, h = height | |
| A sphere: | r = radius of sphere which is the integral of the Surface Area of a sphere | |
| An ellipsoid: | a, b, c = semi-axes of ellipsoid | |
| A pyramid: | A = area of the base, h = height of pyramid | |
| A cone (circular-based pyramid): | r = radius of circle at base, h = distance from base to tip | |
(The units of volume depend on the units of length - if the lengths are in meters, the volume will be in cubic meters, etc.)
Pappus' Theorem
[edit | edit source]The volume of any solid whose cross sectional areas are all the same is equal to that cross sectional area times the distance the centroid(the center of gravity in a physical object) would travel through the solid.
Cavalieri's Principle
[edit | edit source]If two solids are contained between two parallel planes and every plane parallel to these two plane has equal cross sections through these two solids, then their volumes are equal.
Polygons
[edit | edit source]A Polygon is a two-dimensional figure, meaning all of the lines in the figure are contained within one plane. They are classified by the number of angles, which is also the number of sides.
One key point to note is that a polygon must have at least three sides. Normally, three to ten sided figures are referred to by their names (below), while figures with eleven or more sides is an n-gon, where n is the number of sides. Hence a forty-sided polygon is called a 40-gon.
A polygon with three angles and sides.
A polygon with four angles and sides.
- Pentagon
A polygon with five angles and sides.
- Hexagon
A polygon with six angles and sides.
- Heptagon
A polygon with seven angles and sides.
- Octagon
A polygon with eight angles and sides.
- Nonagon
A polygon with nine angles and sides.
- Decagon
A polygon with ten angles and sides.
For a list of n-gon names, go to [3] and scroll to the bottom of the page.
Polygons are also classified as convex or concave. A convex polygon has interior angles less than 180 degrees, thus all triangles are convex. If a polygon has at least one internal angle greater than 180 degrees, then it is concave. An easy way to tell if a polygon is concave is if one side can be extended and crosses the interior of the polygon. Concave polygons can be divided into several convex polygons by drawing diagonals. Regular polygons are polygons in which all sides and angles are congruent.
Triangles
[edit | edit source]A triangle is a type of polygon having three sides and three angles. The triangle is a closed figure formed from three straightline segments joined at their ends. The Line Segments at the ends can be called the corners, angles, or vertices of the triangle. Since any given triangle lies completely within a plane, triangles are often treated as two-dimensional geometric figures. As such, a triangle has no volume and, because it is a two-dimensionally closed figure, the flat part of the plane inside the triangle has an area, typically referred to as the area of the triangle. A triangle must have at least some area, so all three corner points of a triangle cannot lie in the same line. The sum of the lengths of any two sides of a triangle is always greater than the length of the third side. The preceding statement is sometimes called the Triangle Inequality.
Certain types of triangles
[edit | edit source]Categorized by angle
[edit | edit source]The sum of the interior angles in a triangle always equals 180o. This means that no more than one of the angles can be 90o or more. All three angles can all be less than 90oin the triangle; then it is called an acute triangle. One of the angles can be 90o and the other two less than 90o; then the triangle is called a right triangle. Finally, one of the angles can be more than 90o and the other two less; then the triangle is called an obtuse triangle.
Categorized by sides
[edit | edit source]If all three of the sides of a triangle are of different length, then the triangle is called a scalene triangle. If two of the sides of a triangle are of equal length, then it is called an isosceles triangle. In an isosceles triangle, the angle between the two equal sides can be more than, equal to, or less than 90o. The other two angles are both less than 90o. If all three sides of a triangle are of equal length, then it is called an equilateral triangle and all three of the interior angles must be 60o, making it equiangular. Because the interior angles are all equal, all equilateral triangles are also the three-sided variety of a regular polygon and they are all similar, but might not be congruent. However, polygons having four or more equal sides might not have equal interior angles, might not be regular polygons, and might not be similar or congruent. Of course, pairs of triangles which are not equilateral might be similar or congruent. Further discussion of Congruent Triangles and Similar Triangles may be found in those corresponding sections.
Opposite corners and sides in triangles
[edit | edit source]If one of the sides of a triangle is chosen, the interior angles of the corners at the side's endpoints can be called adjacent angles. The corner which is not one of these endpoints can be called the corner opposite to the side. The interior angle whose vertex is the opposite corner can be called the angle opposite to the side. Likewise, if a corner or its angle is chosen, then the two sides sharing an endpoint at that corner can be called adjacent sides. The side not having this corner as one of its two endpoints can be called the side opposite to the corner. The sides or their lengths of a triangle are typicaly labeled with lower case letters. The corners or their corresponding angles can be labeled with capital letters. The triangle as a whole can be labeled by a small triangle symbol and its corner points. In a triangle, the largest interior angle is opposite to longest side, and vice versa. Any triangle can be divided into two right triangles by taking the longest side as a base, and extending a line segment from the opposite corner to a point on the base such that it is perpendicular to the base. Such a line segment would be considered the height or altitude ( h ) for that particular base ( b ). The two right triangles resulting from this division would both share the height as one of its sides. The interior angles at the meeting of the height and base would be 90o for each new right triangle. For acute triangles, any of the three sides can act as the base and have a corresponding height. For more information on right triangles, see Right Triangles and Pythagorean Theorem.
Area of Triangles
[edit | edit source]
If base and height of a triangle are known, then the area of the triangle can be calculated by the formula:
( is the symbol for area)
Ways of calculating the area inside of a triangle are further discussed under Area.
Centres
[edit | edit source]The centroid is constructed by drawing all the medians of the triangle. All three medians intersect at the same point: this crossing point is the centroid. Centroids are always inside a triangle. They are also the centre of gravity of the triangle. The three angle bisectors of the triangle intersect at a single point, called the incentre. Incentres are always inside the triangle. The three sides are equidistant from the incentre. The incentre is also the centre of the inscribed circle (incircle) of a triangle, or the interior circle which touches all three sides of the triangle. The circumcentre is the intersection of all three perpendicular bisectors. Unlike the incentre, it is outside the triangle if the triangle is obtuse. Acute triangles always have circumcentres inside, while the circumcentre of a right triangle is the midpoint of the hypotenuse. The vertices of the triangle are equidistant from the circumcentre. The circumcentre is so called because it is the centre of the circumcircle, or the exterior circle which touches all three vertices of the triangle. The orthocentre is the crossing point of the three altitudes. It is always inside acute triangles, outside obtuse triangles, and on the right vertex of the right-angled triangle. Please note that the centres of an equilateral triangle are always the same point.
Right Triangles and Pythagorean Theorem
[edit | edit source]Right triangles
[edit | edit source]Right triangles are triangles in which one of the interior angles is 90o. A 90o angle is called a right angle.
Right triangles have special properties which make it easier to conceptualize and calculate their parameters in many cases.
The side opposite of the right angle is called the hypotenuse. The sides adjacent to the right angle are the legs. When using the Pythagorean Theorem, the hypotenuse or its length is often labeled with a lower case c. The legs (or their lengths) are often labeled a and b.
Either of the legs can be considered a base and the other leg would be considered the height (or altitude), because the right angle automatically makes them perpendicular. If the lengths of both the legs are known, then by setting one of these sides as the base ( b ) and the other as the height ( h ), the area of the right triangle is very easy to calculate using this formula:
This is intuitively logical because another congruent right triangle can be placed against it so that the hypotenuses are the same line segment, forming a rectangle with sides having length b and width h. The area of the rectangle is b × h, so either one of the congruent right triangles forming it has an area equal to half of that rectangle.
Right triangles can be neither equilateral, acute, nor obtuse triangles. Isosceles right triangles have two 45° angles as well as the 90° angle. All isosceles right triangles are similar since corresponding angles in isosceles right triangles are equal. If another triangle can be divided into two right triangles (see Triangle), then the area of the triangle may be able to be determined from the sum of the two constituent right triangles. Also the Pythagorean theorem can be used for non right triangles. a2+b2=c2-2c
Pythagorean Theorem
[edit | edit source]For history regarding the Pythagorean Theorem, see Pythagorean theorem. The Pythagorean Theorem states that:
- In a right triangle, the square of the length of the hypotenuse is equal to the sum of the squares of the lengths of the other two sides.
Let's take a right triangle as shown here and set c equal to the length of the hypotenuse and set a and b each equal to the lengths of the other two sides. Then the Pythagorean Theorem can be stated as this equation:
Using the Pythagorean Theorem, if the lengths of any two of the sides of a right triangle are known and it is known which side is the hypotenuse, then the length of the third side can be determined from the formula.
Sine, Cosine, and Tangent for Right Triangles
[edit | edit source]Sine, Cosine, and Tangent are all functions of an angle, which are useful in right triangle calculations. For an angle designated as θ, the sine function is abbreviated as sin θ, the cosine function is abbreviated as cos θ, and the tangent function is abbreviated as tan θ. For any
angle θ, sin θ, cos θ, and tan θ are each single determined values and if θ is a known value, sin θ, cos θ, and tan θ can be looked up in a table or found with a calculator. There is a table listing these function values at the end of this section. For an angle between listed values, the sine, cosine, or tangent of that angle can be estimated from the values in the table. Conversely, if a number is known to be the sine, cosine, or tangent of an angle, then such tables could be used in reverse to find (or estimate) the value of a corresponding angle.
These three functions are related to right triangles in the following ways:
In a right triangle,
- the sine of a non-right angle equals the length of the leg opposite that angle divided by the length of the hypotenuse.
- the cosine of a non-right angle equals the length of the leg adjacent to it divided by the length of the hypotenuse.
- the tangent of a non-right angle equals the length of the leg opposite that angle divided by the length of the leg adjacent to it.
For any value of θ where cos θ ≠ 0,
If one considers the diagram representing a right triangle with the two non-right angles θ1and θ2, and the side lengths a,b,c as shown here:
For the functions of angle θ1:
Analogously, for the functions of angle θ2:
Table of sine, cosine, and tangent for angles θ from 0 to 90°
[edit | edit source]| θ in degrees | θ in radians | sin θ | cos θ | tan θ |
|---|---|---|---|---|
| 0 | 0 | 0.0 | 1.0 | 0.0 |
| 1 | 0.017453293 | 0.01745240 | 0.9998477 | 0.017455065 |
| 2 | 0.034906585 | 0.034899497 | 0.99939083 | 0.034920769 |
| 3 | 0.052359878 | 0.052335956 | 0.99862953 | 0.052407779 |
| 4 | 0.06981317 | 0.069756474 | 0.99756405 | 0.069926812 |
| 5 | 0.087266463 | 0.087155743 | 0.9961947 | 0.087488664 |
| 6 | 0.10471976 | 0.10452846 | 0.9945219 | 0.10510424 |
| 7 | 0.12217305 | 0.12186934 | 0.99254615 | 0.12278456 |
| 8 | 0.13962634 | 0.1391731 | 0.99026807 | 0.14054083 |
| 9 | 0.15707963 | 0.15643447 | 0.98768834 | 0.15838444 |
| 10 | 0.17453293 | 0.17364818 | 0.98480775 | 0.17632698 |
| 11 | 0.19198622 | 0.190809 | 0.98162718 | 0.19438031 |
| 12 | 0.20943951 | 0.20791169 | 0.9781476 | 0.21255656 |
| 13 | 0.2268928 | 0.22495105 | 0.97437006 | 0.23086819 |
| 14 | 0.2443461 | 0.2419219 | 0.97029573 | 0.249328 |
| 15 | 0.26179939 | 0.25881905 | 0.96592583 | 0.26794919 |
| 16 | 0.27925268 | 0.27563736 | 0.9612617 | 0.28674539 |
| 17 | 0.29670597 | 0.2923717 | 0.95630476 | 0.30573068 |
| 18 | 0.31415927 | 0.30901699 | 0.95105652 | 0.3249197 |
| 19 | 0.33161256 | 0.32556815 | 0.94551858 | 0.34432761 |
| 20 | 0.34906585 | 0.34202014 | 0.93969262 | 0.36397023 |
| 21 | 0.36651914 | 0.35836795 | 0.93358043 | 0.38386404 |
| 22 | 0.38397244 | 0.37460659 | 0.92718385 | 0.40402623 |
| 23 | 0.40142573 | 0.39073113 | 0.92050485 | 0.42447482 |
| 24 | 0.41887902 | 0.40673664 | 0.91354546 | 0.44522869 |
| 25 | 0.43633231 | 0.42261826 | 0.90630779 | 0.46630766 |
| 26 | 0.45378561 | 0.43837115 | 0.89879405 | 0.48773259 |
| 27 | 0.4712389 | 0.4539905 | 0.89100652 | 0.50952545 |
| 28 | 0.48869219 | 0.46947156 | 0.88294759 | 0.53170943 |
| 29 | 0.50614548 | 0.48480962 | 0.87461971 | 0.55430905 |
| 30 | 0.52359878 | 0.5 | 0.8660254 | 0.57735027 |
| 31 | 0.54105207 | 0.51503807 | 0.8571673 | 0.60086062 |
| 32 | 0.55850536 | 0.52991926 | 0.8480481 | 0.62486935 |
| 33 | 0.57595865 | 0.54463904 | 0.83867057 | 0.64940759 |
| 34 | 0.59341195 | 0.5591929 | 0.82903757 | 0.67450852 |
| 35 | 0.61086524 | 0.57357644 | 0.81915204 | 0.70020754 |
| 36 | 0.62831853 | 0.58778525 | 0.80901699 | 0.72654253 |
| 37 | 0.64577182 | 0.60181502 | 0.79863551 | 0.75355405 |
| 38 | 0.66322512 | 0.61566148 | 0.78801075 | 0.78128563 |
| 39 | 0.68067841 | 0.62932039 | 0.77714596 | 0.80978403 |
| 40 | 0.6981317 | 0.64278761 | 0.76604444 | 0.83909963 |
| 41 | 0.71558499 | 0.65605903 | 0.75470958 | 0.86928674 |
| 42 | 0.73303829 | 0.66913061 | 0.74314483 | 0.90040404 |
| 43 | 0.75049158 | 0.68199836 | 0.7313537 | 0.93251509 |
| 44 | 0.76794487 | 0.69465837 | 0.7193398 | 0.96568877 |
| 45 | 0.78539816 | 0.70710678 | 0.70710678 | 1.0 |
| 46 | 0.80285146 | 0.7193398 | 0.69465837 | 1.03553031 |
| 47 | 0.82030475 | 0.7313537 | 0.68199836 | 1.07236871 |
| 48 | 0.83775804 | 0.74314483 | 0.66913061 | 1.11061251 |
| 49 | 0.85521133 | 0.75470958 | 0.65605903 | 1.15036841 |
| 50 | 0.87266463 | 0.76604444 | 0.64278761 | 1.19175359 |
| 51 | 0.89011792 | 0.77714596 | 0.62932039 | 1.23489716 |
| 52 | 0.90757121 | 0.78801075 | 0.61566148 | 1.27994163 |
| 53 | 0.9250245 | 0.79863551 | 0.60181502 | 1.32704482 |
| 54 | 0.9424778 | 0.80901699 | 0.58778525 | 1.37638192 |
| 55 | 0.95993109 | 0.81915204 | 0.57357644 | 1.42814801 |
| 56 | 0.97738438 | 0.82903757 | 0.5591929 | 1.48256097 |
| 57 | 0.99483767 | 0.82367057 | 0.54463904 | 1.53986496 |
| 58 | 1.01229097 | 0.8480481 | 0.52991926 | 1.60033453 |
| 59 | 1.02974426 | 0.8571673 | 0.51503807 | 1.66427948 |
| 60 | 1.04719755 | 0.8660254 | 0.5 | 1.73205081 |
| 61 | 1.06465084 | 0.87461971 | 0.48480962 | 1.80404776 |
| 62 | 1.08210414 | 0.88294759 | 0.46947156 | 1.88072647 |
| 63 | 1.09955743 | 0.89100652 | 0.4539905 | 1.96261051 |
| 64 | 1.11701072 | 0.89879405 | 0.43837115 | 2.05030384 |
| 65 | 1.13446401 | 0.90630779 | 0.42261826 | 2.14450692 |
| 66 | 1.15191731 | 0.91354546 | 0.40673664 | 2.24603677 |
| 67 | 1.1693706 | 0.92050485 | 0.39073113 | 2.35585237 |
| 68 | 1.18682389 | 0.92718385 | 0.37460659 | 2.47508685 |
| 69 | 1.20427718 | 0.93358043 | 0.35836795 | 2.60508906 |
| 70 | 1.22173048 | 0.93969262 | 0.34202014 | 2.74747742 |
| 71 | 1.23918377 | 0.94551858 | 0.32556815 | 2.90421088 |
| 72 | 1.25663706 | 0.95105652 | 0.30901699 | 3.07768354 |
| 73 | 1.27409035 | 0.95630476 | 0.2923717 | 3.27085262 |
| 74 | 1.29154365 | 0.9612617 | 0.27563736 | 3.48741444 |
| 75 | 1.30899694 | 0.96592583 | 0.25881905 | 3.73205081 |
| 76 | 1.32645023 | 0.97029573 | 0.2419219 | 4.01078093 |
| 77 | 1.34390352 | 0.97437006 | 0.22495105 | 4.33147587 |
| 78 | 1.36135682 | 0.9781476 | 0.20791169 | 4.70463011 |
| 79 | 1.37881011 | 0.98162718 | 0.190809 | 5.14455402 |
| 80 | 1.3962634 | 0.98480775 | 0.17364818 | 5.67128182 |
| 81 | 1.41371669 | 0.98768834 | 0.15643447 | 6.31375151 |
| 82 | 1.43116999 | 0.99026807 | 0.1391731 | 7.11536972 |
| 83 | 1.44862328 | 0.99254615 | 0.12186934 | 8.14434643 |
| 84 | 1.46607657 | 0.9945219 | 0.10452846 | 9.51436445 |
| 85 | 1.48352986 | 0.9961947 | 0.087155743 | 11.4300523 |
| 86 | 1.50098316 | 0.99756405 | 0.069756474 | 14.3006663 |
| 87 | 1.51843645 | 0.99862953 | 0.052335956 | 19.0811367 |
| 88 | 1.53588974 | 0.99939083 | 0.034899497 | 28.6362533 |
| 89 | 1.55334303 | 0.9998477 | 0.01745240 | 57.2899616 |
| 90 | 1.57079633 | 1.0 | 0.0 | not defined |
General rules for important angles:
Polyominoes
[edit | edit source]A polyomino is a plane geometric figure formed by joining one or more equal squares edge to edge.
Domino
[edit | edit source]
A domino is the shape made from attaching unit squares so that they share one full edge. The term polyomino is based on the word domino. There is only one possible domino.
Tromino
[edit | edit source]A polymino made from three squares is called a tromino. There are only two possible trominoes.
Tetromino
[edit | edit source]A polymino made from four squares is called a tetromino. There are five possible combinations and two reflections:
Pentominoes
[edit | edit source]A polymino made from five squares is called a pentomino. There are twelve possible pentominoes, excluding mirror images and rotations.
|
|
|
|
|
|
|
|
|
|
|
|
|
Further Classification
[edit | edit source]Polyominoes can also be classified by dividing one into 2 parts by lines that go through the centroid of the polyomino and evaluating the angle between the orthogonal of such a line that goes through that centroid and a line also going through it and going through the centroid of such a part. Any set of points can be classified by such Half Centroid Deviation Angles HCDA, e.g. a circle is characterized by always having HCDA = 0°.
This is how the centroids S1, S2 and S3 of each of the 3 squares of a non-linear (i.e. a not line-shaped) tromino and its whole-polyomino centroid C are arranged:
S1 S2 C S3
If we want to classify the whole polyomino all HCDA that appear here should be factored in. Starting with the Dividing Line DL that (goes through C and) firstly also goes through S1 and turning clockwise then a phase with a Half Centroid HC only consisting of S2 appears. This phase starts with HCDA = arctan ½ – arctan 1 = – arctan ⅓ and ends with HCDA = 2 arctan ½ = arctan (4/3) when the DL meets S3. Signs depend on where the orthogonal is placed relative to the HC. In the next phase the HC is formed by S2 and S3 ... So the "Σ tan HCDA", the sum of all "tan (end HCDA) – tan (start HCDA)" of all such phases from 0 till 2 pi (0° till 360°) of a polyomino is a rational number about the degree of deviation from a circular shape. In case of this tromino there are 6 phases:
- (DL goes through) S1 → S3, HC: S2, partial Σ tan HCDA = 4/3 + 1/3 = 5/3
- S3 → S2, HC from S2 and S3, part. Σ tan HCDA = 2/3
- S2 → S1, HC: S3, part. Σ tan HCDA = 5/3
- S1 → S3, HC from S1 and S3, part. Σ tan HCDA = 4/3 + 1/3 = 5/3
- S3 → S2, HC: S1, part. Σ tan HCDA = 2/3
- S2 → S1, HC from S1 and S2, part. Σ tan HCDA = 5/3
The sum of all these partial Σ tan HCDA = 8. In the following table of those values of non-linear polyominoes (in a straight line polyomino tan 90° = ∞ is reached between orthogonal and HC), a polyomino is described in a linearized form which assumes the first 2 squares and specifies linearly further squares by "1" each if this place is not previously changed by "o" in a spiral course (comparable with that of Ulam spiral) to another possible place next to an already placed square not already excluded by a previous "o".
- Diagonal symmetry: \, /
- to specification line horizontal symmetry: —, vertical: |, horizontal and vertical: +
- rotational symmertry: •, if rotation also by 90° yields the same: ••
In the next columns the continued fraction expansions of Σ tan HCDA and of tan (greatest absolute deviation angle) are given, with continued fraction terms not separated by commas but by number of decimal digits – 1 apostrophes prepended to the terms:
1 non-linear tromino:
| o1 \ | 8 | 13 |
4 non-linear tetrominoes:
| 1oo1 | | 712 (= 7 ⅔) | 16 (= 7/6) |
| o1oo1 + | 8 | 1 |
| 1o1 | '10 | 2 |
| o11 • | '12 | 3 |
11 non-linear pentominoes:
| 1o1oo1 | 727 | 1 |
| 1oo11 | | 7141112 | 15 |
| 1oo1oooo1 + | 8 | 1 |
| 1o1ooo1 | | 861132 | 121'11 |
| o11oo1 | 84212 | 1112 |
| 1o1o1 \ | 8114 | 1117 |
| o11o1 \ | 91'11 2 | 1117 |
| 11oo1 | '10 12 | 2 |
| o1o11 • | '12 | 3 |
| 1o11 | '12 2'12 | 214 |
| 11o1 | '13 '15 | 233 |

34 non-linear hexominoes:
| Linearized form | Σ tan HCDA | tan (greatest deviation ∢) | * |
|---|---|---|---|
| o11oo1o1 — | 61221122 | 0111112 | 30 |
| 1o1oo11 | 6132118 | 012112 | 24 |
| 11oo11 | 6171'13 18 | 0121132 | 11 |
| o11oo1oo1 / | 61'21 152 | 012 | 33 |
| 1o1o1oo1 | 7418211122 | 015212 | 28 |
| 1oo111 | 72174122 | 1'15 | 29 |
| o111oooo1 | 7113121153'56 | 142 | 23 |
| 1o1oo1o1 + | 712 | 16 | 22 |
| 11oo1o1 | | 715 | 01'23 | 09 |
| 1o1o1ooo1 \ | 8 | 13 | 32 |
| o11o1oo1 | 83'16 6 | 1224 | 34 |
| 1o11oo1 | 81111174 | 112 | 18 |
| o111oo1 | 99122614 | 111'19 | 25 |
| 11o1o1 | 954 | 112 | 10 |
| o111ooo1 | 92214 | 1242 | 19 |
| 11o1ooo1 | 924 | 2 | 07 |
| 11o1oo1 | 91134 | 113 | 06 |
| 11oo1ooooo1 — | 9192 | 2 | 17 |
| 1o11ooo1 • | '10 | 2 | 21 |
| 1o1o11 | '10 52321114 | 26 | 26 |
| o1o1o1oo1 — | '10 3126 | 11226 | 08 |
| 11oo1oooo1 • | '10 2 | 22 | 16 |
| o11oo11 | '10 1152 | 22 | 13 |
| 1oo11o1 | | '10 12 | 2 | 12 |
| 1o11o1 | '11 4111'24 23 | 22113 | 27 |
| o1o11oo1 | '11 111112 | 3 | 14 |
| o111o1 | '12 1152312 | 26 | 31 |
| o11o11 • | '13 | 215 | 35 |
| 111ooooo1 | | '14 24 | 21'11 | 04 |
| o1o1o11 • | '14 2 | 32 | 15 |
| 11o11 | '14 1332 | 39 | 05 |
| 111oo1 | '15 326 | 2162 | 03 |
| 1o111 • | '17 | 42 | 20 |
| 111o1 | '17 141112 | 3313 | 02 |
Heptominoes (There are 107 distinct non-linear free ones):
| 1o1oo11oo1 | | 61118'12 | 0113 |
| 1o11ooooo11 — | 615221'18 2 | 0122 |
| 1oo111ooo1 + | 73 | 1 |
| 111ooooo11 | 72514 | 01'20 |
| 11o1oo1ooooo1 | 71251'10 512112 | 154 |
| o11o1oo1oo1 + | 8 | 1 |
| 11o1o1o1 \ | 851'28 111142 | 16'15 |
| 111oo11 | 831534 | 1'11 12 |
| 111oo1o1 | 9181'13 14 | 1113 |
| 111oo1oo1 | | '10 211613 | 111115 |
| 1o1o111 • | '12 | 3 |
| o11o11o1 \ | '13 '35 | 245 |
| o1o1o1o1oo1 | '13 '33 13 | 21133113 |
| 1oo11oooooo1o1 + | '13 3 | 3 |
| 111oo1oooo1 | '13 183 | 3113 |
| 111oo1ooooo1 | '14 73122 | 37 |
| 111oo1ooo1 • | '14 12 | 4 |
| o111o11 • | '16 | 3 |
| o1o1o1o11 • | '18 12 | 5 |
| 1111ooooo1 | '19 151132 | 44212 |
| 1111oo1 | '20 1''104 | 4352 |
| 1111o1 | '23 3111113 | 432 |
...
Octominoes (There are 52 distinct non-linear symmetric free ones):
| 1o1o1oo1o1oo1 + | 612 | 02 |
| 1o11ooo11oooooo1 •• | 7 | 013 |
| o11oo1o1oo11 + | 73 | 1 |
| 1o1o11ooo1ooo1 • | 7144 | 132 |
| 1o11oo1oo1ooo1 / | 7151132 | 132 |
| 11o1oo1o1o1 + | 812 | 13 |
| 1oo11o11ooo1 + | 95 | 2 |
| 11o11ooo1o1 • | '10 3 | 2 |
| o1o1oo1111 • | '11 '28 1113 | 29 |
| 1o11o111 • | '15 1121123 | 3122 |
| o11o11o11 • | '15 12 | 3 |
| o1111o11 • | '18 | 312 |
| 11o1111 • | '23 12 | 63 |
...
Nonominoes:
| 11oo1111ooooo1 • | 712 | 13 |
| 1o11o11ooo1oo1 • | '10 | 113 |
| 11o1o1111 • | '14 3 | 312 |
| 1o111o111 • | '20 | 42 |
| o11111o11 • | '21 | 42 |
...
10-ominoes:
| 11oo111ooo1o1ooo1 + | 612112 | 02 |
| 11o1o1oo1o1o1oo1 + | 61311154 | 0111212 |
| 11o1oo11oo11oooo1 • | 61'19 1112 | 013 |
| 1oo11o1o1o11ooo1 + | '15 92 | 22 |
| 1o11o11o111 • | '18 ''126 | 417 |
| 11o11o1111 • | '18 1392 | 41118 |
| o111o111o11 • | '21 1'59 | 44 |
| 1o1111o111 • | '23 8113 | 4242 |
| o111111o11 • | '23 1'10 | 54 |
| 111o11111 • | '30 162 | 84 |
...
11-ominoes:
| 111ooooo1111oooo1o1 • | 61'20 | 0122 |
| 111o1o11111 • | '17 2113112 | 42 |
| 11o111o1111 • | '23 16 | 5122 |
| 1o11111o111 • | '26 12 | 5 |
| o1111111o11 • | '27 23 | 62 |
...
12-ominoes:
| o11o1oo11o1oo11o11 + | 62'10 | 03 |
| 11o1o1o1oo1o1o1oo1oo1 + | 62'10 | 03 |
| 11oo11o1ooo11o1ooo1oo1 •• | 612 | 02 |
| 1o11oo1o11oo1o1o1ooo1 + | 614 | 0112 |
| 11o1o1oo1o1o1oo111 + | 6 1'11 | 0125 |
| o111oo111oooo111ooooo1 •• | 61'12 42 | 0122 |
| 1o111o111o111 • | '25 4 | 41'15 |
| 11o1111o1111 • | '27 1'11 | 68 |
...
So the sequence of the number of continued fraction terms of Σ tan HCDA of the non-linear polyominoes sorted that way firstly by the number of their squares and then by their Σ tan HCDA starts with 1, 3,1,1,1, 3,7,1,6,5,4,4,3,1,3,2, 8,7,7,6,10,8,11,3,3,1,4,8,8,3,5,3,5,4,1,9,5,2,5,3,8,7,8,1,3,2,5,4,1,7, ... Every 1 in this sequence means Σ tan HCDA of the certain polyomino is a natural number, which seems to require also some criteria of symmetry or at least similar harmony. The sequence of those naturals is 8, 8,10,12, 8,12, 8,10,13,17, ... The Online Encyclopedia of Integer Sequence currently does not have these 2 sequences, only countings of all possible polyominoes with n cells like that of all free ones, and of all with or without holes or certain symmetries can be found there. So it seems to remain unsolved for now how many polyominoes have natural Σ tan HCDA and which natural numbers can be a polyomino's Σ tan HCDA. Additional editing here for further clarification is welcome.
Here the calculation of a list of the polyomino's square's positions in a complex plane, Σ tan HCDA and absolute value of tan (greatest deviation angle) from a given linearized polyomino specification by using Maxima:
t:"(a:[0,1],e:[],b:1/2,c:2,d:1,f:0,g:0,v:0, for n:1 thru slength(s)do(h:a[c]+d, if charat(s,n)=ascii(49)then (a:endcons(h,a),c:c+1,b:b+(a[c]-b)/c,d:1,f:0,g:0,h:a[c]+d) else e:endcons(h,e), if(charat(s,n)#ascii(49))or member(h,a)or member(h,e)then do (if f=bit_rsh(g,1)then(f:0,g:g+1)else f:f+1, d:d+%i^g,h:a[c]+d, if(member(h+1,a)or member(h-1,a)or member(h+%i,a)or member(h-%i,a)) and not member(h,a)and not member(h,e)then return(0))), print(a),e:[], for n:1 thru c do(a[n]:a[n]-b,e:endcons(carg(a[n]),e)),d:0,f:e[1], do(b:0,g:0,h:%pi*2, for n:1 thru c do(if ((e[n]>f)and(e[n]<=(f+%pi)))or (((e[n]+%pi*2)>f)and((e[n]+%pi)<=f))or (((e[n]-%pi*2)>f)and((e[n]-%pi*3)<=f))then (g:g+1,b:b+(a[n]-b)/g,p:e[n]-f, if p<0 then p:p+%pi*2, if p>%pi*2 then p:p-%pi*2, if(p>0)and(p<h)then(h:p,k:n,q:1))else (p:e[n]-f-%pi, if p<0 then p:p+%pi*2, if p>%pi*2 then p:p-%pi*2, if(p>0)and(p<h)then(h:p,k:n,q:-1))), b:carg(b), w:cot(f-b), d:d+w, w:abs(w), if v<w then v:w, w:cot(e[k]-b), d:d-w, w:abs(w), if v<w then v:w, if q=1 then f:e[k]else(if e[k]>0 then f:e[k]-%pi else f:e[k]+%pi), if f=e[1] then return(0)), d:bfloat(d),v:bfloat(v),[d,cf(d),v,cf(v)])"$s:"o11oo1"$eval_string(t)
In case of the non-linear tromino "o1" this program obtains its 3 points with centroid at (0; 0):
- a[1] = –2/3 – /3
- a[2] = 1/3 – /3
- a[3] = 1/3 + 2 /3
Then adds these 6 differences together:
- cot (arg a[1] + pi/4) – cot (arg a[3] + pi/4) = 1/3 + 1/3
- cot (arg a[3] – arctan ½) – cot (arg a[2] – arctan ½) = 4/3 + 1/3
- cot (arg a[2] – arctan 2) – cot (arg a[1] – arctan 2) = 1/3 + 4/3
- cot (arg a[1] – 3 pi/4) – cot (arg a[3] – 3 pi/4) = 1/3 + 1/3
- cot (arg a[3] – arctan ½) – cot (arg a[2] – arctan ½) = 4/3 + 1/3
- cot (arg a[2] – arctan 2) – cot (arg a[1] – arctan 2) = 1/3 + 4/3
Ellipses
[edit | edit source]Ellipses
[edit | edit source]Ellipses are sometimes called ovals. Ellipses contain two foci. The sum of the distance from a point on the ellipse to one focus and that same point to the other focus is constant
2-Dimensional Functions
[edit | edit source]Geometry/2-Dimensional Functions
3-Dimensional Functions
[edit | edit source]Geometry/3-Dimensional Functions
Area Shapes Extended into 3rd Dimension
[edit | edit source]Geometry/Area Shapes Extended into 3rd Dimension
Area Shapes Extended into 3rd Dimension Linearly to a Line or Point
[edit | edit source]Geometry/Area Shapes Extended into 3rd Dimension Linearly to a Line or Point
Polyhedras
[edit | edit source]Ellipsoids and Spheres
[edit | edit source]Geometry/Ellipsoids and Spheres
Coordinate Systems
[edit | edit source]Suppose you are an astronomer in America. You observe an exciting event (say, a supernova) in the sky and would like to tell your colleagues in Europe about it. Suppose the supernova appeared at your zenith. You can't tell astronomers in Europe to look at their zenith because their zenith points in a different direction. You might tell them which constellation to look in. This might not work, though, because it might be too hard to find the supernova by searching an entire constellation. The best solution would be to give them an exact position by using a coordinate system.
On Earth, you can specify a location using latitude and longitude. This system works by measuring the angles separating the location from two great circles on Earth (namely, the equator and the prime meridian). Coordinate systems in the sky work in the same way.
The equatorial coordinate system is the most commonly used. The equatorial system defines two coordinates: right ascension and declination, based on the axis of the Earth's rotation. The declination is the angle of an object north or south of the celestial equator. Declination on the celestial sphere corresponds to latitude on the Earth. The right ascension of an object is defined by the position of a point on the celestial sphere called the vernal equinox. The further an object is east of the vernal equinox, the greater its right ascension.
A coordinate system is a system designed to establish positions with respect to given reference points. The coordinate system consists of one or more reference points, the styles of measurement (linear measurement or angular measurement) from those reference points, and the directions (or axes) in which those measurements will be taken. In astronomy, various coordinate systems are used to precisely define the locations of astronomical objects.
Latitude and longitude are used to locate a certain position on the Earth's surface. The lines of latitude (horizontal) and the lines of longitude (vertical) make up an invisible grid over the earth. Lines of latitude are called parallels. Lines of longitude aren't completely straight (they run from the exact point of the north pole to the exact point of the south pole) so they are called meridians. 0 degrees latitude is the Earth's middle, called the equator. 0 degrees longitude was tricky because there really is no middle of the earth vertically. It was finally agreed that the observatory in Greenwich, U.K. would be 0 degrees longitude due to its significant role in scientific discoveries and creating latitude and longitude. 0 degrees longitude is called the prime meridian.
Latitude and longitude are measured in degrees. One degree is about 69 miles. There are 60 minutes (') in a degree and 60 seconds (") in a minute. These tiny units make GPS's (Global Positioning Systems) much more exact.
There are a few main lines of latitude:the Arctic Circle, the Antarctic Circle, the Tropic of Cancer, and the Tropic of Capricorn. The Antarctic Circle is 66.5 degrees south of the equator and it marks the temperate zone from the Antarctic zone. The Arctic Circle is an exact mirror in the north. The Tropic of Cancer separates the tropics from the temperate zone. It is 23.5 degrees north of the equator. It is mirrored in the south by the Tropic of Capricorn.
Horizontal coordinate system
[edit | edit source]One of the simplest ways of placing a star on the night sky is the coordinate system based on altitude and azimuth, thus called the Alt-Az or horizontal coordinate system. The reference circles for this system are the horizon and the celestial meridian, both of which may be most easily graphed for a given location using the celestial sphere.
In simplest terms, the altitude is the angle made from the position of the celestial object (e.g. star) to the point nearest it on the horizon. The azimuth is the angle from the northernmost point of the horizon (which is also its intersection with the celestial meridian) to the point on the horizon nearest the celestial object. Usually azimuth is measured eastwards from due north. So east has az=90°, south has az=180°, west has az=270° and north has az=360° (or 0°). An object's altitude and azimuth change as the earth rotates.
Equatorial coordinate system
[edit | edit source]The equatorial coordinate system is another system that uses two angles to place an object on the sky: right ascension and declination.
Ecliptic coordinate system
[edit | edit source]The ecliptic coordinate system is based on the ecliptic plane, i.e., the plane which contains our Sun and Earth's average orbit around it, which is tilted at 23°26' from the plane of Earth's equator. The great circle at which this plane intersects the celestial sphere is the ecliptic, and one of the coordinates used in the ecliptic coordinate system, the ecliptic latitude, describes how far an object is to ecliptic north or to ecliptic south of this circle. On this circle lies the point of the vernal equinox (also called the first point of Aries); ecliptic longitude is measured as the angle of an object relative to this point to ecliptic east. Ecliptic latitude is generally indicated by , whereas ecliptic longitude is usually indicated by .
Galactic coordinate system
[edit | edit source]As a member of the Milky Way Galaxy, we have a clear view of the Milky Way from Earth. Since we are inside the Milky Way, we don't see the galaxy's spiral arms, central bulge and so forth directly as we do for other galaxies. Instead, the Milky Way completely encircles us. We see the Milky Way as a band of faint starlight forming a ring around us on the celestial sphere. The disk of the galaxy forms this ring, and the bulge forms a bright patch in the ring. You can easily see the Milky Way's faint band from a dark, rural location.
Our galaxy defines another useful coordinate system — the galactic coordinate system. This system works just like the others we've discussed. It also uses two coordinates to specify the position of an object on the celestial sphere. The galactic coordinate system first defines a galactic latitude, the angle an object makes with the galactic equator. The galactic equator has been selected to run through the center of the Milky Way's band. The second coordinate is galactic longitude, which is the angular separation of the object from the galaxy's "prime meridian," the great circle that passes through the Galactic center and the galactic poles. The galactic coordinate system is useful for describing an object's position with respect to the galaxy's center. For example, if an object has high galactic latitude, you might expect it to be less obstructed by interstellar dust.
Transformations between coordinate systems
[edit | edit source]
One can use the principles of spherical trigonometry as applied to triangles on the celestial sphere to derive formulas for transforming coordinates in one system to those in another. These formulas generally rely on the spherical law of cosines, known also as the cosine rule for sides. By substituting various angles on the celestial sphere for the angles in the law of cosines and by thereafter applying basic trigonometric identities, most of the formulas necessary for coordinate transformations can be found. The law of cosines is stated thus:
To transform from horizontal to equatorial coordinates, the relevant formulas are as follows:
where is the right ascension, is the declination, is the local sidereal time, is the altitude, is the azimuth, and is the observer's latitude. Using the same symbols and formulas, one can also derive formulas to transform from equatorial to horizontal coordinates:
Transformation from equatorial to ecliptic coordinate systems can similarly be accomplished using the following formulae:
where is the right ascension, is the declination, is the ecliptic latitude, is the ecliptic longitude, and is the tilt of Earth's axis relative to the ecliptic plane. Again, using the same formulas and symbols, new formulas for transforming ecliptic to equatorial coordinate systems can be found:
- Traditional Geometry:
Topology
[edit | edit source]A topological space is a set X, and a collection of subsets of X, C such that both the empty set and X are contained in C and the union of any subcollection of sets in C and the intersection of any finite subcollection of sets in C are also contained within C. The sets in C are called open sets. Their complements relative to X are called closed sets.
Given two topological spaces, X and Y, a map f from X to Y is continuous if for every open set U of Y, f−1(U) is an open set of X.
Erlanger Program
[edit | edit source]Hyperbolic and Elliptic Geometry
[edit | edit source]There are precisely three different classes of three-dimensional constant-curvature geometry: Euclidean, hyperbolic and elliptic geometry. The three geometries are all built on the same first four axioms, but each has a unique version of the fifth axiom, also known as the parallel postulate. The 1868 Essay on an Interpretation of Non-Euclidean Geometry by Eugenio Beltrami (1835 - 1900) proved the logical consistency of the two Non-Euclidean geometries, hyperbolic and elliptic.
The Parallel Postulate
[edit | edit source]
The parallel postulate is as follows for the corresponding geometries.
Euclidean geometry: Playfair's version: "Given a line l and a point P not on l, there exists a unique line m through P that is parallel to l." Euclid's version: "Suppose that a line l meets two other lines m and n so that the sum of the interior angles on one side of l is less than 180°. Then m and n intersect in a point on that side of l." These two versions are equivalent; though Playfair's may be easier to conceive, Euclid's is often useful for proofs.
Hyperbolic geometry: Given an arbitrary infinite line l and any point P not on l, there exist two or more distinct lines which pass through P and are parallel to l.
Elliptic geometry: Given an arbitrary infinite line l and any point P not on l, there does not exist a line which passes through P and is parallel to l.
Hyperbolic Geometry
[edit | edit source]Hyperbolic geometry is also known as saddle geometry or Lobachevskian geometry. It differs in many ways to Euclidean geometry, often leading to quite counter-intuitive results. Some of these remarkable consequences of this geometry's unique fifth postulate include:
1. The sum of the three interior angles in a triangle is strictly less than 180°. Moreover, the angle sums of two distinct triangles are not necessarily the same.
2. Two triangles with the same interior angles have the same area.
Models of Hyperbolic Space
[edit | edit source]The following are four of the most common models used to describe hyperbolic space.
1. The Poincaré Disc Model. Also known as the conformal disc model. In it, the hyperbolic plane is represented by the interior of a circle, and lines are represented by arcs of circles that are orthogonal to the boundary circle and by diameters of the boundary circle.
2. The Klein Model. Also known as the Beltrami-Klein model or projective disc model. In it, the hyperbolic plane is represented by the interior of a circle, and lines are represented by chords of the circle. This model gives a misleading visual representation of the magnitude of angles.
3. The Poincaré Half-Plane Model. The hyperbolic plane is represented by one-half of the Euclidean plane, as defined by a given Euclidean line l, where l is not considered part of the hyperbolic space. Lines are represented by half-circles orthogonal to l or rays perpendicular to l.
4. The Hyperboloid Model. The hyperbolic plane is represented on one of the sheets of a 2-sheeted hyperboloid. This model is used in modern physics to represent velocity space.
Defining Parallel
[edit | edit source]Based on this geometry's definition of the fifth axiom, what does parallel mean? The following definitions are made for this geometry. If a line l and a line m do not intersect in the hyperbolic plane, but intersect at the plane's boundary of infinity, then l and m are said to be parallel. If a line p and a line q neither intersect in the hyperbolic plane nor at the boundary at infinity, then p and q are said to be ultraparallel.
The Ultraparallel Theorem
[edit | edit source]For any two lines m and n in the hyperbolic plane such that m and n are ultraparallel, there exists a unique line l that is perpendicular to both m and n.
Elliptic Geometry
[edit | edit source]Elliptic geometry may be first considered as rotation geometry in 3D space: every rotation has both an axis (say specified by a unit vector r) and a turn, generally ranging from 0 degrees to 180 degrees. A turn in the interval (180, 360) may be interpreted as being about the opposite axis −r with turn taken as the complement in 360.
w:William Rowan Hamilton practiced celestial geometry as an astronomer. He invented 4D quaternion geometry which has a 3D sphere of versors that represent the sides of spherical triangles. The versor algebra has products corresponding to composed rotations. Elliptic geometry looks at this product as a spherical triangle: a side of the triangle is a versor, and quaternion multiplication relates two sides to the third as follows:
To get a versor, start with the formula of Euler
that uses an "imaginary unit" i with i2 = − 1. Now imagine an unit sphere of such units. Call a generic point on this sphere r so r2 = −1. For three points i, j, k at right angles to eachother, the unit vector may be written Hamilton's convention has i, j, and k anticommute, so ij = −ji, etcetera. To this space of imaginaries, Hamilton adjoined a real number axis to form a real quaternion For a given r, its versors lie on a circle through 1 and −1. As r ranges over S2 these circles form the three-sphere. The elliptic geometry of rotations in 3-space has this hypersphere of versors as points. To obtain the distance between versors v and w, first find the versor then use its turn for the distance.
The connection between rotations and versor operators is shown in Associative Composition Algebra/Quaternions.
Affine Geometry
[edit | edit source]Projective Geometry
[edit | edit source]Neutral Geometry
[edit | edit source]Topics
[edit | edit source]- Euclid's First Four Postulates
- Euclid's Fifth Postulate
- Incidence Geometry
- Projective and Affine Planes (necessary?)
- Axioms of Betweenness
- Pasch and Crossbar
- Axioms of Congruence
- Continuity (necessary?)
- Hilbert Planes
- Neutral Geometry
Requests
[edit | edit source]If you would like to request anything in this topic please post it below.
Request Submissions
[edit | edit source]Inversive Geometry
[edit | edit source]- Modern geometry
Algebraic Geometry
[edit | edit source]Differential Geometry
[edit | edit source]Algebraic Topology
[edit | edit source]Noncommutative Geometry
[edit | edit source]Geometry/Noncommutative Geometry
- An Alternative Way and Alternative Geometric Means of Calculating the Area of a Circle =
Geometry/An Alternative Way and Alternative Geometric Means of Calculating the Area of a Circle












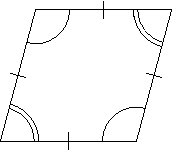



































![{\displaystyle t\in [a,b]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b7f3050ace6dc0dd95250c418528da28eb477ffe)














































































