Trigonometry/Law of Cosines

Law of Cosines

The Pythagorean theorem is a special case of the more general theorem relating the lengths of sides in any triangle, the law of cosines:

$a^{2}+b^{2}-2ab\cos(\theta )=c^{2}$

where $\theta$ is the angle between sides $a$ and $b$ .

Does the formula make sense?

This formula had better agree with the Pythagorean Theorem when $\theta =90^{\circ }$ .

So try it...

When $\theta =90^{\circ }$ , $\cos(\theta )=\cos(90^{\circ })=0$

The $-2ab\cos(\theta )=0$ and the formula reduces to the usual Pythagorean theorem.

Permutations

For any triangle with angles $A,B,C$ and corresponding opposite side lengths $a,b,c$ , the Law of Cosines states that

$a^{2}=b^{2}+c^{2}-2bc\cdot \cos(A)$

$b^{2}=a^{2}+c^{2}-2ac\cdot \cos(B)$

$c^{2}=a^{2}+b^{2}-2ab\cdot \cos(C)$

Proof

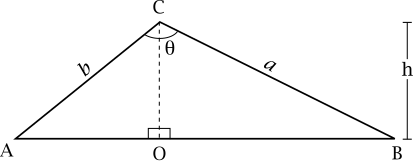
Dropping a perpendicular $OC$ from vertex $C$ to intersect $AB$ (or $AB$ extended) at $O$ splits this triangle into two right-angled triangles $AOC$ and $BOC$ , with altitude $h$ from side $c$ .

First we will find the lengths of the other two sides of triangle $AOC$ in terms of known quantities, using triangle $BOC$ .

$h=a\sin(B)$

Side $c$ is split into two segments, with total length $c$ .

${\overline {OB}}$ has length ${\overline {BC}}\cos(B)=a\cos(B)$

${\overline {AO}}={\overline {AB}}-{\overline {OB}}$ has length $c-a\cos(B)$

Now we can use the Pythagorean Theorem to find $b$ , since $b^{2}={\overline {AO}}^{2}+h^{2}$ .

$b^{2}$	$={\bigl (}c-a\cos(B){\bigr )}^{2}+a^{2}\sin ^{2}(B)$
	$=c^{2}-2ac\cos(B)+a^{2}\cos ^{2}(B)+a^{2}\sin ^{2}(B)$
	$=a^{2}+c^{2}-2ac\cos(B)$

The corresponding expressions for $a$ and $c$ can be proved similarly.

The formula can be rearranged:

$\cos(C)={\frac {a^{2}+b^{2}-c^{2}}{2ab}}$

and similarly for $cos(A)$ and $cos(B)$ .

Applications

This formula can be used to find the third side of a triangle if the other two sides and the angle between them are known. The rearranged formula can be used to find the angles of a triangle if all three sides are known. See Solving Triangles Given SAS.