Quantum Field Theory/Introduction to The Standard Model
Local gauge symmetry. Gauge theories. Non-Abelian gauge theories.
[edit | edit source]Spontaneous symmetry breaking. Goldstone theorem. Higgs mechanism.
[edit | edit source]The Higgs mechanism is a theoretical framework which concerns the origin mass of elementary particles; technically it yields the only consistent explanation, how the masses of the W and Z bosons arise through spontaneous electroweak symmetry breaking. More generally, the Higgs mechanism is the way that the gauge bosons in any gauge theory get a nonzero mass.
Also for other particles, e.g. for fermions, a Higgs mechanism can explain the masses, again in a gauge invariant way.
The simplest realization of the Higgs mechanism in the standard model requires an extra Higgs field which interacts with the gauge fields, and which has a nonzero value in its lowest energy state, a vacuum expectation value. This means that all of space is filled with the background Higgs field, the so-called Higgs condensate. Interaction with this background field changes the low-energy spectrum of the gauge fields and the gauge bosons become massive.
The Higgs field has a non-trivial self-interaction, like the Mexican hat potential, which leads to spontaneous symmetry breaking: in the lowest energy state the symmetry of the potential (which includes the gauge symmetry) is broken by the condensate. Analysis of small fluctuations of the fields near the minimum reveals that the gauge bosons and other particles become massive. In the standard model, the Higgs field is an SU(2) doublet, a complex spinor with four real components, which is charged under the standard model U(1). After symmetry breaking, three of the four degrees of freedom in the Higgs field mix with the W and Z bosons, while the one remaining degree of freedom becomes the Higgs boson – a new scalar particle.
Goldstone Bosons
[edit | edit source]The problem with spontaneous symmetry breaking models in particle physics is that, according to Goldstone's theorem, they come with massless scalar particles. If a symmetry is broken by a condensate, acting with a symmetry generator on the condensate gives a second state with the same energy. So certain oscillations do not have any energy, and the particles associated with these oscillations have zero mass.
In the standard model, at temperatures high enough so that the symmetry is unbroken, all elementary particles except the scalar Higgs boson are massless. At a critical temperature, the Higgs field spontaneously slides from the point of maximum energy in a randomly chosen direction. Once the symmetry is broken, the gauge boson particles, such as the W bosons and Z boson, acquire masses. The mass can be interpreted as the result of the interactions of the particles with the "Higgs ocean".
Fermions, such as the leptons and quarks in the Standard Model, acquire mass as a result of their interaction with the Higgs field, but not in the same way as the gauge bosons.
Superconductivity
[edit | edit source]The Higgs mechanism can be considered as the superconductivity in the vacuum. It occurs when all of space is filled with a sea of particles which are charged, or in field language, when a charged field has a nonzero vacuum expectation value. Interaction with the quantum fluid filling the space prevents certain forces from propagating over long distances.
A superconductor expels all magnetic fields from its interior, a phenomenon known as the Meissner effect. This was mysterious for a long time, because it implies that electromagnetic forces somehow become short-range inside the superconductor. Contrast this with the behavior of an ordinary metal. In a metal, the conductivity shields electric fields by rearranging charges on the surface until the total field cancels in the interior. But magnetic fields can penetrate to any distance, and if a magnetic monopole (an isolated magnetic pole) is surrounded by a metal the field can escape without collimating into a string. In a superconductor, however, electric charges move with no dissipation, and this allows for permanent surface currents, not just surface charges. When magnetic fields are introduced at the boundary of a superconductor, they produce surface currents which exactly neutralize them. The Meissner effect is due to currents in a thin surface layer, whose thickness, the London penetration depth, can be calculated from a simple model.
This simple model, due to Lev Landau and Vitaly Ginzburg, treats superconductivity as a charged Bose–Einstein condensate. Suppose that a superconductor contains bosons with charge . The wavefunction of the bosons can be described by introducing a quantum field, , which obeys the Schrödinger equation as a field equation (in units where , the Planck quantum divided by , is replaced by 1):
The operator annihilates a boson at the point , while its adjoint creates a new boson at the same point. The wavefunction of the Bose–Einstein condensate is then the expectation value of , which is a classical function that obeys the same equation. The interpretation of the expectation value is that it is the phase that one should give to a newly created boson so that it will coherently superpose with all the other bosons already in the condensate.
When there is a charged condensate, the electromagnetic interactions are screened. To see this, consider the effect of a gauge transformation on the field. A gauge transformation rotates the phase of the condensate by an amount which changes from point to point, and shifts the vector potential by a gradient.
When there is no condensate, this transformation only changes the definition of the phase of at every point. But when there is a condensate, the phase of the condensate defines a preferred choice of phase.
The condensate wavefunction can be written as
where is real amplitude, which determines the local density of the condensate. If the condensate were neutral, the flow would be along the gradients of , the direction in which the phase of the Schrödinger field changes. If the phase changes slowly, the flow is slow and has very little energy. But now can be made equal to zero just by making a gauge transformation to rotate the phase of the field.
The energy of slow changes of phase can be calculated from the Schrödinger kinetic energy,
and taking the density of the condensate to be constant,
Fixing the choice of gauge so that the condensate has the same phase everywhere, the electromagnetic field energy has an extra term,
When this term is present, electromagnetic interactions become short-ranged. Every field mode, no matter how long the wavelength, oscillates with a nonzero frequency. The lowest frequency can be read off from the energy of a long wavelength A mode,
This is a harmonic oscillator with frequency . The quantity (=) is the density of the condensate of superconducting particles.
In an actual superconductor, the charged particles are electrons, which are fermions not bosons. So in order to have superconductivity, the electrons need to somehow bind into Cooper pairs. The charge of the condensate is therefore twice the electron charge . The pairing in a normal superconductor is due to lattice vibrations, and is in fact very weak; this means that the pairs are very loosely bound. The description of a Bose–Einstein condensate of loosely bound pairs is actually more difficult than the description of a condensate of elementary particles, and was only worked out in 1957 by Bardeen, Cooper and Schrieffer in the famous BCS theory.
Abelian Higgs model
[edit | edit source]In a relativistic gauge theory, the vector bosons are natively massless, like the photon, leading to long-range forces. This is fine for electromagnetism, where the force is actually long-range, but it means that the description of short-range weak forces by a gauge theory requires a modification.
Gauge invariance means that certain transformations of the gauge field do not change the energy at all. If an arbitrary gradient is added to A, the energy of the field is exactly the same. This makes it difficult to add a mass term, because a mass term tends to push the field toward the value zero. But the zero value of the vector potential is not a gauge invariant idea. What is zero in one gauge is nonzero in another.
So in order to give mass to a gauge theory, the gauge invariance must be broken by a condensate. The condensate will then define a preferred phase, and the phase of the condensate will define the zero value of the field in a gauge invariant way. The gauge invariant definition is that a gauge field is zero when the phase change along any path from parallel transport is equal to the phase difference in the condensate wavefunction.
The condensate value is described by a quantum field with an expectation value, just as in the Landau–Ginzburg model. To make sure that the condensate value of the field does not pick out a preferred direction in space-time, it must be a scalar field. In order for the phase of the condensate to define a gauge, the field must be charged.
In order for a scalar field to be charged, it must be complex. Equivalently, it should contain two fields with a symmetry which rotates them into each other, the real and imaginary parts. The vector potential changes the phase of the quanta produced by the field when they move from point to point. In terms of fields, it defines how much to rotate the real and imaginary parts of the fields into each other when comparing field values at nearby points.
The only renormalizable model where a complex scalar field Φ acquires a nonzero value is the Mexican-hat model, where the field energy has a minimum away from zero.
This defines the following Hamiltonian:
The first term is the kinetic energy of the field. The second term is the extra potential energy when the field varies from point to point. The third term is the potential energy when the field has any given magnitude.
This potential energy has a graph which looks like a Mexican hat, which gives the model its name. In particular, the minimum energy value is not at z=0, but on the circle of points where the magnitude of z is .
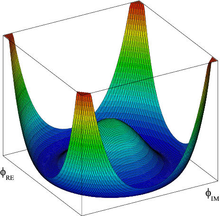
When the field (x) is not coupled to electromagnetism, the Mexican-hat potential has flat directions. Starting in any one of the circle of vacua and changing the phase of the field from point to point costs very little energy. Mathematically, if
with a constant prefactor, then the action for the field , i.e., the "phase" of the Higgs field Φ(x), has only derivative terms. This is not a surprise. Adding a constant to is a symmetry of the original theory, so different values of cannot have different energies. This is an example of Goldstone's theorem: spontaneously broken continuous symmetries lead to massless particles.
The Abelian Higgs model is the Mexican-hat model coupled to electromagnetism:
The classical vacuum is again at the minimum of the potential, where the magnitude of the complex field is equal to . But now the phase of the field is arbitrary, because gauge transformations change it. This means that the field can be set to zero by a gauge transformation, and does not represent any degrees of freedom at all.
Furthermore, choosing a gauge where the phase of the condensate is fixed, the potential energy for fluctuations of the vector field is nonzero, just as it is in the Landau–Ginzburg model. So in the abelian Higgs model, the gauge field acquires a mass. To calculate the magnitude of the mass, consider a constant value of the vector potential A in the x direction in the gauge where the condensate has constant phase. This is the same as a sinusoidally varying condensate in the gauge where the vector potential is zero. In the gauge where A is zero, the potential energy density in the condensate is the scalar gradient energy:
And this energy is the same as a mass term where .
Nonabelian Higgs mechanism
[edit | edit source]The Nonabelian Higgs model has the following action:
where now the nonabelian field is contained in D and in the tensor components and (the relation between and those components is well-known from the Yang–Mills theory).
It is exactly analogous to the Abelian Higgs model. Now the field is in a representation of the gauge group, and the gauge covariant derivative is defined by the rate of change of the field minus the rate of change from parallel transport using the gauge field A as a connection.
Again, the expectation value of Φ defines a preferred gauge where the condensate is constant, and fixing this gauge, fluctuations in the gauge field A come with a nonzero energy cost.
Depending on the representation of the scalar field, not every gauge field acquires a mass. A simple example is in the renormalizable version of an early electroweak model due to Julian Schwinger. In this model, the gauge group is SO(3) (or SU(2)--- there are no spinor representations in the model), and the gauge invariance is broken down to U(1) or SO(2) at long distances. To make a consistent renormalizable version using the Higgs mechanism, introduce a scalar field which transforms as a vector (a triplet) of SO(3). If this field has a vacuum expectation value, it points in some direction in field space. Without loss of generality, one can choose the z-axis in field space to be the direction that is pointing, and then the vacuum expectation value of is , where A is a constant with dimensions of mass ().
Rotations around the z axis form a U(1) subgroup of SO(3) which preserves the vacuum expectation value of , and this is the unbroken gauge group. Rotations around the x and y axis do not preserve the vacuum, and the components of the SO(3) gauge field which generate these rotations become massive vector mesons. There are two massive W mesons in the Schwinger model, with a mass set by the mass scale A, and one massless U(1) gauge boson, similar to the photon.
The Schwinger model predicts magnetic monopoles at the electroweak unification scale, and does not predict the Z meson. It doesn't break electroweak symmetry properly as in nature. But historically, a model similar to this (but not using the Higgs mechanism) was the first in which the weak force and the electromagnetic force were unified.
Standard model Higgs mechanism
[edit | edit source]The gauge group of the electroweak part of the standard model is . The Higgs mechanism is by a scalar field which is a weak SU(2) doublet with weak hypercharge −1, it has four real components or two complex components, and it transforms as a spinor under SU(2) and gets multiplied by a phase under U(1) rotations. Note that this is not the same as two complex spinors which mix under U(1), which would have eight real components, rather this is the spinor representation of the group U(2)--- multiplying by a phase mixes the real and imaginary part of the complex spinor into each other.
The group SU(2) is all unitary matrices, all the orthonormal changes of coordinates in a complex two dimensional vector space. Rotating the coordinates so that the first basis vector in the direction of makes the vacuum expection value of H the spinor . The generators for rotations about the x,y,z axes are by half the Pauli matrices , so that a rotation of angle about the z axis takes the vacuum to:
While the X and Y generators mix up the top and bottom components, the Z rotations only multiply by a phase. This phase can be undone by a U(1) rotation of angle , which multiplies by the opposite phase, since the Higgs has charge −1. Under both an SU(2) z-rotation and a U(1) rotation by an amount , the vacuum is invariant. This combination of generators:
defines the unbroken gauge group, where is the generator of rotations around the z-axis in the SU(2) and Y is the generator of the U(1). This combination of generators--- perform a z rotation in the SU(2) and simultaneously perform a U(1) rotation by half the angle--- preserves the vacuum, and defines the unbroken gauge group in the standard model. The part of the gauge field in this direction stays massless, and this gauge field is the actual photon.
The phase that a field acquires under this combination of generators is its electric charge, and this is the formula for the electric charge in the standard model. In this convention, all the Y charges in the standard model are multiples of . To make all the Y-charges in the standard model integers, you can rescale the Y part of the formula by tripling all the Y-charges if you like, and rewrite the charge formula as , but the normalization with Y/2 is the universal standard.
Affine Higgs mechanism
[edit | edit source]Ernst Stueckelberg discovered a version of the Higgs mechanism by analyzing the theory of quantum electrodynamics with a massive photon. Stueckelberg's model is a limit of the regular Mexican hat Abelian Higgs model, where the vacuum expectation value H goes to infinity and the charge of the Higgs field goes to zero in such a way that their product stays fixed. The mass of the Higgs boson is proportional to H, so the Higgs boson becomes infinitely massive and disappears. The vector meson mass is equal to the product , and stays finite.
The interpretation is that when a U(1) gauge field does not require quantized charges, it is possible to keep only the angular part of the Higgs oscillations, and discard the radial part. The angular part of the Higgs field has the following gauge transformation law:
The gauge covariant derivative for the angle (which is actually gauge invariant) is:
In order to keep fluctuations finite and nonzero in this limit, should be rescaled by H, so that its kinetic term in the action stays normalized. The action for the theta field is read off from the Mexican hat action by substituting .
since is the gauge boson mass. By making a gauge transformation to set , the gauge freedom in the action is eliminated, and the action becomes that of a massive vector field:
To have arbitrarily small charges requires that the U(1) is not the circle of unit complex numbers under multiplication, but the real numbers R under addition, which is only different in the global topology. Such a U(1) group is non-compact. The field transforms as an affine representation of the gauge group. Among the allowed gauge groups, only non-compact U(1) admits affine representations, and the U(1) of electromagnetism is experimentally known to be compact, since charge quantization holds to extremely high accuracy.
The Higgs condensate in this model has infinitesimal charge, so interactions with the Higgs boson do not violate charge conservation. The theory of quantum electrodynamics with a massive photon is still a renormalizable theory, one in which electric charge is still conserved, but magnetic monopoles are not allowed. For nonabelian gauge theory, there is no affine limit, and the Higgs oscillations cannot be too much more massive than the vectors.
Further consequences, e.g. for fermions
[edit | edit source]In spite of the introduction of spontaneous symmetry-breaking, also for fermions the mass terms oppose the gauge invariance. Therefore, also for these fields the mass terms should be replaced by a gauge-invariant "Higgs" mechanism. An obvious possibility is some kind of "Yukawa coupling" (see below) between the fermion field ψ and the Higgs field Φ, with unknown couplings , which after symmetry-breaking (more precisely: after expansion of the Lagrange density around a suitable ground state) again results in the original mass terms, which are now, however (i.e. by introduction of the Higgs field) written in a gauge-invariant way. The Lagrange density for the "Yukawa"-interaction of a fermion field ψ and the Higgs field Φ is
where again the gauge field A only enters (i.e., it is only indirectly visible). The quantities are the Dirac matrices, and is the already-mentioned "Yukawa"-coupling parameter. Already now the mass-generation follows the same principle as above, namely from the existence of a finite expectation value , as descibed above. Again, this is crucial for the existence of the property "mass".

































































