Problems In High School Chemistry/Printable version

Problems In High School Chemistry

The current, editable version of this book is available in Wikibooks, the open-content textbooks collection, at
https://en.wikibooks.org/wiki/Problems_In_High_School_Chemistry

Permission is granted to copy, distribute, and/or modify this document under the terms of the Creative Commons Attribution-ShareAlike 3.0 License.

Inchem/Atoms

How to break down an element

Have you ever wondered how to find how many electrons, protons, neutrons, and the atomic mass of an element? To find these things out about an element you need to know the basics of what the element is made of. An element is made from atoms, atoms are small building blocks that are in anything you breathe, feel or touch that’s matter. Atoms consist of protons, neutrons and electrons.

Now that you understand the basics of atoms You can now begin two determine the basics of an element. The first element that we will be breaking down is Ne which is NEON. A basic table to breakdown and element will look something like this. The first step in breaking down an element is to put the symbol of the element that you are given in the symbol column. Once you put the symbol down you can now put the elements name in the element column, in this case it would be NEON. Now comes the more challenging part, for all elements the number of electrons and protons will be the same. To find the number of electrons and protons you simply look at the number on the top left corner of the element on the periodic table. While the number is not always on the top left, it will likely be there.

In this case it is 20 - 10. The next step is to find the atomic mass. That can also be found on the element box either on the bottom or on the top right corner. The atomic mass for neon is 20. Now to find the neutrons, to find the number of neutrons that an element has, you must subtract the number of protons from the atomic mass. In this case it would be 20 which is the rounded value minus 10 which equals 10.

Symbol	Element	# electrons	# protons	# neutrons	atomic mass
Ne	Neon	10	10	10	20

Congratulations you have successfully broken down an element. Now let's try one that's a little bit harder. All the same steps apply no matter what the element is. The second element that we will be breaking down is Americium / Am.

Symbol	Element	# electrons	# protons	# neutrons	atomic mass
Am	Americium	95	95	148	243

Inchem/Elements

What are elements? Are they all the same? you've always heard of them, but what really are they?

Elements are the things you see when you look at the periodic table of elements, they make up everything we see in our daily lives like water, oxygen, etc. Elements are made up of atoms which are the fundamental unit of matter, of course not all atoms are the same, and consequently not all elements are the same, there are many different elements that are made up of many different atoms. An element will always have the same type of atoms in it, the number may vary, but the one thing that has to remain true is that each element has a specific designated atom, if 2 or more atoms that belong to different elements were to bond together that would create a compound.

Since all elements are different they all have different properties to them some of the biggest properties in elements are malleability (the ability to bend or change shape without breaking), conductivity (the ability to carry or transfer electricity), dissolving (in water),Luster (Shiny enough to be a mirror), and reactivity to air. Depending on the properties that each element has they can be grouped together, for example, Copper (Cu) and Silver (Ag) are both lustrous, conductive, and malleable which makes them very similar elements and so they can be grouped together, how do you know what elements are grouped together? usually you would have to test the elements and then compare their properties, but thanks to years of research a table was created that has already grouped the elements, this table was called the periodic table of elements.

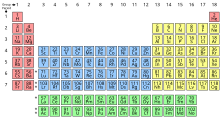
Periodic table The periodic table is a table made to classify and describe all elements. It has 118 elements and some are naturally occurring and some can only be found in a lab. These elements are divided into 2 main groups, metals and non-metals, but if you look closer, there are smaller groups, such as earth metals, noble gases, etc. Just by using the periodic table you can see the elements details, such as atomic mass, atomic number and if they are metals or non-metals. An element's group is determined by the similarity in it's properties, to put it simply, the more similar 2 elements are the more likely they are to both be in the same group, some of the biggest characteristic of metals are being conductive, being lustrous, and being malleable. Non-metals could be described as the complete opposite of metals, their biggest characteristics are being non conductive, being dull (not lustrous), and being brittle (not malleable).

But how do you know what properties and element has if you don't want to use the periodic table?

You test it, there are always tests you can do to find the properties of any element, even though some elements are going to be harder to test you will always be able to test their properties, each designated property for elements has a test that you can use to see whether the element you have possesses that property or not. What are the tests?

Luster: Any object that has the property of luster will be very shiny to the point where you can see your own reflection.

Malleability / Ductility: Malleability and ductility are essentially the same thing, to test it, grab a piece of the element and try to bend it or smash it, if the element does not break or shatter that means the element is malleable.

Conductivity: Since conductivity is the ability to conduct electricity you will need a battery and a light bulb in order to test the conductivity, hook up 2 cables to the battery and then connect the cables to a light bulb, the light bulb should light up, after doing that run the cables through the element and then to the light bulb, if the element is conductive the light bulb should light up, if not then the element is not conductive.

Dissolving: To test if an element is dissolving put it in a beaker or cup full of water and try to stir it, if the element seems like it mixed with the water it is dissolving, if not and the element remains in a solid shape then it is not dissolving.

Reactivity to air: Different elements react to air differently, but a very simple test to see if they are reactive to air would be scratching the surface of the element, if it looks different in the inside it means that it is reactive to air because the inside hasn't reacted to the air so it looks different.

Inchem/Bondtypes

Chemical Bonds

Everyone has this one person in the world that makes them feel good. You are comfortable and more confident when you are around them. In other words, you bond with them. This also happens in chemistry. When an atom's valence electrons (outer shell) are not full, they are not stable. There are elements like hydrogen which only need 2 valence electrons (duet) and then there are elements like chlorine which needs 8 valence electrons (an octet). But when there is another atom, they can try and steal their valence electron, so they have a stable valence shell themselves (covalent bond) or they share it (ionic bond). Overall, we call them chemical bonds. In chemistry, there are three types of bonds: ionic bonds, metallic bonds, and covalent bonds. We are going to focus on ionic and covalent bonds today.

Electron Affinity (EA): how well an atom attracts electrons

Ionization Energy (IE): how well an atom holds onto his electrons

Nonmetals have high IE and AE which means they can attract electrons and hold onto them easily. Metals have low IE and EA which means they cannot attract nor hold onto them easily.

Ionic Bonds

Ionic Bond between Na and F

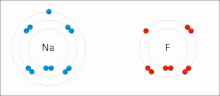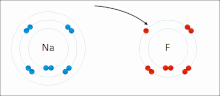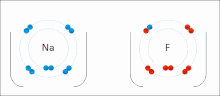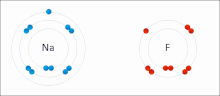
Made of nonmetals and metals. Nonmetals have high IE and high EA, which means they are strong enough to get an electron from the metal and keep their own electron, so they do not lose it. Meanwhile the metal is too “weak” to hold onto its electron let alone steal an electron from the nonmetal.

Covalent Bonds

Covalent bonds in four common molecules

Made of nonmetals. The non-metal atoms have a high IE and high EA. Both are strong enough to take an electron and to protect their own electron, but none is stronger than the other. Neither can steal electrons from the other, so they end up sharing electrons. The sharing of the electron pair between atoms keeps both atoms together, creating the covalent bond.

Inchem/reactions/decomposition

An example of an electrolysis lab setup

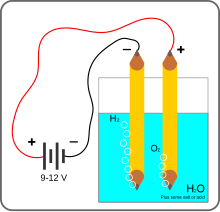
Decomposition is the breaking down a compound back into its components (elements). A form of decomposition is electrolysis, a method of decomposition through adding electricity. When new energy (in the form of electricity) is added to the molecules they become unstable again and start vibrating. After vibrating enough, the element with the higher electron affinity takes the remaining electrons to remain stable, and the other atoms become unstable

PhChem/thermo1/table

Fill in the missing values in the table. The table contains 63 blanks, so we expect a student to solve the table in 90 minutes.

Note: -

1.) Each of the processes are carried out on 1 mole of  ideal gas.

2.) The odd numbered processes are for monoatomic ideal gases, while the rest are for diatomic ideal gases. For a monoatomic ideal gas, Cv = (3/2)R and for a diatomic ideal gas, Cv = (5/2)R. Cp = Cv + R, so theyre (5/2)R and (7/2)R respectively; not considering the vibrational degrees of freedom which we neglect at relatively low temperatures.

3.) Throughout the entire page, ∫PextdV implies the definite integral over the initial and the final stages as the lower and upper limits respectively. It DOES NOT designate the indefinite integral.

The Question

Process

Ti

Vi

Pi

Tf

Vf

Pf

Q

∆E

w

∫PextdV

∆H

1

Rev. Adiabatic

400K

02.0 L

04.0 L

2

Rev. Adiabatic

300K

01.00 atm

02.00 atm

3

Isobaric Rev.

300K

01.00 atm

600K

4

Rev. Isothermal

300K

03.0 L

06.0 L

5

Rev. Isothermal

250K

03.0 L

02.00 atm

6

Rev. Isochoric

02.00 atm

400K

1000 J

7

Irrev. Adiabatic

350K

01.00 atm = ext pressure

1000 J

8

Irrev. Isothermal

01.0 L

10.00 atm = ext pressure

0500 J

 

 

 

 

If you feel stumped by the sheer lack of data in the table, know that this is not an impossible task. It is, actually, a mechanical, mindless work. This is mainly because the table doesn't require a proper understanding of a process and the related intricacies. All it demands is a harmony among the formulae in the student's mind. Filling up this table will give a strong practice of the mathematics involved, and will also benefit the student in simplifying the more complex numerical problems.

 

If you still do not gather any courage to fill the table, you should consider solving the introductory problems.

 

The Answer

 

Process

Ti

Vi

Pi

Tf

Vf

Pf

Q

∆E

W

∫PextdV

∆H

1

Rev. Adiabatic

400K

02.0 L

16.42 atm

252K

04.0 L

05.18 atm

0

-1846 J

-1846J

1846J

-3076J

2

Rev. Adiabatic

300K

24.63 L

01.00 atm

365K

29.96 L

02.00 atm

0

1351J

-1351J

1351J

1891J

3

Isobaric Rev.

300K

24.63 L

01.00 atm

600K

49.26 L

01.00 atm

6236 J

3741J

-2495J

2495J

6236J

4

Rev. Isothermal

300K

03.0 L

8.21 atm

300K

06.0 L

04.10 atm

1729 J

0

-1729J

1729J

0

5

Rev. Isothermal

250K

03.0 L

6.84 atm

250K

10.26 L

02.00 atm

2556 J

0

-2556J

2556J

0

6

Rev. Isochoric

352 K

14.44 L

02.00 atm

400K

14.44 L

02.27 atm

1000 J

1000J

0

0

1397J

7

Irrev. Adiabatic

270 K

38.63 L

0.57 atm

350K

28.73 L

01.00 atm = ext pressure

0

1000J

1000J

- 1000J

1663J

8

Irrev. Isothermal

424 K

01.0 L

34.81

424K

3.48 L

10.00 atm = ext pressure

500 J

0

-500J

0500 J

0

 

Hints

Column 1

(a)Pi - Since three of the gas parameters are known, the fourth parameter can always be found using the ideal gas equation on the initial state.

(b)Tf - Ideal gas equation has four parameters, only two are known, so we need one more equation.

 

Since this is a reversible adiabatic process, the pressure P and Volume V satisfy the relation (P)(V^y) = constant throughout. Also, the ideal gas equation is satisfied throughout in any reversible process. PV = nrt. Devide the first equation by the second, and you'll find that you just eliminated one unknown - P. It is now left to one equation, Ti[Vi^(y-1)] = Tf[Vf^(y-1])  and one unknown, Tf.

 

The final calculations require a simple use of log tables. In competitive exams however, the paper setter will give all the values of the logarithms involved, so you need not concern yourself with the complexity of the calculations.

 

(c)Pf - Now that three quantities in the ideal gas equation are known, you can easily find Pf.

 

Alternatively, you could directly find Pf through using P(V^y) = constant on initial and final stages of the process, and then used the ideal gas equation to find Tf. Notice that we avoided finding Pf when we were asked to find only Tf, by eliminating the unknown.

 

(d)Q - In an adiabatic process, the heat exchanged with the surroundings is ________

 

(e)∆E - Internal energy is fairly simple for an ideal gas, and depends only upon temperature of the ideal gas.  It is nCvTf at Tf, and nCvTi at Ti. So the change, then, is nCv(Tf-Ti). Try to relate it to the expression for heat gained and heat lost learnt in thermal physics in the eleventh and ninth standard in Indian Schools.

 

(f)w - It is the additive inverse of ∫PextdV in Chemistry conventions. In Physics, it is the same as ∫PextdV. See below on how to find ∫PextdV, that is the work done by the gas during the change in volume.

 

(g)∫PextdV - Well, performing the whole integration by substituting P is a good idea. P = nrt/v will not work because even T will vary. We cannot integrate two variables in this way. Instead, substitute P as [Constant]/(V^y) and carry out the integration. After the integration is done, substitute the value of constant as Pf(Vf^y) and Pi(Vi^y) to get the result in terms of known quantities.

 

But, the same work can be done in an easy manner! Use the first law, Q = ∆E + ∫PextdV. You will find that in an adiabatic process, since the heat exchanged is 0, ∫PextdV becomes the additive inverse of the change in the internal energy. In laymen's terms, since in an adiabatic process, the heat input is zero, whatever work that is done by the gas comes at the expence of its internal energy (and vice versa). This explaining subtly why the First Law Of Thermodynamics is actually the manifestation of the law of conservation of energy.
 

**NOTE :: The integral requires the value of Pext. In a reversible process, since the External Pressure is the same as the internal pressure of a gas, the whole substitution makes sence. Remember, Pext is not necessrily equal to nrt/v, but internal pressure = nrt/v.**

(h)∆H - Finally, the enthalpy change of the reaction. It is pleasing to know that this again, depends only on the change in temperature for an ideal gas. That is, ∆H = nCp∆T.

 

The First Column, Done!!!

 

Column 2

(a)Vi - Use the ideal gas equation over the first state.

(b)Tf - Ideal gas equation, this time again, will have two unknowns. We need one more equation, which comes from (P)(V^y) = constant. Going exactly by the steps in column one, however, we won't be eliminating the unknown Vf, while eliminating the known P. Instead, to eliminate Vf, while keeping the knowns, raise both sides of the ideal gas equation by Y and devide the first equation by the second. You will end up with (T^y)[P^(1-Y)] = constant. Use it on the initial and final stages.

 

(c)Vf - Now that three quantities in the ideal gas equation are known, you can easily find Vf.

 

Alternatively, you could directly find Vf through using P(V^y) = constant on initial and final stages of the process, and then used the ideal gas equation to find Tf. Notice that we avoided finding Vf when we were asked to find only Tf, by eliminating the unknown.

 

(d)Q - What is the defining charachteristic of an adiabatic process?

 

(e)∆E - nCv∆T pretty simple. :)

 

(f)w - Tip::Throughout the table, this column is going to be the additive inverse of ∫PextdV

 

(g)∫PextdV - Tip::Although not throughout the table, but in any kind of adiabatic process (reversible or irreversible), ∫PextdV is the abditive inverse of ∆E, according to the first law.

 

**This is because the first law of the thermodynamics is universal, and it doesnt matter if the process is reversible or irreversible to apply it.

 

However, the ideal gas equation and any results derived from it are applicable only in the case of a thermodynamic equilibrium. Since this is not possible in case of an irreversible process, we cannot use the mentioned equations over the course of the reactions. Even then, the ideal gas equation can be used before and after an irreversible process takes place.**
 

(h)∆H -  ∆H = nCp∆T.

 

Second column completed as well.

 

The Third Column

(a)Vi - the _______ equation, fill in the blank mentally.

(b)Vf - Again, two unknowns for the ideal gas equation. But is Pf actually an unknown? Remember that this is an isobaric process, and that the initial pressure is known, so the final pressure is known as well.

(c)Pf - Done

(d)Q - Non zero for the first time!! We will use the first law, Q = ∆E + ∫PextdV. See it again as the law of conservation of energy, the heat supplied is used in heating the gas as well as doing work. Now find the other two terms.

(e)∆E - nCv∆T :)

(f)∫PextdV - Since this is a reversible process, the external pressure is the same as the internal pressure, and the internal pressure is constant. It comes out of the integration. So we are left with  (P)∫dV, which evaluates to P∆V. Do the needful!!

(h)∆H - nCp∆T !!!

 

Note : - In evaluating ∫PextdV, make sure that you use the correct units of each quantity! Since we want the answer to be in joules, i.e. SI units, we need to substitute pressure and volume in Pascals and Cubic Meters.

 

Note : - The enthalpy change of a reaction is the heat exchanged in a reaction when a process is carried out at constant pressure. This, in our case, means that the Q and ∆H entries in column three should be same. [Have you convinced yourself why?] But there may be slight differences between the two entries, this resulting from the rounding off of conversion factors. e.g. 1 atm = 101325 Pascals, but we often substitute 1 atm as 1.01 lac pascals.

 

Third column done as well. :)

 

Column Four

 

(a)Pi  - ;)

(b)Tf - This question is the answer, "what is an isothermal process?"

(c)Pf- The ideal gas equation is OK, but observe that the RHS of it is constant. So, even the LHS would be. Hence, if the volume doubles up, the pressure reduces to half.

(d)Q - ∆E + ∫PextdV, now we will find both the terms and substitute them.

(e)∆E - Well, if the internal energy of an ideal gas depends only on its temperature, and if this is an isothermal process, where does it lead us?

(f)∫PextdV - This is a reversible process. As explained above, Pext is the same as the pressure of the gas molecules at any stage. Substitute Pext as nrt/v. Since the numerator is constant, we take it out of integration, and evaluate nrT ∫(dV/V) which is nrTln(Vf/Vi). Now substitute the values.

(g)∆H - nCp∆T :)

 

Column Five

 

No additional info. This is a clone actually.

 

Column Six

 

We expect the student to be seasoned enough now to realize that the gas parameters are found through ideal gas equation, and then the thermodynamic Joule values are found simply.

 

Note : Since the volume remains constant, change in volume is 0. i.e. dV=0, so ∫PextdV = 0

 

Column Seven

This is the first irreversible process that we are dealing with, in this table. Pf = 1 atm = external pressure in the above table means that the gas expands/contracts against a constant external pressure of 1 atm, and the final pressure of the gas too is 1 atm. [We have some numerical problems where the final pressure isnt equal to the external pressure against which expansion/contraction took place, but do not bother yourself with it at this moment.]

 

Note carefully that all three gas parameters are missing for the initial state. That means, apart from the gas equation, we will require two more equations to find them all. And since this is an irreversible adiabatic process, we cannot use P(V^y)=Constant over the initial and final states. That means we have lost an equation!

 

How are we going to deal with this situation? The first thing to keep in mind is that every problem asked in this book has a solution. And this table, like most of the highschool problems, is all about making equations to find unknowns.

 

(a)Vf - Use the ideal gas equation to get the value.

 

Now try to relate the final parameters to the initial parameters. Obviously, the heat gained, work done, change in internal energy put some constraints on the possible values of the parameters.

 

(b)Ti - ∆E is known to us, but if we write it as nCv∆T, and equate it to 1000 J, we actually end up with an equation. This gives us the value of ∆T, which can be use to find the initial temperature, because the final temperature is known.

 

(c)Vi/∫PextdV - Refer the two reversible adiabatic processes in column 1 and column 2 that we studied. We made a statement over there, that since the characteristic of any kind of adiabatic process is "zero energy exchange", ∫PextdV is always the additive inverse of ∆E for an adiabatic process. Hence ∫PextdV = -1000 J.

 

But this is not all!! If you are thorough with integration, look at the word ∫PextdV with some concentration. The parameter Pext would jump in and out of the integral in your mind's eye once you understand that it is constant for this process. This yields ∫PextdV =Pext(∆V). Just as you found Ti by finding ∆T, find Vi by finding ∆V.

 

Fill up the rest of the entries yourself.

 

Note: This is for you to ponder. In the reversible adiabatic processes,  ∫PextdV = -∆E and actually evaluating the integral gave the same equation. But in the case of an irreversible adiabatic process, evaluating the integral and using the first law to find its value gives two distinct equations! This can be used for our benefit in problems. Please keep this in mind!

 

Column Eight

 

Just as in Column Seven, we lose the equation ∫PextdV = nrtln(Vf/Vi), but we can write it as ∫PextdV = Pext(∆V).

 

An irreversible isothermal process is much shorter than an irreversible adiabatic process to complete.

 

(a)Vf - ∫PextdV = Pext(∆V) use this equation to find the final volume of the gas molecules.

 

(b)Tf - Two gas parameters are known now, use the ideal gas equation to find Tf. And if this is an isothermal process, what does knowing the final temperature mean?

 

The rest of the entries require no new logic, everything has been covered in the above columns.

PhChem/thermo1/Problem1

Read the paragraph in "The Setup" section and answer the questions that follow it. This question is supposed to replace a theory part explaining obvious facts with an interactive session.

The Setup

A horizontally aligned cylinder is divided into two sections by a vertical massless frictionless sliding adiabatic piston. Both the left and the right sections have one mole each of an ideal gas (whose molar specific heat is 20 J/mol K) at 300 K and 2.0 L. The ideal gas in the right section is maintained at a constant temperature by means of a diathermic contact with some external agent. The ideal gas in the left section, however, is in contact with a heater, which heats it slowly so that the left section expands. By the time the heater stops supplying heat, the volume of the right section has decreased to 1.0 L.

Now answer the accompanying questions.

• Note - Before solving the problem, make sure that you understand the whole of it. This problem is of intermediate level. Like most questions in physical chemistry, it seems tough only because of its length. To understand the whole scenario in this (and every other) question, note down the necessary data and diagrams where you are solving the problem. Doing so will familiarize you with the problem, and give you some mental exercise.
• So before you start to solve, draw a cylinder resting on its curved surface, divided into two parts. To each part assign the values of the gas parameters, and note which section will be heated and which one will be maintained at a constant temperature.

The Questions

Question 1

What process is the gas in the right section R undergoing?

Since it is maintained at a constant temperature, it is undergoing an isothermal process. Also, since the volume change is taking place slowly (quasi-statically), it is a reversible process. It is thus, a reversible isothermal process. The diathermic contact with an external agent just means heat can be exchanged between section R and the external agent. This is used to maintain the constant temperature in section R.

Question 2

What is the significance of the adiabatic piston in between Sections L and R?

The adiabatic piston ensures that heat does not flow between the two sections. That is, the heat supplied to section L is utilized in heating the gas and doing PV work only, and is not passed on to section R.

Question 3

What is then, the work done by the gas in the section R?

Use the expression/formulae for isothermal process ∫P_extdV = nRTln(Vf/Vi). All of the values are known, n=1, T = 300 K constant, Vf = 1.0 L and Vi=2.0 L. The answer comes out to be -1.73 x 10^3 J. Since by IUPAC convention, work done by the gas has the same magnitude but opposite sign as ∫P_extdV, the final answer is 1.73 x 10^3 J.

Question 4

∆E and q for the gas in section R are ___J and ___J respectively.

Well, in an isothermal process, ∆E is always zero, because for an ideal gas, Internal energy depends only on temperature, and the temperature remains constant. q is to be found using the First Law Of Thermodynamics, and it comes out to be -1.73 x 10^3 J

Question 5

When the heating stops, the piston stands still. What does this tell us about the pressures of the ideal gases in the two sections?

If the piston stands still, it means it is in a state of mechanical equilibrium. (Can you explain satisfactorily the difference between mechanical and thermodynamic equilibrium? Refer any textbook or Wikipedia and note the answer just now if you cannot.) This makes us conclude that the net force acting on it is zero.

In other words, the force from the gas in section R and the force from the gas in section L are same in magnitude and opposite in sign. Equating their magnitudes, P_r x Piston Area = P_l x Piston Area. That is, the pressures of ideal gases in both the sections are same! This is a very important result, do remember how we concluded it.

Question 6

If the piston had some finite mass, and the cylinder was aligned vertically, would the pressures be same in case of equilibrium?

Definitely not! You can draw a free body diagram of the piston and check. The ideal gas in the bottom section has to counterbalance the force due to the gas above, as well as the gravitational force acting downward. This means, the gas below will be at a higher pressure, by an amount (Piston Mass)g/(Piston Area) pascals. (Convince yourself about this point.)

Question 7

By performing actual integration, find the value of ∫P_extdV for the gas in Section L.

This is a very nice question, because it helps to clarify the significance of each and every variable. P_ext for the gas in section L is nothing but the pressure against which it is expanding. This pressure is the pressure of the gas in section R.

∫P_extdV = ∫P_rdV_l

Now we substitute P_r as nRT_r/V_r.

∫P_extdV = ∫P_rdV_l = ∫(nRT_r/V_r)(dV_l) = nRT_r∫(dV_l)/(V_r)

(The numerator was taken out of integration, because it is a constant)

Now comes the main step of the question. Can we integrate the variable V_r with respect to the variable V_l? Definitely not. So we substitute the variable V_r as [4.0 L - V_l] and carry out the integration from limits 2.0L to 3.0 L. This is because, the total volume of the cylinder will remain constant at 2.0 + 2.0 = 4.0 L, and if the volume of one section increases by some amount, the volume of the other section decreases by the same amount.

(The substitution V_r = 4.0L - V_l is similar to the case of evaluating ∫P_extdV for an ideal gas in an isothermal process. There, since we could not integrate P wrt V, we substituted it as nRT/V)

The definite integration yields the value of the integral to be (nRT_r)(-1)(ln[{4-3}/{4-2}]) = nRT_rln2 = 1.73 x 10^3 J same in magnitude, but opposite in sign, as ∫P_extdV for section R. Note this point carefully.

Question 8

We substituted P_ext = P_r = nRT_r/V_r. = nRT_r/(4.0 - V_l). But we do know that since this is a quasi static process, the piston is always in mechanical equilibrium and hence the pressures of gases in both the section are always same. So why did not we substitute P_ext = P_r = P_l = nRT/V_l?

Definitely we could have done this. But take a look back at Q7. The numerator nRT_r was constant an could be taken out of integration. Is this the case with nRT_l as well? No. We would again need to substitute T_l as some function of V_l, so that we could carry on with the integration. All in all, what we did was correct.

Question 9

Prove that the values of ∫P_extdV for the two sections are always going to be the additive inverse of each other for this scenario.

Consider ∫P_extdV_r + ∫P_extdV_l. To prove our point, we will prove this expression to be zero, no matter what. The first point is, as we discussed above, the P_ext for one section is the pressure of the ideal gas in the other section. Now, both are equal at any point of time in our case (because the process is quasi static, both mechanical and thermodynamic equilibrium are maintained at every stage. Convince yourself that this statement leads to our conclusion.)

Also, we have already proved before that the change in the volume of one section is the additive inverse of the change in the volume of the other section. See Q7, and by the way, this is obvious. So,

dV_r = -dV_l.

Hence, ∫P_extdV_r + ∫P_extdV_l. = ∫P_extdV_r - ∫P_extdV_r = 0.

Question 10

Find the work done w by the gas in section L, its ∆E and the heat supplied by the heater.

• w = - ∫P_extdV = -1.73 x 10^3 J
• ∆E = nCv∆T. Now finding the change in temperature is a different task altogether. But use the skills that you derived from solving the table. The initial temperature is known to us. But for the final state, we only know the volume. The temperature and pressure are not known. We need two equations then. The first is the ideal gas equation. The second is obviously, P_l = P_r. = nRT_r/V_r.
• Substitute this value of P_l in the ideal gas equation (do not obtain the numerical value, it will just increase some calculation). After a lot of cancellation (to our satisfaction), we obtain T_l = 600K. n=1 moles, Cv = 20.0 J/K mol and ∆T = 300K. This gives the answer to be 1.20 x 10^4 J
• The heat supplied by the heater is nothing but q for section l, which is to be found using the first law of thermodynamics and is found equal to, after rounding off, 1.4 x 10^4 J.

PhChem/thermo1/Splmntry

Question 1

1.00 mole of helium gas is allowed to expand from 22.4L to 44.8L isothermally at 273.15 K. The expansion is free expansion type. The external pressure against which the gas is expanding is zero. In other words, the gas is expanding in vacuum. Find the values of q, w, ∆E and ∆H for this process. Assume ideal gas behaviour.

The solution

Although it might seem tiring to find the values of four parameters, a thorough practice of filling up the table we discussed before might make this an obvious mechanical task. Since the process is an isothermal process, the temperature change is zero. And since we are to assume ideal gas behavior, ∆E and ∆H are zero. This is because, for an ideal gas, these two parameters depend solely upon the temperature. Secondly, since the gas is expanding against zero pressure, it is offered zero resistance. Hence, no work is one by or upon the gas. If this sounds unconvincing, consider ∫P_extdV. Since the external pressure is constant, we take it out of the integral, and since the external pressure is zero, the whole term is zero by itself.

w, then, too becomes zero in value.

Q, that is, the heat exchange in the process also has to have the value 0, according to the first law of thermodynamics. Have you noted that it never mattered what the gas was, or what its gas parameters were, so long as we considered it to be ideal? In the question, we have mentioned various data regarding the gas, none of which were used in the solution.

Synopsis

• P=0, so ∫P_extdV = 0
• Using the first law of thermodynamics, we showed that q = 0 as well.

Concluding Notes

Note the whole discussion as, “An isothermal free expansion of an ideal gas is also adiabatic”.

Is the converse true? Is an adiabatic free expansion of an ideal gas isothermal too? Think in terms of conservation of energy. If the idea doesn’t seem obvious, use the expression for the first law of thermodynamics.

Question 2

Two moles of a monoatomic ideal gas occupy a volume of 30 L at 300 K. The gas is allowed to expand to 50L at a constant external pressure of 550 mm Hg adiabatically. Find the work done by the gas and the final temperature of the gas. Keep in mind that the final pressure of the gas is not necessarily equal to the external pressure it expands against.

A little advice

While solving Physical Chemistry, a problem either seems familiar or it does not. And to make sure it does seem familiar in an examination, one should be able to dissect the data given and the scenario into fragments which have been previously dealt with.

Working on these grounds, we realize that we have already solved some problems dealing with irreversible expansion. The only difference between them and this one is that in the latter, we do not know that final pressure of the gas. Isn’t it? Where does it lead to? A simple thought says that we have another unknown, finding which would require an extra piece of data. Indeed, compare this problem with the previous ones from the table, and you’ll realize we have more data here.

The Solution

Here we go. The work done by the gas is nothing but -∫P_extdV when we are in the IUPAC domain.

We now evaluate the integral. Since the pressure is constant, we take it out of the integral sign and the remaining integral amounts to ∆V. We know the change in volume of the gas, and we know the external pressure against which it has expanded. Hence, the work done by the gas is P∆V = (550/760 atm) x (20 L) atm-litres = (550x101000/760) x (20/1000) J =. 1462 J. (Check your level – are you thorough with unit conversions?). w is then equal to -1462 J.

Also, since it is an adiabatic process, ∫P_extdV = - ∆E = w. This is the second step we’re performing. (Can you explain this using the conservation of energy? Since the heat supplied is zero, whatever work is done by the gas is done at the cost of its internal energy.) Equate the change in internal energy to nCv∆T and you’ll find the change in temperature. The number of moles are two, and the Cv is 1.5R (monoatomic ideal gas). This is enough to find the final temperature. The mistake you could have made was finding the final temperature using the ideal gas equation with 550/760 atm as the final pressure.

The final answers are -1462J and 242.4K.

Synopsis

• Evaluated the ∫P_extdV as P∆V and found one answer, w
• Equated ∫P_extdV to –nCv∆T (w = nCv∆T) and found T_f

Concluding Note

Make it a general approach to assume the final pressure not being equal to the external pressure against which the system has expanded in cases of irreversible processes, unless specifically mentioned otherwise. This will help in most questions. Since these questions are easy going by the concepts, and still, mistakes can be made, they are among the deciding questions in exams like AIEEE or even the JEE.

Question 3

One mole of a monoatomic ideal gas occupies a volume of 11.2 L at 273K. The gas is allowed to expand by 22.4L at a constant external pressure of 1 atm isothermally. Find the work done by the gas. The final pressure of the gas is not necessarily equal to the external pressure it expands against.

The Solution

This is basically the same question as above. There may be a few on-the-surface differences, but nothing more than that. The work done, as we know, is nothing but -∫P_extdV = -P∆V = -[1 atm x 22.4 L atm-litres] = -(101000 x 22.4 x 10^-3) Joules = -2262.4 J. The final pressure, can be found using the ideal gas equation, of course, the final temperature is 273K (this is an isothermal process).

Synopsis

• Evaluated P∆V, and answered the value.

Notes

One mistake that people may make is that the gas expands by 22.4 L, not that the final volume is 22.4L. In other words, the data is ∆V = 22.4 L. This mistake should be avoided, because in most competitive objective exams, an option leading from this described mistake will also be put up. Falling prey to that option means that you failed to gain easy marks, and at the same time losing marks which a person not attempting that question wont. This leads, ultimately, to losing ranks.

Question 4

n moles of an ideal gas with molar specific heat Cv is taken from initial state T₁/ V₁ to final final volume V₂ through a reversible adiabatic process. Show that the value of ∫P_extdV is

$nCvT_{1}\left[1-\left({\frac {V_{1}}{V_{2}}}\right)^{\frac {R}{Cv}}\right]$

First Thoughts

To many students, this problem might seem too obscure to induce any desire to solve it. Others might think it is familiar but would not attempt the problem just because of an unknown fear. Relax. The value of ∫P_extdV is nothing but –nCv∆T = nCv(T₁ - T₂).

Our approach would be substituting each and every unknown we encounter, in the hope that if we do not make any mistakes, nothing can go wrong.

The Solution

Write down the required quantity, nCv(T₁ - T₂), and start manipulating it step by step. Take T₁ out of the bracket first, because that is exactly the term that we are supposed to generate.

Now, we are left the bracketed term beside nCvT₁. As we have mentioned before, if we do not make any mistake, we are sure to go home. This means that the complex looking bracket in the question, simplifies to 1 - (T₂)/(T₁). But how? We have done this several times before, while dealing with reversible adiabatic processes: the relation between the Temperature and Volume of the ideal gas at any stage in an adiabatic process, which gives us (T₂)/(T₁) = [(V₁)/(V₂)]^(y-1), where y stands for the adiabatic exponent of the gas.

(Do you remember this? PV^y = constant, and PV = nRT. On eliminating pressure from these two equations, we arrive at the relation TV^{y-1) = constant, giving T₂)/(T₁) = [(V₁)/(V₂)]^(y-1) when applied over the initial and final states. Please refer back to the tables, if you do not know this.)

But, the adiabatic exponent does not appear anywhere in the final term. So? Eliminate it, obviously. We know the molar heat capacity, and can report Universal Gas Constant as well. Y-1 is nothing but (Cp/Cv) -1 which simplifies to R/Cv, just what we need.

Synopsys

• Used (T₂)/(T₁) = [(V₁)/(V₂)]^(y-1) to eliminate T₂
• Used y = Cp/Cv to eliminate the adiabatic exponent, y itself.

Concluding Notes

Do not be bamboozled by the perplexity of any problem, just try to make it seem easy.

PhChem/thermo1/splmntry2

A gas contained in a cylinder fitted with a fractionless piston expands against a constant external pressure of 1atom from a volume of 5litres to a volume of 10 liters .During the process the system absorbs 400J of thermal energy from its surroundings.Determine ∆E for the process (R=8.314Jpre mole per kelvin and 1atom =101325NM^-2

First thoughts

What is this process? Well, it is a phase change of water. Since heat transfer is involved, q, ∆E and ∆H are also associated with this process. How does work w come into being? Actually, the system in question is undergoing a change in volume as well against a pressure. Hence, some work must have been performed.

The Solution

q/∆H - Note that this process has been carried out at constant atmospheric pressure. So, the heat involved q would be nothing but the enthalpy of the reaction. Please make a note that the standard enthalpy quoted here is for 1.00 moles, and for vaporization. Here, we are dealing with 10.00/18.00 moles of water vapour which has to condense. So, the ∆H is 5/9 times of the standard enthalpy in magnitude and opposite in sign.

That is, q = ∆H = (- 5/9)(∆H_vap) = -22.6 kJ. This is obvious, heat should be taken out of the system if it has to be condensed.

W - What is the work done? Consider ∫P_extdV. Since the pressure is constant, it comes out of the integral, and we are left to evaluate P∆V. Now, this is another important point to note. The water vapour would occupy 17.01 L (according to the ideal gas equation), while the water droplets would occupy somewhere around 0.010 L. (assuming the density of water to be 1gm/ml at 100 ^oC). The change in volume is approximately equal to the volume of the water vapour itself.

Hence, in most condensation/vaporization problems, we never bother to find the change in volume. We assume liquids to have negligible volume in comparison to vapours. Even in our case, we will not take the volume of water into account because it is negligible, and because it is calculated through assumptions.

Hence, P∆V amounts to P (=1atm) x ∆V (=17.01 L) = 17.01 atm-L = 17.01 x 101000 x (1/1000) = 1718 J. w = -∫P_extdV = -1718 J

∆E - Now we find ∆E. According to the first law of thermodynamic/Conservation of energy, ∆E = Q – ∫P_extdV = Q + w = -22.6 kJ – 1718 J = -24.3 kJ

Please note that the value of w is in the order of joules, while that of ∆H is of the order of kilojoules. Do not make any mistakes while adding them.

Synopsis

• q and ∆H were equal for this process because it was carried out at constant pressure.
• w for this process was calculated using –P∆V
• ∆E would have been trickier, if going by the popular misconceptions. However, it was calculated through the first law of thermodynamics, since the other two parameters are already known.

Question 2

Water expands when it freezes. Determine the amount of work done in Joules when a system consisting of 1.0L of liquid water freezes under a constant pressure of 1.0 atm and forms 1.1L of ice.

Solution

There has to be no confusion with this problem. The work done is –∫P_extdV = -P∆V = 1.0 atm x 0.1 L = -[1 x 101000 x 0.1 x (1/1000)] = -10.1 J

Notes

Not many examiners will ask this question directly. Most spin-offs will involve the calculation of the volumes and the first law of thermodynamics.

Question 3

When 1 mole of ice melts at 0 ^oC and at constant pressure of 1 atm. 6050 joules of heat are absorbed by the system. The density of ice and water are 0.92 gm/ml and 1.00 gm/ml respectively. Calculate ∆H and ∆E for the reaction in joules. Report the exact values.

First Thoughts

If you have read the concluding notes of the previous question, then you will find that this is exactly what we were talking about. ∆H, as in question 1, is equal to the q of the reaction because this is a constant pressure reaction. ∆E has to be found using the first law of thermodynamics, for which we also require w.

Just as we discussed above, we have not been given the volumes of the two states directly. Rather, we have to find them out using their densities. This is not much of a big task, but complicates the problem nonetheless.

Solution

∆H – Since the process is carried out at constant pressure, the enthalpy change of the reaction is the same as the q value. Q is 6050 J in magnitude, and since heat has been absorbed by the system, it has a +ve sign. So, ∆H = q = +6050 J

∫P_extdV – The integral again simplifies to P∆V. All we have to do now is to find the change in volume. We are dealing with 1 mole of water, which is 18 grams of mass. So, the volume is 18 grams / 0.92 g/ml = 19.56 ml ice and 18/1.00 = 18 ml water. ∆V is then -1.56 ml.

P∆V = -(1 atm x 0.00156 L) = -0.00156 atm-l = -0.16 J

∆E – According to the first law of thermodynamics, it comes out to be 6050.16 J. However, we do not report such values because of the use of significant figures.

Synopsis

• Found ∆H
• Calculated ∫P_extdV for use in the first law expression to ultimately find ∆E.

Concluding Notes

Physical chemistry problems, generally, are easy and use the same concept/logic. In most of the cases, if they are complicated, it is just because of the presented data. Also, this question wasn’t the best example of how this is done. Be prepared for more.

Question 4

A cylinder contains 10 litres of an ideal at 25 atm and 25 ^oC. However, the gas leaks out due to some malfunctioning. The atmospheric pressure is exactly 1 atm and temperature 25 ^oC during the entire leak. Assuming that the process is isothermal, how much work is done on the atmosphere due to the leaking gas?

First thoughts

This is a nice variation of the questions we have been doing. When the gas leaks out at constant temperature, it actually undergoes an isothermal expansion. A part of our system has vanished in the atmosphere, while the remaining is still inside the cylinder. This is intriguing, as we have been dealing with disciplined processes, where, unlike here, the system under question was at one place.

So, all we are left to do is solve the problem with the numerical values.

Solution

The gas will leak out of the cylinder till the pressure in the cylinder has reduced to 1 atm. Since the temperature of the gas is constant, PV = constant for the gas. Now, note that, the gas in the cylinder will be at 1 atm and the gas leaked out will also be at 1 atm. That means, the pressure has reduced 10 times. The volume, therefore, has increased ten times and has become 250 litres. The work done by the gas is then -∫P_extdV = -(1 atm) x (250 L - 10 L) = -240atm-L

The work done on the atmosphere because of the leaking gas will have exactly the same magnitude but opposite sign as above. (To do – Explain this point properly, and in detail) Hence, the final answer is 240 atm-litres = 24240 J

Synopsis

• Found the volume of the gas after the leaking has stopped.
• Evaluated ∫P_extdV for the gas
• The last point.

Question 5

A magnesium strip of mass 20 gm with 20 percent inert impurities is dropped into a beaker of dilute hydrochloric acid. Calculate the work done by the system as a result of the reaction that takes place. The atmospheric pressure is 1.0 atm and temperature 25 ^oC. The molar mass of magnesium is 24.3 grams.

First Thoughts

Again, this is a different situation. Until now, we have been dealing with processes over ideal gas, but this is something different. Actually, the reaction that will take place is a redox reaction. The magnesium in the strip will be oxidized to magnesium ions, while some of the hydrogen ions in the acid will be reduced to molecular hydrogen. This gas will then occupy some volume, which is different from the previous volume occupied by the system. Hence, ∫P_extdV is involved yet again.

Also, note that the amount of acid taken is assumed to be in excess, so that all of the magnesium is oxidized, while still leaving some hydrogen ions.

Solution

For each mole of magnesium ion produced, one mole of molecular hydrogen H₂ is produced. (DIY – write down the redox reaction. This is a fairly simple one, and after the statement above, no complexity is involved whatsoever.)

The question is, how many moles of magnesium are actually reacting? The mass of the strip taken is 20.00 grams, which contains 20 percent inert impurities. So, the mass of magnesium reacting would be 16 grams, which amount to 16/24.3 = 0.66 moles of magnesium. The impurities are inert, and hence do nothing in the reaction. They have to be ignored completely.

In this condition, 0.66 moles of hydrogen will occupy 16.15 L. This volume is in fact, the increase in volume of the acid beaker + strip system. Hence, ∆V = 16.15 L. P∆V = 16.15 atm-ltres = 1631 J. w = -∫P_extdV = -1631 J

Synopsis

• Found the mass of magnesium reacting
• Found the moles of hydrogen gas produced, and the volume it will occupy.
• This volume is equal to ∆V.
• Evaluated ∫P_extdV as usual, and reported with appropriate signs

PhChem/thermo1/advsiso

Question 1

Which of the two – adiabatic or isothermal – processes has a steeper PV graph?

Instructions

If we are talking about the nature of a graph, we need to consider the mathematical nature of it too. So, an adiabatic process is represented as PV^y = constant on a PV graph, while an isothermal process is represented by PV = constant.

Carefully note that the graph of the isothermal process is a rectangular hyperbola on a PV graph, while that of an adiabatic process resembles a hyperbola.

Now, the question is, which Process takes a greater dip down in the PV curve? Visualize what is being asked. Take the question literally. If we are talking about an expansion, which process will register a greater dip in pressure for the same amount of increase in volume? The answer can be obtained by merely looking at the equations.

Arguments

Suppose the volume increases by some factor, say m times. In an adiabatic process, the pressure then HAS TO dip by a factor of m^y to keep the LHS constant. Do the math for an isothermal process, for an increase in volume by a factor of m times, the pressure dips down by the same factor. This means, that the pressure registers a greater dip in adiabatic process than in an isothermal process for the same volume. Note that this is irrespective of the value of the constant on the RHS. 

Try to grasp what has been said, and draw an accompanying graph to this point in your notebook.

Important Note

Remember that m^y is greater than m only if y>1. This is always true, because the adiabatic exponent of any gas is always greater than unity. But suppose, if we were dealing with a process like PV^1/2 = constant, its graph would be less steep than the isothermal process.

Are you confused? Please do not be. Read the note below, and move ahead only after you are sure that you got the essence of what has been said.

Concluding Notes

This is not an uncommon logic, and a common sense dealing with them should evolve in you during an introductory course in the sciences. The whole logic explained above is nothing but one line, what LHS would tend to raise more?

Now suppose, if you are given adiabatic curves of two ideal gases, one monoatomic and the other diatomic, initiating through the same state and registering the same increase in volume. How would you tell which graph belongs to which gas? In other words, what gas would end up with a lower pressure and what would end up with a higher pressure and why?

The answer, of course, is the monoatomic gas will have the lower pressure. But it is up to you to convince yourself about this using the same logic discussed above.

Hint : The value of gamma for the monoatomic gas is greater than that for a diatomic gas.

PhChem/thermo1/problem2

The Setup

Six reversible thermodynamic processes are carried out on one mole of ideal gas in succession to complete a cycle. The processes are two alternate isothermal and adiabatic expansions followed by an isothermal compression and an adiabatic compression to reach to the initial state. The volume changes twofold in the isothermal expansions. Also, the isothermal processes take place at temperatures T1, T2, T3 respectively. The adiabatic exponent of the gas is y.

Questions

Question 1

After the cycle is completed, what is the change in the internal energy of the ideal gas?

No. No need to freak out. The internal energy of the ideal gas depends only on the temperature. After the cycle is completed, the gas returns to its original state – the initial pressure, volume and temperature. That is, after the whole cycle, the temperature does not change and hence, the change in the internal energy ∆E is zero.

This is the consequence of internal energy being a state function.

Question 2

Draw a rough sketch of the whole cycle on a PV graph, clearly showing the six processes.

Is this a difficult question? No. We do know what the graphs of the two processes are shaped like.

The task left, then, is to draw six curves – three of a definite steepness and three of some other definite steepness – one by one such that they form a closed loop. Consider drawing it yourself, and then take a look at the answer below. While sketching, we needed to keep only one point in mind, what graph would be steeper?

Note : The vertices have been labelled for later use. The direction is ABCDEF initiating at A and terminating at F

Since we know the answer to that question, it is relatively easier for us to draw the curve now. Refer to the point on graphs of adiabatic and isothermal processes

Question 3

What is the work done by the gas in every cycle? What is the total work done by the gas?

This becomes a fairly easy problem. The reason is that the work done by the gas in the adiabatic process is nothing but -nCv∆T. From the graph, we find that ∆T in each case is T₂ - T₁, T₃ - T₂ and T₁ - T₃ respectively. Cv is R/y-1. So, the adiabatic processes are dealt with.

Work in isothermal processes

The task reduces to finding the work done in the isothermal processes. Make a mental note that the isothermal processes perform a work –nCvln(V₂/V₁). Do we know each of the value substitutions? Yes. We know n, Cv, the temperatures at which the isothermal processes take place, and the volume ratio = 2 for the expansions. The only unknown is the volume ratio to be substituted inside ln in the isothermal compression. What is this ratio? And how are we to find it?

Finding the required volume ratio

Recall that for an unknown, we need to create an equation. And most of the times, an equation is created because some variables are restricted to some values by a certain law.

Just remember that the initial and final volumes and temperatures (and all intermediate states) in an adiabatic process are constrained to satisfy T₁V₁^y-1 = T₂V₂^y-1. This is the constraint we were talking about.

Let us mark the vertices of this curve as A, B, C, D, E, F respectively, A being the initial point of the T₁ isotherm. Then, what we are to find is the ratio V_E/ V_F.

Here are the three equations: -

• V_B/V_C = (T₂/T₁)^[1/(y-1)]
• V_D/V_E = (T₃/T₂)^[1/(y-1)]
• V_F/V_A = (T₁/T₃)^[1/(y-1)]

Multiplying all three equations, we get the required ratio to be 4. (The RHS after multiplication will reduce to 1, always make a mental note of such cyclic expressions. On the LHS, you will encounter two known ratios an substitute their value as 2.)

Final Answer

• W_AB = -nRT₁ln2
• W_BC = -n[R/(y-1)](T₂ - T₁)
• W_CD = -nRT₂ln2
• W_DE = -n[R/(y-1)](T₃ - T₂)
• W_EF = -nRT₃ln0.25 (Because this is a compression)
• W_FA = -n[R/(y-1)](T₁ - T₃)

Since Cv was not known, we had to express it in the terms of the known adiabatic exponent y. The value of n is one mole, but it has been reported to keep harmony with dimensional formulae.

Synopsis

• Found the work done in adiabatic prcess as –nCv∆T
• Found the work done in isothermal process as -nRT ln(V₂/V₁)
  • Found the ratio V₂/V₁ for the isothermal compression using the condition of reversible adiabatic processes to generate three equations, and multiplying them.

Question 4

Find the heat exchange of the ideal gas in each step.

This is a mechanical step. All you have to do is use the first law of thermodynamics.

Final Answer

• Q_AB = nRT₁ln2
• Q_BC = 0
• Q_CD = nRT₂ln2
• Q_DE = 0
• Q_EF = nRT₃ln0.25 = -2nrtT₃ln2
• Q_FA = 0

Question 5

What is the efficiency of the process?

Now, the efficiency of the process has to be thought of as the ratio of total output and total input. In thermodynamic processes, only the net work done (∫P_extdV over all the processes, not the IUPAC w) qualifies as output. We will NOT consider heat rejected by the system as output.

The input, on the other hand, is not the net heat exchange. It is rather the heat given to the system. The heat rejected by the system does not occur anywhere in the numerator or the denominator.

Then, the output is nR(T₁ + T₂ - 2T₃)ln2. The input is nothing but Q_AB + Q_CD = nR(T₁ + T₂)ln2.

We did not count Q_EF because it was the heat rejected by the system.

The final answer is the ratio ${\displaystyle }$