Electronic Properties of Materials/Quantum Mechanics for Engineers/The Stern-Gerlach Experiment

We discussed in the first chapter a list of historical experiments that highlight the origins of quantum mechanics. In this lecture, I want to present one final experiment. The experiment itself just showed the origin of spin and orbital quantum numbers, but we're going to have to take it a step further and discuss a thought experiment that will demonstrate the fundamental working of quantum mechanics.

The Experiment

Stern–Gerlach experiment: Silver atoms travelling through an inhomogeneous magnetic field, and being deflected up or down depending on their spin; (1) furnace, (2) beam of silver atoms, (3) inhomogeneous magnetic field, (4) classically expected result, (5) observed result

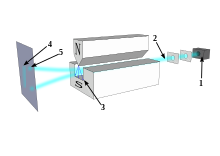
As it happens, for reasons we will discuss during the second half of this class, the Silver (Ag) atom has a very simple magnetic nature. Each atom can be treated as a little dipole with magnetic moment $\mu$.

<EXPLANATION OF EXPERIMENT>

The force on a magnetic moment is:$$F=\nabla (\mu \cdot B)$$

In the z-direction:$$F_{z}={d \over dz}(\mu \cdot B)=\mu _{z}{dB_{z} \over dz}$$

The deflection of the Ag atom is proportional to the z-component of $\mu$.

Expected Results

Based on this, we expect to see atoms of all different orientations of $\mu$, and random magnetic moments, spread out in a single distribution.

<FIGURE> "Classic Theoretical Results of the Stern-Gerlach Experiment" (Atoms are of all different orientations of u, and there is a single distribution across the screen, centered on the main axis.

But this is not what we see...

Actual Results

Rather, we see two separate distributions on either side of the main beam.

<FIGURE> "Actual Results of the Stern-Gerlach Experiment" (Two separate distributions, not on the main axis, are seen instead of the single, classically predicted, distribution.)

As it happens, in quantum mechanics, magnetization is tied to angular momentum. (This of electrons zipping about in a circular orbit.) In Gold we are only looking at the spin of an electron. The directional component of ${\textstyle S}$, say ${\textstyle S_{z}}$, can only take two values, "up" ${\textstyle \left({\hbar \over 2}\right)}$, or "down" ${\textstyle \left({-\hbar \over 2}\right)}$. What we just did was measure ${\textstyle S_{z}}$ of the Silver atoms (electrons?), and separated them into two beams, one with spin-up and the other with spin-down. Is this shocking? Yes. We just took a randomly oriented vector, ${\textstyle S}$, and measured it's projection, ${\textstyle S_{z}}$, and found it could only take two values.

Explaining Quantum Mechanics

Let's keep going. Now that (in principle) we can make a simple measurement we can make a series of thought experiments. Let's pass a beam through a filter, and see what happens...

<FIGURE> "Explaining Quantum Mechanics: The $SG_{\hat {z}}$ Box" (Some beam, $A_{g}$, enters the box, $SG_{\hat {z}}$, and is separated based on up and down spin.)

Let's take some beam, $A_{g}$, have it enter the $SG_{\hat {z}}$ box which separates the beam based on up and down spin. If we take the output from $SG_{\hat {z}}$ measurement, discard the up elements, and remeasure down beam, the resulting beam will still be "down". This is good, no surprise here as this follows with classical logic.

<WHAT IS THIS>

Hypothesis - Polarized sunglasses all y-components are discarded.

1. Not 50/50 in polarized light.
2. Try rotating the box...

Now let's try rotating the ${\textstyle SG_{\hat {z}}}$ box into an ${\textstyle SG_{\hat {y}}}$ box. The $A_{g}$ beam is still being split into up and down spin by the first $SG_{\hat {z}}$box, but now that down group is being filtered based on an ${\textstyle SG_{\hat {y}}}$ box, which is an $SG_{\hat {z}}$ box that has been rotated 90°.

<FIGURE> "Explaining Quantum Mechanics: The $SG_{\hat {y}}$ Component" (Note that the $SG_{\hat {y}}$ box is the same as the $SG_{\hat {z}}$ box, just rotated 90° to measure the y-component of the vector $S$.)

It looks like both boxes have a base probability of 50/50 for up or down spin. Does this make sense? Maybe?

<FIGURE> "Title" (Description)

Now we filter ${\hat {y}}$ to be either up or down 50/50 probability?

Something seems wrong with this picture...

Let's run one more experiment. This is the same as <FIGURE>, but now the up group coming out of the ${\textstyle SG_{\hat {y}}}$ box is again filtered through an ${\textstyle SG_{\hat {z}}}$ box. Looking at the problem, this should result in 100% down spin as the elements were tested to be 100% down spin before they entered the ${\textstyle SG_{\hat {y}}}$ box, but this is not what we see here. Instead the elements coming out of the second ${\textstyle SG_{\hat {z}}}$ box are 50/50 up and down spin.

<FIGURE> "Explaining Quantum Mechanics: The second $SG_{\hat {z}}$ box." (Now the $SG_{\hat {y}}$ up beam is filtered through a second $SG_{\hat {z}}$ box.)

This is definitely weird. $S$ is just some vector. If you measure the sign of $S_{z}$, and you can measure it again and again and again, it doesn't change. BUT after you go and measure $S_{y}$, if you look back at $S_{z}$ it has once again randomized. Classically, this is like taking a bunch of marbles and splitting it into red and blue marbles. You then split the blue marbles in to large and small, but when you look back at the pile, half of the blue marbles have changed into red!

Why does this happen?

The components of $S$ are "incompatible", as we can only know one component at a time. Before we measure $S_{z}$ we can say that the atom's wave function is in a "superposition" of being up and down. By using Born's probabilistic interpretation, or psi wave, we know that the odds of measuring up or down is 50/50. We measure $S_{z}$ and the psi wave "collapses" to $\phi _{S_{z}up}$or $\phi _{S_{z}down}$, depending on the measurement. Subsequent measurements have 100% chance to repeat the initial measurement according to the probabilistic interpretation of $\psi =\phi _{S_{z}down}$. In $\psi =\phi _{S_{z}down}$, the system is in a superposition of being $S_{y,up}+S_{y,down}$. If we measure $S_{y}$ and find $S_{y,up}$, then we cause the wave function to collapse to $\psi =\phi _{S_{z}up}$. In this state we have no information about $S_{z}$. We lost the information we had measured earlier when psi collapsed into $\phi _{S_{y}up}$.

In the next section we will go over the formalism of quantum mechanics, and will readdress the Stern-Gerlach experiment mathematically.