Electrodynamics/Electric Field
Electric Fields
[edit | edit source]The electric field is a physical field that is produced by electrically charged objects and which affects the behaviour of charged objects in the vicinity of the field. The electric field extends indefinitely throughout space and describes the electric interaction.
Using Coulomb's Law, we can place point changes anywhere in relation to other charges, and determine the force on that point change. The electric field is a vector field where every single point in space is assigned a force vector, depending on the field's effect on the point charge.
Mathematical Representation
[edit | edit source]There are different mathematical ways of representing the electric field. The first one views the electric field as a three-dimensional vector field. This vector field has a value defined at every point of space and time and thus is often regarded as a function of the space and time coordinates. As such, it is often written as E(x, y, z, t). If the field is electrostatic, it is customary to leave the t term out of the description. Also, if we are using vector notation instead of Cartesian coordinates, we can write the field as:
The electric field is defined as the limit of the force on a point charge q, divided by the value of the point charge, as the charge goes to zero. Mathematically:
We take the limit for a very important reason: if we add a charge to our system that is greater than 0, then the test point charge will affect the system and change it. We take the limit and reduce our test charge to zero so that it doesn't affect the rest of the system.
Using our charge density function ρ, we can calculate the force on a point charge q induced by a large object with uniform charge (such as a charged dielectric). We do this by extending our version of Coulomb's Law for n charges to a similar law with continuous charge distribution:
At each point r of the field, there is an associated force given by Coulomb's Law.
Electric Field (due to point charges)
[edit | edit source]Another way to consider the E field, instead of an abstract mathematical equation, is to consider a single point charge. We place the point charge into the field at various locations, and "measure" (using Coulomb's Law) the effect of the field on the point charge. We can use these measurements to draw lines of equipotential, lines where the force on the point charge is equal.
We use an infinitely small point charge in making these measurements because the addition of a non-infinitesimal charge to a system would affect the field, and would place a force on the other particles in the field.
Electrostatic Fields
[edit | edit source]If the electric field (E) is non-zero, and is constant in time, the field is said to be an electrostatic field. For instance, if the following relationship holds true, the field is electrostatic:
If this equation holds for all values of x, y, z, t1 and t0, then the field is electrostatic. If this equation does not hold true, then the field changes with time and it is called electrodynamic.
Notation
[edit | edit source]We know that the electric field is a function of position (and possibly time), and so it is customary in most applications to drop the arguments all together. We will denote the electric field with a bold letter E throughout the rest of the book. This is a very standard notation for the electric field, and many books on the subject will refer to it simply as the E field, or "E field" for short. We will also follow this convention throughout the book.
Electric Field Lines
[edit | edit source]To help us visualize the electric field, we use a graphical notation known as electric field lines. Electric field lines are lines that connect charges of opposite signs. As a matter of general convention, we say that lines come out of a positive charge, and go in to a negative charge, as such:

When drawing our field lines, we have a few general rules:
- Lines do not cross or overlap.
- A line must start at a positive charge, and end at a negative charge.
- Lines should curve evenly, and should not have any angles in them.
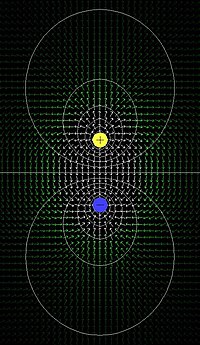
If we draw out all our lines, we will start to see our electric field.
Potential Function
[edit | edit source]For electrostatic fields, the curl is zero (as can be verified). This means that the force is conservative; there is potential energy function, such that the difference in potential energy of any two points is the amount of work needed to move charge from one point to another in any path.
Like electric fields, it would be desirable if we define the potential energy as a property of the field. This could no longer be the potential energy; clearly, the greater the charge, the more work needed to move it. However, we can define a potential that is the work per charge, that is an intrinsic property of the fields. The potential has units of joules per coulomb. This is used so commonly it is given its own name, the volt.
We set φ(r) to be the potential function for a position inside an electric field. We can define the potential function in terms of the E field:
[Potential Function]
Or, if we differentiate both sides, we get:
Often, it will be easier for us to find the potential function then to find the E field directly.
Using the Gauss' Law, we can relate the potential function of a field to the charge density of a point in the field as such:
We will discuss the potential function in more detail when we talk about electrical energy.






