Consciousness Studies/The Philosophical Problem

The philosophical problem of phenomenal consciousness

Chalmers (1996) encapsulated the philosophical problem of phenomenal consciousness, describing it as the Hard Problem. The Hard Problem can be concisely defined as "how to explain a state of consciousness in terms of its neurological basis" Block (2004). A state is an arrangement of things in space over a period of time. It is possible that the Hard Problem has not been solved because the concepts of "space", "time" and "things" are intensely problematic in both science and philosophy.

Some philosophers have argued that changes in state are equivalent to "mental states". That consciousness experience always involves acts, such as acts of acquaintance (Russell 1912). But what is a succession of states in the brain or the physical world?

As an extension of the idea of "acts" as mental states many philosophers have argued that the functional description of a system does not need to contain any reference to qualia within that system. Such ideas, based on nineteenth century materialism, have been expressed by Huxley, Ryle, Smart, Goldman and many others. However, although qualia are not required for classical functions, such as most computations or servo-control, it is far from clear whether this is true for all functions. If a function is described as any thing that mediates a change in state it should be realised that "change" itself is not fully understood in philosophy or science and that some systems, such as quantum mechanical systems, contain state changes that are far from understood. It will be seen below that our scientific knowledge is not yet sufficiently complete to allow the claim that all, or even any, changes can occur without qualia.

Whether a philosopher or scientist is dualist, materialist or physicalist they should have some insight into current theories about the physical world. Certainly, if they are considering the problem of "how to explain a state of consciousness in terms of its neurological basis" then some idea of a "neurological basis" is essential.

The objective of this section is to give an account of the problems of space, time and content and to describe how these affect the problem of consciousness.

Epiphenomenalism and the problem of change

Philosophers have noticed since the time of Leibniz that phenomenal consciousness does not seem to be required for the brain to produce action. As an example there are numerous reflexes that can occur without any awareness that they are happening. In fact it is difficult to think of any response to a stimulus that requires phenomenal consciousness and could not, in principle, be performed in the absence of conscious intervention. T.H. Huxley is often regarded as the originator of the term epiphenomenalism to describe how consciousness seems extraneous to processes in the materialist interpretation of the world although the term may have originated in James' description of Huxley's (1874) ideas.

According to nineteenth century science changes in state cannot explain the existence of phenomenal consciousness so superficially it may appear as if phenomenal consciousness is unnecessary. However, it may come as a shock to the reader to discover that nineteenth century science is also unable to account for any change in state. In the materialist paradigm time is construed to be a succession of instants of no duration, each of which is entirely separate from the others. As a result no instant can cause a change in another instant. It is not only conscious experience that is epiphenomenal, each instant of the nineteenth century concept of the world is epiphenomenal because it cannot give rise to the next instant.

On the one hand it seems that conscious experience is not required for a nineteenth century model of behaviour and on the other hand nineteenth century science seems to be impossible without extraneous input from a conscious observer who contains the idea of change.

The problem of change is closely related to the problem of time which is discussed in depth below. (See Change and Inconsistency, Stanford Encyclopedia of Philosophy).

The reader might consider whether phenomenal consciousness is indeed epiphenomenal. Empirical reports describe it as something that is different from the world beyond the body (see direct realism) - but could we generate empirical reports of an epiphenomenon? If we do indeed generate empirical reports of phenomenal consciousness is there some non-materialist, physical** connection between phenomenal consciousness and the functional state?

In the analysis that follows it is essential that the reader does not dismiss the possibility that conscious experience is largely non-functional in a classical sense. The idea that observation is not action should not be dismissed out of hand. Indeed the claim that something cannot be true if it is "epiphenomenal" in a classical sense is astonishing in the context of modern quantum physics. Everettian approaches (and offshoots like the Bohmian, Consistent Histories and operational (decoherence) approaches) to quantum physics all allow that the classical world is epiphenomenal (cf: Page 1997, Stapp 1998). The Copenhagen Interpretation, however, was less clear on this issue.

It is curious that problems with the nature of phenomenal consciousness are also problems with nineteenth century science - Aristotlean regress in the mind is part of the wider problem of epistemological regress and epiphenomenalism is part of the wider problem of change. Perhaps nineteenth century science is not an appropriate foundation for understanding consciousness.

Recommended reading:

Mortensen, C. (2002) Change. The Stanford Encyclopedia of Philosophy. http://plato.stanford.edu/entries/change/

Page, D.N. (1997). Sensible Quantum Mechanics: Are Only Perceptions Probabilistic? http://arxiv.org/abs/quant-ph/9506010

Rivas, T., & Dongen, H. van (2003). Exit Epiphenomenalism: The Demolition of a Refuge

Stapp, (1998). Quantum Ontology and Mind-Matter Synthesis.[Appeared in proceedings of X-th Max Born Symposium, eds, Blanchard and Jadczyk, Vol 517 Lecture notes in Physics series, Springer-Verlag, 1999 (Quantum Future:from Volta and Como to the present and beyond) ] http://arxiv.org/PS_cache/quant-ph/pdf/9905/9905053.pdf

(**) cf: gravity may affect the rate at which clocks tick without the occurrence of any collisions between particles or anything that can be called a "process".

The problem of time

This section should be read after reading a quick introduction to special relativity

The past century of ideas about time

McTaggart in 1908 set out some of the problems with our idea of time in his classic paper The Unreality of Time. He drew attention to the way that a sequence of things in a list does not describe time because a sequence of things is constant yet events are always changing. These considerations led him to propose that there are three different sequences of things, or series, that are commonly used to describe events. McTaggart's three different time series are summarized in the illustration below.

He argued that only the 'A Series' is a temporal series because it is only in the A Series that change occurs so that events can be given the labels 'future', 'present' and 'past'. He pointed out that although the A Series is used for determining the direction and sequence of events it is not itself 'in time' because it contains relations that are neither a part of the C Series nor the B Series. This led him to propose that time is unreal because change involves a movement along the time series so cannot be fixed within it.

Franck (1994) argued on the basis of Atmanspacher's models of universes with real and imaginary geometries that McTaggart's 'unreality' of time could be avoided by proposing a second, imaginary, time dimension.

"What McTaggart in fact demonstrates is that it is impossible to 
account for temporality within a strictly one-dimensional concept 
of time."(Franck 1994).

This idea is illustrated below:

This idea of time being two dimensional is not new and has also been advanced by such luminaries as Hermann Weyl and CD Broad. Weyl (1920) made the following statement that is extremely apposite to consciousness studies, he wrote that reality is a:

"...four-dimensional continuum which is neither 'time' nor 'space'. 
Only the consciousness that passes on in one portion of this world 
experiences the detached piece which comes to meet it and passes 
behind it, as history, that is, as a process that is going forward 
in time and takes place in space." (Weyl 1920).

McTaggart's objection to time is felt intuitively by anyone who has contemplated the Block Universe of Relativity Theory. If the universe is four dimensional with three space dimensions and one time dimension it would be fixed forever and the observer would be frozen within it. This would occur whether the time dimension was arranged according to Galilean Relativity or Modern Relativity.

Peter Lynds in 2003 has drawn attention to the 'frozen' nature of the observer in a four dimensional universe. He proposes, like Kevin Brown in his popular mathpages, that time must be approached from the viewpoint of quantum physics because simple four dimensional universes would give rise to 'frozen, static' instants and hence no change could occur. Lynds argues that if quantum physics is introduced then no event can have a definite moment of occurrence and that change occurs because of this quantum indeterminacy:

I would suggest that there is possibly much more to be gleaned from the connection between quantum physics and the inherent need for physical continuity, and even go as far to speculate that the dependent relationship may be the underlying explanation for quantum jumping and with static indivisible mathematical time values directly related to the process of quantum collapse. Time will tell."(Lynds 2003).

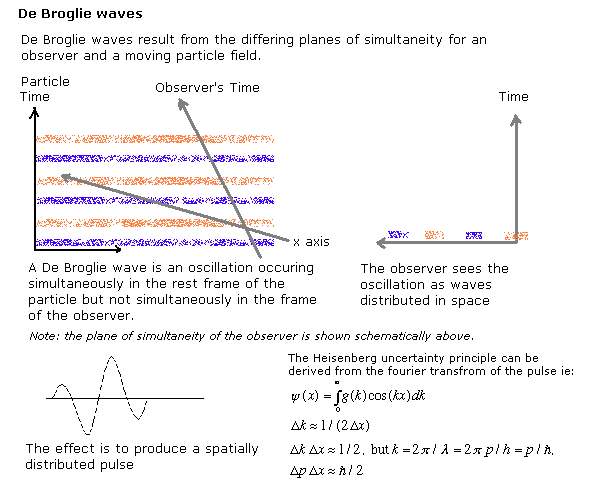
Our knowledge of quantum uncertainty can be traced back to De Broglie's highly successful model of individual particle motions. This model was based on Special Relativity theory and it predicted a wave nature for particles. The Heisenberg Uncertainty Principle can be shown to be a consequence of this wave nature. See the illustration below:

The illustration is based on de Broglie (1925) and Pollock (2004).

So Lynds' argument that change is due to the uncertainty principle is actually an argument that change is due to differing planes of simultaneity between systems that are in relative motion. Kevin Brown is aware of this; he summarises the effect of uncertainty due to special relativity and points out that it provides a resolution of Zeno's arrow paradox:

"The theory of special relativity answers Zeno's concern over the 
lack of an instantaneous difference between a moving and a non-moving 
arrow by positing a fundamental re-structuring the basic way in which 
space and time fit together, such that there really is an instantaneous 
difference between a moving and a non-moving object, insofar as it 
makes sense to speak of "an instant" of a physical system with mutually 
moving elements.  Objects in relative motion have different planes of 
simultaneity, with all the familiar relativistic consequences, so not 
only does a moving object look different to the world, but the world 
looks different to a moving object." (Brown 19??)

Another approach to the way that time has a direction is to suggest that the possible outcomes in quantum mechanics are located in "disjoint space-time regions which exclude one another" (McCall 2000). This does not explain the A Series however because the observer would not have any sense of 'becoming' or temporality as a result of the existence of regions that could not be observed.

Presentism and Four-Dimensionalism

In the past century the philosophical battle lines have been drawn between the Presentists, who believe that only the durationless instant of the present exists and the Four Dimensionalists who consider that things are extended in both space and time (see Rea (2004)). There are two types of Presentism, in its extreme form it is the belief that the past and future are truly non-existent, that what we call time is not an axis for arranging things but a series of changes and records in an enduring present. In its less extreme form, which might be called functional presentism, the present is a durationless instant that can never be connected to the future or past except through predictions and records.

In consciousness studies it is the conventional theory that brain activity occurs in the present instant and that the past can only occur as memories retrieved into this durationless present. So, in consciousness studies functional Presentism seems to be the accepted paradigm.

Presentism cannot explain change. Each instant is durationless and frozen. That said, as seen above, four dimensionalism cannot explain the observation of change although it can explain the difference between moving and stationary objects. Fortunately the debate has been largely resolved by recent scientific experiments which show that time exists and hence Presentism is unlikely.

The existence of time

The issue of whether or not time exists is critical to consciousness studies. If we exist at an instant without duration then how can we know we exist? Clay (1882) coined the term 'specious present' to describe how we seem to exist for a short period containing the immediate past:

"All the notes of a bar of a song seem to the listener to be contained in the present. All the changes of place of a meteor seem to the beholder to be contained in the present. At the instant of the termination of such series, no part of the time measured by them seems to be a past. Time, then, considered relatively to human apprehension, consists of four parts, viz., the obvious past, the specious present, the real present, and the future."

So conscious, phenomenal experience has things that are apparently extended in time. But does time exist?

Recent experiments in quantum physics should change our view of time forever. Lindner et al. (2005) have explored the problem of time by investigating quantum interference between interferometer slits that are separated by time rather than space.

In the famous, spatial 'double slit experiment' in quantum physics single electrons are directed at an apparatus that has the equivalent of two tiny slits separated by a small gap. The electrons pass through the apparatus one at a time and produce flashes of light on a screen or changes in a photographic plate. The electrons produce series of bands on the screen that are typical of interference effects. So each electron is deflected as if it has passed through both slits and interfered with itself.

This experiment provided some of the earliest evidence for the wave-packet nature of the electron.

In an amazing technical tour de force Lindner et al. (2005) have extended the idea of the spatial double slit experiment to an investigation of time. In the double slit experiment in time electrons are produced in an inert gas by extremely short laser pulses. The pulses stimulate a single atom and there is a probability of this atom releasing an electron at each oscillation of the pulse. The apparatus is described by Paulus et al. (2003). The probability (see note 1) of an electron being ejected to the left or right of the apparatus can be adjusted by adjusting the optical pulse. Pulses can be applied with a duration of a few femtoseconds and these create 'slits' extending over an interval of about 500 attoseconds (500 x 10-18 seconds). A single electron has a probability of being emitted at each of the slits. The probability of the single electron going in a particular direction after both slits have been created depends upon the interaction of the probabilities of being emitted in a particular direction at each single slit. As expected, an interference pattern was generated as a result of single electrons interfering with themselves across different times.

This experiment is remarkable because it provides direct evidence that time exists in a similar fashion to the way that space exists. It is consistent with Feynman's theory of Quantum Electrodynamics where all possible paths, both in time and space, interact to produce the final trajectory of a particle and consistent with modern Special Relativity, on which QED is based, where the trajectories of particles occur in an extended four dimensional space-time.

The experiment has not attracted as much attention as it might have done because most physicists are not Presentists. To physicists the experiment is yet another confirmation of modern physics. However it has impressed many:

"This experiment should be included in every textbook on quantum mechanics," says Wolfgang Schleich, a quantum physicist at the University of Ulm in Germany. "It certainly will be in mine." (PhysicsWeb)

Why should a concrete demonstration that time exists affect consciousness studies? The simple answer is that, as Kant, Gombrich, Clay, James and many others have spotted, there can be no conscious, phenomenal experience without time. The fact that time exists should provide new insights and liberate theorists in the field of consciousness studies from the problems of recursion and regression that are inherent in Presentism.

Meanwhile Quantum Theorists are pressing on with the problem of how an organised spacetime could emerge from quantum chaos (cf: Ambjorn et al. (2004)) and even how mind might be involved in the emergence of time itself (cf: Romer (2004)).

The nature of time

The nature of classical time

In the eighteenth century it became apparent that Euclid's parallel postulate could not be explained in terms of the other postulates. The parallel postulate is equivalent to the statement that exactly one line can be drawn through any point not on a given line in such a way that it is parallel to the given line (this is Playfair's simple version). It is also known as the fifth postulate.

The attempts to prove the parallel postulate led to the development of non-Euclidean geometry. It was then possible to show that the parallel postulate is a special case within a range of geometrical forms from spherical geometry, through Euclidean geometry to the hyperbolic geometry of Bolyai and Lobatschefsky. Furthermore it was shown by Taurinus that the axioms of Euclidean geometry, with the exception of the fifth postulate, applied on the surface of a sphere with an imaginary radius. This motivated Hermann Minkowski to propose that Einstein's new theory of relativity was in fact due to the universe being a 'space-time' with four dimensions rather than just a space in which things change (see Walter 1999). In 1909 Minkowski said that:

"Henceforth space by itself and time by itself, are doomed to fade 
away into mere shadows, and only a kind of union of the two will 
preserve an independent reality". (Minkowski 1909).

The earliest idea of the four dimensional universe involved time as an axis with displacements measured in units of the square root of minus one (cf: Einstein (1920)): time was considered to be displacements along the imaginary plane. However, from the moment of Minkowski's proposal mathematicians were aware that other interpretations of time could give almost identical physical results.

According to the differential geometry developed during the nineteenth century a space is defined in terms of a metric tensor which is a matrix of factors that determine how displacements in each independent direction vary with displacements in the other directions. The metric tensor then specifies a metric which is an equation that describes the length of a displacement in any direction in terms of the independent directions, or dimensions.

A derivation of the metric tensor and how it can be used to calculate the metric is given in the Appendix.

The metric of the space considered by Euclid is Pythagoras' theorem where the length of any displacement is given in terms of the displacements along the three independent axes, or dimensions:

$s^{2}=x^{2}+y^{2}+z^{2}$

It is interesting to explore imaginary time from the point of view of consciousness studies. Minkowski's original idea for the geometry of the world proposed that any displacement was a displacement in both time and space given by a four dimensional version of Pythagoras' theorem:

$s^{2}=x^{2}+y^{2}+z^{2}+(ict)^{2}$

which, given that $i^{2}=-1$ equals:

$s^{2}=x^{2}+y^{2}+z^{2}-(ct)^{2}$

Where i is the square root of minus one, c is a constant for converting metres to seconds and t is the displacement in time. The space-time is considered to be flat and all displacements are measured from the origin.

The interesting feature of Minkowski space-time with imaginary time is that displacements in time can subtract from displacements in space.

If we set $r^{2}=x^{2}+y^{2}+z^{2}$ (where r is the radius of a sphere around the origin then:

$s^{2}=r^{2}-(ct)^{2}$

Notice that $s^{2}=0$ when $r^{2}=(ct)^{2}$ so if imaginary time existed there would be times and separations within a spherical volume of things where everything is at a point as well as distributed in space. This idea has distinct similarites with the res cogitans mentioned by Descartes, and the point soul of Reid and Malebranche etc., however, this feature of Minkowski's space-time has not been popular with physicists for some good reasons. Blandford and Thorne point out some of the problems:

One approach, often used in elementary textbooks [and also used in Goldstein's (1980) Classical Mechanics and in the first edition of Jackson's Classical Electrodynamics], is to set $x^{0}=it$, where $i={\sqrt {-1}}$ and correspondingly make the time basis vector be imaginary,... When this approach is adopted, the resulting formalism does not care whether indices are placed up or down; one can place them wherever one's stomach or liver dictate without asking one's brain. However, this $x^{0}=it$ approach has severe disadvantages: (i) it hides the true physical geometry of Minkowski spacetime, (ii) it cannot be extended in any reasonable manner to non-orthonormal bases in flat spacetime, and (iii) it cannot be extended in any reasonable manner to the curvilinear coordinates that one must use in general relativity. For this reason, most advanced texts [including the second and third editions of Jackson (1999)] and all general relativity texts take an alternative approach, which we also adopt in this book. This alternative approach requires introducing two different types of components for vectors, and analogously for tensors: contravariant components denoted by superscripts, and covariant components denoted by subscripts." Blandford & Thorne (2004).

What Blandford and Thorne are saying is that the metric of space-time appears to be the result of the interaction of two coordinate systems and cannot be explained by a single coordinate system with imaginary time. When a more complicated geometrical analysis is applied it is evident that there are two possibilities for the time coordinate. In the first the metric can be assumed from the outset to be

$s^{2}=x^{2}+y^{2}+z^{2}-(ct)^{2}$

and the metric tensor simply adjusted by inserting -1 in the principle diagonal so that the negative sign in front of the time coordinate occurs. With this assumption and adjustment the time coordinate can be assumed to be real. In the second possibility the time coordinate in the world can be assumed to be imaginary and the time coordinate of the observer can be assumed to be real. This gives rise to the same metric tensor and metric as the first possibility but does not assume the resulting metric from the outset.

The three ideas of classical time (imaginary, real and mixed) are shown in the illustration below:

The light cone is divided into three regions: events on the surface of the light cone, such as photons converging on the observer, are said to be lightlike separated from the observer, events inside the future or past light cones are said to be timelike separated and events outside the lightcone are said to be spacelike separated from the observer.

The physical theory of relativity consists of four dimensional geometry plus the assumption of causality and the assumption that physical laws are invariant between observers. It should be noted that space-time could contain preferred frames of reference and is not, by itself, a theory of relativity. The assumption that physical laws are invariant between observers leads to the postulate that nothing can travel faster than c metres per second. This means that the constant c, which in Minkowski space-time is the conversion factor from seconds to metres then has a new significance as the maximum velocity.

A result of c being a maximum velocity is that nothing can travel from regions of the light cone that are spacelike separated to the observer at coordinates (0,0,0,0). This is problematic for observers if time is real because, as Stein (1968) wrote:

“in Einstein-Minkowski space-time an event's present is constituted by itself alone.” (Stein 1968).

However, to each of us it seems that the present is characterised by many things simultaneously. As will be discussed below, this simultaneity of present things also results in the appearance of phenomenal space. But in Minkowski space-time with real time the plane of simultaneity is entirely space-like separated from the observation point. If real time is accepted it would appear that we cannot have the space of phenomenal experience. The regions of the light-cone and the spacelike separation of present events are shown in the illustration below:

So can the time in Minkowski space-time be real? If time were in some way related to the imaginary plane then all the content of the surface of the light cone could be simultaneously at the position of the observer and phenomenal experience containing space is possible, but then general relativity may be problematic. So can the time in Minkowski space-time be imaginary?

There is another problem with Minkowski space-time known as the "Rietdijk-Putnam-Penrose" argument or the Andromeda paradox (Penrose 1989). Moving observers have different planes of simultaneity. The plane of simultaneity of an observer moving towards you slopes upward relative to your plane of simultaneity (see the illustration on "De Broglie waves" above). Suppose an alien civilisation in the Andromeda galaxy decided to launch a fleet of spacecraft intent on the invasion of earth just as you passed Jim in your car. Your plane of simultaneity would slope upwards ever so slightly compared with Jim's, Jim's plane of simultaneity could contain earlier events on Andromeda than yours. At the distance of the Andromeda galaxy it could be another week or two for the Andromedean's to launch their invasion fleet in Jim's slice of the universe. Penrose considers that this example shows that the events in the universe must be fixed:

"Two people pass each other on the street; and according to one of the two people, an Andromedean space fleet has already set off on its journey, while to the other, the decision as to whether or not the journey will actually take place has not yet been made. How can there still be some uncertainty as to the outcome of that decision? If to either person the decision has already been made, then surely there cannot be any uncertainty. The launching of the space fleet is an inevitability." (Penrose 1989).

If the decision to invade and a time previous to this decision are both part of the present instant on earth then, in a 4D classical universe, the decision to invade must be inevitable. This lack of free will in a 4D universe is known as chronogeometrical determinism (Toretti 1983). However, as de Broglie demonstrated, it is sloping planes of simultaneity that do indeed introduce uncertainty into our universe. It should also be noted that nothing on the plane of simultaneity is observable to the owner of that plane because, to observe it would involve the transmission of data at velocities greater than the speed of light.

Petkov (2002)considers a version of the Andromeda paradox in depth. He concludes that:

"If the relativity of simultaneity is explicitly discussed in terms of the dimensionality of reality, the fact that observers in relative motion have different sets of simultaneous events can be explained either by assuming that existence is also relativized (preserving the views of the present and objective becoming) or by considering existence absolute which means that reality is a 4D world. Although the option of relativizing existence appears completely unacceptable from a philosophical point of view, that option is eliminated within the framework of SR by demonstrating that the twin paradox would not be possible if existence were not absolute."

According to Petkov Special Relativity describes the universe as a frozen space-time where things are eternally arranged in four dimensions. Petkov introduces the possibility of change as a feature of consciousness and in support of this quotes Weyl's intuition that only the conscious observer moves in time.

Relationalism, Substantivalism, the Hole Argument and General Covariance

Relationalism and Substantivalism

The view that the universe could be an extended space and time with things in it, a sort of unbounded container, is known as substantivalism. It was championed by Newton and Clarke in the seventeenth century. The view that the space and time in the universe depends upon the relations between the objects in the universe is known as relationalism and was championed by Leibniz.

Leibniz attacked substantivalism by arguing that if there were two universes which only differed by things in one universe being displaced by five feet compared with things in the other universe then there is no reason why the two universes should be discernably different.

Newton supported substantivalism by arguing that when the water in a bucket rotates it adopts a concave surface that is independent of other motions and provides evidence of the possibility of absolute motion. This argument is called the bucket argument. Newton also introduces the globe argument in which he proposes that the state of motion of two globes connected by a taut thread can be gauged from the tension in the thread alone. When the globes are stationary with respect to each other there is no tension in the thread.

Ernst Mach in 1893 introduced a relationalist account of the bucket argument by claiming that the water rotates in relation to the fixed stars. He stated this in what has become known as Mach's principle:

"The inertia of any system is the result of the interaction of that system and the rest of the universe. In other words, every particle in the universe ultimately has an effect on every other particle."

The relationalist position is interesting from the viewpoint of consciousness studies because phenomenal consciousness appears as a projection that overlies physical space. As an example, the stars on the ceiling of a planetarium appear to be at huge distances from the observer even though they are reflected lights that are only a few metres away. In general a projection where positions depend upon angular separations will be subject to relationalism. It is also probable that the space of phenomenal consciousness is a continuum of some field in the brain, if this is the case then the way we conceive of space as an existent entity is actually a conception involving the angular relations between the perturbations of the substance that is the field. Substantivalism would then literally be space as a substance. It is intriguing in this respect that Kant believed that space was a form of intuition and hence a property of mind.

Kant raised another type of argument for the justification of absolute space. He asked whether handedness was due to relations or a property of space. The right and left hands are enantiomorphs (mirror images). The relations within the right and left hands are identical but they still differ, for instance a right hand cannot be moved on to a left hand so that it exactly overlies it. Kant proposed that handedness was property inherent in space itself rather than a set of relations.

Gardner introduced a version of Kant's problem with the "Ozma" argument: "Is there any way to communicate the meaning of the word "left" by a language transmitted in the form of pulsating signals? By the terms of the problem we may say anything we please to our listeners, ask them to perform any experiment whatever, with one proviso: there is to be no asymmetric object or structure that we and they can observe in common." (Gardner 1990).

Although it is probably impossible to provide an answer to the Ozma argument it is possible to relate handedness to a conceptual point observer who spans more than an instant of time. If a point observer is at the centre of a field of inward pointing space-time vectors then relative to any given vector there are positive and negative angular separations. The body is asymmetric and the point observer would lie within this so always have available a 'head' direction or a 'foot direction' and hence a left and right. Unlike the time extended observer an instantaneous observer would not contain vectors that contained directional information and would be no more than a collection of points in space.

Pooley (2002) discusses handedness in depth and introduces the problem of parity violation in the Weak Interaction.

General Covariance and the Hole Argument

The proposal that the universe is four dimensional does not in itself produce a full physical theory. The assumptions of causality and the invariance of physical laws between observers are also required to create modern Relativity Theory. The second assumption, that the laws of physics are the same for all observers is closely related to the requirement of general covariance.

The principle of general covariance requires that a manifold of events can be smoothly mapped to another manifold of the same dimension and back again. This mapping should always give the same result. General covariance is assumed in General Relativity.

Einstein realised that there was an apparent problem with this assumption in certain circumstances. In his hole argument he considers a special region of space-time that is devoid of matter and where the stress-energy tensor vanishes. He then labels the same events outside the hole with two different coordinate systems. These coordinate systems could differ by something as simple as having origins that are separate so the difference is entirely passive. Both systems will give the same values for the gravitational field outside the hole. It turns out however that that the systems predict different fields within the hole (see MacDonald (2001) for the calculation and Norton (1993), (1999) for a discussion). Einstein overcame this problem by considering active mappings where particles are actually transferred through the hole. He concluded that the points where particles meet can be transformed according to general covariance and hence a relativistic theory could indeed be constructed. Solutions to the field equations that were inconsistent with the points defined by interacting particles were discarded as non-physical.

The hole argument led Einstein to abandon the idea of space and time as something separate from the material content of the universe. The General Theory of Relativity becomes a theory of observables. He wrote that:

"That the requirement of general covariance, which takes away from space and time the last remnant of physical objectivity, is a natural one, will be seen from the following reflection. All our space-time verifications invariably amount to a determination of space-time coincidences. If, for example, events consisted merely in the motion of material points, then ultimately nothing would be observable but the meetings of two or more of these points. Moreover, the results of our measurings are nothing but verifications of such meetings of the material points of our measuring instruments with other material points, coincidences between the hands of the a clock and points on the clock dial, and observed point-events happening at the same place at the same time. The introduction of a system of reference serves no other purpose than to facilitate the description of the totality of such coincidences". (Einstein 1916).

This is what would be expected from a four dimensional block universe with real time. It is a frozen universe of the type discussed earlier. As Earman (2002) puts it when discussing change:

"First, the roots of the problem lie in classical GTR, and even if it was decided that it is a mistake to quantize GTR, there would remain the problem of reconciling the frozen dynamics of GTR with the B-series notion of change that is supported not only by common sense but by every physical theory prior to GTR. Second, although the aspect of the problem that grabs attention is that of time and change, no solution will be forthcoming without tackling the more general issue of what an “observable” of classical GTR is."

In such a universe action at a distance is not possible. From the viewpoint of consciousness studies the limitation of physical concepts to interactions between particles is a restatement of Ryle's regress and the recursion version of the homunculus problem. If events are no more than space-time coincidences then we are doomed to the endless transfer of data from point to point without any conscious observation. This seems to forbid any true simultaneity in experience and means that only measurements are possible.

The reduction of physics to the study of particle interactions is fully relationalist and allows space-time to become a property of these interactions rather than vice-versa. Once it becomes possible to consider space-time as a dependent property it is then feasible to equate observation with measurement. Observation is normally the representation of an event in an observer's space-time coordinate system. Measurement is the change in state of a system in response to an encounter with an event. If we maintain that space-time does not exist and can be replaced by encounters between particles then observation can be replaced by measurement. This may well be a way forward for some approximations to physical reality and may allow us to understand how a space-time is selected within an observer. As part of this approach the word "observable" is often used interchangeably with "measurable".

Quantum theory and time

The general problem of QM and time

Quantum physics provides many fundamental insights into the nature of time. At the simplest level the energy-time version of the Heisenberg Uncertainty Principle predicts that Quantum Mechanical (QM) interference should occur between a particle and earlier versions of itself. Such interference has been observed (see "The existence of time" above).

Two of the most complete reviews of the problem of time in quantum theory available at present are Zeh (2001) and Isham (1993).

Perhaps the most interesting aspect of QM and time is that it can provide an argument that time does not exist in the universe as a whole. The argument can be approached from many directions (See Rovelli 2003) but is clear in the Wheeler-de Witt equation which describes the wavefunction of the entire universe. This wavefunction has no reference to time. De Witt explained the emergence of time by proposing that the universe can be divided into an observer with measuring instruments and the rest of the universe so that the rest of the universe changes with respect to the observer.

Rovelli (2003) supports this idea of partition, he considers in depth the problems of the "hole argument" and quantum physics and notes that, given the assumption that events are just successions of relations:

"The unique account of the state of the world of the classical theory is thus shattered into a multiplicity of accounts, one for each possible "observing" physical system. Quantum mechanics is a theory about the physical description of physical systems relative to other systems, and this is a complete description of the world. (Rovelli 2003).

Barbour (1997) and Hartle and Gell-Mann have both proposed that an observer is a partition or region with memories that contain the trace of histories. The histories would represent a B Series. Unfortunately this leaves the A Series unexplained so time would have a direction but there would be no 'becoming'.

Hawking introduces the observer into the problem of time by asking what sort of universe is compatible with human life. This application of the Anthropic Principle leads to constraints on the form of the universe, for instance the universe should have galaxies and last for more than a few million years. The Anthropic Principle is actually a restatement of the observer problem - if being an observer leads to a certain division of the universe into observer and observed then the observed part will have the form given by the Anthropic Principle. Hartle and Hawking () also tackled the "boundary problem" of cosmology by proposing that there is no boundary. This proposal involves adding a fifth, time-like, dimension on the imaginary plane so that the universe at its beginning is a de Sitter or anti de Sitter space-time.

A de Sitter space-time is characterised by the metric:

$ds^{2}=dx^{2}+dy^{2}+dz^{2}+(idt)^{2}+du^{2}$

An anti de Sitter space time has the metric:

$ds^{2}=dx^{2}+dy^{2}+dz^{2}+(idt)^{2}-du^{2}$

A de Sitter space time is fascinating from the view point of consciousness studies because it contains three space-like dimensions, one real, time-like dimension (u) and one imaginary time-like dimension. This might give the real and imaginary time-like axes that Franck proposed were needed to produce the McTaggart A Series. However, the extra dimension could only be related to the observer in the universe as it is at present because the extra dimension does not appear to be required to explain measurables.

The interpretation of QM

Time is also of interest in the interpretation of quantum mechanics and entanglement. There are many interpretations of QM such as the Operational Interpretation (Decoherence Theory), the Transactional Interpretation, the Relational Interpretation, the Many Worlds Interpretation, the Copenhagen Interpretation, the Bohm Interpretation, the Many Minds Interpretation etc.

Some of these interpretations, such as the Transactional Interpretation, allow the connection of entangled quantum states backwards in time along the path of particles.

Decoherence theory is of particular interest because it allows the calculation of how long an entangled state can persist. Tegmark (2000) and Hagan et al. (2002) have used this technique to calculate the decoherence time of entanglement in microtubules and have differed by a factor of $10^{10}$ because of differing assumptions about the biophysics of microtubules in the brain.

Time and conscious experience

In a four dimensional universe time is an independent direction for arranging things. As an independent direction things arranged in time do not overlie things arranged in space. This also appears to be the case in conscious experience where whole words or "bars of a tune" can be experienced arranged in time. This extension in time is easy to experience but the independence of the time dimension is difficult to conceive, for instance Le Poidevin (2000) reflects that:

"If events e1 and e2 are registered in a single specious present, then we perceive them both as present, and so as simultaneous. But we do not see, e.g., the successive positions of a moving object as simultaneous, for if we did we would see a blurred object and not a moving one."

This assumes that arrangements in time do not occur in an independent direction for arranging things and hence would overlay space. In fact the mystery of conscious experience is deeply related to how we can experience many things as events that are separate from each other. Our experience of two dimensional patterns containing many things is as much a mystery as how we experience temporal patterns extended in time. The problem is illustrated below:

It is as if patterns in conscious experience are being viewed from a point in at least four dimensions. How our experience can be like the 'view' of a conceptual point observer at the apex of a light cone without the data being overlaid and obscured is a profound mystery, obviously the data cannot be transferred into the apparent observation point and appears as nebulous vectors directed at the point. Some philosophers have noticed this problem.

(This is a stub, requires an elaboration of Specious Present Theory and Husserl's ideas)

Le Poidevin (2000). The experience and perception of time. Stanford Encyclopedia of Philosophy. http://plato.stanford.edu//archives/spr2001/entries/time-experience/#4

Readers who are unfamiliar with the developments to Newtonian mechanics that occurred in the eighteenth and nineteenth centuries should read Consciousness Studies/The Philosophical Problem/Appendixs

See overleaf for the philosophical problem continued..:

The problems of space, qualia, machine and digital consciousness

Click on the above link.

Notes and References

More...