Abstract Algebra/Binary Operations

A binary operation on a set $A$ is a function $*:A\times A\rightarrow A$. For $a,b\in A$, we usually write $*(a,b)$ as $a*b$.

Properties

The property that $a*b\in A$ for all $a,b\in A$ is called closure under $*$.

Example: Addition between two integers produces an integer result. Therefore addition is a binary operation on the integers. Whereas division of integers is an example of an operation that is not a binary operation. $1/2$ is not an integer, so the integers are not closed under division.

To indicate that a set $A$ has a binary operation $*$ defined on it, we can compactly write $(A,*)$. Such a pair of a set and a binary operation on that set is collectively called a binary structure. A binary structure may have several interesting properties. The main ones we will be interested in are outlined below.

Definition: A binary operation $*$ on $A$ is associative if for all $a,b,c\in A$, $(a*b)*c=a*(b*c)$.

Example: Addition of integers is associative: $(1+2)+3=6=1+(2+3)$. Notice however, that subtraction is not associative. Indeed, $2=1-(2-3)\neq (1-2)-3=-4$.

Definition: A binary operation $*$ on $A$ is commutative is for all $a,b\in A$, $a*b=b*a$.

Example: Multiplication of rational numbers is commutative: ${\frac {a}{b}}\cdot {\frac {c}{d}}={\frac {ac}{bd}}={\frac {ca}{bd}}={\frac {c}{d}}\cdot {\frac {a}{b}}$. Notice that division is not commutative: $2\div 3={\frac {2}{3}}$ while $3\div 2={\frac {3}{2}}$. Notice also that commutativity of multiplication depends on the fact that multiplication of integers is commutative as well.

Exercise

• Of the four arithmetic operations, addition, subtraction, multiplication, and division, which are associative? commutative and identity?

Answer

operation	associative	commutative
Addition	yes	yes
Multiplication	yes	yes
Subtraction	No	No
Division	No	No

Algebraic structures

A structure with one binary operation may be one of several types, depending on the conditions satisfied by the binary operation.

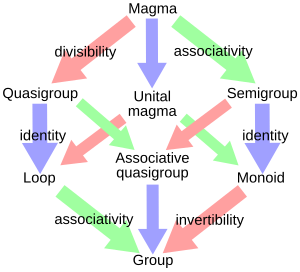
Binary operations are the working parts of algebraic structures:

One binary operation

A closed binary operation o on a set A is called a magma (A, o ).

If the binary operation respects the associative law a o (b o c) = (a o b) o c, then the magma (A, o ) is a semigroup.

If a magma has an element e satisfying e o x = x = x o e for every x in it, then it is a unital magma. The element e is called the identity with respect to o. If a unital magma has elements x and y such that x o y = e, then x and y are inverses with respect to each other.

A magma for which every equation a x = b has a solution x, and every equation y c = d has a solution y, is a quasigroup. A unital quasigroup is a loop.

A unital semigroup is called a monoid. A monoid for which every element has an inverse is a group. A group for which x o y = y o x for all its elements x and y is called a commutative group. Alternatively, it is called an abelian group.

Two binary operations

A pair of structures, each with one operation, can used to build those with two: Take (A, o ) as a commutative group with identity e. Let A_ denote A with e removed, and suppose (A_ , * ) is a monoid with binary operation * that distributes over o:

a * (b o c) = (a * b) o (a * c). Then (A, o, * ) is a ring.

In this construction of rings, when the monoid (A_ , * ) is a group, then (A, o, * ) is a division ring or skew field. And when (A_ , * ) is a commutative group, then (A, o, * ) is a field.

The two operations sup (v) and inf (^) are presumed commutative and associative. In addition, the absorption property requires: a ^ (a v b) = a, and a v (a ^ b) = a. Then (A, v, ^ ) is called a lattice.

In a lattice, the modular identity is (a ^ b) v (x ^ b) = ((a ^ b) v x ) ^ b. A lattice satisfying the modular identity is a modular lattice.

Three binary operations

A module is a combination of a ring and a commutative group (A, B), together with a binary function A x B → B. When A is a field, then the module is a vector space. In that case A consists of scalars and B of vectors. The binary operation on B is termed addition.

Four binary operations

Suppose (A, B) is a vector space, and that B has a second operation called multiplication. Then the structure is an algebra over the field A.